Skip to main content
SpringerLink
Log in

Stochastic Optimal Control of Finite Ensembles of Nanomagnets

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

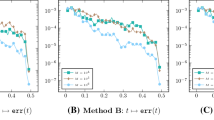
Abstract

We control ferromagnetic N-spin dynamics in the presence of thermal fluctuations by minimizing a quadratic functional subject to the stochastic Landau–Lifshitz–Gilbert equation. Existence of a weak solution of the stochastic optimal control problem is shown. The related first order optimality conditions consist of a coupled forward–backward SDE system, which is numerically solved by a structure-inheriting discretization, the least squares Monte-Carlo method to approximate related conditional expectations, and the new stochastic gradient method. Computational experiments are reported which motivate optimal controls in the case of interacting anisotropy, stray field, exchange energies, and acting noise.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

References

  1. Alouges, F., Beauchard, K.: Magnetization switching on small ferromagnetic ellipsoidal samples. ESAIM Control Optim. Calc. Var. 15(3), 676–711 (2009). doi:10.1051/cocv:2008047

    Article  MathSciNet  MATH  Google Scholar 

  2. Agarwal, S., Carbou, G., Labbe, S., Prieur, C.: Control of a network of magnetic ellipsoidal samples. Math. Control Relat. Fields 1(2), 129–147 (2011). doi:10.3934/mcrf.2011.1.129

    Article  MathSciNet  MATH  Google Scholar 

  3. Baňas, Ľ., Brzeźniak, Z., Neklyudov, M., Prohl, A.: Stochastic Ferromagnetism: Analysis and Computation, vol. 58, De Gruyter Studies in Mathematics (2013)

  4. Bertotti, G., Mayergoyz, I., Serpico, C.: Nonlinear Magnetization Dynamis in Nanosystems, Elsevier Series in Electromagnetism. Elsevier, London (2009)

    MATH  Google Scholar 

  5. Bender, C., Zhang, J.: Time Discretization and Markovian iteration for coupled FBSDEs. Ann. Appl. Probab. 18(1), 143–177 (2008). doi:10.1214/07-AAP448

    Article  MathSciNet  MATH  Google Scholar 

  6. Dunst, T., Klein, M., Prohl, A., Schäfer, A.: Optimal control in evolutionary micromagnetism. IMA J. Numer. Anal. 35(3), 1342–1380 (2015). doi:10.1093/imanum/dru034

    Article  MathSciNet  MATH  Google Scholar 

  7. Dunst, T., Prohl, A.: The forward-backward stochastic heat equation: numerical analysis and simulation. SIAM J. Sci. Comput. 38(5), A2725–A2755 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  8. El Karoui, N., Huang, S.-J.: A general result of existence and uniqueness of backward stochastic differential equations. In: El Karoui, N., et al. (eds.) Backward Stochastic Differential Equations, pp. 27–36. Chapman and Hall/CRC, Boca Raton (1997)

    Google Scholar 

  9. Friedman, J.R., Sarachik, M.P.: Single-molecule nanomagnets. Annu. Rev. Condens. Matter Phys. 1, 109–128 (2010). doi:10.1146/annurev-conmatphys-070909-104053

    Article  Google Scholar 

  10. Fahim, A., Touzi, N., Warin, X.: A probabilistic numerical method for fully nonlinear parabolic PDEs. Ann. Appl. Probab. 21(4), 1322–1364 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  11. Gobet, E., Lemor, J., Warin, X.: A regression-based Monte Carlo method to solve backward stochastic differential equations. Ann. Appl. Probab. 15(3), 2172–2202 (2005). doi:10.1214/105051605000000412

    Article  MathSciNet  MATH  Google Scholar 

  12. Mentink, J.H., Tretyakov, M.V., Fasolino, A., Katsnelson, M.I., Rasing, T.: Stable and fast semi-implicit integration of the stochastic Landau-Lifshitz equation. J. Phys.: Condens. Matter (2010). doi:10.1088/0953-8984/22/17/176001

    Google Scholar 

  13. Neklyudov, M., Prohl, A.: The role of noise in finite ensembles of nanomagnetic particles. Arch. Ration. Mech. Anal. 210(2), 499–534 (2013). doi:10.1007/s00205-013-0654-4

    Article  MathSciNet  MATH  Google Scholar 

  14. Yong, J., Zhou, X.: Stochastic Controls. Hamiltonian Systems and HJB Equations, 2nd edn. Springer, Berlin (1999)

    MATH  Google Scholar 

  15. Zhang, J.: A numerical scheme for BSDEs. Ann. Appl. Probab. 14, 459–488 (2004). doi:10.1214/aoap/1075828058

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Mathematisches Institut der Universität Tübingen, Auf der Morgenstelle 10, 72076, Tübingen, Germany

    Thomas Dunst & Andreas Prohl

Authors
  1. Thomas Dunst
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Andreas Prohl
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Andreas Prohl.

Additional information

The authors are grateful to helpful discussions with U. Nowak (Universität Konstanz). This work was performed on the computational resource bwUniCluster funded by the Ministry of Science, Research and the Arts Baden-Württemberg and the Universities of the State of Baden-Württemberg, Germany, within the framework program bwHPC.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Dunst, T., Prohl, A. Stochastic Optimal Control of Finite Ensembles of Nanomagnets. J Sci Comput 74, 872–894 (2018). https://doi.org/10.1007/s10915-017-0474-z

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-017-0474-z

Keywords

Search

Navigation