No Heading
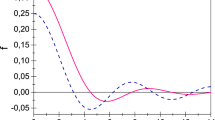
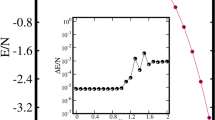
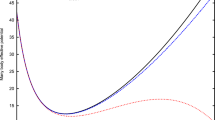
We investigate the possibility that the BEC-like phenomena recently detected on two-dimensional finite trapped systems consist of fragmented condensates. We derive and diagonalize the one-body density matrix of a two-dimensional isotropically trapped Bose gas at finite temperature. For the ideal gas, the procedure reproduces the exact harmonic-oscillator eigenfunctions and the Bose distribution. We use a new collocation-minimization method to study the interacting gas in the Hartree-Fock approximation and obtain a ground-state wavefunction and condensate fraction consistent with those obtained by other methods. The populations of the next few eigenstates increase at the expense of the ground state but continue to be negligible; this supports the conclusion that two-dimensional BEC is into a single state.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
1. W. J. Mullin, J. Low Temp. Phys. 106, 615 (1997).
2. A. Görlitz et al., Phys. Rev. Lett. 87, 130402 (2001); D. Rychtarik, B. Engeser, H.-C. Nägerl, and R. Grimm, Phys. Rev. Lett. 92, 173003 (2004).
3. S. D. Heinrichs and W. J. Mullin, J. Low Temp. Phys. 113, 231 (1998); ibid. 114, 571 (1999).
4. W. J. Mullin, M. Holzmann, and F. Laloë, J. Low Temp. Phys. 121, 263 (2000).
5. P. Nozières, in Bose-Einstein Condensation, edited by A. Griffin, D. W. Snoke, and S. Stringari (Cambridge University Press, Cambridge, 1993), p. 15.
6. O. Penrose and L. Onsager, Phys. Rev. 104, 576 (1956).
7. C. Gies, B. P. van Zyl, S. A. Morgan, and D. A. W. Hutchinson, Phys. Rev. A 69, 023616 (2004); L. Pricoupenko, Phys. Rev. A 70, 013601 (2004).
8. M. Holzmann and Y. Castin, Eur. Phys. J. D 7, 425 (1999).
9. W. Krauth, Phys. Rev. Lett. 77, 3695 (1996).
10. W. H. Press, S. A. Teukolsky, W. A. Vetterling, and B. P. Flannery, Numerical Recipes in C (Cambridge University Press, Cambridge, 1992), 2nd ed.
11. J. A. C. Weideman and S. C. Reddy, ACM Trans. of Math. Software 26, 465 (2000).
Author information
Authors and Affiliations
Additional information
PACS numbers: 03.75.Hh, 05.30.Jp, 05.70 Fh, 32.80.Pj
Rights and permissions
About this article
Cite this article
Fernández, J., Mullin, W. Absence of Fragmentation in Two-Dimensional Bose-Einstein Condensation. J Low Temp Phys 138, 687–692 (2005). https://doi.org/10.1007/s10909-005-2287-y
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10909-005-2287-y