Abstract
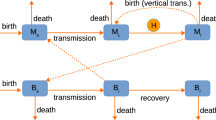
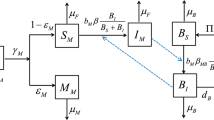
West Nile virus (WNv) transmission shows both seasonal pattern in every single year and cyclic pattern over years. In this paper we formulate a compartmental model with bird demographics and maturation time of mosquitoes during metamorphosis to study the impact of ambient temperature on the transmission and recurrence of disease. We show that avian birds serve as a reservoir of viruses, whilst maturation time affects disease transmission in sophisticated ways. It turns out that large maturation delay will lead to the extinction of mosquitoes and the disease; small maturation delay will stabilize the epidemic level of the disease; and intermediate maturation delay will cause sustainable oscillations of mosquito population, recurrence of diseases, and even mixed-mode oscillation of diseases with an alternation between oscillations of distinct large and small amplitudes. With bifurcation theory, we prove that temperature can drive the oscillation of mosquito population, which leads recurrence of WNv through the incidence interaction between mosquitoes and hosts, while the biting and transmission process itself will not generate sustained oscillations. Our results provide a sound explanation for understanding interactions between vectors and hosts, and driving mechanisms of periodic phenomena in the transmission of WNv and other mosquito-borne diseases.








Similar content being viewed by others
References
Abdelrazec, A., Lenhart, S., Zhu, H.: Transmission dynamics of west nile virus in mosquitoes and corvids and non-corvids. J. Math. Biol. 68, 1553–1582 (2014)
Arino, J., Wang, L., Wolkowicz, G.S.: An alternative formulation for a delayed logistic equation. J. Theor. Biol. 241, 109–119 (2005)
Berezansky, L., Braverman, E., Idels, L.: Nicholson’s blowflies differential equations revisited: main results and open problems. Appl. Math. Model. 34, 1405–1417 (2010)
Bowman, C., Gumel, A.B., van Den Driessche, P., Wu, J., Zhu, H.: A mathematical model for assessing control strategies against West Nile virus. Bull. Math. Biol. 67, 1107–1133 (2005)
Brust, R.: Weight and develpoment time of different stadia of mosquitoes reared at various constant temperature. Can. Entomol. 99, 986–993 (1967)
Campbell, L.G., Martin, A.A., Lanciotti, R.S., Guble, D.J.: West Nile virus. Lancet Infect. Dis. 2, 519–529 (2002)
Centers for disease control and prevention, 2002. West Nile virus: virus history and distribution. http://www.cdc.gov/ncidod/dvbid/westnile/background.htm (2002)
Centers for disease control and prevention, 2012. West Nile virus update: November 6, http://www.cdc.gov/ncidod/dvbid/westnile/index.htm (2002)
Cooke, K.L., van den Driessche, P., Zou, X.: Interaction of maturation delay and nonlinear birth in population and epidemic models. J. Math. Biol. 39, 332–352 (1999)
Cruz-Pacheco, G., Esteva, L., Montano-Hirose, J.A., Vargas, C.: Modelling the dynamics of West Nile virus. J. Math. Biol. 67, 1157–1172 (2005)
Cruz-Pacheco, G., Esteva, L., Vargas, C.: Seasonality and outbreaks in West Nile virus infection. Bull. Math. Biol. 71, 1378–1393 (2009)
Dohm, D.J., Sardelis, M.R., Turell, M.J.: Experimental vertical transmission of West Nile virus by Culex pipiens (Diptera: Culicidae). J. Med. Entomol. 39, 640–644 (2002)
Fan, G., Liu, J., van den Driessche, P., Wu, J., Zhu, H.: A delay differential equations model for the impact of temperature on West Nile virus between birds and mosquitoes. Math. Biosci. 228(2), 119–126 (2010)
Garett-Jones, C.: Prognosis for interruption of malaria transmission through assessment of mosquitoes vectorical capacity. Nature 204, 1173–1175 (1964)
Goddard, L.B., Roth, A.E., Reisen, W.K., Scott, T.W.: Vertical transmission of West Nile virus by three California Culex (Diptera: Culicidae) species. J. Med. Entomol. 40, 743–746 (2003)
Gurney, W., Blythe, S., Nisbet, R.: Nicholson’s blowflies revisited. Nature 287(4), 17–21 (1980)
Hagstrum, D.W., Workman, E.B.: Interaction of temperature and feeding rate in determining the rate of development of larval Culex tarsalis. Ann. Entomol. Soc. Am. 64, 668–671 (1971)
Hale, J.K., Lunel, S.M.: Introduction to Functional Differential Equations. Applied Mathematical Sciences, 99. Springer, New York (1993)
Komar, N., Langevin, S., Nemeth, N., Edwards, E., Hettler, D., Davis, B., Bowen, R., Bunning, M.: Experimental infection of North American bird with the New York 1999 strain of West Nile virus. Emerg. Infect. Dis. 9(2003), 311–322 (1999)
Laperriere, V., Brugger, K., Rubel, F.: Simulation of the seasonal cycles of bird, equine and human West Nile virus cases. Prev. Vet. Med. 98, 99–110 (2011)
Lassiter, M., Apperson, C., Roe, R.: Juvenile hormone metabolism during the fourth stadium and pupal stage of the south house mosquito Culex quinquefasciatus say. J. Insect Physiol. 41, 869–876 (1995)
Lewis, M., Renclawowicz, J., van den Driessche, P.: Travelling waves and spread rates for a West Nile virus model. Bull. Math. Biol. 68, 3–23 (2006)
Lord, C.C., Day, J.F.: Simulation studies of St. Louis encephalitis virus in south Florida. Vector Borne Zoonotic Dis. 1(4), 299–315 (2001)
Maidana, N.A., Yang, H.M.: Spatial spreading of west nile virus described by traveling waves. J. Theor. Biol. 258, 403–417 (2009)
Munga, S., Minakawa, N., Zhou, G.: Survivorship of immature stages of Anopheles gambiae s.l. (Diptera: Culicidae) in natural habitats in western kenya highlands. J. Med. Entomol. 44(5), 758–764 (2007)
Sander, E., Yorke, J.: Connecting period-doubling cascades to chaos. Int. J. Bifurc. Chaos Appl. Sci. Eng. 22, 1250022 (2012)
Shu, H., Wang, L., Wu, J.: Global dynamics of nicholson’s blowflies equation revisited: onset and termination of nonlinear oscillations. J. Differ. Equ. 255, 2565–2586 (2013)
Simpson, J.E., Hurtado, P.J., Medlock, J., Molaei, G., Andreadis, T.G., Galvani, A.P., Diuk-Wasser, M.A.: Vector host-feeding preference drive transmission of multi-host pathogens: West Nile virus as a model system. Proc. R. Soc. B 279, 924–933 (2012)
Smith, H.J.: Monotone Dynamical Systems: An Introduction to the Theory of Competitive and Cooperative Systems. Mathematical Surveys and Monographs, vol. 41. American Mathematical Society, Providence, RI (1995)
Thomas, D., Weedermann, M., Billings, L., Hoffacker, J., Washington -Allen, R.A.: When to spray: a time-scale calculus approach to controlling the impact of West Nile virus. Ecol. Soc. 14(2) (2009)
Tuno, N., Okeka, W., Minakawa, N., Takagi, M.: Survivorship of Anopheles gambiae sensu stricto (Diptera: Culicidae) larvae in western kenya highland forest. J. Med. Entomol. 42(3), 270–277 (2005)
van den Driessche, P., Watmough, J.: Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math. Biosci. 180, 29–48 (2002)
Wan, H., Zhu, H.: A new model with delay for mosquito population dynamics. Math. Biosci. Eng. 11(6), 1395–1410 (2014)
Wang, J., Ogden, N.H., Zhu, H.: The impact of weather conditions on Culex pipiens and Culex restuans (Diptera: Culicidae) abundance: a case study in Peel region. J. Med. Entomol. 48(2), 468–475 (2011)
Wei, J., Li, M.Y.: Hopf bifurcation analysis in a delayed nicholson blowflies equation. Nonlinear Anal. 60, 1351–1367 (2005)
Wolkowicz, G.S.K., Xia, H.: Global asymptotic behavior of a chemostat model with discrete delays. SIAM J. Appl. Math. 57, 1019–1043 (1997)
Wonham, M .J., de Camino Beck, T., Lewis, M .A.: An epidemiological model for West Nile virus: invasion analysis and control applications. Proc. R. Soc. Lond. Ser. B 271, 501–507 (2004)
Wonham, M.J., Lewis, M.A., Renclawowicz, J., van den Driessche, P.: Transmission asumptions generate confflicting prediction in host-vector disease models: a case study in West Nile virus. Ecol. Lett. 9, 706–725 (2006)
Acknowledgements
Funding was provided by Natural Sciences and Engineering Research Council of Canada (CA), Canadian Institute of Health Research (CIHR) (CA).
Author information
Authors and Affiliations
Corresponding author
Additional information
Dedicated to Professor John (Joseph Douglas) Mallet-Paret in celebration of his 60th birthday.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Shan was partially supported by the startup fund 110799 from the University of Toledo and the Simons Foundation-Collaboration Grants for Mathematicians 523360. Fan was partially supported by 2018 Summer Research Incentive Grant from the Dean of College of Letters and Sciences at Columbus State University. Zhu was partially supported by NSERC and CIHR of Canada.
Rights and permissions
About this article
Cite this article
Shan, C., Fan, G. & Zhu, H. Periodic Phenomena and Driving Mechanisms in Transmission of West Nile Virus with Maturation Time. J Dyn Diff Equat 32, 1003–1026 (2020). https://doi.org/10.1007/s10884-019-09758-x
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10884-019-09758-x