Skip to main content
SpringerLink
Log in

The Parametrically Forced Pendulum: A Case Study in 1 1/2 Degree of Freedom

  • Published:
Journal of Dynamics and Differential Equations Aims and scope Submit manuscript

Abstract

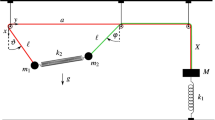
This paper is concerned with the global coherent (i.e., non-chaotic) dynamics of the parametrically forced pendulum. The system is studied in a === degree of freedom Hamiltonian setting with two parameters, where a spatio-temporal symmetry is taken into account. Our explorations are restricted to large regions of coherent dynamics in phase space and parameter plane. At any given parameter point we restrict to a bounded subset of phase space, using KAM theory to exclude an infinitely large region with rather trivial dynamics. In the absence of forcing the system is integrable. Analytical and numerical methods are used to study the dynamics in a parameter region away from integrability, where the analytic results of a perturbation analysis of the nearly integrable case are used as a starting point. We organize the dynamics by dividing the parameter plane in fundamental domains, guided by the linearized system at the upper and lower equilibria. Away from integrability some features of the nearly integrable coherent dynamics persist, while new bifurcations arise. On the other hand, the chaotic region increases.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Arnol’d V.I., Avez A. (1968). Ergodic Problems of Classical Mechanics, Mathematical Physics Monograph Series, W.A., Benjamin.

  • D. Acheson (1995) ArticleTitleMultiple-nodding oscillations of a driven inverted pendulum Proc. Royal Soc. London A. 448 89–95

    Google Scholar 

  • V.I. Arnol’d (1962) ArticleTitleOn the behavior of an adiabatic invariant under slow periodic variation of the Hamiltonian Soviet Math. Dokl. 3 136–140

    Google Scholar 

  • V.I. Arnol’d (1963) ArticleTitleProof of a theorem of A.N. Kolmogorov on the preservation of conditionally periodic motions under a small perturbation of the Hamiltonian (in Russian) Russian Math. Surv. 18 IssueID5 9–36

    Google Scholar 

  • Arnol’d V.I. (1988). Geometrical Methods in the Theory of Ordinary Differential Equations, 2nd edn. Springer-Verlag.

  • Arnol’d, V.I. (1989). Mathematical Methods of Classical Mechanics, 2nd edn., volume 60 of Graduate Texts in Mathematics, Springer-Verlag.

  • Arnol’d, V.I. (1993). Dynamical Systems. III, Springer-Verlag. Mathematical aspects of classical and celestial mechanics, Translation of Current Problems in Mathematics. Fundamental Directions, Vol. 3 (Russian), Akad. Nauk SSSR, Vessoyuz.Inst. Nauchn. i Tekhn. Inform., Moscow, 1985, Translation by A. Iacob.

  • Arnol’d, V.I. (1994). Dynamical Systems. V, Springer-Verlag, 1994. Bifurcation theory and catastrophe theory, Translation of Current problems in mathematics. Fundamental directions. Vol. 5 (Russian), Akad. Nauk SSSR, Vsesoyuz. Inst. Nauchn. i Tekhn. Inform., Moscow, 1986, Translation by N.D. Kazarinoff.

  • Benettin G. (1988). Nekhoroshev-like results for Hamiltonian dynamical systems. In Nonlinear Evolution and Chaotic Phenomena (Noto, (1987), Plenum, New York, 121–146.

  • A. Back J. Guckenheimer M.R. Myers F.J. Wicklin P.A. Worfolk (1992) ArticleTitleDstool: Computer assisted exploration of dynamical systems Notices Am. Math. Soc. 39 303–309

    Google Scholar 

  • H.W. Broer I. Hoveijn G.A. Lunter G. Vegter (1998) ArticleTitleResonances in a spring-pendulum: algorithms for equivariant singularity theory Nonlinearity. 11 IssueID16 1569–1605

    Google Scholar 

  • Broer H.W., Hoveijn I., and van Noort M. (1998). A reversible bifurcation analysis of the inverted pendulum. Phys. D 112(1–2): 50–63. Time-reversal symmetry in dynamical systems (Warwick, 1996).

    Google Scholar 

  • H.W. Broer I. Hoveijn M. Noort Particlevan G. Vegter (1999) ArticleTitleThe inverted pendulum: a singularity theory approach J. Diff. Eq. 157 120–149

    Google Scholar 

  • Broer H.W., Huitema G.B., Sevryuk M.B. (1996). Quasi-Periodic Motions in Families of Dynamical Systems. Order Amidst Chaos, vol. 1645 of Lecture Notes in Mathematics, Springer-Verlag.

  • H.W. Broer G.B. Huitema F. Takens (1990) ArticleTitleUnfoldings of quasi-periodic tori Mem. Am. Math. Soc. 83 IssueID421 1–81

    Google Scholar 

  • G.D. Birkhoff (1913) ArticleTitleProof of Poincaré’s geometric theorem Trans. Am. Math. Soc. 14 IssueID1 14–22

    Google Scholar 

  • G.D. Birkhoff (1925) ArticleTitleAn extension of Poincaré’s last geometric theorem Acta Math. 47 297–311

    Google Scholar 

  • H.W. Broer M. Levi (1995) ArticleTitleGeometrical aspects of stability theory for Hill’s equations Arch. Rational Mech. Anal. 131 IssueID3 225–240

    Google Scholar 

  • Broer, H.W., Levi, M., and Simó, C. In preparation.

  • Broer H.W., Lunter G.A., Vegter G. (1998). Equivariant singularity theory with distinguished parameters. Two case studies of resonant Hamiltonian systems. Phys. D, 112(1–2): 64–80. Time-reversal symmetry in dynamical systems (Warwick, 1996).

  • Broer, H.W., van Noort, M., and Simó, C. In preparation.

  • Broer, H.W., and Roussarie, R. (2001). Exponential confinement of chaos in the bifurcation sets of real analytic diffeomorphisms. In Broer, H.W., Krauskopf, B., and Vegter, G. (eds.), Global Analysis of Dynamical Systems-Festschrift Dedicated to Floris Takens for his 60th Birthday, Inst. Phys. 167–210.

  • H.W. Broer R. Roussarie C. Simó (1996) ArticleTitleInvariant circles in the Bogdanov-Takens bifurcation for diffeomorphisms. Ergodic Theo Dynam. Syst. 16 IssueID6 1147–1172

    Google Scholar 

  • H.W. Broer C. Simó (1998) ArticleTitleHill’s equation with quasi-periodic forcing: resonance tongues, instability pockets and global phenomena Bol. Soc. Brasil. Mat.(N.S.). 29 IssueID2 253–293

    Google Scholar 

  • H.W. Broer C. Simó (2000) ArticleTitleResonance tongues in Hill’s equation: a geometric approach J. Diff. Eq. 166 IssueID2 290–327

    Google Scholar 

  • H.W. Broer C. Simó J.C. Tatjer (1998) ArticleTitleTowards global models near homoclinic tangencies of dissipative diffeomorphisms Nonlinearity. 11 IssueID3 667–770

    Google Scholar 

  • H.W. Broer F. Takens (1989) ArticleTitleFormally symmetric normal forms and genericity Dyn. Report. 2 39–59

    Google Scholar 

  • H.W. Broer G. Vegter (1992) ArticleTitleBifurcational aspects of parametric resonance Dyn. Report.(N.S.). 1 1–53

    Google Scholar 

  • E. Castellà À. Jorba (2000) ArticleTitleOn the vertical families of two-dimensional tori near the triangular points of the bicircular problem Celestial Mech. Dyn. Astronom. 76 IssueID1 35–54

    Google Scholar 

  • L. Chierchia E. Zehnder (1989) ArticleTitleAsymptotic expansions of quasiperiodic solutions Ann. Scuola Norm. Sup. Pisa Cl. Sci.(4) 16 IssueID2 245–258

    Google Scholar 

  • E. Doedel H.B. Keller J.-P. Kernévez (1991) ArticleTitleNumerical analysis and control of bifurcation problems. I. Bifurcation in finite dimensions Int. J. Bifur. Chaos Appl. Sci. Engrg. 1 IssueID3 493–520

    Google Scholar 

  • E. Doedel H.B. Keller J.-P. Kernévez (1991) ArticleTitleNumerical analysis and control of bifurcation problems. II. Bifurcation in infinite dimensions Int. J. Bifur. Chaos Appl. Sci. Engrg. 1 IssueID4 745–772

    Google Scholar 

  • H.R. Dullin J.D. Meiss D. Sterling (2000) ArticleTitleGeneric twistless bifurcations Nonlinearity. 13 IssueID1 203–224

    Google Scholar 

  • Duistermaat, J.J. (1984). Bifurcation of periodic solutions near equilibrium points of Hamiltonian systems. In Bifurcations Theory and Applications (Montecatini, 1983), Vol. 1057 of Lecture Notes in Mathematics, Springer Verlag, 57–105.

  • Y. Elskens D.F. Escande (1991) ArticleTitleSlowly pulsating separatrices sweep homoclinic tangles where islands must be small: an extension of classical adiabatic theory Nonlinearity. 4 IssueID3 615–667

    Google Scholar 

  • F. Fassò (1990) ArticleTitleLie series method for vector fields and Hamiltonian perturbation theory Z. Angew. Math. Phys. 41 IssueID6 843–864

    Google Scholar 

  • Guckenheimer, J., and Holmes, P. (1983). Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields, Vol. 42 of Applied Mathematical Sciences, Springer-Verlag.

  • Giorgilli, A., Lazutkin, V.F., and Simó, C. (1998). Visualization of a hyperbolic structure in area preserving maps. Reg. Chaotic Dyn. 2(3–4): 47–61. Correction in same journal, 3(2): 115.

    Google Scholar 

  • Hale, J.K. (1992). Oscillations in Nonlinear Systems, McGraw-Hill, Re-publication: Dover publications.

  • J.K. Hale (1980) Ordinary Differential Equations EditionNumber2 Wiley-Interscience Krieger

    Google Scholar 

  • M. Hénon C. Heiles (1964) ArticleTitleThe applicability of the third integral of motion; some numerical experiments Astronom. J. 69 73–79

    Google Scholar 

  • Huitema, G.B. (1988). Unfoldings of Quaisperiodic Tori. PhD thesis, University of Groningen.

  • À. Jorba J. Villanueva (1997) ArticleTitleOn the normal behaviour of partially elliptic lower-dimensional tori of Hamiltonian systems Nonlinearity. 10 IssueID4 783–822

    Google Scholar 

  • Jorba, À., Zou M. On the numerical integration of ode by means of high-order taylor methods. preprint.

  • Kim, S. -Y., and Hu, B. (1998). Resurrection of an inverted pendulum. Chatoic Dynamics, Electronic journal: http://xxx.lanl.gov/abs/chaodyn/9802015vl.

  • Krauskopf, B., and Osinga, H.M. (2000). Investigating torus bifurcations in the forced van der Pol oscillator. In Numerical Methods for Bifurcation Problems and Large-scale Dynamical Systems (Minneapolis, MN, 1997), Vol. 119 of IMA Vol. Math. Appl. Springer-Verlag, New York, 199–208.

  • Kolmogorov, A.N. (1954). On conservation of conditionally periodic motions for a small change in Hamilton’s function (in Russian). Dokl. Akad. Nauk SSSR (N.S), 98 527–530. English translation in Lecture Notes in Physics 93, 51–56.

  • A.N. Kolmogorov (1957) Théorie générale des systèmes dynamiques et mécanique classique P. Erven N. Noordhoff V. Groningen (Eds) ␣ North-Holland Publishing Co. Amsterdam

    Google Scholar 

  • Kuznetsov, (1995). Elements of Applied Bifurcation Theory, Vol. 112 of Applied Mathematical Sciences, Springer-Verlag.

  • M. Levi (1988) ArticleTitleStability of the inverted pendulum – a topological explanation SIAM Rev. 30 IssueID4 639–644

    Google Scholar 

  • M. Levi (1900) ArticleTitleKAM theory for particles in periodic potentials Ergod. Th. Dyn. Sys. 10 777–785

    Google Scholar 

  • M. Levi (1991) ArticleTitleQuasiperiodic motions in superquadratic time-periodic potentials Comm. Math. Phys. 143 IssueID1 43–83

    Google Scholar 

  • S. Laederich M. Levi (1991) ArticleTitleInvariant curves and time-dependent potentials Ergod. Th. Dyn. Sys. 11 IssueID2 365–378

    Google Scholar 

  • Lichtenberg, A.J., and Lieberman, M.A. (1992). Regular and Chatoic Dynamics, Vol. 38 of Applied Mathematical Sciences, 2nd edn., Springer-Verlag.

  • Lunter G. (1999). Bifurcations in Hamiltonian systems: computing singularities by Gröbner bases. PhD thesis, Rijksuniversiteit roningen.

  • M. Levi W. Weckesser (1995) ArticleTitleStabilization of the inverted linearized pendulum by high frequency vibrations SIAM Rev. 37 IssueID2 219–223

    Google Scholar 

  • É. Mathieu (1868) ArticleTitleMémoire sur le mouvement vibratoire d’une membrane de forme elliptique J. Math. Pures Appl. 13 137–203

    Google Scholar 

  • J.B. McLaughlin (1981) ArticleTitlePeriod-doubling bifurcations and chaotic motion for a parametrically forced pendulum J. Stat. Phys. 24 IssueID2 375–388

    Google Scholar 

  • K.R. Meyer (1970) ArticleTitleGeneric bifurcation of periodic points Trans. Am. Math. Soc. 149 95–107

    Google Scholar 

  • J. Moser (1962) ArticleTitleOn invariant curves of area-preserving mappings of an annulus Nachr. Akad. Wiss. Göttingen. Math-Phys. Kl. II 1–20

    Google Scholar 

  • J. Moser (1967) ArticleTitleConvergent series expansions for quasi- periodic motions Math. Ann. 169 136–176

    Google Scholar 

  • J. Moser (1968) ArticleTitleLectures on Hamiltonian systems Mem. Am. Math. Soc. 81 1–60

    Google Scholar 

  • Moser, J. (1989). Minimal foliations on a torus. In Giaquinta, M. (ed.), Topics in Calculus of Variations (Montecatini Terme, 1987), Vol. 1365 of Lecture Notes in Mathematics, Springer-Verlag, 62–99.

  • J. Moser (1989) ArticleTitleQuasi-periodic solutions of nonlinear elliptic partial differential equations Bol. Soc. Brasil. Mat.(N.S.). 20 IssueID1 29–45

    Google Scholar 

  • Meixner, J., and Schäfke, F.W. (1954). Mathieusche Funktionen und Sphäroidfunktionen, Springer-Verlag.

  • A.I. Neishtadt (1984) ArticleTitleThe separation of motions in systems with rapidly rotating phase J. Appl. Math. Mech. 48 IssueID2 133–139 Occurrence Handle10.1016/0021-8928(84)90078-9 Occurrence Handle86j:34043

    Article  MathSciNet  Google Scholar 

  • S.E. Newhouse (1974) ArticleTitleDiffeomorphisms with infinitely many sinks Topology. 13 9–18

    Google Scholar 

  • A.I. Neishtadt V.V. Sidorenko D.V. Treschev (1997) ArticleTitleStable periodic motions in the problem on passage through a separatrix Chaos. 7 IssueID1 2–11

    Google Scholar 

  • A. Olvera C. Simó (1993) ArticleTitleElliptic non-Birkhoff periodic orbits in the twist maps Int. J. Bifur. Chaos Appl. Sci. Engrg. 3 IssueID1 165–185

    Google Scholar 

  • J. Pöschel (1982) ArticleTitleIntegrability of Hamiltonian systems on Cantor sets Comm. Pure Appl. Math. 35 IssueID5 653–696

    Google Scholar 

  • B. Po Particlevan der M.J.O. Strutt (1928) ArticleTitleOn the stability of the solutions of Mathieu’s equation London Edinburgh Dublin Philos. Mag. 5 18–38

    Google Scholar 

  • Robinson R.C. (1970). Generic properties of conservative systems I, II. Am. J. Math. 92, 562–603 and 897–906.

    Google Scholar 

  • Simó, C. (1989). On the analytical and numerical approximation of invariant manifolds. In Benest, D., and Froeschlé, C. (eds.), Les méthodes modernes de la mécanique céleste, Goutelas, 285–329.

  • C. Simó (1994) Averaging under fast quasiperiodic forcing. In Hamiltonian mechanics Plenum New York 13–34

    Google Scholar 

  • C. Simó (1998) ArticleTitleInvariant curves of analytic perturbed nontwist area preserving maps Regul. Chaotic Dyn. 3 IssueID3 180–195

    Google Scholar 

  • Simó, C. (2001). Global dynamics and fast indicators. In Broer, H.W., Krauskopf, B., and Vegter G. (eds.), Global Analysis of Dynamical Systems-Festschrift Dedicated to Floris Takens for his 60 the Birthday, Institute of Physics Publishing. 373–389.

  • Simó, C., and Treschev, D.V. Evolution of the “last” invariant curve in a family of area preserving maps. preprint.

  • Simó, C., and Treschev, D.V. Stability islands in the vicinity of separatrices of near-integrable symplectic maps. preprint.

  • J.J. Stoker (1950) Nonlinear Vibrations in Mechanical and Electrical Systems Interscience Publishers New York

    Google Scholar 

  • Takens, F. (2001). Forced oscillations and bifurcations. In Applications of global analysis I (Sympos., Utrecht State Univ., Utrecht, 1973), number 3 in Comm. Math. Inst. Rijksuniv. Utrecht, Math. Inst. Rijksuniv. Utrecht, Utrecht, 1–59, 1974 reproduced in Global analysis of dynamical systems – Festschrift dedicated to Floris Takens for his 60th birthday, Institute of Physics Publishing, 1–61.

  • Takens, F. (1974). Introduction to Global Analysis, Mathematisch Instituut, Rijksuniversiteit Utrecht, Utrecht. Lectures held at Utrecht State University, October–December, 1972, Communications of the Mathematical Institute, Rijksuniversiteit Utrecht, No. 1973-2.

  • Wiggins, S. (1990). Introduction to Applied Nonlinear Dynamical Systems and Chaos, Vol. 2 of Texts in Applied Mathematics, Springer-Verlag.

  • M.I. Weinstein J.B. Keller (1988) ArticleTitleHill’s equation with a large potential SIAM J. Appl. Math. 45 IssueID2 200–214

    Google Scholar 

  • M.I. Weinstein J.B. Keller (1987) ArticleTitleAsymptotic behaviour of stability regions for Hill’s equation SIAM J. Appl. Math. 47 IssueID5 941–958

    Google Scholar 

  • J. You (1990) ArticleTitleInvariant tori and Lagrange stability of pendulum-type equations J. Diff. Eq. 85 54–65

    Google Scholar 

Download references

Authors
  1. H. W. Broer
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. I. Hoveijn
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. van Noort
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. C. Simó
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. G. Vegter
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

2000 Mathematical Subject Classification: 37J20, 37J40, 37M20, 70H08.

Dedicated to Professor Shui-Nee Chow on the occasion of his 60th birthday.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Broer, H.W., Hoveijn, I., Noort, M.v. et al. The Parametrically Forced Pendulum: A Case Study in 1 1/2 Degree of Freedom. J Dyn Diff Equat 16, 897–947 (2004). https://doi.org/10.1007/s10884-004-7829-5

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10884-004-7829-5

Keywords

Search

Navigation