Online bottleneck matching

Abstract

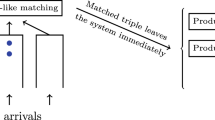
We consider the online bottleneck matching problem, where $k$ server-vertices lie in a metric space and $k$ request-vertices that arrive over time each must immediately be permanently assigned to a server-vertex. The goal is to minimize the maximum distance between any request and its server. Because no algorithm can have a competitive ratio better than $O(k)$ for this problem, we use resource augmentation analysis to examine the performance of three algorithms: the naive Greedy algorithm, Permutation, and Balance. We show that while the competitive ratio of Greedy improves from exponential (when each server-vertex has one server) to linear (when each server-vertex has two servers), the competitive ratio of Permutation remains linear when an extra server is introduced at each server-vertex. The competitive ratio of Balance is also linear with an extra server at each server-vertex, even though it has been shown that an extra server makes it constant-competitive for the min-weight matching problem.