Skip to main content

Advertisement

SpringerLink
Log in

Atomistic modeling of Mg/Nb interfaces: shear strength and interaction with lattice glide dislocations

  • Interface Behavior
  • Published:
Journal of Materials Science Aims and scope Submit manuscript

Abstract

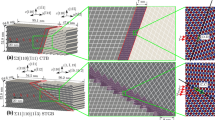
Using a newly developed embedded-atom-method potential for Mg–Nb, the semi-coherent Mg/Nb interface with the Kurdjumov–Sachs orientation relationship is studied. Atomistic simulations have been carried out to understand the shear strength of the interface, as well as the interaction between lattice glide dislocations and the interface. The interface shear mechanisms are dependent on the shear loading directions, through either interface sliding between Mg and Nb atomic layers or nucleation and gliding of Shockley partial dislocations in between the first two atomic planes in Mg at the interface. The shear strength for the Mg/Nb interface is found to be generally high, in the range of 0.9–1.3 GPa depending on the shear direction. As a consequence, the extents of dislocation core spread into the interface are considerably small, especially when compared to the case of other “weak” interfaces such as the Cu/Nb interface.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Figure 1
Figure 2
Figure 3
Figure 4
Figure 5
Figure 6
Figure 7
Figure 8
Figure 9
Figure 10
Figure 11
Figure 12

Similar content being viewed by others

References

  1. Pollock TM (2010) Weight loss with magnesium alloys. Science 328(5981):986–987. doi:10.1126/science.1182848

    Article  Google Scholar 

  2. Ardeljan M, Beyerlein IJ, Knezevic M (2014) A dislocation density based crystal plasticity finite element model: application to a two-phase polycrystalline HCP/BCC composites. J Mech Phys Solids 66:16–31. doi:10.1016/j.jmps.2014.01.006

    Article  Google Scholar 

  3. Ardeljan M, Knezevic M, Nizolek T, Beyerlein IJ, Mara NA, Pollock TM (2015) A study of microstructure-driven strain localizations in two-phase polycrystalline HCP/BCC composites using a multi-scale model. Int J Plast 74:35–57. doi:10.1016/j.ijplas.2015.06.003

    Article  Google Scholar 

  4. Knezevic M, Nizolek T, Ardeljan M, Beyerlein IJ, Mara NA, Pollock TM (2014) Texture evolution in two-phase Zr/Nb lamellar composites during accumulative roll bonding. Int J Plast 57:16–28. doi:10.1016/j.ijplas.2014.01.008

    Article  Google Scholar 

  5. Junkaew A, Ham B, Zhang X, Talapatra A, Arróyave R (2013) Stabilization of bcc Mg in thin films at ambient pressure: experimental evidence and ab initio calculations. Mater Res Lett 1(3):161–167

    Article  Google Scholar 

  6. Ham B, Zhang X (2011) High strength Mg/Nb nanolayer composites. Mater Sci Eng A Struct 528(4–5):2028–2033. doi:10.1016/j.msea.2010.10.101

    Article  Google Scholar 

  7. Kumar A, Beyerlein IJ, Wang J (2014) First-principles study of the structure of Mg/Nb multilayers. Appl Phys Lett 105(7):071602

    Article  Google Scholar 

  8. Pathak S, Velisavljevic N, Baldwin JK, Jain M, Zheng S, Mara NA, Beyerlein IJ (2017) Strong, ductile, and thermally stable bcc-Mg Nanolaminates. Sci Rep Uk 7(1):8264. doi:10.1038/s41598-017-08302-5

    Article  Google Scholar 

  9. Chen Y Unpublished

  10. Wang J, Hoagland RG, Hirth JP, Misra A (2008) Atomistic modeling of the interaction of glide dislocations with “weak” interfaces. Acta Mater 56(19):5685–5693. doi:10.1016/j.actamat.2008.07.041

    Article  Google Scholar 

  11. Daw MS, Baskes MI (1984) Embedded-atom method—derivation and application to impurities, surfaces, and other defects in metals. Phys Rev B 29(12):6443–6453. doi:10.1103/Physrevb.29.6443

    Article  Google Scholar 

  12. Liu XY, Adams JB, Ercolessi F, Moriarty JA (1996) EAM potential for magnesium from quantum mechanical forces. Model Simul Mater Sci 4(3):293–303

    Article  Google Scholar 

  13. Ackland GJ, Thetford R (1987) An improved N-body semiempirical model for body-centered cubic transition-metals. Philos Mag A 56(1):15–30

    Article  Google Scholar 

  14. Zhang RF, Wang J, Beyerlein IJ, Germann TC (2011) Twinning in bcc metals under shock loading: a challenge to empirical potentials. Philos Mag Lett 91(12):731–740. doi:10.1080/09500839.2011.615348

    Article  Google Scholar 

  15. Zhang RF, Germann TC, Liu XY, Wang J, Beyerlein IJ (2014) Layer size effect on the shock compression behavior of fcc–bcc nanolaminates. Acta Mater 79:74–83. doi:10.1016/j.actamat.2014.07.016

    Article  Google Scholar 

  16. Zhang L, Martinez E, Caro A, Liu X-Y, Demkowicz MJ (2013) Liquid-phase thermodynamics and structures in the Cu–Nb binary system. Model Simul Mater Sci 21(2):025005

    Article  Google Scholar 

  17. Demkowicz MJ, Hoagland RG (2009) Simulations of Collision Cascades in Cu–Nb Layered Composites Using an Eam Interatomic Potential. Int J Appl Mech 1(3):421–442

    Article  Google Scholar 

  18. Liu XY, Hoagland RG, Wang J, Germann TC, Misra A (2010) The influence of dilute heats of mixing on the atomic structures, defect energetics and mechanical properties of fcc–bcc interfaces. Acta Mater 58(13):4549–4557. doi:10.1016/j.actamat.2010.05.008

    Article  Google Scholar 

  19. Liu XY, Hoagland RG, Demkowicz MJ, Nastasi M, Misra A (2012) The influence of lattice misfit on the atomic structures and defect energetics of face centered cubic-body centered cubic interfaces. J Eng Mater T Asme 134(2):021012

    Article  Google Scholar 

  20. Wei QM, Liu XY, Misra A (2011) Observation of continuous and reversible bcc-fcc phase transformation in Ag/V multilayers. Appl Phys Lett 98(11):111907

    Article  Google Scholar 

  21. Chen Y, Shao S, Liu XY, Yadav SK, Li N, Mara N, Wang J (2017) Misfit dislocation patterns of Mg–Nb interfaces. Acta Mater 126:552–563. doi:10.1016/j.actamat.2016.12.041

    Article  Google Scholar 

  22. Wang J, Hoagland R, Liu X, Misra A (2011) The influence of interface shear strength on the glide dislocation–interface interactions. Acta Mater 59(8):3164–3173

    Article  Google Scholar 

  23. Barnett DM, Lothe J (1974) An image force theorem for dislocations in anisotropic bicrystals. J Phys F Met Phys 4(10):1618

    Article  Google Scholar 

  24. Wang J, Hoagland R, Hirth J, Misra A (2008) Atomistic simulations of the shear strength and sliding mechanisms of copper–niobium interfaces. Acta Mater 56(13):3109–3119

    Article  Google Scholar 

  25. Pilania G, Thijsse BJ, Hoagland RG, Lazic I, Valone SM, Liu XY (2014) Revisiting the Al/Al2O3 interface: coherent interfaces and misfit accommodation. Sci Rep Uk 4:4485

    Article  Google Scholar 

  26. Hirth J, Pond R, Hoagland R, Liu X-Y, Wang J (2013) Interface defects, reference spaces and the Frank-Bilby equation. Prog Mater Sci 58(5):749–823

    Article  Google Scholar 

  27. Li J (2003) AtomEye: an efficient atomistic configuration viewer. Model Simul Mater Sci 11(2):173–177

    Article  Google Scholar 

  28. Fan HD, El-Awady JA (2015) Molecular dynamics simulations of orientation effects during tension, compression, and bending deformations of magnesium nanocrystals. J Appl Mech T Asme 82(10):101006

    Article  Google Scholar 

Download references

Acknowledgements

This work was supported by the US Department of Energy, Office of Science, Office of Basic Energy Sciences. The authors acknowledge helpful discussions with Richard G. Hoagland at LANL.

Author information

Authors and Affiliations

  1. Department of Metallurgical and Materials Engineering, Indian Institute of Technology (IIT) Madras, Chennai, 600036, India

    S. K. Yadav

  2. Materials Science and Technology Division, MST-8, Los Alamos National Laboratory, Los Alamos, NM, 87545, USA

    S. K. Yadav, S. Shao & X.-Y. Liu

  3. Department of Mechanical and Industrial Engineering, Louisiana State University, Baton Rouge, LA, 70803, USA

    S. Shao

  4. Materials Physics and Applications Division, MPA-CINT, Los Alamos National Laboratory, Los Alamos, NM, 87545, USA

    Y. Chen

  5. Department of Mechanical and Materials Engineering, University of Nebraska–Lincoln, Lincoln, NE, 68583, USA

    J. Wang

Authors
  1. S. K. Yadav
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. Shao
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Y. Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. J. Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. X.-Y. Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. K. Yadav.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yadav, S.K., Shao, S., Chen, Y. et al. Atomistic modeling of Mg/Nb interfaces: shear strength and interaction with lattice glide dislocations. J Mater Sci 53, 5733–5744 (2018). https://doi.org/10.1007/s10853-017-1703-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10853-017-1703-4

Keywords

Search

Navigation