Skip to main content
SpringerLink
Log in

On Multiple Secondary Task Execution of Redundant Nonholonomic Mobile Manipulators

  • Published:
Journal of Intelligent and Robotic Systems Aims and scope Submit manuscript

Abstract

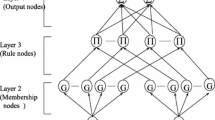
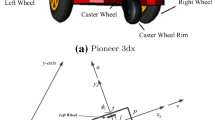
This paper investigates self-motion control of redundant nonholonomic mobile manipulators, to execute multiple secondary tasks including tip-over prevention, singularity removal, obstacle avoidance and physical limits escape. An extended gradient projection method (EGPM) is proposed to determine self-motion directions, and a real-time fuzzy logic self-motion planner (FLSMP) is devised to generate the corresponding self-motion magnitudes. Unlike the task-priority allocation method and the extended Jacobian method, the proposed scheme is simple to implement and is free from algorithm singularities. The proposed dynamic model is established with consideration of nonholonomic constraints of the mobile platform, interactive motions between the mobile platform and the onboard manipulator, as well as self-motions allowed by redundancy of the entire robot. Furthermore, a robust adaptive neural-network controller (RANNC) is developed to accomplish multiple secondary tasks without affecting the primary one in the workspace. The RANNC does not rely on precise prior knowledge of dynamic parameters and can suppress bounded external disturbance effectively. In addition, the RANNC does not require any off-line training and can ensure the control performance by online adjusting the neural-network parameters through adaptation laws. The effectiveness of the proposed algorithm is verified via simulations on a three-wheeled redundant nonholonomic mobile manipulator.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. Jamisola, R., Ang, M.H. Jr., Oetomo, D., Khatib, O., Lim, T.M., Lim, S.Y.: The operational space formulation implementation to aircraft canopy polishing using a mobile manipulator. In: Proc. of IEEE Intl. Conf. on Rob. and Autom., pp. 400–405. Washington, DC (2002)

  2. Kurabayashi, D., Ota, J., Arai, T., Yoshida, E.: Cooperative sweeping by multiple mobile robots. In: Proc. of IEEE Intl. Conf. on Rob. and Autom., pp. 1744–1749. Minneapolis, Minnesota (1996)

  3. Yu, Q., Chen, I.: A general approach to the dynamics of nonholonomic mobile manipulator systems. ASME Trans. Dyn. Syst. Meas. Control 124(4), 512–521 (2002)

    Article  MathSciNet  Google Scholar 

  4. Chung, J.H., Velinsky, S.A., Hess, R.A.: Interaction control of a redundant mobile manipulator. Int. J. Rob. Res. 17(12), 1302–1309 (1998)

    Article  Google Scholar 

  5. Yamamoto, Y., Yun, X.: Effect of the dynamic interaction on coordinated control of mobile manipulators. IEEE Trans. Robot. Autom. 12(5), 816–824 (1996)

    Article  Google Scholar 

  6. Rey, D.A., Papadopoulos, E.: On-line automatic tipover prevention for mobile manipulators. In: Proc. IEEE/RSJ Conf. Intell. Robots and Syst., pp. 1273–1278. Grenoble, France (1997)

  7. Li, Y., Liu, Y.: A new task-consistent overturn prevention algorithm for redundant mobile modular manipulators. In: Proc. of IEEE/RSJ Intl. Conf. on Intelligent Rob. Syst., pp. 1563–1568. Alberta, Canada (2005)

  8. Li, Y., Liu, Y.: Real-time tip-over prevention and path following control for redundant nonholonomic mobile modular manipulators via fuzzy and neural-fuzzy approaches. ASME Trans. Dyn. Syst. Meas. Control 128(4), 753–764 (2006)

    Article  Google Scholar 

  9. Tan, J., Xi, N., Wang, Y.: Integrated task planning and control for mobile manipulators. Int. J. Rob. Res. 22(5), 337–354 (2003)

    Article  Google Scholar 

  10. Papadopoulos, E., Poulakakis, I., Papadimitriou, I.: On path planning and obstacle avoidance for nonholonomic platforms with manipulators: a polynomial approach. Int. J. Rob. Res. 21(4), 367–383 (2002)

    Article  Google Scholar 

  11. Carriker, W.F., Khosla, P.K., Krogh, B.H.: Path Planning for mobile manipulators for multiple task execution. IEEE Trans. Robot. Autom. 7(3), 403–408 (1991)

    Article  Google Scholar 

  12. Tanner, H.G., Loizou, S.G., Kyriakopoulos, K.J.: Nonholonomic navigation and control of cooperating mobile manipulators. IEEE Trans. Robot. Autom. 19(1), 53–64 (2003)

    Article  Google Scholar 

  13. Sugar, T.G., Kumar, V.: Control of cooperating mobile manipulators. IEEE Trans. Robot. Autom. 18(1), 94–103 (2002)

    Article  Google Scholar 

  14. Brock, O., Khatib, O., Viji, S.: Task-consistent obstacle avoidance and motion behavior for mobile manipulation. In: Proc. of IEEE Intl. Conf. on Rob. and Autom., pp. 388–393. Washington DC, USA (2002)

  15. De Luca, A., Oriolo, G., Giordano, P.R.: Kinematic modeling and redundancy resolution for nonholonomic mobile manipulators. In: Proc. of IEEE Intl. Conf. on Rob. and Autom., pp. 1867–1873. Orlando, Florida (2006)

  16. Assal, S.F.M., Watanabe, K., Izumi, K.: Neural network-based kinematic inversion of industrial redundant robots using cooperative fuzzy hint for the joint limits avoidance. IEEE/ASME Trans. Mechatron. 11(5), 593–603 (2006)

    Article  Google Scholar 

  17. Chan, T.F., Dubey, R.V.: A weighted least-norm solution based scheme for avoiding joint limits for redundant joint manipulators. IEEE Trans. Robot. Autom. 11(2), 286–292 (1995)

    Article  Google Scholar 

  18. Liu, Y., Li, Y.: A new method of executing multiple auxiliary tasks by redundant nonholonomic mobile manipulators. In: Proc. IEEE/RSJ Intl. Conf. on Intelligent Rob. and Syst., pp. 1–6. Beijing, China (2006)

  19. Cheng, F.T., Lu, Y.T., Sun, Y.Y.: Window-shaped obstacle avoidance for a redundant manipulator. IEEE Trans. Syst. Man Cybern., Part B, Cybern. 28(6), 806–815 (1998)

    Article  Google Scholar 

  20. Maciejewski, A.A., Klein, C.A.: Obstacle avoidance for kinematically redundant manipulators in dynamically varying environments. Int. J. Rob. Res. 4(3), 109–117 (1985)

    Article  Google Scholar 

  21. Chiaverini, S.: Singularity-robust task-priority redundancy resolution for real-time kinematic control of robot manipulators. IEEE Trans. Robot. Autom. 13(3), 398–410 (1997)

    Article  Google Scholar 

  22. Kim, J., Marani, G., Chung, W.K., Yuh, J., Oh, S.R.: Dynamic task priority approach to avoid kinematic singularity for autonomous manipulation. In: Proc. of IEEE/RSJ Intl. Conf. on Intell. Rob. and Syst., pp. 1942–1947. Switzerland (2002)

  23. Nenchev, D.N., Tsumaki, Y., Uchiyama, M.: Singularity-consistent parameterization of robot motion and control. Int. J. Rob. Res. 19(2), 159–182 (2000)

    Article  Google Scholar 

  24. Jamisola, R.S., Maciejewski, A.A., Roberts, R.G.: Failure-tolerant path planning for kinematically redundant manipulators anticipating locked-joint failures. IEEE Trans. Robot. 22(4), 603–612 (2006)

    Article  Google Scholar 

  25. Ding, H., Tso, S.K.: A fully neural-network-based planning scheme for torque minimization of redundant manipulators. IEEE Trans. Ind. Electron. 46(1), 199–206 (1999)

    Article  Google Scholar 

  26. Ma, S.: A new formulation technique for local torque optimization of redundant manipulators. IEEE Trans. Ind. Electron. 43(4), 462–468 (1996)

    Article  Google Scholar 

  27. Gravagne, I., Walker, I.D.: On the structure of minimum effort solutions with application to kinematic redundancy resolution. IEEE Trans. Robot. Autom. 16(6), 855–863 (2000)

    Article  Google Scholar 

  28. Klein, C.A., Chu-Jenq, C., Ahmed, S.: A new formulation of the extended jacobian method and its use in mapping algorithmic singularities for kinematically redundant manipulators. IEEE Trans. Robot. Autom. 11(1), 50–55 (1995)

    Article  Google Scholar 

  29. Oh, Y., Chung, W.K.: Disturbance-observer-based motion control of redundant manipulators using inertially decoupled dynamics. IEEE/ASME Trans. Mechatron. 4(2), 133–146 (1999)

    Article  Google Scholar 

  30. Maciejewski, A.A., Klein, C.A.: The singular value decomposition: computation and applications to robotics. Int. J. Rob. Res. 8(6), 63–79 (1989)

    Article  Google Scholar 

  31. Chang, P.H., Park, K.C., Lee, S.: An extension to operational space for kinematically redundant manipulators: kinematics and dynamics. IEEE Trans. Robot. Autom. 16(5), 592–596 (2000)

    Article  Google Scholar 

  32. O’Neil, K.: Divergence of linear acceleration-based redundancy resolution schemes. IEEE Trans. Robot. Autom. 18(4), 625–631 (2002)

    Article  Google Scholar 

  33. Park, J., Chung, W.K., Youm, Y.: Characterization of instability of dynamic control for kinematically redundant manipulators. In: Proc. of IEEE Intl. Conf. on Rob. and Autom., pp. 2400–2405. Washington DC (2002)

  34. Slotine, J.J.E., Li, W.: On the adaptive control of robot manipulators. Int. J. Rob. Res. 6(3), 49–59 (1987)

    Article  Google Scholar 

  35. Cheah, C.C., Liu, C., Slotine, J.J.J.E.: Adaptive Jacobian tracking control of robots with uncertainties in kinematic, dynamic and actuator models. IEEE Trans. Automat. Contr. 51(6), 1024–1029 (2006)

    Article  MathSciNet  Google Scholar 

  36. Ge, S.S., Hang, C.C., Woon, L.C.: Adaptive neural network control of robot manipulators in task space. IEEE Trans. Ind. Electron. 44(6), 746–752 (1997)

    Article  Google Scholar 

  37. Ge, S.S., Lee, T.H., Harris, C.J.: Adaptive Neural Network Control of Robotic Manipulators. World Scientic, Singapore (1998)

    Google Scholar 

  38. Lewis, F.L., Yesildirek, A., Liu, K.: Multilayer neural-net robot controller with guaranteed tracking performance. IEEE Trans. Neural Netw. 7(2), 388–399 (1996)

    Article  Google Scholar 

  39. Fierro, R., Lewis, F.L.: Control of a nonholonomic mobile robot using neural networks. IEEE Trans. Neural Netw. 9(4), 589–600 (1998)

    Article  Google Scholar 

  40. Sheng, L., Goldenberg, A.: Neural-network control of mobile manipulators. IEEE Trans. Neural Netw. 12(5), 1121–1133 (2001)

    Article  Google Scholar 

  41. Kiguchi, K., Fukuda, T.: Intelligent position/force controller for industrial robot manipulators—application of fuzzy neural networks. IEEE Trans. Ind. Electron. 44(6), 753–761 (1997)

    Article  Google Scholar 

  42. Liu, Y., Li, Y.: Sliding mode adaptive neural-network control for nonholonomic mobile modular manipulators. J. Intell. Robot. Syst. 44(3), 203–224 (2005)

    Article  Google Scholar 

  43. de Wit, C.C., Siciliano, B., Bastin, G.: Theory of Robot Control. Springer, New York (1996)

    MATH  Google Scholar 

  44. Horn, R., Johnson, C.: Matrix Analysis. Cambridge University Press, Cambridge (1991)

    MATH  Google Scholar 

  45. Gupta, M.M., Jin, L., Homma, N.: Static and Dynamic Neural Networks—From Fundamentals to Advanced Theorey. Wiley, New York (2003)

    Book  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Aerospace Engineering, Ryerson University, Toronto, Ontario, Canada, M5B 2K3

    Yugang Liu & Guangjun Liu

Authors
  1. Yugang Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Guangjun Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Guangjun Liu.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Liu, Y., Liu, G. On Multiple Secondary Task Execution of Redundant Nonholonomic Mobile Manipulators. J Intell Robot Syst 56, 365 (2009). https://doi.org/10.1007/s10846-009-9323-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10846-009-9323-0

Keywords

Search

Navigation