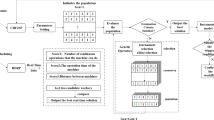
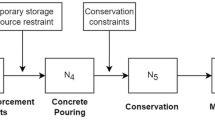
Abstract
Fashion products require a significant amount of customization due to differences in body measurements, diverse preferences on style and replacement cycle. It is necessary for today’s apparel industry to be responsive to the ever-changing fashion market. Just-in-time production is a must-go direction for apparel manufacturing. Apparel industry tends to generate more production orders, which are split into smaller jobs in order to provide customers with timely and customized fashion products. It makes the difficult task of production planning even more challenging if the due times of production orders are fuzzy and resource competing. In this paper, genetic algorithms and fuzzy set theory are used to generate just-in-time fabric-cutting schedules in a dynamic and fuzzy cutting environment. Two sets of real production data were collected to validate the proposed genetic optimization method. Experimental results demonstrate that the genetically optimized schedules improve the internal satisfaction of downstream production departments and reduce the production cost simultaneously.
Similar content being viewed by others
References
U. Bagchi R.S. Sullivan Y.L. Chang (1986) ArticleTitleMinimizing mean absolute deviation of completion times about a common due date Naval Research Logistics Quarterly 33 227–240
U. Bagchi R.S. Sullivan Y.L. Chang (1987) ArticleTitleMinimizing mean square deviation of completion times about a common due date Management Sciences 33 894–906
K.R. Baker G.D. Scudder (1990) ArticleTitleSequencing with earliness and tardiness penalties: A review Operations Research 38 IssueID1 22–36
Z.L. Chen C.Y. Lee (2002) ArticleTitleParallel machine scheduling with a common due window European Journal of Operational Research 136 IssueID3 512–517 Occurrence Handle10.1016/S0377-2217(01)00068-6
R. Cheng M. Gen T. Tozawa (1995) ArticleTitleMinmax earliness/tardiness scheduling in identical parallel machine system using genetic algorithms Computers & Industrial Engineering 29 513–517
T.C.E. Cheng Z.L. Chen (1994) ArticleTitleParallel-machine scheduling problems with earliness and tardiness penalties Journal of Operational Research Society 45 IssueID6 685–695
T.C.E. Cheng Z.L. Chen C.L. Li (1996) ArticleTitleParallel-machine scheduling with controllable processing times IIE Transactions 28 IssueID2 177–180
T.C.E. Cheng C.C.S. Sin (1990) ArticleTitleA state-of-the-art review of parallel-machine scheduling research European Journal of Operational Research 47 271–292 Occurrence Handle10.1016/0377-2217(90)90215-W
De Jong K.A. (1975). An analysis of the behavior of a class of genetic adaptive systems, Doctoral dissertation. University of Michigan, Ann Arbor
P. De J.B. Ghosh C.E. Wells (1991) ArticleTitleScheduling to minimize weighted earliness and tardiness about a common due-date Computers & Operations Research 18 IssueID5 465–475
P. De J.B. Ghosh C.E. Wells (1993) ArticleTitleOn the general solution for a class of early/tardy problems Computers & Operations Research 20 IssueID2 141–149 Occurrence Handle10.1016/0305-0548(93)90070-Y
D. Dubois H. Fargier P. Fortemps (2003) ArticleTitleFuzzy scheduling: modeling flexible constraints vs. coping with incomplete knowledge European Journal of Operational Research 147 231–252
Goldberg, D.E. (1989). Genetic algorithms in search, optimisation, and machine learning, Addison-Wesley.
N.G. Hall M.E. Posner (1991) ArticleTitleEarliness-tardiness scheduling problems, I: weighted deviation of completion times about a common due date Operations Research 39 IssueID5 836–846
N.G. Hall W. Kubiak S.P. Sethi (1991) ArticleTitleEarliness-tardiness scheduling problems, II: deviation of completion times about a restrictive common due date Operations Research 39 IssueID5 847–856
S. Han H. Ishii S. Fujii (1994) ArticleTitleOne machine scheduling problem with fuzzy duedates European Journal of Operational Research 79 1–12 Occurrence Handle10.1016/0377-2217(94)90391-3
J.H. Holland (1975) Adaptation in natural and artificial systems University of Michigan Press Ann Arbor, MI
J.A. Hoogeveen S.L. Velde Particlevan de (1991) ArticleTitleScheduling around a small common due date European Journal of Operational Research 55 237–242 Occurrence Handle10.1016/0377-2217(91)90228-N
H. Ishibuchi T. Murata (2000) Flowshop scheduling with fuzzy duedate and fuzzy processing time R. Słowiński M. Hapke (Eds) Scheduling under Fuzziness Physica-Verlag Heidelberg, New York 113–143
H. Ishibuchi N. Yamamoto S. Misaki H. Tanaka (1994) ArticleTitleLocal search algorithms for low shop scheduling with fuzzy due-dates International Journal of Production Economics 33 53–66 Occurrence Handle10.1016/0925-5273(94)90118-X
J.J. Kanet (1981) ArticleTitleMinimizing the average deviation of job completion times about a common due date Naval Research Logistics Quarterly 28 643–651
Mok P.Y., Kwong C.K., Wong W.K. (2004). Optimisation of fault-tolerant fabric-cutting schedules using genetic algorithms and fuzzy set theory. European Journal of Operational Research, forthcoming.
W. Szwarc (1989) ArticleTitleSingle machine scheduling to minimize absolute deviation of completion times from a common due date Naval Research Logistics Quarterly 36 663–674
R. Słowiński M. Hapke (2000) Scheduling under fuzziness Physica-Verlag Heidelberg, New York
J.A. Ventura D. Kim (2003) ArticleTitleParallel machine scheduling with earliness-tardiness penalties and additional resource constraints Computers & Operations Research 30 IssueID13 1945–1958 Occurrence Handle10.1016/S0305-0548(02)00118-1
Wong, W.K., Kwong, C.K., Mok, P.K., Ip, W.H., & Chan, C.K. (2005). Optimization of manual fabric-cutting process in apparel manufacturing using genetic algorithms. International Journal of Advanced ManufacturingTechnology, DOI 10.1007/s00170-004-2161-0.
L.A. Zadeh (1965) ArticleTitleFuzzy sets Information and Control 8 338–353 Occurrence Handle10.1016/S0019-9958(65)90241-X
Author information
Authors and Affiliations
Corresponding author
Additional information
Received: November 2004 / Accepted: September 2005
Rights and permissions
About this article
Cite this article
Wong, W.K., Kwong, C.K., Mok, P.Y. et al. Genetic Optimization of JIT Operation Schedules for Fabric-cutting Process in Apparel Manufacture. J Intell Manuf 17, 341–354 (2006). https://doi.org/10.1007/s10845-005-0007-8
Issue Date:
DOI: https://doi.org/10.1007/s10845-005-0007-8