Abstract
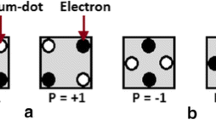
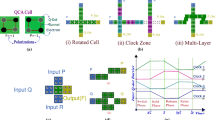
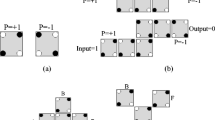
This paper analyzes the effect of random phase shifts in the underlying clock signals on the operation of several basic Quantum-dot Cellular Automata (QCA) building blocks. Such phase shifts can result from manufacturing variations or from uneven path lengths in the clocking network. We perform numerical simulations of basic building blocks using two different simulation engines available in the QCADesigner tool. We assume that the phase shifts are characterized by a Gaussian distribution with a mean value of \(i \frac{\pi}{2}\), where i is the clock number and a standard deviation, σ, which is varied in each simulation. Our results indicate that the sensitivity of building blocks to phase shifts depends primarily on the layout while the reliability of all building blocks starts to drop once the standard deviation, σ exceeds 4°. A full adder was simulated to analyze the operation of a circuit featuring a combination of the building blocks considered here. Results are consistent with expectations and demonstrate that the carry output of the full adder is better able to withstand the phase shifts in the clocking network than the Sum output which features a larger combination of the simulated building blocks.
















Similar content being viewed by others
References
Amlani I, Orlov A, Snider GL, Lent CS (1998) Demonstration of a functional quantum-dot cellular automata cell. J Vac Sci Technol B 16:3795–3799
Amlani I, Orlov AO, Toth G, Bernstein GH, Lent CS, Snider GL (1999) Digital logic gate using quantum-dot cellular automata. Science 284:289–291
Bhanja S, Ottavi M, Pontarelli S, Lombardi F (2006) Novel designs for thermally robust coplanar crossing in qca. In: Proc of design, automation and test in Europe, 2006. DATE ’06., vol 1
Bernstein GH, Imre A, Metlushko V, Ji L, Orlov A, Csaba G, Porod W (2005) Magnetic QCA systems. Microelectronics J 36:619–624
Chau R, Kavalieros J, Roverts B, Schenker R, Lionberger D, Barlag D, Doyle B, Arghavani R, Murthy A, Deweyt G (2000) 30-nm physical gate length cmos transistors with 1.0-ps nmos and 1.7-ps pmos gate delays. In IEDM Tech. Dig., pp 45–48
Chaudhary A, Chen DJ, Hu XS, Niemier MT, Ravichandran R, Whitton K (2005) Eliminating wire crossings for molecular quantum-dot cellular automata implementation. In: IEEE/ACM international conference on computer-aided design, 2005. IICAD-2005, pp 564–571
Chaudhary A, Chen DJ, Hu XS, Niemier MT, Ravichandran R, Whitton K (2007) Fabricatable interconnect and molecular QCA circuits. IEEE Trans On CAD of IC 26(11):1978–1991
Frank DJ, Dennard RH, Nowak E, Solomon PM, Taur Y, Wong HSP (2001) Device scaling limits of si mosfets and their application dependencies. In: Proc. of IEEE, vol 89, pp 259–288
Gin A, Tougaw PD, Williams S (1999) An alternative geometry for quantum-dot cellular automata. J App Phys 85(12):8281–8286
György C, et al. (2002) Nanocomputing by field-coupled nanomagnets. IEEE Trans Nano 1(4):209–213
György C, Porod W (2002) Simulation of field coupled computing architectures based on magnetic dot arrays. J Comp Elec 1(1):87–91
Imre A, Csaba G, Ji L, Orlov A, Bernstein GH, Porod W (1999) Majority logic gate for magnetic quantum-dot cellular automata. Science 311(5758):205–208
International Technology Roadmap for Semiconductors (ITRS) (2004) [Online] http://public.itrs.net
Jiao J, Long GJ, Grandjean F, Beatty AM, Fehlner TP (2003) Building blocks for the molecular expression of quantum cellular automata. isolation and characterization of a covalently bonded square array of two ferrocenium and two ferrocene complexes. J Am Chem Soc 125(25):7522–7523
Jin Z (2006) Fabrication and measurement of molecular quantum cellular automata (QCA) device. PhD thesis, University of Notre Dame, Notre Dame, IN 46556
Kummamuru RV, Timler J, Toth G, Lent CS, Ramasubramaniam R, Orlov A, Bernstein GH (2002) Power gain and dissipation in a quantum-dot cellular automata latch. Appl Phys Lett 81:1332–1334
Lent CS (1993) Quantum cellular automata. Nanotechnology 4:49–57
Lent CS, Snider GL, Bernstein GH, Porod W, Orlov A, Lieberman M, Fehlner T, Niemier MT, Kogge P (2003) Quantum-dot cellular automata. Kluwer Academic Publishers, Boca Raton, FL, USA
Lent CS, Isaksen B, Lieberman M (2003) Molecular quantum-dot cellular automata. J Am Chem Soc 125:1056–1063
Lent CS, Isaksen B (2003) Clocked molecular quantum-dot cellular automata. IEEE Trans Electron Devices 50(9):1890–1896
Li Z, Fehlner TP (2003) Molecular qca cells. 2. Characterization of an unsymmetrical dinuclear mixed-valence complex bound to a au surface by an organic linker. Inorg Chem 42(18):5715–5721
Li Z, Beatty AM, Fehlner TP (2003) Molecular qca cells. 1. Structure and functionalization of an unsymmetrical dinuclear mixed-valence complex for surface binding. Inorg Chem 42(18):5707–5714
Lu Y, Lent CS (2005) Theoretical study of molecular quantum-dot cellular automata. J Comput Elec 4:115–118
Momenzadeh M, Ottavi M, Lombardi F (2005) Modeling qca defects at molecular-level in combinational circuits. In: Proc IEEE conf on defect and fault tolerance, pp 208–216
Moore GE (1995) Lithography and the future of moore’s law. In: Proc of SPIE, advances in resist technology and processing, vol 2438, pp 2–17
Natarajan S, Breuer MA, Gupta SK (1998) Process variations and their impact on circuit operation. In: Proc IEEE conf on defect and fault tolerance, pp 73–81
Orlov AO, Kummamuru RK, Ramasubramaniam R, Lent CS, Berstein GH, Snider GL (2003) Clocked quantum-dot cellular automata shift register. Surf Sci 532–535:1193–1198
Parish MCB (2003) Modeling of physical constraints on bistable magnetic quantum cellular automata. PhD thesis, University of London
Qi H, Sharma S, Li Z, Snider GL, Orlov AO, Lent CS, Fehlner TP (2003) Molecular quantum cellular automata cells. Electric field driven switching of a silicon surface bound array of vertically oriented two-dot molecular quantum cellular automata. J Am Chem Soc 125(49):15250–15259
Risch L (2001) How small can mosfets get? In: Proc of SPIE, advances in microelectronic device technology, vol 4600, pp 1–9
Schulhof G, Walus K, Jullien GA (2007) Simulation of random cell displacements in QCA. J Emerg Technol Comput Syst 3(1):2
Snider GL, Amlani I, Orlov A, Toth G, Bernstein G, Lent CS, Merz JL, Porod W (1999) Quantum-dot cellular automata: line and majority gate logic. Jpn J Appl Phys 38:7227–7229
Tahoori MB, Momenzadeh M, Huang J, Lombardi F (2003) Defects and faults in quantum cellular automata at nano scale. In: Proc IEEE VLSI test symposium, pp 291–296
Toth G, Lent CS (1999) Quasiadiabatic switching of metal-island quantum-dot cellular automata. J Appl Phys 85(5):2977–2984
Tougaw PD, Lent CS (1994) Logical devices implemented using quantum cellular automata. J Appl Phys 75(3):1818–1825
Walus K, Jullien GA (2006) Design tools for an emerging SoC technology: quantum-dot cellular automata. Proc. IEEE, 94(6):1225–1244
Walus K, Dysart TJ, Jullien GA, Budiman RA (2004) QCADesigner: a rapid design and simulation tool for quantum-dot cellular automata. IEEE Trans Nano 3(1):26–31
Walus K, Schulhof G (2001) QCADesigner Homepage. [Online] http://www.qcadesigner.ca/
Walus K, Mazur M, Schulhof G, Jullien GA (2005) Simple 4-bit processor based on quantum-dot cellular automata (QCA). In: Proc of application specific architectures, and processors conference
Welland ME, Cowburn RP (2000) Room temperature magnetic quantum cellular automata. Science 287:1466–1468
Author information
Authors and Affiliations
Corresponding author
Additional information
Responsible Editors: C. Bolchini and Y.-B. Kim
M. Ottavi is currently with Advanced Micro Devices Inc. This work done when the author was with the ECE Department of Northeastern University, Boston MA.
V. Vankamamidi is currently with EMC Corporation.
Rights and permissions
About this article
Cite this article
Karim, F., Ottavi, M., Hashempour, H. et al. Modeling and Evaluating Errors Due to Random Clock Shifts in Quantum-Dot Cellular Automata Circuits. J Electron Test 25, 55–66 (2009). https://doi.org/10.1007/s10836-008-5088-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10836-008-5088-9