Skip to main content
SpringerLink
Log in

Bandgap nanoengineering of graphene tunnel diodes and tunnel transistors to control the negative differential resistance

  • Published:
Journal of Computational Electronics Aims and scope Submit manuscript

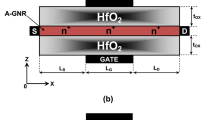
Abstract

By means of numerical simulation based on the Green’s function formalism on a tight binding Hamiltonian, we investigate different possibilities of achieving a strong effect of negative differential resistance in graphene tunnel diodes, the operation of which is controlled by the interband tunneling between both sides of the PN junction. We emphasize on different approaches of bandgap nanoengineering, in the form of nanoribbons (GNRs) or nanomeshes (GNMs), which can improve the device behaviour. In particular, by inserting a small or even zero bandgap section in the transition region separating the doped sides of the junction, the peak current and the peak-to-valley ratio (PVR) are shown to be strongly enhanced and weakly sensitive to the length fluctuations of the transition region, which is an important point regarding applications. The study is extended to the tunneling FET which offers the additional possibility of modulating the interband tunneling and the PVR. The overall work suggests the high potential of GNM lattices for designing high performance devices for either analog or digital applications.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Mizuta, H., Tanoue, T.: The Physics and Application of Resonant Tunnelling Diodes. Cambridge University Press, Cambridge (1995)

    Book  Google Scholar 

  2. Katsnelson, M.I., Novoselov, K.S., Geim, A.K.: Chiral tunnelling and the Klein paradox in graphene. Nat. Phys. 2, 620–625 (2006)

    Article  Google Scholar 

  3. Schwierz, F.: Graphene transistors. Nat. Nanotechnol. 5, 487–496 (2010)

    Article  Google Scholar 

  4. Cresti, A., Grosso, G., Parravicini, G.P.: Valley-valve effect and even-odd chain parity in p-n graphene junctions. Phys. Rev. B 77, 233402 (2008)

    Article  Google Scholar 

  5. Wang, Z.F., Li, Q., Shi, Q.W., Wang, X., Yang, J., Hou, J.G., Chen, J.: Chiral selective tunneling induced negative differential resistance in zigzag graphene nanoribbon: a theoretical study. Appl. Phys. Lett. 92, 133114 (2008)

    Article  Google Scholar 

  6. Nam Do, V., Dollfus, P.: Negative differential resistance in zigzag-edge graphene nanoribbon junctions. J. Appl. Phys. 107, 063705 (2010)

    Article  Google Scholar 

  7. Cheraghchi, H., Esmailzade, H.: A gate-induced switch in zigzag graphene nanoribbons and charging effects. Nanotechnology 21, 205306 (2010)

    Article  Google Scholar 

  8. Habib, K.M.M., Zahid, F., Lake, R.K.: Negative differential resistance in bilayer graphene nanoribbons. Appl. Phys. Lett. 98, 192112 (2011)

    Article  Google Scholar 

  9. Ren, H., Li, Q.-X., Luo, Y., Yang, J.: Graphene nanoribbon as a negative differential resistance device. Appl. Phys. Lett. 94, 173110 (2009)

    Article  Google Scholar 

  10. Teong, H., Lam, K.-T., Khalid, S.B., Liang, G.: Shape effects in graphene nanoribbon resonant tunneling diodes: a computational study. J. Appl. Phys. 105, 084317 (2009)

    Article  Google Scholar 

  11. Hung Nguyen, V., Bournel, A., Dollfus, P.: Resonant tunneling structures based on epitaxial graphene on SiC. Semicond. Sci. Technol. 26, 125012 (2011)

    Article  Google Scholar 

  12. González, J.W., Pacheco, M., Rosales, L., Orellana, P.A.: Transport properties of graphene quantum dots. Phys. Rev. B 83, 155450 (2011)

    Article  Google Scholar 

  13. Mazzamuto, F., Hung Nguyen, V., Apertet, Y., Caër, C., Chassat, C., Saint-Martin, J., Dollfus, P.: Enhanced thermoelectric properties in graphene nanoribbons by resonant tunneling of electrons. Phys. Rev. B 83, 235426 (2011)

    Article  Google Scholar 

  14. Rodríguez-Vargas, I., Madrigal-Melchor, J., Oubram, O.: Resonant tunneling through double barrier graphene systems: a comparative study of Klein and non-Klein tunneling structures. J. Appl. Phys. 112, 073711 (2012)

    Article  Google Scholar 

  15. Hung Nguyen, V., Mazzamuto, F., Bournel, A., Dollfus, P.: Resonant tunneling diode based on graphene/h-BN heterostructure. J. Phys. D, Appl. Phys. 45, 325104 (2012)

    Article  Google Scholar 

  16. Ferreira, G.J., Leuenberger, M.N., Loss, D., Egues, J.C.: Low-bias negative differential resistance in graphene nanoribbon superlattices. Phys. Rev. B 84, 125453 (2011)

    Article  Google Scholar 

  17. Nam Do, V., Hung Nguyen, V., Dollfus, P., Bournel, A.: Electronic transport and spin-polarized effects of relativistic-like particles in graphene structures. J. Appl. Phys. 104, 063708 (2008)

    Article  Google Scholar 

  18. Wu, Y., Farmer, D.B., Zhu, W., Han, S.J., Dimitrakopoulos, C.D., Bol, A.A., Avouris, P., Lin, Y.-M.: Three-terminal graphene negative differential resistance devices. ACS Nano 6, 2610–2616 (2012)

    Article  Google Scholar 

  19. Wu, Y., Perebeinos, V., Lin, Y.-M., Low, T., Xia, F., Avouris, P.: Quantum behavior of graphene transistors near the scaling limit. Nano Lett. 12, 1417–1423 (2012)

    Article  Google Scholar 

  20. Majumdar, K., Kallatt, S., Bhat, N.: High field carrier transport in graphene: insights from fast current transient. Appl. Phys. Lett. 101, 123505 (2012)

    Article  Google Scholar 

  21. Alarcón, A., Hung Nguyen, V., Berrada, S., Saint-Martin, J., Bournel, A., Dollfus, P.: Negative differential conductance and chiral effects in graphene field-effect transistors. In: Proc. IWCE 2012 (2012). doi:10.1109/IWCE.2012.6242820

    Google Scholar 

  22. Hung Nguyen, V., Bournel, A., Dollfus, P.: Large peak-to-valley ratio of negative differential conductance in graphene p-n junctions. J. Appl. Phys. 109, 093706 (2011)

    Article  Google Scholar 

  23. Fiori, G.: Negative differential resistance in mono and bilayer graphene p-n junctions. IEEE Electron Device Lett. 32, 1334–1336 (2011)

    Article  MathSciNet  Google Scholar 

  24. Hung Nguyen, V., Mazzamuto, F., Saint-Martin, J., Bournel, A., Dollfus, P.: Giant effect of negative differential conductance in graphene nanoribbon p-n heterojunctions. Appl. Phys. Lett. 99, 042105 (2011)

    Article  Google Scholar 

  25. Hung Nguyen, V., Mazzamuto, F., Saint-Martin, J., Bournel, A., Dollfus, P.: Graphene nanomesh-based devices exhibiting a strong negative differential conductance effect. Nanotechnology 23, 065201 (2012)

    Article  Google Scholar 

  26. Hung Nguyen, V., Niquet, Y.-M., Dollfus, P.: Gate-controllable negative differential conductance in graphene tunneling transistors. Semicond. Sci. Technol. 27, 105018 (2012)

    Article  Google Scholar 

  27. Giovannetti, G., Khomyakov, P.A., Brocks, G., Kelly, P.J., van den Brink, J.: Substrate-induced band gap in graphene on hexagonal boron nitride: ab initio density functional calculations. Phys. Rev. B 76, 073103 (2007)

    Article  Google Scholar 

  28. Kharche, N., Nayak, S.K.: Quasiparticle band gap engineering of graphene and graphone on hexagonal boron nitride substrate. Nano Lett. 11, 5274–5278 (2011)

    Article  Google Scholar 

  29. Xu, Y., Guo, Z., Chen, H., Yuan, Y., Lou, J., Lin, X., Gao, H., Chen, H., Yu, B.: In-plane and tunneling pressure sensors based on graphene/hexagonal boron nitride heterostructures. Appl. Phys. Lett. 99, 133109 (2011)

    Article  Google Scholar 

  30. Fan, Y., Zhao, M., Wang, Z., Zhang, X., Zhang, H.: Tunable electronic structures of graphene/boron nitride heterobilayers. Appl. Phys. Lett. 98, 083103 (2011)

    Article  Google Scholar 

  31. Zomer, P.J., Dash, S.P., Tombros, N., van Wees, B.J.: A transfer technique for high mobility graphene devices on commercially available hexagonal boron nitride. Appl. Phys. Lett. 99, 232104 (2011)

    Article  Google Scholar 

  32. Bai, J., Zhong, X., Jiang, S., Huang, Y., Duan, X.: Graphene nanomesh. Nat. Nanotechnol. 5, 190–194 (2010)

    Article  Google Scholar 

  33. Oswald, W., Wu, Z.: Energy gaps in graphene nanomeshes. Phys. Rev. B 85, 115431 (2012)

    Article  Google Scholar 

  34. Hung Nguyen, V., Chung Nguyen, M., Viet Nguyen, H., Dollfus, P.: Disorder effects on energy bandgap and electronic transport in graphene-nanomesh-based structures. J. Appl. Phys. 113, 013702 (2012)

    Article  Google Scholar 

  35. Yang, H.-X., Chshiev, M., Boukhvalov, D.W., Waintal, X., Roche, S.: Inducing and optimizing magnetism in graphene nanomeshes. Phys. Rev. B 84, 214404 (2011)

    Article  Google Scholar 

  36. Hung Nguyen, V., Nam Do, V., Bournel, A., Lien Nguyen, V., Dollfus, P.: Controllable spin-dependent transport in armchair graphene nanoribbon structures. J. Appl. Phys. 106, 053710 (2009)

    Article  Google Scholar 

  37. Reich, S., Maultzsch, J., Thomsen, C.: Tight-binding description of graphene. Phys. Rev. B 66, 035412 (2002)

    Article  Google Scholar 

  38. Castro Neto, A.H., Guinea, F., Peres, N.M.R., Novoselov, K.S., Geim, A.K.: The electronic properties of graphene. Rev. Mod. Phys. 81, 109–162 (2009)

    Article  Google Scholar 

  39. Son, Y.-W., Cohen, M.L., Louie, S.G.: Energy gaps in graphene nanoribbons. Phys. Rev. Lett. 97, 216803 (2006)

    Article  Google Scholar 

  40. Fiori, G., Iannaccone, G.: On the possibility of tunable-gap bilayer graphene FET. IEEE Electron Device Lett. 30, 261–264 (2009)

    Article  Google Scholar 

  41. Guo, J., Datta, S., Lundstrom, M., Anantram, M.P.: Towards multi-scale modeling of carbon nanotube transistors. Int. J. Multiscale Comput. Eng. 2, 257–260 (2004)

    Article  Google Scholar 

  42. Lopez Sancho, M.P., Lopez Sancho, J.M., Rubio, J.: Quick iterative scheme for the calculation of transfer matrices: application to Mo (100). J. Phys. F, Met. Phys. 14, 1205–1215 (1984)

    Article  Google Scholar 

  43. Anantram, M.P., Lundstrom, M.S., Nikonov, D.E.: Modeling of nanoscale devices. Proc. IEEE 96, 1511–1550 (2008)

    Article  Google Scholar 

  44. Ren, Z.: Nanoscale MOSFETs: physics, simulation, and design. Ph.D. Dissertation, Purdue University, West Lafayette, USA (2001)

  45. Huard, B., Sulpizio, J.A., Stander, N., Todd, K., Yang, B., Goldhaber-Gordon, D.: Transport measurements across a tunable potential barrier in graphene. Phys. Rev. Lett. 98, 236803 (2007)

    Article  Google Scholar 

  46. Brenner, K., Murali, R.: Single step, complementary doping of graphene. Appl. Phys. Lett. 96, 063104 (2010)

    Article  Google Scholar 

  47. Liu, G., Wu, Y., Lin, Y.-M., Farmer, D.B., Ott, J.A., Bruley, J., Grill, A., Avouris, P., Pfeiffer, D., Balandin, A.A., Dimitrakopoulos, C.: Epitaxial graphene nanoribbon array fabrication using BCP-assisted nanolithography. ACS Nano 6, 6786–6792 (2012)

    Article  Google Scholar 

  48. Liang, X., Jung, Y.-S., Wu, S., Ismach, A., Olynick, D.L., Cabrini, S., Bokor, J.: Formation of bandgap and subbands in graphene nanomeshes with sub-10 nm ribbon width fabricated via nanoimprint lithography. Nano Lett. 10, 2454–2460 (2010)

    Article  Google Scholar 

  49. Seabaugh, A.C., Zhang, Q.: Low-voltage tunnel transistors for beyond CMOS logic. Proc. IEEE 98, 2095–2110 (2010)

    Article  Google Scholar 

  50. Leburton, J.-P., Kolodzey, J., Briggs, S.: Bipolar tunneling field-effect transistor: a three terminal negative differential resistance device for high-speed applications. Appl. Phys. Lett. 52, 1608–1610 (1988)

    Article  Google Scholar 

  51. Omura, Y.: Negative conductance properties in extremely thin silicon-on-insulator (SOI) insulated-gate pn-junction devices (SOI surface tunnel transistors). Jpn. J. Appl. Phys. 35, L1401–L1403 (1996)

    Article  Google Scholar 

  52. Koga, J., Toriumi, A.: Three-terminal silicon surface junction tunneling device for room temperature operation. IEEE Electron Device Lett. 20, 529–531 (1999)

    Article  Google Scholar 

Download references

Acknowledgements

This work was partially supported by the French ANR through projects NANOSIM_GRAPHENE (ANR-09-NANO-016) and MIGRAQUEL (ANR-10-BLAN-0304). The work at Hanoi was supported by the Vietnamese National Foundation for Science and Technology Development (NAFOSTED) under Projects No. 103.02.64.09 and 103.02.76.09.

Author information

Authors and Affiliations

  1. Institute of Fundamental Electronics (IEF), CNRS, UMR 8622, Univ. Paris-Sud, Orsay, France

    Viet Hung Nguyen, Jérôme Saint-Martin, Damien Querlioz, Fulvio Mazzamuto, Arnaud Bournel & Philippe Dollfus

  2. Center for Computational Physics, Institute of Physics, Vietnam Academy of Science and Technology, Hanoi, Vietnam

    Viet Hung Nguyen

  3. L_Sim, SP2M, UMR-E CEA/UJF-Grenoble 1, INAC, Grenoble, France

    Viet Hung Nguyen & Yann-Michel Niquet

Authors
  1. Viet Hung Nguyen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jérôme Saint-Martin
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Damien Querlioz
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Fulvio Mazzamuto
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Arnaud Bournel
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Yann-Michel Niquet
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Philippe Dollfus
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Philippe Dollfus.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Hung Nguyen, V., Saint-Martin, J., Querlioz, D. et al. Bandgap nanoengineering of graphene tunnel diodes and tunnel transistors to control the negative differential resistance. J Comput Electron 12, 85–93 (2013). https://doi.org/10.1007/s10825-013-0434-2

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10825-013-0434-2

Keywords

Search

Navigation