Abstract
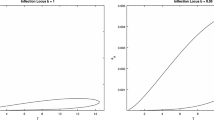
The nonbarochronic regular partially invariant submodel of the equations of gas dynamics is studied. The submodel reduces to an implicit ordinary differential equation of the first order for an auxiliary function X = X(x). The physical quantities (velocity, density, and pressure) are expressed in terms of the function X. The properties of the solutions of the equation are investigated and interpreted physically in terms of gas motion. The existence of a shock-wave solution is proved. The properties of the shock adiabat are studied. It is shown that the results obtained are new and differ significantly from the results for the case of no constant force.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
L. V. Ovsiannikov, Group Analysis of Differential Equations, Academic Press, New York (1982).
L. V. Ovsyannikov, Lectures on the Fundamentals of Gas Dynamics [in Russian], Nauka, Moscow (1981).
A. P. Chupakhin, “Nonbarochronic submodels of types (1, 2) and (1, 1) of the equations of gas dynamics,” Preprint No. 1-99, Institute of Hydrodynamics, Siberian Division, Russian Academy of Sciences, Novosibirsk (1999).
K. P. Stanyukovich, Unsteady Motion of Continuous Media, Pergamon Press, New York (1960).
V. I. Arnold, Geometrical Methods in the Theory of Ordinary Differential Equations, Springer-Verlag, New York-Heidelberg-Berlin (1983).
V. V. Prasolov, Polynomials, Springer, Berlin (2004).
A. O. Remizov, “On regular singular points of ordinary differential equations that are not resolved for the derivative,” Differ. Uravn., 38, 1053–1062 (2002).
A. V. Pkhakadze and A. A. Shestakov, “Classiffication of the singular points of a first-order differential equation that is not resolved for the derivative,” Mat. Sb., 49, No. 1, 1–7 (1959).
P. Hartman, Ordinary Differential Equations, Wiley, New York (1964).
A. F. Andreev, Singular Points of Differential Equations [in Russian], Vysheyshaya Shkola, Minsk (1979).
A. P. Chupakhin, “Self-conjugation of solutions via a shock wave: Limiting shock,” J. Appl. Mekh. Tech. Phys., 44, No. 3, 324–335 (2003).
Author information
Authors and Affiliations
Additional information
__________
Translated from Prikladnaya Mekhanika i Tekhnicheskaya Fizika, Vol. 47, No. 6, pp. 3–16, November–December, 2006.
Rights and permissions
About this article
Cite this article
Parshin, D.V., Chupakhin, A.P. On a gas source in a constant force field. J Appl Mech Tech Phys 47, 773–784 (2006). https://doi.org/10.1007/s10808-006-0115-z
Received:
Issue Date:
DOI: https://doi.org/10.1007/s10808-006-0115-z