Skip to main content
SpringerLink
Log in

Longitudinal Vibrations of Cantilever Rods: Determination of Mechanical Quantities

  • Published:
International Applied Mechanics Aims and scope

Abstract

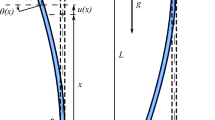
Mechanical vibrating systems described by a one-dimensional wave equation are considered. Solutions are found in the form of Fourier series with terms decreasing at a certain rate. A relationship between the rate of convergence of the series and the intensity of the initial statical load is established. An approach is proposed to estimate the necessary number of terms, which is consistent with the degree of validity of the mathematical model. A method to minimize the error due to the Gibbs phenomenon for discontinuous periodic processes is justified

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

REFERENCES

  1. V. S. Vladimirov, Equations of Mathematical Physics [in Russian], Nauka, Moscow (1976).

    Google Scholar 

  2. V. T. Grinchenko, Equilibrium and Steady-State Vibrations of Finite Elastic Bodies [in Russian], Naukova Dumka, Kiev (1978).

    Google Scholar 

  3. V. P. Zhuravlev and D. M. Klimov, Solid-State Wave Gyroscope [in Russian], Nauka, Moscow (1985).

    Google Scholar 

  4. A. M. Kurzanov, “Seismic design of buildings using the traveling-wave model,” Prom. Grazhd. Stroit., No. 6, 53–55 (1996).

  5. M. P. Plakhtienko, “Some questions of developing International Seismic Design Standards for the CIS Countries,” Budivn. Standartiz., No. 1, 2–8 (2001).

  6. S. B. Smirnov, “Features of the operation and strength design of buildings subject to impulsive seismic loads,” Zhilishchn. Stroit., No. 3, 14–16 (1995).

  7. Building Regulations: Building in Earthquake Areas (SNiP-II-7-81) [in Russian], Minstroi Rossii, Moscow (1995).

  8. S. P. Timoshenko, Vibrations in Engineering [in Russian], Fizmatgiz, Moscow (1967).

    Google Scholar 

  9. M. M. Filonenko-Borodich, Theory of Elasticity [in Russian], Fizmatgiz, Moscow (1959).

    Google Scholar 

  10. G. M. Fikhtengol’ts, A Course of Differential and Integral Calculus [in Russian], Vol. 3, Nauka, Moscow (1969).

    Google Scholar 

  11. S. E. Frish and A. V. Timoreva, Electric and Electromagnetic Phenomena, Vol. 2. of the three-volume series A Course of General Physics [in Russian], GITTL, Moscow (1973).

    Google Scholar 

  12. A. N. Guz, V. D. Kubenko, and A. E. Babaev, “Dynamics of shell systems interacting with a liquid,” Int. Appl. Mech., 38, No.3, 260–301 (2002).

    Article  Google Scholar 

  13. I. A. Guz and J. J. Rushchitsky, “Comparing the evolution characteristics of wave in nonlinearly elastic micro-and nanocomposites with carbon fillers,” Int. Appl. Mech., 40, No.7, 785–793 (2004).

    Article  Google Scholar 

  14. P. Z. Lugovoi, “Propagation of harmonic waves in an orthotropic cylindrical shell on elastic foundation,” Int. Appl. Mech., 40, No.3, 297–303 (2004).

    Article  Google Scholar 

  15. N. A. Shul’ga and T. V. Ratushnyak, “Oscillation modes of magnetoelastic Love-type waves in periodic ferrite-dielectric media,” Int. Appl. Mech., 40, No.8, 886–892 (2004).

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. S. P. Timoshenko Institute of Mechanics, National Academy of Sciences of Ukraine, Kiev, Ukraine

    N. P. Plakhtienko

Authors
  1. N. P. Plakhtienko
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

__________

Translated from Prikladnaya Mekhanika,Vol. 41, No. 5, pp.131–140, May 2005.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Plakhtienko, N.P. Longitudinal Vibrations of Cantilever Rods: Determination of Mechanical Quantities. Int Appl Mech 41, 573–581 (2005). https://doi.org/10.1007/s10778-005-0126-0

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10778-005-0126-0

Keywords

Search

Navigation