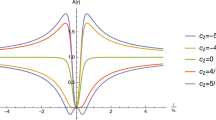
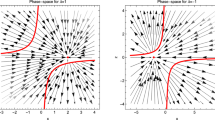
Abstract
In this paper, we will discuss a simple non-Abelian gauge theory in the broken Lorentz spacetime background. We will study the partial breaking of Lorentz symmetry down to its sub-group. We will use the formalism of very special relativity for analysing this non-Abelian gauge theory. Moreover, we will discuss the quantisation of this theory using the BRST symmetry. Also, we will analyse this theory in the maximal Abelian gauge.
Similar content being viewed by others
References
’t Hooft, G.: Quantization of point particles in (2 + 1)-dimensional gravity and space-time discreteness, Class. Quant. Grav. 13, 1023 (1996)
Amelino-Camelia, G., Ellis, J.R., Mavromatos, N.E., Nanopoulos, D.V., Sarkar, S.: Tests of quantum gravity from observations of gamma-ray bursts. Nature 393, 763 (1998)
Gambini, R., Pullin, J.: Nonstandard optics from quantum space-time. Phys. Rev. D 59, 124021 (1999)
Carroll, S.M., Harvey, J.A., Kostelecky, V.A., Lane, C. D., Okamoto, T.: Noncommutative field theory and Lorentz violation. Phys. Rev. Lett. 87, 141601 (2001)
Horava, P.: Quantum gravity at a lifshitz point. Phys. Rev. D 79, 084008 (2009)
Chakravarty, S., Dasgupta, K., Ganor, O.J., Rajesh, G.: Pinned branes and new nonLorentz invariant theories. Nucl. Phys. B 587, 228 (2000)
Kostelecky, V.A., Samuel, S.: Gravitational phenomenology in higher dimensional theories and strings. Phys. Rev. D 40, 1886 (1989)
Cohen, A.G., Glashow, S.L.: Very special relativity. Phys. Rev. Lett. 97, 021601 (2006)
Sheikh-Jabbari, M.M., Tureanu, A.: Realization of Cohen-Glashow very special relativity on noncommutative space-time. Phys. Rev. Lett. 101, 261601 (2008)
Cohen, A.G., Freedman, D.Z.: SIM(2) and SUSY. JHEP 0707, 039 (2007)
Petras, S., von Unge, R., Vohanka, J.: SIM(2) and supergraphs. JHEP 1107, 015 (2011)
Vohanka, J.: Gauge theory and SIM(2) superspace. Phys. Rev. D 85, 105009 (2012)
Vohánka, J., Faizal, M.: Chern–simons theory in SIM(1) superspace. Eur. Phys. J. C 75(12), 592 (2015)
Vohánka, J., Faizal, M.: Supersymmetric Chern–Simons theory in presence of a boundary in the light-like direction. Nucl. Phys. B 904, 327 (2016)
Vohánka, J., Faizal, M.: Super-Yang-Mills theory in SIM(1) superspace. Phys. Rev. D 91(4), 045015 (2015)
Kouretsis, A.P., Stathakopoulos, M., Stavrinos, P.C.: The general very special relativity in finsler cosmology. Phys. Rev. D 79, 104011 (2009)
Muck, W.: Very special relativity in curved Space-Times. Phys. Lett. B 670, 95 (2008)
Shah, M.B., Ganai, P.A.: A study of gaugeon formalism for QED in lorentz violating background. Commun. Theor. Phys. 69(2), 166 (2018)
Masood, S., Shah, M.B., Ganai, P.A.: Spontaneous symmetry breaking in Lorentz violating background. Int. J. Geom. Meth. Mod. Phys. 15(02), 1850021 (2017)
Shah, M.B., Ganai, P.A.: Quantum gauge freedom in the Lorentz violating background. Int. J. Geom. Meth. Mod. Phys. 15(01), 1850009 (2017)
Bufalo, R., Upadhyay, S.: Axion mass bound in very special relativity. Phys. Lett. B 772, 420 (2017)
Upadhyay, S.: Reducible gauge theories in very special relativity. Eur. Phys. J. C 75(12), 593 (2015)
Ahluwalia, D.V., Horvath, S.P.: Very special relativity as relativity of dark matter: The Elko connection. JHEP 1011, 078 (2010)
Chang, Z., Li, M.H., Li, X., Wang, S.: Cosmological model with local symmetry of very special relativity and constraints on it from supernovae. Eur. Phys. J. C 73(6), 2459 (2013)
Alfaro, J., Rivelles, V.O.: Non Abelian fields in very special relativity. Phys. Rev. D 88, 085023 (2013)
Alfaro, J., González, P., Ávila, R.: Electroweak standard model with very special relativity. Phys. Rev. D 91, 105007 (2015)
Alfaro, J., González, P., Ávila, R.: Addendum: Phys. Rev. D 91(12), 129904 (2015)
Becchi, C., Rouet, A., Stora, R.: Renormalization of gauge theories. Annals Phys. 98, 287 (1976)
Tyutin, I.V.: Gauge invariance in field theory and statistical physics in operator formalism
Costa, G., Julve, J., Marinucci, T., Tonin, M.: Nonabelian gauge theories and triangle anomalies. Nuovo Cim. A 38, 373 (1977)
Kugo, T., Ojima, I.: Manifestly covariant canonical formulation of Yang-Mills field theories: Physical state subsidiary conditions and physical s matrix unitarity. Phys. Lett. 73B, 459 (1978)
Hata, H., Kugo, T.: Subsidiary conditions and physical s matrix unitarity in covariant canonical formulation of supergravity. Nucl. Phys. B 158, 357 (1979)
Faizal, M., Khan, M.: A superspace formulation of the BV action for higher derivative theories. Eur. Phys. J. C 71, 1603 (2011)
Baulieu, L., Thierry-Mieg, J.: The principle of BRS symmetry: an alternative approach to Yang-Mills theories. Nucl. Phys. B 197, 477 (1982)
Azevedo, T., Jusinskas, R.L.: Background constraints in the infinite tension limit of the heterotic string. JHEP 1608, 133 (2016)
Mafra, C.R., Schlotterer, O.: One-loop superstring six-point amplitudes and anomalies in pure spinor superspace. JHEP 1604, 148 (2016)
Jusinskas, R.L.: On the field-antifield (a)symmetry of the pure spinor superstring. JHEP 1512, 136 (2015)
Jusinskas, R.L.: Notes on the ambitwistor pure spinor string. JHEP 1605, 116 (2016)
Oda, I.: Covariant matrix model of superparticle in the pure spinor formalism. Mod. Phys. Lett. A 18, 1023 (2003)
Faizal, M.: Aspects of ABJ theory. JHEP 1301, 156 (2013)
Faizal, M.: Chern-Simons-Matter theory. Int. J. Mod. Phys. A 28, 1350012 (2013)
Faizal, M., Upadhyay, S., Mandal, B.P.: IR Finite graviton propagators in de Sitter spacetime. Eur. Phys. J. C 76(4), 189 (2016)
Chang, L.N., Soo, C.p.: BRST cohomology and invariants of 4-D gravity in Ashtekar variables. Phys. Rev. D 46, 4257 (1992)
Kachru, S.: Extra states symmetries in D < 2 closed string theory. Nucl. Phys. B 390, 173 (1993)
Terao, H.: Quantum analysis of Jackiw and Teitelboim’s model for (1 + 1)-D gravity and topological gauge theory. Nucl. Phys. B 395, 623 (1993)
Lee, H.Y., Nakamichi, A., Ueno, T.: Topological two form gravity in four-dimensions. Phys. Rev. D 47, 1563 (1993)
Ohta, N., Suzuki, H.: Interactions of discrete states with nonzero ghost number in c = 1 2-D gravity. Mod. Phys. Lett. A 7, 2723 (1992)
Anselmi, D., Fre, P.: Twisted N = 2 supergravity as topological gravity in four-dimensions. Nucl. Phys. B 392, 401 (1993)
Faizal, M.: Noncommutativity and non-anticommutativity in perturbative quantum gravity. Mod. Phys. Lett. A 27, 1250075 (2012)
Faizal, M.: Noncommutative quantum gravity. Mod. Phys. Lett. A 28, 1350034 (2013)
Faizal, M.: Noether‘s charge in the super-group field cosmology. Grav. Cosmol. 20(2), 132 (2014)
Faizal, M.: Absence of black holes information paradox in group field cosmology. Int. J. Geom. Meth. Mod. Phys. 11, 1450010 (2014)
Faizal, M.: Super-group field cosmology. Class. Quant. Grav. 29, 215009 (2012)
Troost, J.: Massless particles on supergroups and A d S 3 x S 3 supergravity. JHEP 1107, 042 (2011)
Faizal, M.: The BV formalization of Chern-Simons theory on deformed superspace. Commun. Theor. Phys. 58, 704 (2012)
Banerjee, R., Deguchi, S.: A superspace formulation of Yang-Mills theory on sphere. J. Math. Phys. 51, 052301 (2010)
Ulker, K.: N = 2 SYM action as a BRST exact term, topological Yang-Mills and instantons. Phys. Rev. D 68, 085005 (2003)
Shah, M.B., Faizal, M., Ganai, P.A., Zaz, Z., Bhat, A., Masood, S.: Boundary effects in Super-Yang–Mills theory. Eur. Phys. J. C 77(5), 309 (2017)
Lehum, A.C., Nascimento, J.R., Petrov A.Y., da Silva, A. J.: Supergauge theories in aether superspace. Phys. Rev. D 88, 045022 (2013)
Weinreb, P., Faizal, M.: Generalized Faddeev–Popov method for a deformed supersymmetric Yang–Mills theory. Phys. Lett. B 748, 102 (2015)
Upadhyay, S., Panigrahi, P.K.: Quantum gauge freedom in very special relativity. Nucl. Phys. B 915, 168 (2017)
Upadhyay, S., Shah, M.B., Ganai, P.A.: Lorentz violating p-form gauge theories in superspace. Eur. Phys. J. C 77(3), 157 (2017)
Capri, M.A.L., Sobreiro, R.F., Sorella, S. P.: Interpolating among the Landau, Coulomb and maximal Abelian gauges. Phys. Rev. D 73, 041701 (2006)
Dudal, D., Gracey, J.A., Lemes, V.E.R., Sarandy, M.S., Sobreiro, R.F., Sorella, S.P., Verschelde, H.: An analytic study of the off-diagonal mass generation for Yang-Mills theories in the maximal Abelian gauge. Phys. Rev. D 70, 114038 (2004)
Maldacena, J.M.: The large N limit of superconformal field theories and supergravity. Int. J. Theor. Phys. 38, 1113 (1999)
Maldacena, J.M.: Adv. Theor. Math. Phys. 2, 231 (1998)
Gustavsson, A.: Selfdual strings and loop space Nahm equations. JHEP 0804, 083 (2008)
Bagger, J., Lambert, N.: Comments on multiple M2-branes. JHEP 0802, 105 (2008)
Bagger, J., Lambert, N.: Gauge symmetry and supersymmetry of multiple M2-branes. Phys. Rev. D 77, 065008 (2008)
Faizal, M.: Deformation of the ABJM theory. Europhys. Lett. 98, 31003 (2012)
Aharony, O., Bergman, O., Jafferis, D.L., Maldacena, J.: N = 6 superconformal Chern-Simons-matter theories, M2-branes and their gravity duals. JHEP 0810, 091 (2008)
Aharony, O., Bergman, O., Jafferis, D.L.: Fractional M2-branes. JHEP 0811, 043 (2008)
Faizal, M.: Non-anticommutative ABJ theory. Nucl. Phys. B 869, 598 (2013)
Queiruga, J.M., Lehum, A.C., Faizal, M.: Kählerian effective potentials for Chern–Simons-matter theories. Nucl. Phys. B 902, 58 (2016)
Antonyan, E., Tseytlin, A.A.: On 3d N = 8 Lorentzian BLG theory as a scaling limit of 3d superconformal N = 6 ABJM theory. Phys. Rev. D 79, 046002 (2009)
Berman, D.S., Perry, M.J., Sezgin, E., Thompson, D.C.: Boundary conditions for interacting membranes. JHEP 1004, 025 (2010)
Aprile, F., Niarchos, V.: \(\mathcal {N} = 2\) supersymmetric field theories on 3-manifolds with A-type boundaries. JHEP 1607, 126 (2016)
Faizal, M.: Gauge and supersymmetric invariance of a boundary Bagger-Lambert-Gustavsson theory. JHEP 1204, 017 (2012)
Belyaev, D.V., van Nieuwenhuizen, P.: Simple d = 4 supergravity with a boundary. JHEP 0809, 069 (2008)
Faizal, M., Luo, Y., Smith, D.J., Tan, M.C., Zhao, Q.: Gauge and supersymmetry invariance of N = 2 boundary Chern–Simons theory. Nucl. Phys. B 914, 577 (2017)
Faizal, M.: Boundary effects in the BLG theory. Mod. Phys. Lett. A 29(31), 1450154 (2014)
Berman, D.S., Thompson, D.C.: Membranes with a boundary. Nucl. Phys. B 820, 503 (2009)
Faizal, M., Smith, D.J.: Nonanticommutativity in the presence of a boundary. Phys. Rev. D 87(2), 025019 (2013)
Bilal, A.: Supersymmetric boundaries and junctions in four dimensions. JHEP 1111, 046 (2011)
Belyaev, D.V., Pugh, T.G.: The Supermultiplet of boundary conditions in supergravity. JHEP 1010, 031 (2010)
Faizal, M., Awad, A.: Four dimensional supersymmetric theories in presence of a boundary. Phys. Lett. B 748, 414 (2015)
Belyaev, D.V., van Nieuwenhuizen, P.: Rigid supersymmetry with boundaries. JHEP 0804, 008 (2008)
Gribov, V.N.: Quantization of nonabelian gauge theories. Nucl. Phys. B 139, 1 (1978)
Singer, I.M.: Some remarks on the gribov ambiguity. Commun. Math. Phys. 60, 7 (1978)
Shigemoto, K.: Field strength method and Gribov ambiguity in two-dimensional Nonabelian gauge theory. Lett. Nuovo Cim. 24, 495 (1979)
Killingback, T.P.: The gribov ambiguity in gauge theories on the 4 torus. Phys. Lett. 138B, 87 (1984)
Zwanziger, D.: Nonperturbative modification of the Faddeev-popov formula and banishment of the naive vacuum. Nucl. Phys. B 209, 336 (1982)
Zwanziger, D.: Local and renormalizable action from the gribov horizon. Nucl. Phys. B 323, 513 (1989)
Capri, M. A. L., Dudal, D., Pereira, A. D., Fiorentini, D., Guimaraes, M.S., Mintz, B.W., Palhares, L.F., Sorella, S.P.: Nonperturbative aspects of Euclidean Yang-Mills theories in linear covariant gauges: Nielsen identities and a BRST-invariant two-point correlation function. Phys. Rev. D 95(4), 045011 (2017)
Zwanziger, D.: Action from the gribov horizon. Nucl. Phys. B 321, 591 (1989)
Guimaraes, M.S., Pereira, A.D., Sorella, S.P.: Remarks on the effects of the Gribov copies on the infrared behavior of higher dimensional Yang-Mills theory. Phys. Rev. D 94(11), 116011 (2016)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Ganai, P.A., Shah, M.B., Syed, M. et al. Non-Abelian Gauge Theory in the Lorentz Violating Background. Int J Theor Phys 57, 1974–1982 (2018). https://doi.org/10.1007/s10773-018-3722-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10773-018-3722-6