Skip to main content
SpringerLink
Log in

Protection of Quantum Correlation Through the Quantum Erasing Effect

  • Published:
International Journal of Theoretical Physics Aims and scope Submit manuscript

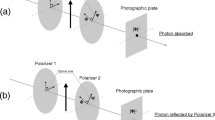
Abstract

By taking into account the quantum erasing effect(QEE), the quantum discord (QD) behavior of a two-qubit system with different initial states are investigated in detail. We find that the quantum correlation can be saved under a scheme of two spatially separated atoms, each located in a leaky cavity through the quantum erasing method. It is shown that QEE can weaken the effects of decoherence, and preserve the maximum information of the coherent item. No matter whether the two atoms are in the mixted or pure state, one can robusty save their initial quantum correlation even the number of erasing events is finite. If one limit the erasing events N → ∞, the QEE can be used to protect the initial quantum correlation independently of the state in which it is stored, the values of QD is always nearly equal to the initial QD values, and it is nearly independent of the decoherence, which imply us more encourage strategy for protecting the quantum correlation properties in some quantum systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Ollivier, H., Zurek, W.H.: Phys. Rev. Lett. 88, 017901 (2001)

    Article  ADS  Google Scholar 

  2. Quan, H.T., Song, Z., Liu, X.F., Zanardi, P., Sun, C.P.: Phys. Rev. Lett. 96, 140604 (2006)

    Article  ADS  Google Scholar 

  3. Cheng, W.W., Liu, J.M.: Phys. Rev. A 79, 052320 (2009)

    Article  ADS  Google Scholar 

  4. Bennett, C.H., Divincenzo, D.P., Fuchs, C.A., et al.: Phys. Rev. A 59, 1070 (1999)

    Article  ADS  MathSciNet  Google Scholar 

  5. Pan, J.W., Bouwmeester, D., Weinfurter, H., Zeilings, A.: Rev. Lett 80, 3891 (1998)

    Article  ADS  Google Scholar 

  6. Horodecki, M., Horodecki, P., Horodecki, R., et al.: Phys. Rev. A 71, 062307 (2005)

    Article  ADS  Google Scholar 

  7. Ollivier, H., Zurek, W.H.: Phys. Rev. Lett. 88, 017901 (2001)

    Article  ADS  Google Scholar 

  8. Henderson, L., Vedral, V.: J. Phys. Rev. A 34, 6899 (2001)

    ADS  MathSciNet  Google Scholar 

  9. Luo, S.: Phys. Rev. A 77, 022301 (2008)

    Article  ADS  Google Scholar 

  10. Dakic, B., Vedral, V., Brukner, C.: Phys. Rev. Lett. 105, 190502 (2010)

    Article  ADS  Google Scholar 

  11. Luo, S., Fu, S.: Phys. Rev. Lett. 106, 120401 (2011)

    Article  ADS  Google Scholar 

  12. Chen, L., Chitambar, E., Modi, K., Vacanti, G.: Phys. Rev. A 83, 020101 (2011)

    Article  ADS  Google Scholar 

  13. Datta, A., Shaji, A., Caves, C.M.: Phys. Rev. Lett. 100, 050502 (2008)

    Article  ADS  Google Scholar 

  14. Lanyon, B.P., Barbieri, M., Almeida, M.P., White, A.G.: Phys. Rev. Lett. 101, 200501 (2008)

    Article  ADS  Google Scholar 

  15. Datta, A., Gharibian, S.: Phys. Rev. A 79, 042325 (2009)

    Article  ADS  Google Scholar 

  16. Ekert, A., Macchiavello, C.: Phys. Rev. Lett. 77, 2585 (1996)

    Article  ADS  Google Scholar 

  17. Vitali, D., et al.: Phys. Rev. Lett. 79, 2442 (1997)

    Article  ADS  Google Scholar 

  18. Viola, L., Lloyd, S.: Phys. Rev. A 58, 2733 (1998)

    Article  ADS  MathSciNet  Google Scholar 

  19. Zhang, G., Keitel, C.H.: Phys. Rev. A 66, 022306 (2002)

    Article  ADS  MathSciNet  Google Scholar 

  20. Filip, R.: Phys. Rev. A 67, 014308 (2003)

    Article  ADS  Google Scholar 

  21. Xiang, S.H., Deng, X.P., Song, K.H.: Chin. Phy. Lett. 29, 050304 (2012)

    Article  ADS  Google Scholar 

  22. Wang, C.Z., Li, C.X., et al.: J. Phys. A, Math. Theor. 44, 015503 (2011)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Physics and Electronics Engineering, Shanxi University, Taiyuan, 030006, People’s Republic of China

    Hui-Yun Xu

  2. School of Physics and Information Engineering, Shanxi Normal University, Linfen, 041004, People’s Republic of China

    Guo-Hui Yang

Authors
  1. Hui-Yun Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Guo-Hui Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Guo-Hui Yang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Xu, HY., Yang, GH. Protection of Quantum Correlation Through the Quantum Erasing Effect. Int J Theor Phys 55, 2460–2468 (2016). https://doi.org/10.1007/s10773-015-2883-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10773-015-2883-9

Keywords

Search

Navigation