Skip to main content
SpringerLink
Log in

Aspects of Solutions of Massive Spin-3/2 Equation in Schwarzschild Space-Time

  • Published:
International Journal of Theoretical Physics Aims and scope Submit manuscript

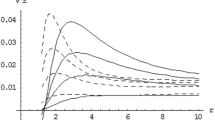
Abstract

The general massive spin-(3/2) (Rarita–Schwinger) field equation in Schwarzschild geometry, previously separated by variable separation, is further studied. The orthogonality of the solutions of the angular equations is exploited. The study of the radial equations, that are proposed in the most detailed form, is reduced to the study of four coupled differential equations. The equations are discussed and integrated near the Schwarzschild radius and for zero and large values of the radial coordinate. A covariant product of states is considered that is induced by a conserved current. It is shown the existence of states that are bound in the scalar product without implying the existence of a discrete energy spectrum.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Abramovitz, W., Stegun, I.E.: Handbook of Mathematical Functions. Dover, New York (1970)

    Google Scholar 

  2. Chandrasekhar, S.: The Mathematical Theory of Black Holes. Oxford University Press, New York (1983)

    MATH  Google Scholar 

  3. Hawking, S.W., Ellis, G.F.R.: The Large Scale Structure of Space-Time. Cambridge University Press, New York (1973)

    MATH  Google Scholar 

  4. Illge, R.: Massive fields of arbitrary spin in curved space-times. Commun. Math. Phys. 158, 433 (1993)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  5. Illge, R., Schimming, R.: Consistent field equations for higher spin on curved spacetime. Ann. Phys. 8, 319 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  6. Newman, E.T., Penrose, R.: An approach to gravitational radiation by a method of spin coefficients. J. Math. Phys. 3, 566 (1962)

    Article  ADS  MathSciNet  Google Scholar 

  7. Zecca, A.: Scalar field equation in Schwarzschild space-time. Nuovo Cimento B 625, 167 (2000)

    Google Scholar 

  8. Zecca, A.: Separation of massive field equations of arbitrary spin in Robertson–Walker space-time. Nuovo Cimento B 121, 167 (2006)

    ADS  MathSciNet  Google Scholar 

  9. Zecca, A.: Massive field equations of arbitrary spin in Schwarzschild geometry: separation induced by spin-3/2 case. Int. J. Theor. Phys. 45, 2241 (2006)

    MathSciNet  Google Scholar 

  10. Zecca, A.: Field equations of arbitrary spin in space-time with torsion. Int. J. Theor. Phys. 46, 1045 (2007). doi: 10.1007/s10773-006-9258-1

    Article  MATH  MathSciNet  Google Scholar 

  11. Zecca, A.: Bound states of spin 1 equation in Schwarzschild space-time. Nuovo Cimento B (2007). doi: 10.1393/ncb/i2006-10163-1

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Fisica dell’Università, Via Celoria 16, 20133, Milano, Italy

    Antonio Zecca

  2. Istituto Nazionale di Fisica Nucleare, Sezione di Milano, Milano, Italy

    Antonio Zecca

  3. Gruppo Nazionale per la Fisica Matematica, Sesto Fiozentino (Fi), Italy

    Antonio Zecca

Authors
  1. Antonio Zecca
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Antonio Zecca.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zecca, A. Aspects of Solutions of Massive Spin-3/2 Equation in Schwarzschild Space-Time. Int J Theor Phys 46, 3060–3066 (2007). https://doi.org/10.1007/s10773-007-9419-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10773-007-9419-x

Keywords

Search

Navigation