Abstract
We study the phenomena of superradiance for F(R)-Maxwell black holes in an AdS space-time. The AdS boundary plays the role of a mirror and provides a natural confining system that makes the superradiant waves bouncing back and forth between the region near the horizon and the reflective boundary, causing a possible superradiant instability. We obtain numerical solutions for static hairy black holes in this scenario and investigate their instability and explicitly address the stability of such solutions for spherical perturbations under specific conditions for the scalar charge and AdS radius. It is shown that for a small scalar charge or AdS radius the static hairy solution is stable under spherical perturbations. We conclude that under such conditions, new hairy black holes emerge as a possible endpoint of superradiant instability of the system.












Similar content being viewed by others
Notes
Given that the charge Q is constrained by the positive definiteness of Hawking temperature \(T_{H}=\frac{N'(r_{h})}{4\pi }=\frac{1}{4\pi }\left[ \frac{1}{r_{h}}-\frac{Q^2}{(1+f_{R}(R_{0}))r_{h}^3}- \frac{R_{0}r_{h}}{4}\right] \), then \(Q\le \sqrt{(1+f_{R}(R_{0}))(r_{h}^2+\frac{3r_{h}^4}{L^2})}\equiv Q_{c}\), where \(Q_{c}\) is the critical charge [51, 61]. For a small black hole \(\frac{r_{h}}{L} \ll 1\) and the critical charge becomes \(Q_{c}=\sqrt{(1+f_{R}(R_{0}))}r_{h}(1+\frac{3r_{h}^2}{2L^2})\ll L\).
Due to the behavior of the radial function near the horizon (15), an observer far from the black hole could see that the waves are coming out from the black hole provided that \(\omega \) satisfies superradiant condition (16). In contrast, due to the negative group velocity, a local observer of the black hole sees only waves going into the horizon and therefore the boundary condition is satisfied [61].
Note that the superradiant condition is given by \( \omega r_{h}-q Q< 0\) for the RN black hole.
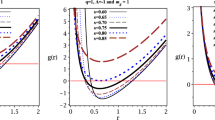
We construct hairy black hole solutions near the critical frequency (\(\omega _{c}\)) which is the onset of superradiant instability [34], since it seems sensible to expect superradiant instability to end as a hairy black hole.
The hairy black hole for which the scalar field has only one node (\(n=0\)) at the AdS boundary is described as a weakly interacting mix of a RNAdS black hole and a condensate of the ground state of the scalar field [65].
The solutions with more nodes correspond to weakly interacting mix of the RNAdS black hole and an excited state of the scalar field. They are unstable and presumably decay to the ground state of the hairy black hole [65].
References
Townsend, P.K.: Lect. Notes Phys. arXiv:9707012 [gr-qc]
Herdeiro, C.A., Radu, E.: Int. J. Mod. Phys. D 24, 1542014 (2015). arXiv:1504.08209 [gr-qc]
Schwarzschild, K.: Math. phys. 189 (1916). arXiv:9905030 [physics]
Bicak, J.: Math. Phys. 2, 165 (2006). arXiv:0604102 [gr-qc]
Ruffini, R., Wheeler, J.A.: Phys. Today 24, 30 (1971)
Israel, W.: Phys. Rev. 164, 1776 (1967)
Volkov, M.S.: arXiv:1601.08230 [gr-qc]
Volkov, M.S., Gal’Tsov, D.V.: JETP Lett. 50, 346 (1989)
Volkov, M.S., Gal’Tsov, D.V.: Sov. J. Nucl. Phys. 51, 747 (1990)
Dolan, S.R., Ponglertsakul, S., Winstanley, E.: Phys. Rev. D 92, 124047 (2015). arXiv:1507.02156 [gr-qc]
Nolan, B.C., Winstanley, E.: Class. Quant. Grav. 29, 235024 (2012). arXiv:1208.3589 [gr-qc]
Bekenstein, J.D.: Ann. Phys. 82, 535 (1974)
Bekenstein, J.D.: Ann. Phys. 91, 75 (1975)
Sudarsky, D., Zannias, T.: Phys. Rev. D 58, 087502 (1998). arXiv:9712083 [gr-qc]
Brito, R., Cardoso, V., Pani, P.: Lect. Notes Phys. 906 (2015). arXiv:1501.06570 [gr-qc]
Klein, O.: Zeitschrift für Phys. 53, 157 (1929)
Calogeracos, A., Dombey, N.: Contemp. Phys. 40, 313 (1999). arXiv:9905076 [quant-ph]
Brito, R., Cardoso, V., Pani, P.: Phys. Rev. D 89, 104045 (2014). arXiv:1405.2098 [gr-qc]
Press, W.H., Teukolsky, S.A.: Nature 238, 211–212 (1972)
Cardoso, V.: Gen. Rel. Grav. 45, 2079 (2013). arXiv:1307.0038 [gr-qc]
Hod, S.: Phys. Lett. B 708, 320 (2012). arXiv:1205.1872 [gr-qc]
Hod, S.: Phys. Lett. B 758, 181 (2016). arXiv:1606.02306 [gr-qc]
Hod, S.: Phys. Rev. D 90, 024051 (2014). arXiv:1406.1179 [gr-qc]
Ponglertsakul, S., Winstanley, E.: Phys. Lett. B 764, 87 (2017). arXiv:1610.00135 [gr-qc]
Hod, S.: Phys. Rev. D 88, 064055 (2013). arXiv:1310.6101 [gr-qc]
Degollado, J.C., Herdeiro, C.A., Rúnarsson, H.F.: Phys. Rev. D 88, 063003 (2013). arXiv:1305.5513 [gr-qc]
Degollado, J.C., Herdeiro, C.A.: Phys. Rev. D 89, 063005 (2014). arXiv:1312.4579 [gr-qc]
Ganchev, B.: arXiv:1608.01798 [hep-th]
Cardoso, V., Dias, O.J.: Phys. Rev. D 70, 084011 (2004). arXiv:0405006 [hep-th]
Green, S.R., Hollands, S., Ishibashi, A., Wald, R.M.: Class. Quant. Grav. 33, 125022 (2016). arXiv:1512.02644 [gr-qc]
González, P.A., Papantonopoulos, E., Saavedra, J., Vásquez, Y.: Phys. Rev. D 95, 6 (2017). arXiv:1702.00439 [gr-qc]
Gual, N.S., Degollado, J.C., Montero, P.J., Font, J.A., Herdeiro, C.: Phys. Rev. Lett. 116, 141101 (2016). arXiv:1512.05358 [gr-qc]
Bosch, P., Green, S.R., Lehner, L.: Phys. Rev. Lett. 116, 141102 (2016). arXiv:1601.01384 [gr-qc]
Dias, O.J., Masachs, R.: JHEP 128, 2 (2017). arXiv:1610.03496 [hep-th]
Dias, O.J., Figueras, P., Minwalla, S., Mitra, P., Monteiro, R., Santos, J.E.: JHEP 117, 8 (2012). arXiv:1112.4447 [hep-th]
Sotiriou, T.P., Faraoni, V.: Rev. Mod. Phys. 82, 451 (2010). arXiv:0805.1726 [gr-qc]
Faraoni, V.: arXiv:0810.2602 [gr-qc]
De Felice, A., Tsujikawa, S.: Living Rev. Rel. 13, 3 (2010). arXiv:1002.4928 [gr-qc]
Zhang, Z., Ma, Y.: Phys. Rev. Lett. 106, 17 (2011). arXiv:1101.1752 [gr-qc]
Capozziello, S., Stabile, A., Troisi, A.: Phys. Rev. D 76, 104019 (2007). arXiv:0708.0723 [gr-qc]
Hendi, S.H., Eslam Panah, B.E., Mousavi, S.M.: Gen. Rel. Grav. 44, 835 (2012). arXiv:1102.0089 [hep-th]
Elizalde, E., Nojiri, S.L., Odintsov, S.D., Wang, P.: Phys. Rev. D 71, 103504 (2005). arXiv:0502082 [hep-th]
Myrzakulov, R., Sebastiani, L., Zerbini, S.: Int. J. Mod. Phys. D 22, 1330017 (2013). arXiv:1302.4646 [gr-qc]
Sebastiani, L., Zerbini, S.: Eur. Phys. J. C 71, 1591 (2011). arXiv:1012.5230 [gr-qc]
Multamäki, T., Vilja, I.: Phys. Rev. D 74, 064022 (2006). arXiv:0606373 [astro-ph]
Sotitiou, T.P.: PhD thesis. arXiv:0710.4438 [gr-qc]
Bazeia, D., Losano, L., Olmo, G.J., Rubiera-Garcia, D.: Phys. Rev. D 90, 4 (2014). arxiv:1405.0208 [hep-th]
Natsuume, M.: Book (903). Springer, Germany (2015). arXiv:1409.3575 [hep-th]
Herzog, C.P.: J. Phys. A 42, 34 (2009). arXiv:0904.1975 [hep-th]
Hartnoll, S.A.: Chapter of the book ‘Black Holes in Higher Dimensions’, arXiv:1106.4324 [hep-th]
Moon, T., Myung, Y.S., Son, E.J.: Gen. Rel. Grav. 43, 3079 (2011). arXiv:1101.1153 [gr-qc]
Cembranos, J.A.R., de la Cruz-Dombriz, A., Jimeno Romero, P.: Int. J. Geom. Meth. Mod. Phys. 11, 1450001 (2011). arXiv:1109.4519 [gr-qc]
Myung, Y.S.: Phys. Rev. D 88, 104017 (2013). arXiv:1309.3346 [gr-qc]
Myung, Y.S.: Phys. Rev. D 84, 024048 (2011). arXiv:1104.3180 [gr-qc]
Guarnizo, A.: M.Sc. Thesis. arXiv:1211.2444 [gr-qc]
Nojiri, S.I., Odintsov, S.D.: Phys. Rep. 505, 2–4 (2011). arXiv:1011.0544 [gr-qc]
Nojiri, S., Odintsov, S.D., Oikonomou, V.K.: Phys. Rept 692 (2017). arXiv:1705.11098 [gr-qc]
Faraoni, V.: Phys. Rev. D 74, 10 (2006). arXiv:0610734 [astro-ph]
de La Cruz-Dombriz, A., Dobado, A., Maroto, A.L.: Phys. Rev. D 80, 12 (2009). arXiv:0907.3872 [gr-qc]
Sheykhi, A.: Phys. Rev. D 86, 2 (2012). arXiv:1209.2960 [hep-th]
Uchikata, N., Yoshida, S.: Phys. Rev. D 83, 064020 (2011). arXiv:1109.6737 [gr-qc]
Horowitz, G.T.: Lect. notes. arXiv:1002.1722 [hep-th]
Huang, Y., Liu, D.J., Li, X.Z.: Int. J. Mod. Phys. D 26, 1750141 (2017). arXiv:1606.00100 [gr-qc]
Peng, Y., Wang, B., Liu, Y.: Eur. Phys. J. C 78, 3 (2018). arXiv:1708.01411 [hep-th]
Basu, P., Bhattacharya, J., Bhattacharyya, S., Loganayagam, R., Minwalla, S., Umesh, V.: JHEP 45, 10 (2010). arXiv:1003.3232 [hep-th]
Winstanley, E.: Phys. Rev. D 64, 104010 (2001). arXiv:0106032 [gr-qc]
Heisenberg, L., Tsujikawa, S.: Phys. Lett. B 780, 638 (2018). arXiv:1802.07035 [gr-qc]
Myung, Y.S., Moon, T., Son, E.J.: Phys. Rev. D 83, 12 (2011). arXiv:1103.0343 [gr-qc]
Myung, Y.S., Zou, D.C.: arXiv:1804.03003 [gr-qc]
Acknowledgements
M. Honardoost would like to thank Iran National Science Foundation (INSF) and Research Council of Shahid Beheshti University for financial support. We also thank M. Khodadi for a careful reading of the manuscript and helpful comments.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix A
By assuming constant curvature, the field equation (3) in vacuum becomes
The trace of (44) leads to
Now, substituting for f(R) in (45), one gets \(R_{0}=\pm \sqrt{3} \mu ^2 \) for \(f(R)=-\frac{\mu ^{4}}{R}\) and \(\lambda = \frac{R_{0} e^{\alpha R_{0}}}{2+\alpha R_{0}}\) for \(f(R)=-\lambda e^{-\alpha R}\), where \(\alpha \) is a free parameter, representing solutions which correspond to a topological Schwarzschild-AdS(dS) black hole. In the numerical solution, we fix \(R_{0}\) to get \(\mu ^{2}\) and \(\lambda \). In [59], by considering thermodynamics of black holes in an AdS space-time, the authors found that the necessary conditions for F(R) to support AdS black holes are
In addition, the condition \(\frac{d^{2}f}{dR^{2}}\) guarantees that there is no tachyonic instability. These conditions may impose extra restrictions on \(R_{0}\). In the first model, only \(R_{0}=\mp \sqrt{3} \mu ^2\) would allow compliance with a topological Schwarzschild-AdS black hole. However, in the second model with the assumption \(\alpha >0\), these conditions require \(-\frac{1}{\alpha }<R_{0}<0\).
Appendix B
Perturbations of dynamical equations (30–36), to first order, can be derived using definition of the perturbed quantities defined in the text with the result
The perturbed scalar field is a complex quantity, written as \(\delta \psi =\delta u+i \delta {\dot{w}}\). For the real part, we have
and the imaginary part takes on the form
Integration with respect to time of equations (51, 53) yields
where \(\delta F(r) \) and \(\delta g(r) \) are arbitrary functions of the radial coordinate. Integration of equation (56) now yields
where \(\delta \textit{H(r)}\) is an arbitrary function of the radial coordinate. If an arbitrary function of r is added to \(\delta w\), due to the form of \(\delta \psi \), it does not change which gives us the freedom to set \(\delta \textit{H(r)}=0\).
A first-order differential equation is constructed from (48) and (52), using equations (57–59)
Another first-order differential equation is obtained by plugging (48) and (52) in (57)
Substitution of (57) and (58) in (59) then gives
Let us now substitute equations (50, 57–59, 61) in (55) and obtain
Using equations (49, 58, 59) then results in
Since ingoing boundary conditions (40) must exist for all perturbations, including perturbation of metric variables (\(\delta N\) and \(\delta h\)) [10], equations (57) and (58) are needed to satisfy such conditions, leading to \(\delta \textit{F(r)}=0\) and \(\delta \textit{g(r)}=0\) at \(r=r_{h}\). Therefore, \(\delta \textit{F(r)}\), \(\delta \textit{g(r)}\), \(\delta \textit{F(r)}'\) and \(\delta \textit{g(r)}'\) are removed from perturbation equations and \(\delta R\) at \(r=r_{h}\) must be zero on account of equations (60, 61).
Rights and permissions
About this article
Cite this article
Rahmani, A., Honardoost, M. & Sepangi, H.R. Superradiant instability and asymptotically AdS hairy black holes in F(R)-charged scalar field theory. Gen Relativ Gravit 52, 53 (2020). https://doi.org/10.1007/s10714-020-02707-w
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10714-020-02707-w