Skip to main content
SpringerLink
Log in

Thermodynamic geodesics of a Reissner Nordström black hole

  • Research Article
  • Published:
General Relativity and Gravitation Aims and scope Submit manuscript

Abstract

Starting from a Geometrothermodynamics metric for the space of thermodynamic equilibrium states in the mass representation, we use numerical techniques to analyse the thermodynamic geodesics of a supermassive Reissner Nordström black hole in isolation. Appropriate constraints are obtained by taking into account the processes of Hawking radiation and Schwinger pair-production. We model the black hole in line with the work of Hiscock and Weems (Phys Rev D 41:1142–1151, 1990). It can be deduced that the relation which the geodesics establish between the entropy S and electric charge Q of the black hole extremises changes in the black hole’s mass. Indeed, the expression for the entropy of an extremal black hole is an exact solution to the geodesic equation. We also find that in certain cases, the geodesics describe the evolution brought about by the constant emission of Hawking radiation and charged-particle pairs.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Notes

  1. To be precise, Weinhold worked in the tangent space defined at a general point of the equilibrium manifold, although it is possible to use his metric as a measure of distance in the manifold itself [14].

  2. \(\wedge \) stands for the exterior product, ‘\(\text {d}\)’ the exterior derivative, and \((\text {d}\varTheta )^n\) is equal to \(\text {d}\varTheta \wedge \cdots \wedge \text {d}\varTheta \), where \(\text {d}\varTheta \) appears n times.

  3. The full value and its uncertainty, in SI units, are given in [40].

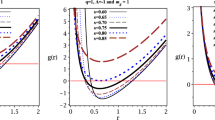
  4. Note that it is also possible to choose Q as the dependent variable. This will be treated in greater detail in Sect. 4.

  5. Two values are given in [1]—one for the emission of three massless neutrino species, and another (0.26792) valid when no massless neutrinos are produced, where by massless is meant a mass less than about \(10^{-10}\,\hbox {eV}\) (the black hole would be too cold to emit anything heavier) [1]. Since the upperbounds available nowadays for the mass of neutrino flavours [48] are much greater than this value, we use \(\alpha =0.26792\) when comparing our work with [1].

  6. The full values and uncertainties, in SI units, are given in [40].

  7. The rest energy of the positron (the particle with the same-sign charge as the black hole) is not taken into account; the large charge-to-mass ratio of this particle implies that the energy \(eQ/r_+\) it gains when repelled to infinity is much greater [1].

  8. Here, \(\lambda _\alpha \) stands for the intensive thermodynamic variables of the system. At equilibrium, \(\lambda _\alpha =\frac{\partial U}{\partial E^\alpha }\Bigr |_{\begin{array}{c} E_0^\alpha \end{array}}\) [52].

  9. Several authors are of the opinion that a black hole which is exactly extremal has zero entropy; see, for instance, [54,55,56,57,58].

  10. For instance, in the case of the geodesic with constraints given by Eq. (29), we set \(Q(t=0) = 8\times 10^{8}\,{\hbox {m}}\) and \(M(t=0) = 3\times 10^{9}\,{\hbox {m}}\).

References

  1. Hiscock, W.A., Weems, L.D.: Evolution of charged evaporating black holes. Phys. Rev. D 41, 1142–1151 (1990)

    Article  ADS  Google Scholar 

  2. Wheeler, J.A., Ruffini, R.: Introducing the black hole. Phys. Today 24(1), 30–41 (1971)

    Article  Google Scholar 

  3. Michell, J.: On the Means of discovering the Distance, Magnitude, etc. of the Fixed Stars. Philos. Trans. R. Soc. 74, 35–57 (1784)

    Article  Google Scholar 

  4. Gillispie, C.C.: Pierre-Simon Laplace, 1749–1827: A Life in Exact Science, Chapter 19. Princeton, Princeton University Press (1997). (reprinted in 2000)

    MATH  Google Scholar 

  5. Bekenstein, J.D.: Black holes and the second law. Lett. Nuovo Cim. 4, 737–740 (1972)

    Article  ADS  Google Scholar 

  6. Bardeen, J.M., Carter, B., Hawking, S.W.: The four laws of black hole mechanics. Commun. Math. Phys. 31, 161–170 (1973)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  7. Hawking, S.W.: Black hole explosions? Nature 248, 30–31 (1974)

    Article  ADS  Google Scholar 

  8. Quevedo, H.: Geometrothermodynamics. J. Math. Phys. 48, 013506 (2007)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  9. Quevedo, H., Quevedo, M.N.: Fundamentals of Geometrothermodynamics. In: Electronic Journal of Theoretical Physics—Zacatecas Proceedings II, pp. 1–16 (2011). Edited by Dvoeglazov, V.V., Molgado A., Ortiz, C. (Workshop Editors) and López Bonilla, J. L., Licata I., Sakaji, A. (EJTP Editors)

  10. Gibbs, J.W.: The Collected Works of J. Willard Gibbs, 1st edn. Yale University Press, New Haven (1948)

    MATH  Google Scholar 

  11. Carathéodory, C.: Untersuchungen über die Grundlagen der Thermodynamik. Math. Ann. 67, 355–386 (1909). English translation by Kestin, J.: Investigation into the foundations of thermodynamics. In: Kestin, J. (ed.) The Second Law of Thermodynamics, pp. 229–256. Dowden, Hutchinson and Ross, Stroudsburg (1976)

  12. Rao, C.R.: Information and accuracy attainable in the estimation of statistical parameters. Bull. Calcutta Math. Soc. 37(3), 81–91 (1945)

    MathSciNet  MATH  Google Scholar 

  13. Hermann, R.: Geometry, Physics, and Systems. M. Dekker, New York (1973)

    MATH  Google Scholar 

  14. Andresen, B., Berry, R.S., Gilmore, R., Ihrig, E., Salamon, P.: Thermodynamic geometry and the metrics of Weinhold and Gilmore. Phys. Rev. A 37, 845–848 (1988)

    Article  ADS  MathSciNet  Google Scholar 

  15. Weinhold, F.: Metric geometry of equilibrium thermodynamics. J. Chem. Phys. 63, 2479–2483 (1975)

  16. Weinhold, F.: Metric geometry of equilibrium thermodynamics. II. J. Chem. Phys. 63, 2484–2487 (1975)

  17. Weinhold, F.: Metric geometry of equilibrium thermodynamics. III. J. Chem. Phys. 63, 2488–2495 (1975)

  18. Weinhold, F.: Metric geometry of equilibrium thermodynamics. IV. J. Chem. Phys. 63, 2496–2501 (1975)

  19. Weinhold, F.: Metric geometry of equilibrium thermodynamics. V. J. Chem. Phys. 65, 559–564 (1976)

  20. Ruppeiner, G.: Thermodynamics: a Riemannian geometric model. Phys. Rev. A 20, 1608–1613 (1979)

    Article  ADS  Google Scholar 

  21. Salamon, P., Nulton, J., Ihrig, E.: On the relation between entropy and energy versions of thermodynamic length. J. Chem. Phys. 80, 436–437 (1984)

    Article  ADS  Google Scholar 

  22. Nulton, J., Salamon, P., Andresen, B., Anmin, Qi: Quasistatic processes as step equilibrations. J. Chem. Phys. 83, 334–338 (1985)

    Article  ADS  Google Scholar 

  23. Diósi, L., Kulacsy, K., Lukács, B., Rácz, A.: Thermodynamic length, time, speed, and optimum path to minimize entropy production. J. Chem. Phys. 105, 11220–11225 (1996)

    Article  ADS  Google Scholar 

  24. Crooks, G.E.: Measuring thermodynamic length. Phys. Rev. Lett 99, 100602 (2007)

    Article  ADS  Google Scholar 

  25. Quevedo, H., Sánchez, A.: Geometrothermodynamics of asymptotically anti-de Sitter black holes. J. High Energy Phys. 09, 034 (2008)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  26. Salamon, P., Ihrig, E., Berry, R.S.: A group of coordinate transformations which preserve the metric of Weinhold. J. Math. Phys. 24, 2515–2520 (1983)

    Article  ADS  MathSciNet  Google Scholar 

  27. Mrugala, R., Nulton, J.D., Schön, J.C., Salamon, P.: Statistical approach to the geometric structure of thermodynamics. Phys. Rev. A 41, 3156–3160 (1990)

    Article  ADS  MathSciNet  Google Scholar 

  28. Bravetti, A., Lopez-Monsalvo, C.S., Nettel, F., Quevedo, H.: The conformal metric structure of Geometrothermodynamics. J. Math. Phys. 54, 033513 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  29. Bravetti, A., Momeni, D., Myrzakulov, R., Quevedo, H.: Geometrothermodynamics of higher dimensional black holes. Gen. Relativ. Gravit. 45, 1603–1617 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  30. Quevedo, H., Sánchez, A., Vázquez, A.: Relativistic like structure of classical thermodynamics. Gen. Relativ. Gravit. 47, 36 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  31. Álvarez, J.L., Quevedo, H., Sánchez, A.: Unified geometric description of black hole thermodynamics. Phys. Rev. D 77, 084004 (2008)

    Article  ADS  MathSciNet  Google Scholar 

  32. Quevedo, H.: Geometrothermodynamics of black holes. Gen. Relativ. Gravit. 40, 971–984 (2008)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  33. Bravetti, A., Luongo, O.: Dark energy from geometrothermodynamics. Int. J. Geom. Methods Mod. Phys. 11(8), 1450071 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  34. Han, Y., Chen, G.: Thermodynamics, geometrothermodynamics and critical behavior of (2+1)-dimensional black holes. Phys. Lett. B 714, 127–130 (2012)

    Article  ADS  MathSciNet  Google Scholar 

  35. Bravetti, A., Lopez-Monsalvo, C.S., Nettel, F., Quevedo, H.: Representation invariant Geometrothermodynamics: applications to ordinary thermodynamic systems. J. Geom. Phys. 81, 1–9 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  36. Hendi, S.H., Panahiyan, S., Panah, B.E., Momennia, M.: A new approach toward geometrical concept of black hole thermodynamics. Eur. Phys. J. C 75, 507 (2015)

    Article  ADS  Google Scholar 

  37. Hendi, S.H., Panahiyan, S., Panah, B.E.: Charged black hole solutions in Gauss–Bonnet-massive gravity. J. High Energy Phys. 2016(1), 129 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  38. Hendi, S.H., Panahiyan, S., Panah, B.E., Armanfard, Z.: Phase transition of charged black holes in Brans–Dicke theory through geometrical thermodynamics. Eur. Phys. J. C 76, 396 (2016)

    Article  ADS  Google Scholar 

  39. Vázquez, A., Quevedo, H., Sánchez, A.: Thermodynamic systems as extremal hypersurfaces. J. Geom. Phys. 60, 1942–1949 (2010)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  40. The NIST Reference on Constants, Units, and Uncertainty. http://physics.nist.gov/cuu/index.html (2015). Accessed 2 Oct 2016

  41. Caprio, M.A.: LevelScheme: a level scheme drawing and scientific figure preparation system for Mathematica. Comput. Phys. Commun. 171, 107–118 (2005). http://scidraw.nd.edu/levelscheme

  42. Bekenstein, J.D.: Black holes and entropy. Phys. Rev. D 7, 2333–2346 (1973)

    Article  ADS  MathSciNet  Google Scholar 

  43. Hawking, S.W.: Particle creation by black holes. Commun. Math. Phys. 43, 199–220 (1975)

    Article  ADS  MathSciNet  Google Scholar 

  44. Smarr, L.: Mass formula for Kerr black holes. Phys. Rev. Lett. 30, 71–73 (1973)

    Article  ADS  Google Scholar 

  45. Gibbons, G.W.: Vacuum polarization and the spontaneous loss of charge by black holes. Commun. Math. Phys. 44, 245–264 (1975)

    Article  ADS  MathSciNet  Google Scholar 

  46. Schwinger, J.: On gauge invariance and vacuum polarization. Phys. Rev. 82, 664–679 (1951)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  47. Parentani, R., Spindel, P.: Hawking radiation. http://www.scholarpedia.org/article/Hawking_radiation (2011). Accessed 15 Jan 2016

  48. Particle Data Group: Leptons. Phys. Lett. B 667, 479–548 (2008)

    Article  ADS  Google Scholar 

  49. Hobson, M.P., Efstathiou, G., Lasenby, A.N.: General Relativity: An Introduction for Physicists, Chapter 11. Cambridge University Press, New York (2006)

    Book  MATH  Google Scholar 

  50. Thornton, S.T., Rex, A.: Modern Physics for Scientists and Engineers, Chapter 15, 4th edn. Cengage Learning, Boston (2013)

    Google Scholar 

  51. Walecka, J.D.: Introduction to General Relativity, Chapter 14. World Scientific, Singapore (2007)

    Book  MATH  Google Scholar 

  52. Gilmore, R.: Length and curvature in the geometry of thermodynamics. Phys. Rev. A 30, 1994–1997 (1984)

    Article  ADS  MathSciNet  Google Scholar 

  53. Salamon, P., Andresen, B., Gait, P.D., Berry, R.S.: The significance of Weinhold’s length. J. Chem. Phys. 73, 1001–1002 (1980). Erratum: J. Chem. Phys. 73, 5407 (1980)

  54. Hawking, S.W., Horowitz, G.T., Ross, S.F.: Entropy, area, and black hole pairs. Phys. Rev. D 51, 4302–4314 (1995)

    Article  ADS  MathSciNet  Google Scholar 

  55. Hod, S.: Evidence for a null entropy of extremal black holes. Phys. Rev. D 61, 084018 (2000)

    Article  ADS  MathSciNet  Google Scholar 

  56. Teitelboim, C.: Action and entropy of extreme and nonextreme black holes. Phys. Rev. D 51, 4315–4318 (1995)

    Article  ADS  MathSciNet  Google Scholar 

  57. Carroll, S.M., Johnson, M.C., Randall, L.: Extremal limits and black hole entropy. J. High Energy Phys. 11, 109 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  58. Das, S., Dasgupta, A., Ramadevi, P.: Can extremal black holes have nonzero entropy? Mod. Phys. Lett. A 12, 3067–3079 (1997)

    Article  ADS  MathSciNet  Google Scholar 

Download references

Acknowledgements

C. F. would like to thank Prof. H. Quevedo of the Universidad Nacional Autónoma de México, Prof. J. Muscat (Department of Mathematics, University of Malta) and Prof. K. Zarb Adami (Institute of Space Sciences and Astronomy, University of Malta).

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, University of Malta, Msida, MSD 2080, Malta

    Christine Farrugia & Joseph Sultana

Authors
  1. Christine Farrugia
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Joseph Sultana
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Christine Farrugia.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Farrugia, C., Sultana, J. Thermodynamic geodesics of a Reissner Nordström black hole. Gen Relativ Gravit 49, 4 (2017). https://doi.org/10.1007/s10714-016-2169-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10714-016-2169-4

Keywords

Search

Navigation