Abstract
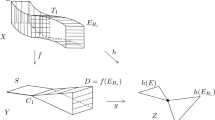
New historical aspects of the classification, by Cayley and Cremona, of ruled quartic surfaces and the relation to string models and plaster models are presented. In a ‘modern’ treatment of the classification of ruled quartic surfaces the classical one is corrected and completed. The string models of Series XIII of some ruled quartic surfaces (manufactured by L. Brill and by M. Schilling) are based on a result of Rohn concerning curves in \({\mathbb{P}^1\times \mathbb{P}^1}\) of bi-degree (2, 2). This is given here a conceptional proof.
Article PDF
Similar content being viewed by others
References
Barth W., Peters C., van der Ven A.: Compact complex surfaces. Springer, Berlin (1984)
Bottema O.: A classification of rational quartic ruled surfaces. Geometriae Dedicata 1(3), 349–355 (1973)
Cayley A.: A third memoir on skew surfaces, otherwise scrolls. Philos. Trans. R. Soc. Lond. 159, 111–126 (1869)
Chasles M.: Sur les six droites qui peuvent être les directions de six forces en équilibre. Propriétés de l’hyperboloïde à une nappe et d’une certaine surface du quatrième ordre. Comptes Rendus des Séances de l’Académie des Sciences. Paris 52, 1094–1104 (1861)
Cremona, L.: Sulle Superficie Gobbe di Quarto Grado. Memorie dell’ Accademia delle Science dell’ Istituto di Bologna serie II, tomo VIII (1868), 235–250. Opere II, 420
Dolgachev, I.V.: Topics in Classical Algebraic Geometry, http://www.math.lsa.umich.edu/~idolga/topics1.pdf
Edge W.L.: The theory of ruled surfaces. Cambridge University Press, Cambridge (1931)
Hartshorne R.: Algebraic geometry. Springer, Berlin (1977)
Meyer W.: Fr. Flächen vierter und höherer Ordnung. Encyklopädie der mathematischen Wissenschaften mit Einschluss ihrer Anwendungen IIIC 10b, 1744–1759 (1930)
Mohrmann H.: Die Flächen vierter Ordnung mit gewundener Doppelkurve. Mathematische Annalen 89, 1–31 (1923)
Pascal, E.: Repertorium der Höheren Mathematik (Definitionen, Formeln, Theoreme, Literatur). II. Theil: die Geometrie. Teubner (1902)
Rohn K.: Ueber die Flächen vierter Ordnung mit dreifachem Punkte. Mathematische Annalen 24(1), 55–151 (1884)
Rohn, K.: Die verschiedenen Arten der Regelflächen vierter Ordnung, (1886). Mathematische Abhandlungen aus dem Verlage Mathematischer Modelle von Martin Schilling. Halle a. S., 1904. Mathematische Annalen, vol. 28, No. 2, pp. 284–308 (1886)
Salmon G.: A treatise on the analytic geometry of three dimensions. Hodges, Figgis & Co., Dublin (1882)
Schilling, M.: Catalog mathematischer Modelle für den höheren mathematischen Unterrich. Verlag von Martin Schilling, Leipzig (1911)
Segre C.: Etude des différentes surfaces du 4e ordre à conique double ou cuspidale (générale ou décomposée) considérées comme des projections de l’intersection de deux variétés quadratiques de l’espace à quatre dimensions. Mathematische Annalen 24(3), 313–444 (1884)
Sturm R.: Die Gebilde ersten und zweiten Grades der Liniengeometrie in synthetischer Behandlung I. B.G. Teubner, Leipzig (1892)
Swinnerton-Dyer H.P.F.: An enumeration of all varieties of degree 4. Am J Math 95(2), 403–418 (1973)
Urabe T.: Dynkin graphs and combinations of singularities on quartic surfaces. Proc. Japan. Acad. Ser. A 61, 266–269 (1985)
Urabe T.: Classification of non-normal quartic surfaces. Tokyo J Math 9(2), 265–295 (1986)
Urabe T.: Elementary transformations of Dynkin graphs and singularities on quartic surfaces. Inventiones mathematicae 87, 549–572 (1987)
Urabe T.: Tie transformations of Dynkin graphs and singularities on quartic surfaces. Inventiones mathematicae 100, 207–230 (1990)
Wong B.C.: A study and classifications of ruled quartic surfaces by means of a point-to-line transformation. Univ. Calif. Publ. Math. 1(17), 371–387 (1923)
Acknowledgement
The authors thank the referee for his very useful remarks.
Open Access
This article is distributed under the terms of the Creative Commons Attribution Noncommercial License which permits any noncommercial use, distribution, and reproduction in any medium, provided the original author(s) and source are credited.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This is an open access article distributed under the terms of the Creative Commons Attribution Noncommercial License (https://creativecommons.org/licenses/by-nc/2.0), which permits any noncommercial use, distribution, and reproduction in any medium, provided the original author(s) and source are credited.
About this article
Cite this article
Polo-Blanco, I., van der Put, M. & Top, J. Ruled quartic surfaces, models and classification. Geom Dedicata 150, 151–180 (2011). https://doi.org/10.1007/s10711-010-9500-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10711-010-9500-0