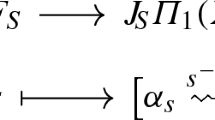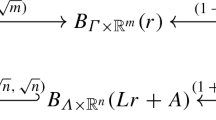Skip to main content
SpringerLink
Log in

Coarse Equivalences Between Warped Cones

  • Published:
Geometriae Dedicata Aims and scope Submit manuscript

Abstract

Warped cones were introduced by J. Roe in Geometry Topol. 9 (2005) 163–178 where he discussed Property A of these spaces. In this paper, we discuss the coarse equivalence of warped cones on the circle with the \(\mathbb{Z}\)-action by irrational rotations. First, we prove that two irrational numbers related by PSL(2, \(\mathbb{Z}\)) give coarsely equivalent warped cones. Second, we prove that there are at least countably many warped cones that are not coarsely equivalent to each other by using a ‘secondary growth function’.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bell G., Dranishnikov A. (2001) On asymptotic dimension of groups. Algebraic Geom. Topol. 1, 57–71

    Article  MATH  MathSciNet  Google Scholar 

  2. Gromov M.: Asymptotic Invariants of Infinite Groups Vol. 2, Geometric Group Theory, Cambridge University Press, 1993

  3. Hardy G. and Wright E.: An Introduction to the Theory of Numbers, 5th edition, Clarencdon Press, Oxford, 1979

  4. Higson N., Roe J. (2000) Amenable group actions and the Novikov conjecture. J. Reine Angew. Math. 519 143–153

    MATH  MathSciNet  Google Scholar 

  5. Hurewicz W., Wallman H., (1948) Dimension Theory, Rev. edn, Princeton University Press, Princeton

    Google Scholar 

  6. Kim H.: Coarse geometry of Warped cones, Ph.D Thesis, Penn State University, 2005.

  7. Lorentzen L. and Waadeland H.: Continued Fractions, Stud. Comput. Math 3, North-Holland, Amsterdam.

  8. Rieffel M. A.: C*-algebras associated with irrational rotations, Pacific. J. Math. 93(2) (1981).

  9. Roe J. Coarse cohomology and index theory for complete Riemanninan manifold, Mem. Amer. Math. Soc. (497) (1993).

  10. Roe J. From foliations to coarse geometry and back. In: Masa X., Macias-Virgos E., Alvarez Lopez J.A., (eds), Analysis and Geometry in Foliated Manifolds, World Scientific, Singapore, 1995 195–206

  11. Roe J. (1996). Index Theory, Coarse Geometry, and the Topology of Manifolds, Vol 90 CBMS Conference Proceedings. Amer. Math. Soc. Providence R.I

    Google Scholar 

  12. Roe, J.: Lectures on Coarse Geometry, University Lecture Series, 31, Amer. Math. Soc., 2003.

  13. Roe J. (2005), Warped Cones and Property A. Geometry Topol. 9: 163–178

    Article  MATH  MathSciNet  Google Scholar 

  14. Yu G. (1998), The Novikov conjecture for groups with finite asymptotic dimension. Ann. Math. 147(2): 325–355

    Article  MATH  Google Scholar 

  15. Yu G. (2000), The coarse Baum-Connes conjecture for spaces which admit a uniform embedding into Hilbert space. Invent. Math., 139: 201–240

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Penn State University, University Park, USA

    Hyun Jeong Kim

Authors
  1. Hyun Jeong Kim
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Kim, H.J. Coarse Equivalences Between Warped Cones. Geom Dedicata 120, 19–35 (2006). https://doi.org/10.1007/s10711-005-9001-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10711-005-9001-8

Keywords

Mathematics Subject Classifications (2000)

Search

Navigation