Analytical homogenization modeling and computational simulation of intergranular fracture in polycrystals

Abstract

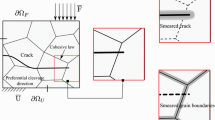
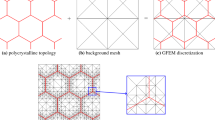
Failure at grain boundaries has a critical effect on the overall fracture behaviour of polycrystalline aggregates, as is the case in many metals. In the case of dynamic embrittlement, segregation of impurities occurs at grain boundaries, lowering their cohesive strength. The material response is then dominantly determined by grain boundary properties. The self-consistent scheme is extended to account for grain boundary decohesion by using a cohesive law to represent crack initiation and propagation. After introducing the imperfect interface conditions into the Eshelby’s equivalent inclusion solution, the effective tensile response and brittle intergranular fracture of a Cu–Ni–Si alloy is predicted. The proposed analytical model allows for the identification of parameters for both crystal plasticity and cohesive constitutive laws, from a single macroscopic tensile curve. Afterwards, multiscale computations of artificial microstructures are done using the analytically calibrated values of material parameters. Comparison of the results with experimental data shows a satisfactory agreement.

Appendices

Appendix 1: Expression of the classical Eshelby tensor $S_{ijkl}$

In the case where the matrix (equivalent homogeneous medium) is isotropic, and for a spherical inclusion, the classical Eshelby tensor is given by:

$$\begin{aligned} S_{ijkl}= & {} \frac{4-5\nu _0 }{15\left( {1-\nu _0 } \right) }\left( {\delta _{ik} \delta _{jl} +\delta _{il} \delta _{jk} } \right) \nonumber \\&+\,\frac{5\nu _0 -1}{15\left( {1-\nu _0 } \right) }\delta _{ij} \delta _{kl} \end{aligned}$$

(38)

where $\nu _0$ denotes the Poisson’s ratio of the equivalent homogeneous medium.

Appendix 2: Expression of the fourth-rank tensor $\varGamma _{ijkl}$

Computation of the average over $\varOmega$ of tensor $\varGamma _{ijkl}$, as defined in Eq. (21), results from long algebraic developments (Othmani et al. 2011). Accounting for the isotropy of the stiffness tensor and considering a spherical inclusion of radius $a$, one can find:

$$\begin{aligned}&\left\langle {\varGamma _{ijkl} \left( {\varvec{x}} \right) } \right\rangle _\varOmega =\frac{E_0 \left( {3\alpha \!+\!2\beta } \right) \left( {7-5\nu _0 } \right) }{150a\left( {1-\nu _0^2 } \right) }\left( {\delta _{ik} \delta _{jl} \!+\!\delta _{il} \delta _{jk} } \right) \nonumber \\&\quad +\,\frac{E_0 \left( {4\beta \left( {3+5\nu _0 } \right) \!-\!\alpha \left( {7-5\nu _0 } \right) } \right) }{75a\left( {1\!-\!\nu _0^2 } \right) }\delta _{ij} \delta _{kl} \end{aligned}$$

(39)

where $E_0$ and $\nu _0$ are the Young’s modulus and the Poisson’s ratio of the equivalent homogeneous medium, and $\alpha$ and $\beta$ are the interface compliances in the tangential and normal directions.

Appendix 3: Expression of the fourth-rank tensor $R_{ijkl}$

The expression of tensor $R_{ijkl}$ depends on the interface properties (through the compliances $\alpha$ and $\beta )$ and the geometry of the inclusion. In the case of a sphere of radius $a$, it is can be simplified as (Qu 1993b):

$$\begin{aligned} R_{ijkl}= & {} \frac{\alpha }{2a}\left( {\delta _{ik} \delta _{jl} +\delta _{il} \delta _{jk} } \right) \nonumber \\&+\,\frac{\left( {\beta -\alpha } \right) }{5a}\left( {\delta _{ik} \delta _{jl} +\delta _{il} \delta _{jk} +\delta _{ij} \delta _{kl} } \right) \end{aligned}$$

(40)

Appendix 4: Computational chart for the self-consistent scheme with imperfect interfaces

a.  $\bullet$ :

Microstructure generation with $N_g$ grains of random crystallographic orientations

b.  $\bullet$ :

Beginning of a new step with stepsize set to the trial value ${\Delta } t_{n+1}$

• Prescribe the macroscopic strain tensor $\underline{{\varvec{E}}}\left( {t_{n+1} } \right)$ to the polycrystal

• Estimate the macroscopic stress tensor using the effective secant moduli of the damaged polycrystal (i.e. with degraded interfaces), $\underline{\varSigma }\left( {t_{n+1} } \right) ={\tilde{\underline{\underline{{\varvec{L}}}}}} ^{eff}:\underline{{\varvec{E}}}\left( {t_{n+1} } \right)$

• For each slip system $s$ of grain $g$:

  • – Compute state variables based on embedded Runge-Kutta formulas in Eqs. (17)

  • – Update at the same time $\underline{{\varvec{\varepsilon }}}^{pg}$ according to Eq. (9), $\underline{{\varvec{\sigma }}}^{g}$ with the transition rule in Eq. (27), and $\tau ^{s}$ according to Eq. (5)

• Evaluate the truncation error according to Eq. (18)

• Evaluate ${\Delta } t_{opt}$ according to Eq. (19)

• If $Err>Tol$ then

  • – Reduce stepsize ${\Delta } t_{n+1} \!=\!\max \left( \! {\Delta } t_{opt}, 0.1{\Delta } t_{n+1} \right)$

  • – Goto b for another try

  Else

  • – Compute stepsize of next step ${\Delta } t_{n+1} =\min$ $\left( {{\Delta } t_{opt} ,5{\Delta } t_{n+1} } \right)$

  End if

• Update the macroscopic stress tensor $\underline{\varSigma }$ with Eq. (1)

• Update the macroscopic plastic strain $\underline{{\varvec{E}}}^{p}$ with Eq. (13)

• Update the secant moduli of the equivalent medium and the secant compliances of the interfaces

• Update the elastic stiffness tensor of the damaged polycrystal (i.e. with degraded interfaces) according to Eq. (28)

• Goto b for next step

Appendix 5: Implementation of viscous regularization

Firstly, it is recalled that, in 3D finite element implementation of cohesive elements, the effective opening displacement $\delta$ is based on three components because a second tangential displacement is introduced. Consequently, the three components of traction are given according to:

$$\begin{aligned}&\delta =\sqrt{\left\langle {\Delta u_n^2 } \right\rangle +\psi ^{2}\Delta u_t^2 }\quad \hbox {with}\quad \Delta u_t^2 =\Delta u_{t_1 }^2 +\Delta u_{t_2 }^2 \nonumber \\ \end{aligned}$$

(41)

$$\begin{aligned}&T_n =\left( {1-d} \right) k_0 \Delta u_n ,\quad T_{t_1 } =\left( {1-d} \right) \psi ^{2}k_0 \Delta u_{t_1 } ,\nonumber \\&\quad T_{t_2 } =\left( {1-d} \right) \psi ^{2}k_0 \Delta u_{t_2 } \end{aligned}$$

(42)

where $n,t_1 ,t_2$ denotes the normal and the two shear directions of the cohesive element, respectively (Fig. 16). The normal direction always points out of the plane of the cohesive element. The parameters $k_0$ and $\psi$ are the undamaged normal stiffness and the coupling parameter, respectively. From there, the regularization process requires the use of a viscous damage variable, $d_v$, which is computed based on the damage variable, $d$, evaluated in the inviscid case:

$$\begin{aligned} \dot{d}_v =-\frac{1}{\mu }\left( {d_v -d} \right) \end{aligned}$$

(43)

where $\mu$ is the viscosity parameter. The implicit backward Euler algorithm, as proposed by Simo et al. (1988), is used in the present study giving a first order accurate formula for $d_v$ at time $t^{\left( {n+1} \right) }$:

$$\begin{aligned} d_v^{\left( {n+1} \right) } =\frac{d_v^{\left( n \right) } +{d^{\left( {n+1} \right) }\Delta t}/\mu }{1+{\Delta t}/\mu } \end{aligned}$$

(44)

The quantities with exponent $n$ are the converged values at the last time increment $t^{\left( n \right) }$. Eventually, the computational procedure for viscous regularization is completed by providing the user subroutine with the components of the traction stress vector and the consistent tangent operator at the end of the increment:

$$\begin{aligned} T_i^{\left( {n+1} \right) }= & {} \left( {1-d_v^{\left( {n+1} \right) } } \right) k_i \Delta u_i^{\left( {n+1} \right) } \end{aligned}$$

(45)

$$\begin{aligned} D_{ij}^{\left( {n+1} \right) }= & {} \frac{\partial T_i^{\left( {n+1} \right) } }{\partial \Delta u_j^{\left( {n+1} \right) } }=\left( {1-d_v^{\left( {n+1} \right) } } \right) k_i \delta _{ij}\nonumber \\&-\,k_i \Delta u_i^{\left( {n+1} \right) } \frac{\partial d_v^{\left( {n+1} \right) } }{\partial \Delta u_j^{\left( {n+1} \right) } } \end{aligned}$$

(46)

where indices $i,j$ take values $n,t_1 ,t_2$. The elastic stiffnesses along the three material directions are linked by $k_n ={k_{t_1 } }/{\psi ^{2}}={k_{t_2 } }/{\psi ^{2}}=k_0$.

Fig. 16

Cohesive fracture separation along the local coordinate system