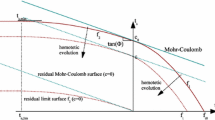
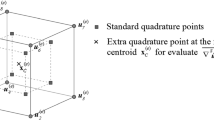
Abstract
Due to the softening behaviour of quasi-brittle materials, in particular the localisation of initially diffused cracking, convergence problems are often found using an iterative procedure, such as the Newton–Raphson method. This is why a new non-iterative procedure is adopted in this paper, which is inspired by the sequentially linear approach(SLA) (Rots et al. in Eng Fract Mech 75(3–4):590–614, 2008). However, several important differences between the present approach and the SLA are presented. In the present model, multi-linear material laws are adopted such that non-linearities occur only due to changes in loading/unloading states. An incremental solution is obtained until non-convergence occurs, upon which a secant approach is used in a corresponding step. The update of the stiffness in the secant approach is based on information obtained from the previous incremental solution. This method is applied to: (i) softening materials, within the scope of the discrete crack approach, and to (ii) hardening materials. As a consequence, conversely to the smeared crack approach adopted in the SLA, no mesh size sensitivity problems are obtained and there is no need to adjust material parameters. Several numerical examples are shown in order to illustrate the proposed formulation.
Similar content being viewed by others
References
Alfaiate J, Simone A, Sluys LJ (2003) Non-homogeneous displacement jumps in strong embedded discontinuities. Int J Solids Struct 40: 5799–5817. doi:10.1016/S0020-7683(03)00372-X
Alfaiate J, Sluys LJ (2002) Analysis of a compression test on concrete using strong embedded discontinuities. In: Mang HA, Rammerstorfer FG, Eberhardsteiner J (eds) WCCM V, fifth world congress on computational mechanics. Wien, Austria
Alfaiate J, Wells GN, Sluys LJ (2002) On the use of embedded discontinuity elements with crack path continuity for mode I and mixed mode fracture. Eng Fract Mech 69(6): 661–686. doi:10.1016/S0013-7944(01)00108-4
Barpi F, Valente S (2000) Numerical simulation of prenotched gravity dam models. J Eng Mech 126(6): 611–619. doi:10.1061/(ASCE)0733-93399(2000)126:6(611)
Billington SL (2009) Nonlinear and sequentially linear analysis of tensile strain hardening cement-based composite beams in flexure. In: Hendriks M, Billington SL (eds) Computational modeling workshop on concrete, masonry and on fiber-reinforced composites, pp 7–10, Delf, The Netherlands
Burns NH, Seiss CP (1962) Load-deformation characteristics of beam-column connections in reinforced concrete. Report technical report, Engineering Studies SRS 234. Department of Civil Engineering, University of California, Berkeley
CEB: (1991) CEB-FIP Model Code 1990. Thomas Telford, London
Crisfield MA (1984) Difficulties with current numerical models for reinforced-concrete and some tentative solutions. Comput Aided Anal Des Concr Struct (1):331–358
Dias-da-Costa D, Alfaiate J, Sluys LJ, Júlio E (2009) Towards a generalization of a discrete strong discontinuity approach. Comput Methods Appl Mech Eng 198(47–48): 3670–3681. doi:10.1016/j.cma.2009.07.013
Dias-da-Costa D, Alfaiate J, Sluys LJ, Júlio E (2010) A comparative study on the modelling of discontinuous fracture by means of enriched nodal and element techniques and interface elements. Int J Fract 161(1): 97–119. doi:10.1007/s10704-009-9432-6
Gago A, Milosevic J, Lopes M, Bento R (2011, submitted) Shear strength of rubble stone masonry walls. Bull Earthq Eng
Galvez JC, Elices M, Guinea GV, Planas J (1998) Mixed mode fracture of concrete under proportional and nonproportional loading. Int J Fract 94(3): 267–284. doi:10.1023/A:1007578814070
Graça-e-Costa R (2005) Modelação de vigas de betão armado reforçadas com chapas metálicas. MSc thesis, Instituto Superior Técnico, Universidade Técnica de Lisboa, Portugal
Graça-e-Costa R, Alfaiate J (2006) The numerical analysis of reinforced concrete beams using embedded discontinuities. SDHM Struct Durab Health Monit 1: 11–17. doi:10.3970/sdhm.2006.002.011
Gutiérrez MA (2004) Energy release control for numerical simulations of failure in quasi-brittle solids. Commun Numer Methods Eng 20(1): 19–29. doi:10.1002/cnm.649
Invernizzi S, Trovato D, Hendriks MAN, van Graaf AV (2011) Sequentially linear modelling of local snap-back in extremely brittle structures. Eng Struct 33(5): 1617–1625. doi:10.1016/j.engstruct.2011.01.031
Lowes LN (1999) Finite element modeling of reinforced concrete beam-column bridge connections. PhD thesis, University of California, Berkeley
Milosevic J, Bento R, Gago A, Lopes M (2010) Seismic vulnerability of old masonry buildings—SEVERES project. Report 1. Instituto Superior Técnico, Lisbon. www.severes.org
Oliver J, Huespe AE, Cante JC (2008) An implicit/explicit integration scheme to increase computability of non-linear material and contact/friction problems. Comput Methods Appl Mech Eng 197(21–24): 1865–1889. doi:10.1016/j.cma.2007.11.027
Rots JG (2001) The role of structural modelling in preserving Amsterdam architectural city heritage. In: Lourenço PB, Roca P (eds) Historical constructions. Guimarães, Portugal, pp 685–696
Rots JG, Belletti B, Invernizzi S (2008) Robust modeling of RC structures with an ”event-by-event” strategy. Eng Fract Mech 75(3–4): 590–614. doi:10.1016/j.engfracmech.2007.03.027
Schlangen E (1993) Experimental and numerical analysis of fracture process in concrete. PhD thesis, Delft University of Technology, The Netherlands
Slobbe AT, Hendriks MAN, Rots JG (2012) Sequentially linear analysis of shear critical reinforced concrete beams without shear reinforcement. Finite Elem Anal Des 50(0): 108–124. doi:10.1016/j.finel.2011.09.002
Verhoosel CV., Remmers JJC, Gutiérrez MA (2009) A dissipation-based arc-length method for robust simulation of brittle and ductile failure. Int J Numer Methods Eng 77(9): 1290–1321. doi:10.1002/nme.2447
Xing H, Zhang J (2009) Finite element modelling of non-linear deformation of rate-dependent materials using a R-minimum strategy. Acta Geotechnica 4(2): 139–148. doi:10.1007/s11440-009-0090-7
Yamada Y, Yoshimura N, Sakurai T (1968) Plastic stress-strain matrix and its application for the solution of elastic-plastic problems by the finite element method. Int J Mech Sci 10(5): 343–354. doi:10.1016/0020-7403(68)90001-5
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Graça-e-Costa, R., Alfaiate, J., Dias-da-Costa, D. et al. A non-iterative approach for the modelling of quasi-brittle materials. Int J Fract 178, 281–298 (2012). https://doi.org/10.1007/s10704-012-9768-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10704-012-9768-1