Abstract
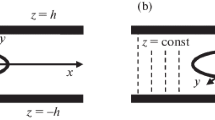
Transversely-isotropic material with an arbitrarily oriented penny-shaped crack is considered. We calculate fourth-rank compliance contribution tensor of the crack and second-rank crack opening displacement tensor and examine their dependence on crack orientation. It is shown that this dependence for the crack opening displacement tensor is negligible if transverse isotropy has elliptic character, i.e. if material symmetry can be described in terms of a certain second rank tensor.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Bristow J.R. (1960) Microcracks, and the static and dynamic elastic constants of annealed heavily cold-worked metals. British J. Appl. Phys 11, 81–85
Choy C.L., Leung W.P., Kowk K.W., Lau F.P. (1992) Elastic moduli and thermal conductivity of injection-molded short fiber reinforced thermoplastics, Polymer Composites 13, 69–80
Dunn M.L., Wienecke H.A. (1997) Inclusions and inhomogeneities in transversely isotropic piezoelectric solids. International Journal of Solids and Structures 34, 3571–3582
Fabrikant, V.I. (1989)Applications of potential theory in mechanics: A selection of new results. Kluwer.
Giraud A., Huynh Q.V., Hoxha D., Kondo D. (2007) Effective poroelastic properties of transversely isotropic rock-like composites with arbitrarily oriented ellipsoidal inclusions. Mechanics of Materials 39, 1006–1024
Hashin Z. (1983). Analysis of composite materials - a survey. J.Appl.Mech. 50, 481–505
Kachanov, M. (1993) Elastic Solids with Many Cracks and Related Problems. In: Advances in Applied Mechanics, 30 (eds. J. Hutchinson and T. Wu), Academic Press, 256–426.
Levin V., Michelitsch Th., Sevostianov I. (2000) Spheroidal inhomogeneity in the transversely isotropic piezoelectric medium, Archive for Applied Mechanics 70, 673–693
Mauge C., Kachanov M. (1994) Effective elastic properties of an anisotropic material with arbitrarily oriented interacting cracks. Journal of the Mechanics and Physics of Solids 42, 561–584
Mikata Y. (2000) Determination of piezoelectric Eshelby tensor in transversely isotropic piezoelectric solids. International Journal of Engineering Sciences 38, 605–641
Pan Y.C., Chou T.W. (1976). Point force solution for an infinite transversely-isotropic solid. Journal of Applied Mechanics 43, 608–612
Sevostianov I., Kachanov M. (2008) On approximate elastic symmetries and elliptic orthotropy. International Journal of Engineering Sciences 46, 211–223
Sevostianov I., Yilmaz N., Kushch V., Levin V. (2005) Effective elastic properties of matrix composites with transversely-isotropic phases. International Journal of Solids and Structures 42, 455–476
Tsukrov, I. and Kachanov, M. (2000) Effective moduli of an anisotropic material with elliptical holes of arbitrary orientational distribution. International Journal of Solids and Structures, 37, 5919–
Van Buskirk, W.C. and Ashman, R.B. (1981) The elastic moduli of bone. In:Mechanical Properties of Bone (Ed. by Cowin, S. C.), AMD-36, 131–143, ASME, New York.
Withers P.J. (1989) The determination of the elastic field of an ellipsoidal inclusion in a transversely isotropic medium and its relevance to composite materials. Phylosophical Magazine A-59, 759–781
Yu H.Y., Sanday S.C., Chang C.I. (1994) Elastic inclusions and inhomogeneities in transversely isotropic solids. Proceedings of the Royal Society of London A-444, 239–252
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Guerrero, F., Sevostianov, I. & Giraud, A. On an Arbitrarily Oriented Crack in a Transversely-isotropic Medium. Int J Fract 148, 273–279 (2007). https://doi.org/10.1007/s10704-008-9191-9
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10704-008-9191-9