Skip to main content
SpringerLink
Log in

On interval portfolio selection problem

  • Published:
Fuzzy Optimization and Decision Making Aims and scope Submit manuscript

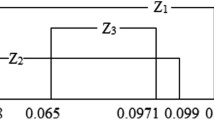
Abstract

The future returns of each securities cannot be correctly reflected by the data in the past, therefore the expert’s judgements and experiences should be considered to estimate the security returns for the future. In this paper, we propose an interval portfolio selection model in which both the returns and the risks of assets are defined as intervals. By using interval and convex analysis, we solve this model and get the noninferior solution. Finally, an example is given to illustrate our results. The interval portfolio selection model improves and generalizes the Markowitz’s mean-variance model and the results of Deng et al. (Eur J Oper Res 166(1):278–292, 2005).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Bertsimas, D., & Thiele, A. (2006). Robust and data-driven optimization: Modern decision making under uncertainty. In Tutorials in operations research (pp. 95–122). INFORMS.

  • Best, M. J., & Grauer, R. R. (1991). On the sensitivity of mean-variance-efficient portfolios to changes in asset means: some analytical and computational results. Review of Financial Studies, 4(2), 315–342.

    Article  Google Scholar 

  • Best, M. J., & Grauer, R. R. (1991). Sensitivity analysis for mean-variance portfolio problems. Management Science, 37(8), 980–989.

    Article  MATH  Google Scholar 

  • Deng, X. T., Li, Z. F., & Wang, S. Y. (2005). A minimax portfolio selection strategy with equilibrium. European Journal of Operational Research, 166(1), 278–292.

    Article  MathSciNet  MATH  Google Scholar 

  • Elton, E. J., Gruber, M. J., & Urich, T. J. (1978). Are betas best? Journal of Finance, 33(5), 1375–1384.

    Google Scholar 

  • Fan, K. (1953). Minimax theorems. Proceedings of the National Academy of Sciences of the United States of America, 39(1), 42–47.

    Article  MathSciNet  MATH  Google Scholar 

  • Giove, S., Funari, S., & Nardelli, C. (2006). An interval portfolio selection problem based on regret function. European Journal of Operational Research, 170(1), 253–264.

    Article  Google Scholar 

  • Inuiguchi, M., & Ramík, J. (2000). Possibilistic linear programming: A brief review of fuzzy mathematical programming and a comparison with stochastic programming in portfolio selection problem. Fuzzy Sets and Systems, 111(1), 3–28.

    Article  MathSciNet  MATH  Google Scholar 

  • Inuiguchi, M., & Sakawa, M. (1995). Minimax regret solution to linear programming problems with an interval objective function. European Journal of Operational Research, 86(3), 526–536.

    Article  MathSciNet  MATH  Google Scholar 

  • Konno, H., & Yamazaki, H. (1991). Mean-absolute deviation portfolio optimization model and its applications to Tokyo stock market. Management Science, 37(5), 519–531.

    Article  Google Scholar 

  • Lai, K. K., Wang, S. Y., Xu, J. P., Zhu, S. S., & Fang, Y. (2002). A class of linear interval programming problems and its application to portfolio selection. IEEE Transactions on Fuzzy Systems, 10(6), 698–704.

    Article  Google Scholar 

  • Lin, C. C., & Liu, Y. T. (2008). Genetic algorithms for portfolio selection problems with minimum transaction lots. European Journal of Operational Research, 185(1), 393–404.

    Article  MATH  Google Scholar 

  • Mao, J. C. T. (1970). Models of capital budgeting, E-V VS E-S. Journal of Financial and Quantitative Analysis, 4(5), 657–675.

    Article  Google Scholar 

  • Markowitz, H. M. (1952). Portfolio selection. Journal of Finance, 7(1), 77–91.

    Google Scholar 

  • Markowitz, H. M. (1987). Mean-variance analysis in portfolio choice and capital markets. Oxford: Basil Blackwell.

    MATH  Google Scholar 

  • Markowitz, H. M. (1991). Portfolio selection: Efficient diversification of investments (2nd ed.). Oxford: Basil Blackwell.

    Google Scholar 

  • Neumaier, A. (1990). Interval methods for systems of equations. Cambridge: Cambridge University Press.

    MATH  Google Scholar 

  • Rohn, J. (1989). Systems of linear interval equations. Linear algebra and its applications, 126, 39–78.

    Article  MathSciNet  MATH  Google Scholar 

  • Rohn, J. (1993). Inverse interval matrix. SIAM Journal on Numerical Analysis, 30(3), 864–870.

    Article  MathSciNet  MATH  Google Scholar 

  • Sengupta, A., & Pal, T. K. (2000). On comparing interval numbers. European Journal of Operational Research, 127(1), 28–43.

    Article  MathSciNet  MATH  Google Scholar 

  • Wang, S. Y., & Zhu, S. S. (2002). On fuzzy portfolio selection problems. Fuzzy Optimization and Decision Making, 1(4), 361–377.

    Article  MathSciNet  MATH  Google Scholar 

  • Xia, Y. S., Liu, B. D., Wang, S. Y., & Lai, K. K. (2000). A model for portfolio selection with order of expected returns. Computers and Operations Research, 27(5), 409–422.

    Article  MATH  Google Scholar 

  • Yoshimoto, A. (1996). The mean-variance approach to portfolio optimization subject to transaction costs. Journal of the Operations Research Society of Japan, 39(1), 99–117.

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

This work was supported by the National Natural Science Foundation of China (71101099, 70831005) and the Special Funds of Sichuan University of the Fundamental Research Funds for the Central Universities (SKQY201330).

Author information

Authors and Affiliations

  1. College of Business Administration, Sichuan University, Chengdu, 610064, Sichuan, People’s Republic of China

    Meng Wu & Jiu-ping Xu

  2. The Wang Yanan Institute for Studies in Economics, Xiamen University, Xiamen, 361005, Fujian, China

    De-wang Kong

  3. Department of Mathematics, Sichuan University, Chengdu, 610064, Sichuan, China

    Nan-jing Huang

Authors
  1. Meng Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. De-wang Kong
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jiu-ping Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Nan-jing Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Meng Wu.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Wu, M., Kong, Dw., Xu, Jp. et al. On interval portfolio selection problem. Fuzzy Optim Decis Making 12, 289–304 (2013). https://doi.org/10.1007/s10700-013-9155-z

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10700-013-9155-z

Keywords

Search

Navigation