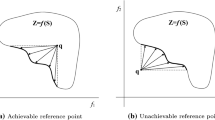
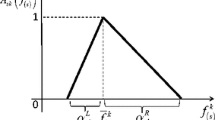
Abstract
This paper reviews the credibilistic portfolio selection approaches which deal with fuzzy portfolio selection problem based on credibility measure. The reason for choosing credibility measure is given. Several mathematical definitions of risk of an investment in the portfolio are introduced. Some credibilistic portfolio selection models are presented, including mean-risk model, mean-variance model, mean-semivariance model, credibility maximization model, α-return maximization model, entropy optimization model and game models. A hybrid intelligent algorithm for solving the optimization models is documented. In addition, as extensions of credibilistic portfolio selection approaches, the paper also gives a brief review of some hybrid portfolio selection models.
Similar content being viewed by others
References
Arditti F.D. (1971) Another look at mutual fund performance. Journal of Financial and Quantitative Analysis 6: 909–912
Arenas-Parra M., Bilbao-Terol A., Rodríguez-Uría M.V. (2001) A fuzzy goal programming approach to portfolio selection. European Journal of Oprational Research 133: 287–297
Bilbao-Terol A., Pérez-Gladish B., Arenas-Parra M., Rodríguez-Uría M.V. (2006) Fuzzy compromise programming for portfolio selection. Applied Mathematics and Computation 173: 251–264
Bruckley J.J., Hayashi Y. (1994) Fuzzy genetic algorithm and applications. Fuzzy Sets and Systems 61: 129–136
Bruckley J.J., Feruing T. (2000) Evolution algorithm solution to fuzzy problems: Fuzzy linear programming. Fuzzy Sets and Systems 109: 35–53
Carlsson C., Fullér R., Majlender P. (2002) A possibilistic approach to selecting portfolios with highest utility score. Fuzzy Sets and Systems 131: 13–21
Chunhachinda P., Dandapani K., Hamid S., Prakash A.J. (1997) Portfolio selection and skewness: Evidence from international stock market. Journal of Banking and Finance 21: 143–167
Colubi A., Fernández-García C., Gil M.A. (2002) Simulation of random fuzzy variables: An empirical approach to statistical/probabilistic studies with fuzzy experimental data. IEEE Transactions on Fuzzy Systems 10: 384–390
De Cooman G. (1997) Possibility theory I–III. International Journal of General Systems 25: 291–371
Dubois D., Prade H. (1988) Possibility theory: An approach to computerized processing of uncertainty. Plenum, New York
Fama E. (1965) Portfolio analysis in a stable paretian market. Management Science 11: 404–419
Gupta P., Mehlawat M.K., Saxena A. (2008) Asset portfolio optimization using fuzzy mathe matical programming. Information Sciences 178: 1734–1755
Holland J. (1975) Adaptation in natural and artificial systems. University of Michigan Press, Ann Arbor
Huang, X. (2004). Portfolio selection with fuzzy returns. Proceedings of the Second Annual conference on Uncertainty (pp. 21–29). Qinhuangdao July 16–20.
Huang X. (2006) Fuzzy chance-constrained portfolio selection. Applied Mathematics and Computation 177: 500–507
Huang X. (2007a) Two new models for portfolio selection with stochastic returns taking fuzzy information. European Journal of Operational Research 180: 396–405
Huang X. (2007b) A new perspective for optimal portfolio selection with random fuzzy returns. Information Sciences 177: 5404–5414
Huang X. (2007c) Portfolio selection with fuzzy returns. Journal of Intelligent & Fuzzy Systems 18: 383–390
Huang X. (2008a) Mean-semivariance models for fuzzy portfolio selection. Journal of Computational and Applied Mathematics 217: 1–8
Huang X. (2008b) Risk curve and fuzzy portfolio selection. Computers and Mathematics with Applications 55: 1102–1112
Huang X. (2008c) Expected model for portfolio selection with random fuzzy returns. International Journal of General Systems 37: 319–328
Huang X. (2008d) Portfolio selection with a new definition of risk. European Journal of Operational Research 186: 351–357
Huang X. (2008e) Mean-entropy models for fuzzy portfolio selection. IEEE Transactions on Fuzzy Systems 16: 1096–1101
Huang, X. (2008f). Minimax mean-variance models for fuzzy portfolio selection, Technical Report.
Huang, X. (2008g). Fuzzy minimax chance constrained models for portfolio selection, Technical Report.
Huang, X. (2008h). Mean-variance model for hybrid portfolio selection with randomness and fuzziness, Technical Report.
Ida M. (2002) Mean-variance portfolio optimization model with uncertain coefficients. Proceedings of IEEE International Conference on Fuzzy Systems 3: 1223–1226
Inuiguchi M., Ramík J. (2000) Possibilistic linear programming: A brief review of fuzzy mathematical programming and a comparison with stochastic programming in portfolio selection problem. Fuzzy Sets and Systems 111: 3–28
Katagiri H., Ishii H. (1999) Fuzzy portfolio selection problem. Proceedings of the IEEE International Conference on Systems, Man and Cybernetics 3: III-973–III-978
Kaufman A., Gupta M.M. (1985) Introduction to fuzzy arithmetic: Theory and applications. Van Nostrand Reinhold, New York
Klir G.J., Yuan B. (1995) Fuzzy sets and fuzzy logic: Theory and applications. Prentice-Hall, New Jersey
Lacagnina V., Pecorella A. (2006) A stochastic soft constraints fuzzy model for a portfolio selection problem. Fuzzy Sets and Systems 157: 1317–1327
León T., Liern V., Vercher E. (2002) Viability of infeasible portfolio selection problems: A fuzzy approach. European Journal of Operational Research 139: 178–189
Li J., Xu J. (2009) A novel portfolio selection model in a hybrid uncertain environment. Omega 37: 439–449
Li P., Liu B. (2008) Entropy of credibility distributions for fuzzy variables. IEEE Transactions on Fuzzy Systems 16: 123–129
Li X., Liu B. (2007) Maximum entropy principle for fuzzy variable. International Journal of Uncertainty, Fuzziness & Knowledge-Based Systems 15(Supp. 2): 43–52
Lin C.C., Liu Y.T. (2008) Genetic algorithm for portfolio selection problems with minimum transaction lots. European Journal of Operational Research 185: 393–404
Liu B. (1998) Minimax chance constrained programming models for fuzzy decision systems. Information Sciences 112: 25–38
Liu B. (2002) Theory and practice of uncertain programming. Physica-Verlag, Heidelberg
Liu B. (2004) Uncertainty theory: An introduction to its axiomatic foundations. Springer–Verlag, Berlin
Liu B. (2006a) A survey of credibility theory. Fuzzy Optimization and Decision Making 5: 387–408
Liu B. (2007) Uncertainty theory, 2nd edn. Springer–Verlag, Berlin
Liu B., Iwamura K. (1998) Chance constrained programming with fuzzy parameters. Fuzzy Sets and Systems 94: 227–237
Liu B., Liu Y.K. (2002) Expected value of fuzzy variable and fuzzy expected value models. IEEE Transactions on Fuzzy Systems 10: 445–450
Liu Y.K. (2006b) Convergent results about the use of fuzzy simulation in fuzzy optimization problems. IEEE Transactions on Fuzzy Systems 14: 295–304
Markowitz H. (1952) Portfolio selection. Journal of Finance 7: 77–91
Markowitz H. (1959) Portfolio selection: efficient diversification of investments. Wiley, New York
Nahmias S. (1978) Fuzzy variables. Fuzzy Sets and Systems 1: 97–110
Oh K.J., Kim T.Y., Min S.H., Lee H.Y. (2006) Portfolio algorithm based on portfolio beta using genetic algorithm. Expert Systems with Applications-Intelligent Information Systems for Financial Engineering 30: 527–534
Rahib H.A., Mustafa M. (2007) Fuzzy portfolio selection using genetic algorithm. Soft Computing—A Fusion of Foundations, Methodologies and Applications 11: 1157–1163
Rom B.M., Ferguson K.W. (1994) Post-modern portfolio theory comes of age. Journal of Investing 3: 11–17
Shoaf, J., & Foster, J. A. (1996). The efficient set GA for stock portfolios. Proceedings of the Decision Science Institute, Orlando (pp. 571–573).
Simkowitz M., Beedles W. (1978) Diversification in a three moment world. Journal of Financial and Quantitative Analysis 13: 927–941
Skolpadungket, P., Dahal, K., & Harnpornchai, N. (2007). Portfolio optimization using multi-objective genetic algorithms. Proceeding of 2007 IEEE Congress on Evolutionary Computation (pp. 516–523).
Tanaka H., Guo P. (1999) Portfolio selection based on upper and lower exponential possibility distributions. European Journal of Operational Research 114: 115–126
Tanaka H., Guo P., Türksen B. (2000) Portfolio selection based on fuzzy probabilities and possibility distributions. Fuzzy Sets and Systems 111: 387–397
Tiryaki F., Ahlatcioglu M. (2005) Fuzzy stock selection using a new fuzzy ranking and weighting algorithm. Applied Mathematics and Computation 170: 144–157
Unser M. (2000) Lower partial moments as measures of perceived risk: An experimental study. Journal of Economic Psychology 21: 253–280
Vercher E., Bermúdez J.D., Segura J.V. (2007) Fuzzy portfolio optimization under downside risk measures. Fuzzy Sets and Systems 158: 769–782
Watada J. (1997) Fuzzy portfolio selection and its applications to decision making. Tatra Mountains Mathematical Publication 13: 219–248
Yan W., Miao R., Li S. (2007) Multi-period semi-variance portfolio selection: Model and numerical solution. Applied Mathematics and Computation 194: 128–134
Zadeh L. (1965) Fuzzy sets. Information and Control 8: 338–353
Zadeh L. (1978) Fuzzy sets as a basis for a theory of possibility. Fuzzy Sets and Systems 1: 3–28
Zhang W.G., Nie Z.K. (2004) On admissible efficient portfolio selection problem. Applied Mathematics and Computation 159: 357–371
Zhang W.G., Wang Y.L., Chen Z.P., Nie Z.K. (2007) Possibilistic mean-variance models and efficient frontiers for portfolio selection problem. Information Sciences 177: 2787–2801
Zimmermann H.J. (1985) Fuzzy set theory and its applications. Kluwer Academic Publisher, Boston
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Huang, X. A review of credibilistic portfolio selection. Fuzzy Optim Decis Making 8, 263–281 (2009). https://doi.org/10.1007/s10700-009-9064-3
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10700-009-9064-3