Skip to main content
SpringerLink
Log in

On the Parameterized OWA Operators for Fuzzy MCDM Based on Vague Set Theory

  • Published:
Fuzzy Optimization and Decision Making Aims and scope Submit manuscript

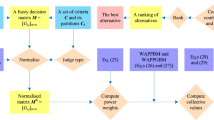
Abstract

The family of Ordered Weighted Averaging (OWA) operators, as introduced by Yager, appears to be very useful in multi-criteria decision-making (MCDM). In this paper, we extend a family of parameterized OWA operators to fuzzy MCDM based on vague set theory, where the characteristics of the alternatives are presented by vague sets. These families are specified by a few parameters to aggregate vague values instead of the intersection and union operators proposed by Chen. The proposed method provides a “soft” and expansive way to help the decision maker to make his decisions. Examples are shown to illustrate the procedure of the proposed method at the end of this paper.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • S. M. Chen J. M. Tan (1994) ArticleTitle“Handling Multicriteria Fuzzy Decision-making Problems Based on Vague set Theory” Fuzzy Sets and Systems 67 163–172 Occurrence Handle10.1016/0165-0114(94)90084-1 Occurrence Handle95f:90006

    Article  MathSciNet  Google Scholar 

  • Filev, D. P. and R. R. Yager. (1994). “Learning OWA Operator Weights from Data.” In Proceedings of the 3rd IEEE International Conference Fuzzy Systems, Orlando, FL, pp. 468–473.

  • D. P. Filev R. R. Yager (1998) ArticleTitle“On the Issue of Obtaining OWA Operator Weights” Fuzzy Sets Systems 94 157–169 Occurrence Handle10.1016/S0165-0114(96)00254-0 Occurrence Handle98m:68261

    Article  MathSciNet  Google Scholar 

  • Larsen, H. L. (1999). “Importance Weighted OWA Aggregation of Multicriteria Queries,” In Proceeding of the North American Fuzzy Information Processing Society conference (NAFIPS’99), New York, 10–12 June 1999, pp. 740–744.

  • W.-L. Gau D. J. Buehrer (1993) ArticleTitle“Vague Sets” IEEE Transactions on Systems, Man and Cybernetics 23 IssueID2 610–614 Occurrence Handle10.1109/21.229476

    Article  Google Scholar 

  • D. J. Hong C. H. Choi (2000) ArticleTitle“Multicriteria Fuzzy Decision-making Problems Based on Vague set Theory” Fuzzy Sets and Systems 114 103–113 Occurrence Handle10.1016/S0165-0114(98)00271-1

    Article  Google Scholar 

  • C. L. Hwang K. Yoon (1981) Multiple Attributes Decision Making Methods and Applications Springer Berlin, Heidelberg

    Google Scholar 

  • W. J. M. Kickert (1978) Fuzzy Theories on Decision Making: A Critical Review Kluwer Boston

    Google Scholar 

  • P. J. M. Laarhoven W. Pedrycz (1983) ArticleTitle“A Fuzzy Extension of Saaty’s Priority Theory” Fuzzy Sets and System 11 229–241 Occurrence Handle85f:03056

    MathSciNet  Google Scholar 

  • H. B. Mitchell D. D. Estrakh (1997) ArticleTitle“A Modified OWA Operator and its use in Lossless DPCM Image Compression” International Journal of Uncertainty, Fuzziness, Knowledge Based Systems 5 429–436

    Google Scholar 

  • O’Hagan, M. (1988) “Aggregating Template or rule antecedents in Real-time expert Systems with Fuzzy set Logic,” In Proceedings of the 22nd Annual IEEE Asilomar Conference Signals, Systems, Computers, Pacific Grove, CA, pp. 81–689.

  • R. R. Yager (1978) ArticleTitle“Fuzzy Decision Making Including Unequal Objectives” Fuzzy Sets and Systems 1 87–95 Occurrence Handle0378.90011

    MATH  Google Scholar 

  • R. R. Yager (1988) ArticleTitle“On Ordered Weighted Averaging Aggregation Operators in Multicriteria Decision Making” IEEE Trans. Systems Man Cybernetics 18 183–190 Occurrence Handle0637.90057 Occurrence Handle89a:90073

    MATH  MathSciNet  Google Scholar 

  • R. R. Yager (1993) ArticleTitle“Families of OWA Operator” Fuzzy Sets and System 59 125–148 Occurrence Handle0790.94004 Occurrence Handle95g:68115

    MATH  MathSciNet  Google Scholar 

  • R. R. Yager (1997) “On the Inclusion of Importances in OWA Aggregations” R. R. Yager J. Kacprzyk (Eds) The Ordered Weighted Averaging Operators: Theory and Applications Kluwer Norwell, MA 41–59

    Google Scholar 

  • R. R. Yager D. Filev (1999) ArticleTitle“Induced Ordered Weighted Averaging Operators” IEEE Trans. on Systems, Man and Cybernetics. Part B: Cybernetics 29 141–150

    Google Scholar 

  • R. R. Yager J. Kacprzyk (1997) The Ordered Weighted Averaging Operators: Theory and Applications Kluwer Norwell, MA

    Google Scholar 

  • L. A. Zadeh (1965) ArticleTitleFuzzy Sets Information and Control 8 338–356 Occurrence Handle10.1016/S0019-9958(65)90241-X Occurrence Handle0139.24606 Occurrence Handle36 #2509

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Science, Xidian University, Xi’an, Shaanxi, 710071, P. R. China

    Jue Wang, San-Yang Liu & Jie Zhang

  2. Institute of Systems Science, Academy of Mathematics and Systems Sciences, Chinese Academy of Sciences, Beijing, 100080, P. R. China

    Jue Wang & Shou-Yang Wang

Authors
  1. Jue Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. San-Yang Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jie Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Shou-Yang Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jue Wang.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Wang, J., Liu, SY., Zhang, J. et al. On the Parameterized OWA Operators for Fuzzy MCDM Based on Vague Set Theory. Fuzzy Optim Decis Making 5, 5–20 (2006). https://doi.org/10.1007/s10700-005-4912-2

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10700-005-4912-2

Keywords

Search

Navigation