Abstract
The present paper aims to investigate the transport of suspended sediment in an open channel turbulent flow. The method of moments is used to solve the unsteady two-dimensional suspended sediment transport equation where the moment equations are evaluated using a standard finite difference implicit scheme. The distribution of concentration is obtained using the Hermite polynomial representation of central moments. The solution exists for arbitrary form of eddy diffusivity and in contrast to other existing works, the present model considers the most general boundary condition at the channel bed which may be absorbing, reflecting or both. According to such nature of channel bed, the transport process has been distinguished into two phases, viz., suspension phase and deposition phase together with re-entrainment of particles. The model in its present form can be well applicable for sediment transportation in environmental processes.
Similar content being viewed by others
1 Introduction
In open channel turbulent flow, the transport of suspended sediment has been an important topic of research for centuries as it helps in understanding the erosion, transportation, deposition and re-entrainment process that play a crucial role in geology and engineering practices. The materials are transported mainly as bed load where the particles move very near to the bed by sliding, rolling and jumping and as suspended load where the particles are carried out by turbulence remaining in suspension. Materials carried out in suspension form the major part of the total load. Researchers devoted themselves to develop several mathematical models on suspended sediment concentration involving both analytical and numerical methods. But due to the random behavior of turbulence, any prediction is difficult and research on this topic seems to be continued.
Most of the models developed on suspended sediment transport are based on steady and one-dimensional (vertical) distribution [1,2,3,4,5,6,7,8] that result into an ordinary differential equation (ODE). If the variation of concentration is considered along vertical direction as well as along main flow direction or a combination of one or both with time, the resulting governing equation becomes a partial differential equation (PDE). Partial differential equations are more difficult to handle than ordinary differential equations and researchers who took into account partial differential equations had to put restriction on the model regarding consideration of different turbulent features in order to solve the PDEs. Considering constant diffusivity and a fixed value boundary condition at the bottom, Mei [9] studied the distribution of suspended sediment in two-dimensional open channel flow under steady state. Hjelmfelt and Lenau [10] investigated transportation of sediment in two-dimensional steady condition and solved the problem by classical method of separating variables. Cheng [11] proposed a generalized bottom boundary condition for non-equilibrium transport of sediment in terms of reflectivity coefficient of the bottom surface and an equilibrium bottom concentration. With this boundary condition, he proposed an analytical solution of the diffusion equation by Laplace transform method. Using the finite element method, van Rijn [12] solved the two-dimensional transport equation for non-uniform flow, where the profile method is applied to compute the fluid velocities and mixing coefficients as suggested by Coles [13]. By considering a steady two-dimensional transport equation, Celik and Rodi [14] discussed the transport of suspended sediment in an open channel turbulent flow which is applicable to both net-deposition and net-entrainment non-equilibrium situations. Considering vertical settling–dispersion problem, Pritchard [15] analytically investigated the temporal response (or rate of deposition) of suspended sediments. Dorrell and Hogg [16] established that in the complex process of unsteady turbulent suspension and sedimentation, the form of eddy diffusivity matters little in the regimes of large and small settling parameter. They gave asymptotic solution for the unsteady two-dimensional advection diffusion equation in the regimes of settling parameter much less and much greater than one. In literature, a number of articles [17,18,19,20,21] are found who considered the suspended sediment transport under non-equilibrium situations. Recently, Liu and Nayamatullah [22] solved the same problem as Cheng [11] applying Generalized Integral Transform Technique (GITT) with arbitrary eddy viscosity. Later on, with the same technique, Liu [23] solved the steady two-dimensional problem with arbitrary advection velocity and eddy diffusivity distribution. Jing et al. [24] studied the suspension for a steady two-dimensional uniform flow with effect of concentration-dependent settling velocity and solved the non-linear PDE by numerical method.
Suspension is a significant mode of transport of sediment when particle fall velocities are smaller than the turbulent velocity fluctuations. In rivers, the sediments are normally get transported due to the performance of bed load. If the water discharge is large enough, then suspension turns to be the dominating mechanism for transportation and the relaxation length becomes four to five orders of magnitude higher than for bed load [25]. The modeling of the exchange (pick-up and deposition) of sediment particles with the movable bed surface is an important part of sediment transport phenomena. Various researchers [26,27,28] have investigated the pick-up rate of particles in sediment transportation. Turbidity current is one of several types of sediment transport mechanisms in which sediment is suspended by fluid turbulence. It has the ability that can exchange sediment particles with the lake or ocean floor. A number of works [29,30,31] provide the information about the nature of turbidity currents in the transport of sediments.
The sediment transport problem has been looked at by the researchers from the two-phase point of view also. Revil-Baudard and Chauchat [32] used two-phase model to describe the sheet flow regime of sediment transport considering a dilute suspension layer and a dense sediment layer. In the sediment bed layer they obtained the concentration profile from dilatancy law and in the suspension layer from Rouse profile. Chiodi et al. [33] theoretically investigated the transition between bed load and suspended load and developed a two phase flow model of sediment transport. Chauchat et al. [34] considered turbulence-averaged two-phase flow governing equations for determining particle and fluid volume concentrations and for the numerical solution they used the solver SED Foam-2.0. Recently, Cheng et al. [35] developed a Reynolds-averaged Euler–Lagrange sediment transport model for turbulent suspension and simulated the interactions between the turbulent eddies and particles with an eddy interaction model.
Apart from the studies on total sediment concentration, researchers also investigated particle concentration in suspension for different size classes. Mazumder et al. [36] did experiments with varied bed roughness to examine its influence on size distribution as well as total concentration in suspension. For modeling the experimental results, they considered Hunt [2] diffusion equation for n-th grain size and it gave rise to a series of ODEs, solution of each of which provided the concentration of a particular grain size in suspension at different heights. Dorrell et al. [37] considered the vertical distribution of a large number of different grain sizes which they termed polydisperse suspension and modeled it using Reynolds-averaged mass continuity equation. Their governing equations were a series of PDEs as they considered the time scale of the suspending turbulent flow. Recently, Dorrell et al. [38] showed that the prediction of the threshold between the entrainment and deposition of particles in suspended load depends on polydisperse particle size modeling approach.
All the aforementioned works mostly studied the suspended sediment concentration putting importance mainly on suspension phase. But apart from suspension phase, whenever sediments are carried out in suspension, the transportation process consists another phase, which is the deposition phase together with the re-entrainment of particles. The mass exchange of sediment between flow and river bed has been a fundamental issue for the study of non-capacity sediment transport [39]. Whether the sediments move due to flow over an erodible sediment bed or move due to the release of sand particles into the flow, their movement occurs due to the continued action of flow velocity, turbulence and gravity. The particles may continue to travel being in suspension or may fall due to gravity depending on the flow and particle characteristics and may enter again into the main flow region. In other words, while being transported remaining in suspension, the particles have a tendency to settle and are deposited on the bed and some of them are again bounced back in the flow [40]. So the diffusion process of sediment particles in an open channel turbulent flow includes continuous exchange of particles between the bed and the flow. Monin [41] proposed a bottom boundary condition considering the deposition part only and re-entrainment part was ignored. Dobbins [42] though considered both deposition and re-entrainment, but his formulation assumed both the re-entrainment rate and deposition flux to be constant which is an unnecessary constraint. Cheng [11] developed a more generalized bottom boundary condition introducing a parameter \(\beta \) which was interpreted as the reflectivity coefficient of the bottom surface, i.e., perfectly reflecting surface is represented by \(\beta =0\), and perfectly absorbing surface is represented by \(\beta \rightarrow \infty \). This boundary condition was used by Liu and Nayamatullah [22] and Liu [23]. But this boundary condition has also restriction in the sense that the reflectivity coefficient \(\beta \), which can also be regarded as deposition velocity, is some fixed parameter present only in the boundary condition. But it is essential to consider the deposition and the re-entrainment of particles in the governing equation as well in order to study these features appropriately in the transport process.
Sufficient insights have been gained for the mechanism of convection and diffusion of suspended particles, but the convection and diffusion together with deposition and re-entrainment are yet to be explored. The main objective of the present work is to study two-dimensional unsteady suspended sediment transport in an open channel turbulent flow by considering the deposition and re-entrainment in the governing equation and in the boundary condition as well unlike the existing works. The concept of statistical moments and Hermite polynomials are used together to get the solution of the transport equation.
2 Mathematical formulation
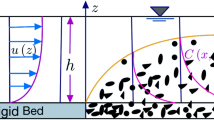
A unidirectional turbulent flow through an open channel with a separation width \({\hat{h}}\) is considered. The flow geometry is described with a Cartesian coordinate frame where the axial and vertical direction of flow are assumed as \({\hat{x}}\) and \({\hat{y}}\)-axis, respectively (the cap represents the dimensional quantity). It is assumed that the flow is uniform in the axial direction and independent of time and hence velocity \({\hat{u}}\) varies only in the vertical direction. It has also been assumed that due to advection velocity, an amount of sediment particle is entrained into the flow and remains in the suspension, whereas some of its portion is settled and stored at the bed. This situation is referred to as suspension phase (particles that exist in the suspension) and deposition phase (particles that are stored at the bed).
Let \({\hat{C}}({\hat{t}},{\hat{x}},{\hat{y}})\) be the concentration of sediment in suspension which satisfies the transport equation:
where \({\hat{u}}({\hat{y}})\) is the flow velocity, \({\hat{\omega }}_s\) is the constant sediment settling velocity and \({\hat{\varepsilon }}({\hat{y}})\) is the eddy viscosity. The initial condition is supposed to be
where \(\delta ({\hat{x}})\) is the Dirac delta function representing an instantaneous source uniformly distributed over the cross section of the channel. The boundary conditions are
where \({\hat{W}}({\hat{t}},{\hat{x}})\) is the concentration of sediment in the deposition phase (stored per unit area of bed surface). Equation (4) states that there is no net transport of mass across the upper surface and Eq. (5) is the most general boundary condition for the present study describing the behavior of bed barrier either to be absorbing or reflecting. \(\theta \) is the bed absorbency coefficient representing the probability that sediment coming into contact with the bed is deposited and \({\hat{\gamma }}\) is the rate of entrainment, respectively. Clearly, the combination of first two terms (upward diffusive flux and average rate of entrainment) in Eq. (5) enables the sediment entrainment. Here, if \(\theta =0\), the bed behaves like a fully reflecting boundary, i.e., no sediment particle will store or settle at the bottom and if \(\theta =1\), an opposite nature of bed may be experienced, i.e., particle never returns to the suspension once it comes into contact with the bed. The ideal condition is when \(0<\theta <1\) which means that the bed is partially absorbing and the value of \(\theta \) refers to the fraction of particles that are deposited on the bed and the remaining part (\(1-\theta \)) is re-entrained in suspension.
To study the sediment transport processes in consideration with bed deformation, several authors [43,44,45,46] followed the multi-layer concept where the vertical bed structure is divided into different layers. They assumed that the vertical bed structure consists of one active layer at the top surface and several substrate layers under active layer. Depending on various factors the sediment particles can move between these layers. In the present work directly we did not consider the bed deformation, but phenomena like deposition, bed absorption and re-entrainment of particles are assumed by means of a two-phase model. In conjunction with the channel bed condition, an equation (describing the exchange of the suspension and deposition phases) for the deposition distribution function \({\hat{W}}({\hat{t}},{\hat{x}})\) is considered as [40]
and the initial condition for Eq. (6) is
Now we introduce the following dimensionless quantities:
where U is the depth-averaged velocity which is the average velocity at any vertical section of a channel cross section and \(C_0\) is the reference concentration. Substituting the above dimensionless quantities, Eqs. (1)–(7) can be re-written in dimensionless form as
Transport equation for sediment in suspension:
Initial and boundary conditions:
where \(\gamma ={{\hat{\gamma }}{\hat{h}}}/{U}\) is the dimensionless entrainment rate coefficient which measures the amount of deposited particle that returns from bed to suspension. The entrainment rate coefficient \(\gamma \) is defined in a way that \(\gamma \varDelta t\) is the probability that a particle resting on the bed is entrained in the flow during a short time interval of duration \(\varDelta t\). Consequently \(\gamma W\) represents an average rate of entrainment [40].
Exchange of two phases with initial condition:
with
Next section describes the Aris’s method of moments and the present work solves Eqs. (8) and (12) by the method of moments subject to the conditions [Eqs. (9)–(11) and Eq. (13)].
3 Method of moments
Following Aris [47], the p-th moment of the distribution of the sediment concentration in the suspension phase at time t can be described as
and likewise for the deposition phase we may assume
Using Eqs. (14) and (15), the transport equation (8) can be written as
subject to the initial and boundary conditions
Likewise the deposition mass distribution equation (12) can be written as
The p-th order central moment about mean of the concentration distribution can be defined as
where \(x_g={\int xC\,\text{ d }x }/{\int C\,\text{ d }x}={{C^{(1)}}}/{{C^{(0)}}}\) is the first-order moment of the distribution of sediment, normalized by \(C^{(0)}\). The zeroth moment, \(C^{(0)}\), represents the total mass of the sediment in the flowing stream. The first-order moment, \(x_g\), is the location of the center of mass of the concentration distribution curve and it signifies the average distance traveled by the suspended sediment over time, t. From Eq. (21), the higher order central moments can be obtained as
The second-order central moment, \(\mu _2\), is a measure of the spread of the concentration distribution; the third, \(\mu _3\), and the fourth \(\mu _4\) are the measure of the curve’s skewness \(\nu _2(=\mu _3/\mu _2^{\frac{3}{2}})\) and kurtosis \(\nu _3(=\mu _4/\mu _2^2-3)\), respectively. Utilizing these four moments, it is possible to approximate the concentration distribution C(t, x, y) of tracers within the flow region with the assistance of Hermite polynomial [48,49,50,51]) and is given by
where \( \eta = (x - x_g)/\sqrt{ 2 \mu _2}\), and \(H_i\), the Hermite polynomials, satisfy the recurrence relation as
The coefficients \(a_i\)’s are \(a_0 = 1/\left( 2 \pi \mu _2 \right) ^{1/2}\), \( a_1=a_2=0\), \(a_3 = 2^{1/2} a_0 \nu _2 /24\), \(a_4 = a_0 \nu _3 /96.\) Therefore, given the statistical parameters in Eq. (22), the concentration distribution can be estimated from Eq. (23) at any given location in space and time.
4 Solution procedure
To solve Eqs. (16)–(20), a standard finite difference method based on Crank–Nicolson implicit scheme has been adopted. The whole width of the channel is divided into \((M-1)\) equal parts of mesh size \(\varDelta y\), represented by the grid point j and the general formula is \({y_j = y_0 + (j-1) \times \varDelta y}\), where \(y_0\) is the reference hight. The grid point i is assumed for the time index and say the step size is \(\varDelta t\). Then the general formula for time discretization is \({t_i = \varDelta t\times (i-1)}\) and hence the initial time can be obtained for \(i=1\). Thus \(C^{(p)}(i,j)\) indicates the value of \(C^{(p)}\) at the i-th grid point along the t-axis and j-th grid point along y-axis. The resulting finite-difference equations become simultaneous linear algebraic equations with a tridiagonal coefficient matrix:
where \(P_j\), \(Q_j\), \(R_j\) and \(S_j\) are the matrix elements.
Concentration for deposition phase \(W^{(p)}(i)\) can be computed from the relation:
The finite difference form of the initial condition is
and that of boundary conditions are
A matlab code is developed to solve the moment equation (24) using the Thomas algorithm [52]. The computation steps are as follows:
-
(i)
Following the above space discretization, the velocity distribution is derived at all grid points from log-law as [23]
$$\begin{aligned} u(y)=\frac{1-y_0}{y_0-\ln (y_0)-1}\ln \frac{y}{y_0}\quad \mathrm {for}\quad y_0\le y\le 1. \end{aligned}$$(28) -
(ii)
Similarly, the eddy diffusivity \(\varepsilon (y)\) is calculated from
$$\begin{aligned} \left. \begin{array}{lcl} \varepsilon (y) &{}=&{} \left\{ \begin{array}{ll} {\kappa }/{\alpha _1} &{}\quad \text{ Constant, }\\ {\kappa y}/{\alpha _2} &{}\quad \text{ Linear, }\\ \kappa y(1-y) &{}\quad \text{ Parabolic, } \end{array} \right. \\ \end{array} \right. ,\end{aligned}$$(29) -
(iii)
The concentration moment \(C^{(p)}\) is calculated from Eq. (16) as the value of u(y) and \(\varepsilon (y)\) is obtained from the previous steps.
-
(iv)
From Eq. (20), \(W^{(p)}\) is computed with the use of \(C^{(p)}\).
-
(v)
Finally, the suspended sediment concentration distribution C(t, x, y) is obtained from Eq. (23) using the values obtained from steps (i)–(iv). Using the same approach we can calculate the deposited phase concentration W(t, x).
Numerical calculation has been performed for different forms of eddy diffusivity, bed absorbency coefficient, re-entrainment rate, etc., to investigate the concentration distribution of suspended sediment and deposited sediment. Because of Crank–Nicolson implicit method, the numerical scheme is unconditionally stable for any fixed values of \(\varDelta t/ (\varDelta y)^2\). Sufficiently small mesh size of the spatial (\(\varDelta y=(1-y_0)/(M-1)\), \(M=1000\), \(y_0=0.1\)) and temporal (\(\varDelta t=0.00001\)) discretization ensures precision of \(10^{-5}\) in the results.
5 Results and discussion
The present study focuses on unsteady two-dimensional concentration distribution of suspended sediment in an open channel turbulent flow. Two differential equations are used to formulate the problem out of which one describes the suspension and other describes the deposition. The exchange of particles between the flow and the bed is allowed. It has already been stated that the present study solves the suspended sediment transport equation with arbitrary distribution of eddy diffusivity. The method of moments is used because of the significance of each of the central moments on transport of materials as well as it is useful to find the complete concentration distribution using the Hermite polynomial representation of first few central moments. The study can have significant applications on transport of sediment in many natural processes, in particular transport in rivers, because primarily there could be three phases that control sediment transportation in river flow, viz., suspension, deposition and combination of both suspension and deposition phases. They are controlled by some factors such as settling velocity \(\omega _s\), bed absorbency coefficient \(\theta \), re-entrainment rate \(\gamma \), etc. To the best of author’s knowledge, there is hardly any work who has identified the deposition phase separately and in this respect the present work is more significant than the existing works. Due to this reason, it is difficult to validate the model exactly with some published article. But, as Cheng [11] and Liu and Nayamatullah [22] had partially considered this effect, we could still compare the present model with their models under the consideration of the parameter \(\gamma =0\). Fig. 1(a) shows the concentration profile of sediment due to the constant eddy diffusivity for the fixed times \(t= 0, 0.1, 0.4, 0.8\), respectively. This kind of behavior has also been obtained by Cheng [11] when there is no deposition phase. The concentration distribution of suspended sediment along the vertical has been shown in Fig. 1(b) for various forms (viz., constant, linear and parabolic) of eddy diffusivity at a fixed time \(t=0.35\). The result trend qualitatively agrees with Liu and Nayamatullah [22]. In a different work, Mazumder and Paul [54] considered both the phases to understand their effect on the axial dispersion coefficient where they also used method of moments. When \(p=0\), the equation for the zeroth-order concentration moment, \(C_0(y,t)\) in the present case, is similar to Mazumder and Paul [54]. Fig. 2 shows the variation of \(C_0(y,t)\) with respect to channel height for different values of settling velocity and for fixed values of parameters at time \(t=0.5\). As the settling velocity increases, a significant change in the slopes of the distribution is observed which is similar to the findings of Mazumder and Paul [54]. However the quantitative differences realized in the present study are attributed to differing normalization scales adopted in the present study.
Vertical distribution of suspended sediment concentration: (a) for different times and with constant eddy diffusivity, when \(x=0.5\), \(y_0=0.1\), \(\omega _s=0.35\), \(\theta =0.5\), and \(\gamma =0\); (b) for various forms of eddy diffusivity, when \(t=0.35\), \(x=0.5\), \(y_0=0.1\), \(\omega _s=0.2\), \(\theta =0.5\), and \(\gamma =0\)
In the study of suspended sediment transportation, sediment re-entrainment is believed to be of important concern. In oceanic environments, due to the presence of salt ions in water, almost all the suspended sediment may be settled down [55]. This is one of the reasons that oceans and other marine ecosystems tend to have lower turbidity levels (greater water clarity) than freshwater environments. While estuaries and other tidal areas may be considered marine, they are not necessarily clearer than freshwater. Estuaries are the collection point for suspended sediment coming down the river. Furthermore, in a tidal zone, the constant water movement causes the bottom sediment to continuously re-entrained, preventing high water clarity during tidal periods [56]. Actually, at higher flow velocities the settling velocities near the bed may be significantly reduced due to the presence of disruptive velocity gradients in the boundary layer, and thus the larger floes broken down in smaller floes, the latter being resuspended in the flow. The re-entrainment rate coefficient \(\gamma \) measures the amount of sediment particle re-entrained from bed to suspension. From Figs. 3(a, b), it can be seen that with the increase of re-entrainment rate \(\gamma \), the profile of concentration distribution increases, which completely satisfies the nature of the parameter. Further, in Figs. 3(c, d), the effect of bed absorbency coefficient on the distribution of sediment concentration has been observed. Results are well appreciable, because for \(\theta =0\), the area under the distribution curve is maximum whereas for \(\theta =1\) it is minimum. In general, as \(\theta \) increases, the bed absorbency increases and hence the concentration distribution in the vertical direction decreases. It can be seen from Fig. 3 that the distribution tends to its equilibrium state more quicker for \(t=0.5\) than \(t=0.25\) which is obvious.
In Fig. 4, the time assessment of concentration distribution for the deposition phase has been shown under the variation of re-entrainment rate and bed absorbency coefficient, respectively. Deposited phase concentration distribution is found to increase with time and after a fixed time, it reaches to a maximum local peak followed by strictly decreasing function of time [Figs. 4(a, b)]. After a sufficient time the distribution reaches an equilibrium state. It is important to note that as re-entrainment rate increases, the area under distribution curve decreases, whereas an opposite trend has been observed for bed absorbency coefficient. This happens because of the increment of re-entrainment rate or bed absorbency coefficient, larger amount of sediment particle moves from bed to suspension or stuck to bed, respectively. The axial distribution of the deposited phase concentration is given in Fig. 5 for fixed time \(t=0.25\) and 0.5. The peak of the concentration distribution is found to increase as bed absorbency rate \(\theta \) increases [Figs. 5(c, d)], whereas an opposite trend is observed for the re-entrainment rate \(\gamma \) [Figs. 5(a, b)]. The reason behind this kind of behavior of deposited phase concentration distribution completely obeys the nature of parameter \(\theta \) and \(\gamma \). As the time increases, the distributions become flat and also the peak height decreases.
Vertical distribution of suspended sediment concentration for parabolic form of eddy diffusivity when \(x=0.5\), \(y_0=0.1\), and \(\omega _s=0.2\). (a, b) For different values of re-entrainment rate \(\gamma \) and fixed \(\theta =0.5\); (c, d) for different values of bed absorbency coefficient \(\theta \) and fixed \(\gamma =2\)
The response of the total suspended sediment as a function of time subject to re-entrainment rate \(\gamma \) and bed absorbency coefficient \(\theta \) is shown in Fig. 6. The effect of re-entrainment rate \(\gamma \) (for fixed bed absorbency coefficient \(\theta =0.5\)) on the distribution of total suspended sediment is shown in Fig. 6(a) and Fig. 6(b) shows the effect of bed absorbency coefficient \(\theta \) (for fixed re-entrainment rate \(\gamma =2\)). It can be observed from Fig. 6 that during the initial time, the total suspended sediment concentration is maximum. After a small interval of time, a decreasing nature of the sediment concentration is found with time and after a sufficiently large time the total suspended sediment reaches an equilibrium state. Further, it can be seen from Fig. 6(a) that increase in \(\gamma \) leads to increase of the total suspended sediment; as we increase the value of \(\gamma \), the sediment has the tendency to move towards the free surface. An opposite behavior of total suspended sediment due to bed absorbency coefficient can be observed from Fig. 6(b) which is obvious since more amount of sediment settles towards the bed for high absorbency. The effect of \(\gamma \) and \(\theta \) on total suspended sediment flux can be better recognized from Fig. 7 where the sediment flux is found to be an increasing function of \(\gamma \) (Fig. 7(a)), though for a large value of \(\gamma \), the increment is not significant which can also be realized from Fig. 6(a). Further it can be observed from Fig. 7(b) that sediment flux reduces almost linearly as \(\theta \) increases.
Overall, all the behaviors of concentration distribution with the variation of re-entrainment factor \(\gamma \) and bed absorbency coefficient \(\theta \) can well explain the physical phenomena of transportation of sediment. It can be stated that the present work is more updated as compared to several other works existing in literature and can be useful for the modeling of the transport of contaminant associated with fine sediments.
6 Conclusion
The paper investigates the unsteady two-dimensional transport of suspended sediment in an open channel turbulent flow. Apart from the suspension phase, deposition and re-entrainment of particles are considered in the governing equation as well as in the boundary condition. Integral moment method with a standard finite difference implicit scheme is adopted to solve the transport equations. The results are verified with some existing works under limiting condition. Vertical distribution of suspended sediment concentration is plotted with different values of re-entrainment rate and bed absorbency coefficient. The results are physically realistic as it shows that the more is the re-entrainment rate, the more is the concentration in suspension and reverse is the result for absorbency coefficient. A similar kind of results is observed also from the analysis of total suspended sediment. The time variation of concentration distribution and the axial distribution of deposited phase concentration are also investigated. All the results are in accordance with real phenomena and are interpreted physically.
References
Rouse H (1937) Modern conceptions of the mechanics of fluid turbulence. Trans ASCE 102:463–505
Hunt JN (1954) The turbulent transport of suspended sediment in open channels. Proc R Soc Lond Ser A 224(1158):322–335
Ni JR, Qian Wang G (1991) Vertical sediment distribution. J Hydraul Eng 117(9):1184–1194
Umeyama M (1992) Vertical distribution of suspended sediment in uniform open-channel flow. J Hydraul Eng 118(6):936–941
Graf WH, Cellino M (1998) Suspension flow in open channels. Stochastic hydraulics, vol 2000. CRC Press, Boca Raton, pp 85–93
Mazumder BS, Ghoshal K (2006) Velocity and concentration profiles in uniform sediment-laden flow. Appl. Math. Model. 30(2):164–176
Kundu S, Ghoshal K (2014) Effects of secondary current and stratification on suspension concentration in an open channel flow. Environ Fluid Mech 14(6):1357–1380
Kundu S, Ghoshal K (2017) A mathematical model for type ii profile of concentration distribution in turbulent flows. Environ Fluid Mech 17(3):449–472
Mei CC (1969) Nonuniform diffusion of suspended sediment. J Hydraul Div 95(1):581–584
Hjelmfelt AT, Lenau CW (1970) Nonequilibrium transport of suspended sediment. J Hydraul Div HY7
Cheng KJ (1984) Bottom-boundary condition for nonequilibrium transport of sediment. J Geophys Res Oceans 89(C5):8209–8214
van Rijn LC (1986) Mathematical modeling of suspended sediment in nonuniform flows. J Hydraul Eng 112(6):433–455
Coles D (1956) The law of the wake in the turbulent boundary layer. J Fluid Mech 1(2):191–226
Celik I, Rodi W (1988) Modeling suspended sediment transport in nonequilibrium situations. J Hydraul Eng 114(10):1157–1191
Pritchard D (2006) Rate of deposition of fine sediment from suspension. J Hydraul Eng 132(5):533–536
Dorrell RM, Hogg AJ (2011) Length and time scales of response of sediment suspensions to changing flow conditions. J Hydraul Eng 138(5):430–439
Ashida K, Okabe T (1982) On the calculation method of the concentration of suspended sediment under non-equilibrium condition. Proc Jpn Conf Hydraul 26:153–158
Ouillon S, Le Guennec B (1996) Modelling non-cohesive suspended sediment transport in 2d vertical free surface flows. J Hydraul Res 34(2):219–236
Wu W, Wang SS (2007) One-dimensional modeling of dam-break flow over movable beds. J Hydraul Eng 133(1):48–58
Brown GL (2008) Approximate profile for nonequilibrium suspended sediment. J Hydraul Eng 134(7):1010–1014
Zhang S, Duan JG, Strelkoff TS (2013) Grain-scale nonequilibrium sediment-transport model for unsteady flow. J Hydraul Eng 139(1):22–36
Liu X, Nayamatullah M (2014) Semianalytical solutions for one-dimensional unsteady nonequilibrium suspended sediment transport in channels with arbitrary eddy viscosity distributions and realistic boundary conditions. J Hydraul Eng 140(5):04014011
Liu X (2016) Analytical solutions for steady two-dimensional suspended sediment transport in channels with arbitrary advection velocity and eddy diffusivity distributions. J Hydraul Res 54(4):389–398
Jing H, Chen G, Wang W, Li G (2018) Effects of concentration-dependent settling velocity on non-equilibrium transport of suspended sediment. Environ Earth Sci 77(15):549
Claudin P, Charru F, Andreotti B (2011) Transport relaxation time and length scales in turbulent suspensions. J Fluid Mech 671:491–506
Einstein HA (1950) The bed-load function for sediment transportation in open channel flows. 1026. US Government Printing Office
Yalin MS (1977) Mechanics of sediment transport, 2nd edn. Pergamon Press, Oxford
Van Rijn LC (1984) Sediment pick-up functions. J Hydraul Eng 110(10):1494–1502
Parker G, Garcia M, Fukushima Y, Yu W (1987) Experiments on turbidity currents over an erodible bed. J Hydraul Res 25(1):123–147
Abd El-Gawad S, Cantelli A, Pirmez C, Minisini D, Sylvester Z, Imran J (2012) Three-dimensional numerical simulation of turbidity currents in a submarine channel on the seafloor of the niger delta slope. J Geophys Res Oceans 117:C05026
Cantero-Chinchilla FN, Dey S, Castro-Orgaz O, Ali SZ (2015) Hydrodynamic analysis of fully developed turbidity currents over plane beds based on self-preserving velocity and concentration distributions. J Geophys Res Earth Surf 120(10):2176–2199
Revil-Baudard T, Chauchat J (2013) A two-phase model for sheet flow regime based on dense granular flow rheology. J Geophys Res Oceans 118(2):619–634
Chiodi F, Claudin P, Andreotti B (2014) A two-phase flow model of sediment transport: transition from bedload to suspended load. J Fluid Mech 755:561–581
Chauchat J, Cheng Z, Nagel T, Bonamy C, Hsu TJ (2017) Sedfoam-2.0: a 3-d two-phase flow numerical model for sediment transport. Geosci Model Dev 10(12):4367–4392
Cheng Z, Chauchat J, Hsu TJ, Calantoni J (2018) Eddy interaction model for turbulent suspension in reynolds-averaged euler-lagrange simulations of steady sheet flow. Adv Water Resour 111:435–451
Mazumder BS, Ghoshal K, Dalal DC (2005) Influence of bed roughness on sediment suspension: experimental and theoretical studies. J Hydraul Res 43(3):245–257
Dorrell RM, Hogg AJ, Pritchard D (2013) Polydisperse suspensions: Erosion, deposition, and flow capacity. J Geophys Res Earth Surf 118(3):1939–1955
Dorrell RM, Amy LA, Peakall J, McCaffrey WD (2018) Particle size distribution controls the threshold between net sediment erosion and deposition in suspended load dominated flows. Geophys Res Lett 45(3):1443–1452
Cao Z, Carling PA (2002) Mathematical modelling of alluvial rivers: reality and myth. part 2: Special issues. In: Proceedings of the Institution of Civil Engineers-Water and maritime engineering, vol 154, pp 297–307. Thomas Telford Ltd
Sayre WW (1967) Dispersion of mass in open-channel flow. Hydrology papers (Colorado State University); no. 75
Monin AS (1959) On the boundary condition on the earth surface for diffusing pollution. Adv Geophys 6:435–436
Dobbins WE (1943) Effect of turbulence on sedimentation. Proc Am Soc Civil Eng 69(2):235–262
Cui Y (2007) The unified gravel-sand (tugs) model: simulating sediment transport and gravel/sand grain size distributions in gravel-bedded rivers. Water Resour Res 43(10):W10436
Sulaiman M, Fujita M, Tsutsumi D (2007) Bed variation model considering porosity change in riverbed material. J Japan Soc Eros Control Eng 60(1):11–18
Horvat Z, Isic M, Spasojevic M (2015) Two dimensional river flow and sediment transport model. Environ Fluid Mech 15(3):595–625
Bui VH, Bui MD, Rutschmann P (2019) Advanced numerical modeling of sediment transport in gravel-bed rivers. Water 11(3):550
Aris R (1956) On the dispersion of a solute in a fluid flowing through a tube. Proc R Soc Lond Ser A 235(1200):67–77
Mehta RV, Merson RL, McCoy BJ (1974) Hermite polynomial representation of chromatography elution curves. J Chromatogr A 88(1):1–6
Wang P, Chen GQ (2016) Transverse concentration distribution in Taylor dispersion: Gill’s method of series expansion supported by concentration moments. Int J Heat Mass Transf 95:131–141
Debnath S, Saha AK, Mazumder BS, Roy AK (2019) Transport of a reactive solute in a pulsatile non-Newtonian liquid flowing through an annular pipe. J Eng Math 116(1):1–22
Debnath S, Saha AK, Mazumder BS, Roy AK (2017) Dispersion phenomena of reactive solute in a pulsatile flow of three-layer liquids. Phys Fluids 29(9):097107
Anderson DA, Tannehill JC, Pletcher RH (1984) Computational fluid mechanics and heat transfer. Hemisphere Publishing Corporation, New York
Van Rijn LC (1987) Mathematical modelling of morphological processes in the case of suspended sediment transport
Mazumder BS, Paul S (2012) Dispersion of settling particles in oscillatory turbulent flow subject to deposition and re-entrainment. Eur J Mech B 31:80–90
Håkanson L (2006) The relationship between salinity, suspended particulate matter and water clarity in aquatic systems. Ecol Res 21(1):75–90
Hathaway JC, Nelson BW (1973) Environmental framework of coastal plain estuaries, vol 133. Geological Society of America
Acknowledgements
The authors greatly acknowledge the valuable suggestions and comments of editor and reviewers. They are thankful to the Science and Engineering Research Board (SERB), Department of Science and Technology (DST), Govt. of India, for providing the funding through the research project with No. EMR/2015/002434. The authors are thankful to Prof. Subhasish Dey, Dept. of Civil Engineering, IIT Kharagpur, the advisor of this project, for his valuable suggestions.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Debnath, S., Ghoshal, K. & Kumar, J. Unsteady two-dimensional suspended sediment transport in open channel flow subject to deposition and re-entrainment. J Eng Math 126, 6 (2021). https://doi.org/10.1007/s10665-020-10070-7
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10665-020-10070-7