Skip to main content
SpringerLink
Log in

Stability and dynamics of self-similarity in evolution equations

  • Published:
Journal of Engineering Mathematics Aims and scope Submit manuscript

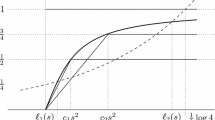
Abstract

A methodology for studying the linear stability of self-similar solutions is discussed. These fundamental ideas are illustrated on three prototype problems: a simple ODE with finite-time blow-up, a second-order semi-linear heat equation with infinite-time spreading solutions, and the fourth-order Sivashinsky equation with finite-time self-similar blow-up. These examples are used to show that self-similar dynamics can be studied using many of the ideas arising in the study of dynamical systems. In particular, the use of dimensional analysis to derive scaling invariant similarity variables is discussed, as well as the role of symmetries in the context of stability of self-similar dynamics. The spectrum of the linear stability problem determines the rate at which the solution will approach a self-similar profile. For blow-up solutions it is demonstrated that the symmetries give rise to positive eigenvalues associated with the symmetries, and it is shown how this stability analysis can identify a unique stable (and observable) attracting solution from a countable infinity of similarity solutions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Barenblatt GI (1996) Scaling, self-similarity, and intermediate asymptotics. Cambridge University Press, Cambridge, p 386

    MATH  Google Scholar 

  2. Barenblatt GI (2003) Scaling. Cambridge University Press, Cambridge, p 171

    MATH  Google Scholar 

  3. Kadanoff LP (1997) Singularities and blowups. Phys Today 50: 11–13

    Article  Google Scholar 

  4. Eggers J, Fontelos MA (2009) The role of self-similarity in singularities of partial differential equations. Nonlinearity 22: R1–R44

    Article  MATH  MathSciNet  Google Scholar 

  5. Tanner LH (1979) The spreading of silicone oil drops on horizontal surfaces. J Phys D 12: 1473–1484

    Article  ADS  Google Scholar 

  6. Oron A, Davis SH, Bankoff SG (1997) Long-scale evolution of thin liquid films. Rev Mod Phys 69: 931–980

    Article  ADS  Google Scholar 

  7. Witelski TP, Bernoff AJ (1998) Self-similar asymptotics for linear and nonlinear diffusion equations. Stud Appl Math 100: 153–193

    Article  MATH  MathSciNet  Google Scholar 

  8. Zel’dovich YB, Raizer YP (2002) Physics of shock waves and high temperature hydrodynamic phenomena. Dover, New York, p 944

    Google Scholar 

  9. Kleinstein G, Ting L (1971) Optimum one-term solutions for heat conduction problems. Z Angew Math Mech 51: 1–16

    Article  MATH  MathSciNet  Google Scholar 

  10. Kloosterziel RC (1990) On the large-time asymptotics of the diffusion equation on infinite domains. J Eng Math 24: 213–236

    Article  MATH  MathSciNet  Google Scholar 

  11. Bernoff AJ, Lingevitch JF (1994) Rapid relaxation of an axisymmetric vortex. Phys Fluids 6: 3717–3723

    Article  MATH  ADS  Google Scholar 

  12. Gallay T, Wayne CE (2002) Invariant manifolds and the long-time asymptotics of the Navier–Stokes and vorticity equations on R 2. Arch Ration Mech Anal 163: 209–258

    Article  MATH  MathSciNet  Google Scholar 

  13. Bebernes J, Eberly D (1989) Mathematical problems from combustion theory vol 83 of Applied Mathematical Sciences. Springer, New York, p 177

    Google Scholar 

  14. Stevens A, Othmer HG (1997) Aggregation, blowup, and collapse. SIAM J Appl Math 57: 1044–1081

    Article  MATH  MathSciNet  Google Scholar 

  15. Levine HA (1989) Quenching, nonquenching, and beyond quenching for solution of some parabolic equations. Ann Math Pura Appl 155: 243–260

    Article  MATH  Google Scholar 

  16. Galaktionov VA, Vázquez JL (2002) The problem of blow-up in nonlinear parabolic equations. Discret Contin Dyn Syst 8: 399–433

    Article  MATH  Google Scholar 

  17. Guo YJ, Pan ZG, Ward MJ (2005) Touchdown and pull-in voltage behavior of a MEMS device with varying dielectric properties. SIAM J Appl Math 66: 309–338

    Article  MATH  MathSciNet  Google Scholar 

  18. Flores G, Mercado G, Pelesko JA, Smyth N (2007) Analysis of the dynamics and touchdown in a model of electrostatic MEMS. SIAM J Appl Math 67: 434–446

    Article  MATH  MathSciNet  Google Scholar 

  19. Brenner MP, Lister JR, Stone HA (1996) Pinching threads, singularities and the number 0.0304.... Phys Fluids 8: 2827–2836

    Article  MATH  MathSciNet  ADS  Google Scholar 

  20. Eggers J, Villermaux E (2008) Physics of liquid jets. Rep Prog Phys 71: 1–79

    Article  Google Scholar 

  21. Bernoff AJ, Bertozzi AL, Witelski TP (1998) Axisymmetric surface diffusion: dynamics and stability of self-similar pinchoff. J Stat Phys 93: 725–776

    Article  MATH  MathSciNet  ADS  Google Scholar 

  22. Zhang WW, Lister JR (1999) Similarity solutions for van der Waals rupture of a thin film on a solid substrate. Phys Fluids 11: 2454–2462

    Article  MATH  MathSciNet  ADS  Google Scholar 

  23. Vaynblat D, Lister JR, Witelski TP (2001) Rupture of thin viscous films by van der Waals forces I: evolution and self-similarity. Phys Fluids 13: 1130–1140

    Article  ADS  Google Scholar 

  24. Vaynblat D, Lister JR, Witelski TP (2001) Symmetry and self-similarity in rupture and pinchoff: a geometric bifurcation. Eur J Appl Math 12: 209–232

    Article  MATH  MathSciNet  Google Scholar 

  25. Witelski TP, Bernoff AJ (1999) Stability of self-similar solutions for van der Waals driven thin film rupture. Phys Fluids 11: 2443–2445

    Article  MATH  MathSciNet  ADS  Google Scholar 

  26. Witelski TP, Bernoff AJ (2000) Dynamics of three-dimensional thin film rupture. Physica D 147: 155–176

    Article  MATH  MathSciNet  ADS  Google Scholar 

  27. Levine HA (1990) The role of critical exponents in blowup theorems. SIAM Rev 32: 262–288

    Article  MATH  MathSciNet  Google Scholar 

  28. Bandle C, Brunner H (1998) Blowup in diffusion equations: a survey. J Comput Appl Math 97: 3–22

    Article  MATH  MathSciNet  Google Scholar 

  29. Samarskii AA, Galaktionov VA, Kurdyumov SP, Mikhailov AP (1995) Blow-up in quasilinear parabolic equations. Walter de Gruyter & Co, Berlin, p 535

    MATH  Google Scholar 

  30. Hydon PE (2000) Symmetry methods for differential equations. Cambridge University Press, Cambridge, p 213

    MATH  Google Scholar 

  31. Bluman GW, Anco SC (2002) Symmetry and integration methods for differential equations. Springer, New York, p 419

    MATH  Google Scholar 

  32. Dresner L (1999) Applications of Lie’s theory of ordinary and partial differential equations. Institute of Physics Publishing, Bristol, p 225

    Book  MATH  Google Scholar 

  33. Olver PJ (1993) Applications of Lie groups to differential equations. Springer, New York, p 513

    MATH  Google Scholar 

  34. Merle F, Zaag H (2002) O.D.E. type behavior of blow-up solutions of nonlinear heat equations. Discret Contin Dyn Syst 8: 435–450

    Article  MATH  MathSciNet  Google Scholar 

  35. Berger M, Kohn RV (1988) A rescaling algorithm for the numerical calculation of blowing-up solutions. Commun Pure Appl Math 41: 841–863

    Article  MATH  MathSciNet  Google Scholar 

  36. Giga Y, Kohn RV (1985) Asymptotically self-similar blow-up of semilinear heat equations. Commun Pure Appl Math 38: 297–319

    Article  MATH  MathSciNet  Google Scholar 

  37. Giga Y, Kohn RV (1987) Characterizing blowup using similarity variables. Indiana Univ Math J 36: 1–40

    Article  MATH  MathSciNet  Google Scholar 

  38. Velazquez JJ, Galaktionov VA, Herrero MA (1991) The space structure near a blow-up point for semilinear heat equations: a formal approach. Comput Math Math Phys 31: 46–55

    MathSciNet  Google Scholar 

  39. Galaktionov VA, Kurdyumov SP, Samarskiĭ AA (1985) Asymptotic “eigenfunctions” of the Cauchy problem for a nonlinear parabolic equation. Mat Sb (N.S.) 126(168):435–472, 592

    Google Scholar 

  40. Kamin S, Peletier LA (1985) Singular solutions of the heat equation with absorption. Proc Am Math Soc 95: 205–210

    Article  MATH  MathSciNet  Google Scholar 

  41. Brezis H, Peletier LA, Terman D (1986) A very singular solution of the heat equation with absorption. Arch Ration Mech Anal 95: 185–209

    Article  MATH  MathSciNet  Google Scholar 

  42. Wayne CE (1997) Invariant manifolds for parabolic partial differential equations on unbounded domains. Arch Ration Mech Anal 138: 279–306

    Article  MATH  MathSciNet  Google Scholar 

  43. Bricmont J, Kupiainen A, Lin G (1994) Renormalization group and asymptotics of solutions of nonlinear parabolic equations. Commun Pure Appl Math 47: 893–922

    Article  MATH  MathSciNet  Google Scholar 

  44. Bricmont J, Kupiainen A (1996) Stable non-Gaussian diffusive profiles. Nonlinear Anal 26: 583–593

    Article  MATH  MathSciNet  Google Scholar 

  45. Filippas S, Kohn RV (1992) Refined asymptotics for the blowup of u t −δu = u p. Commun Pure Appl Math 45: 821–869

    Article  MATH  MathSciNet  Google Scholar 

  46. Bebernes J, Bricher S (1992) Final time blowup profiles for semilinear parabolic equations via center manifold theory. SIAM J Math Anal 23: 852–869

    Article  MATH  MathSciNet  Google Scholar 

  47. Galaktionov VA, Williams JF (2004) On very singular similarity solutions of a higher-order semilinear parabolic equation. Nonlinearity 17: 1075–1099

    Article  MATH  MathSciNet  ADS  Google Scholar 

  48. Galaktionov VA, Vázquez JL (2004) A stability technique for evolution partial differential equations: a dynamical systems approach. Birkhäuser, Boston, MA, p 377

    MATH  Google Scholar 

  49. Sarocka DC, Bernoff AJ, Rossi LF (1999) Large-amplitude solutions to the Sivashinsky and Riley–Davis equations for directional solidification. Physica D 127: 146–176

    Article  MATH  MathSciNet  ADS  Google Scholar 

  50. Sivashinsky GI (1983) On cellular instability in the solidification of a dilute binary alloy. Physica D 8: 243–248

    Article  MathSciNet  ADS  Google Scholar 

  51. Childress S, Spiegel EA (2004) Pattern formation in a suspension of swimming microorganisms: nonlinear aspects. In: Givoli D, Grote MJ, Papanicolaou GC (eds) A celebration of mathematical modeling. Kluwer, Dordrecht, pp 33–52

    Google Scholar 

  52. Novick-Cohen A (1990) On Cahn-Hilliard type equations. Nonlinear Anal 15: 797–814

    Article  MATH  MathSciNet  Google Scholar 

  53. Novick-Cohen A (1992) Blow up and growth in the directional solidification of dilute binary alloys. Appl Anal 47: 241–257

    Article  MathSciNet  Google Scholar 

  54. Straughan B (1998) Explosive instabilities in mechanics. Springer, Berlin, p 196

    MATH  Google Scholar 

  55. Bernoff AJ, Bertozzi AL (1995) Singularities in a modified Kuramoto–Sivashinsky equation describing interface motion for phase transition. Physica D 85: 375–404

    Article  MATH  MathSciNet  Google Scholar 

  56. Bertozzi AL, Pugh MC (1998) Long-wave instabilities and saturation in thin film equations. Commun Pure Appl Math 51: 625–661

    Article  MathSciNet  Google Scholar 

  57. Bertozzi AL, Pugh MC (2000) Finite-time blow-up of solutions of some long-wave unstable thin film equations. Indiana Univ Math J 49: 1323–1366

    Article  MATH  MathSciNet  Google Scholar 

  58. Evans JD, Galaktionov VA, King JR (2007) Source-type solutions of the fourth-order unstable thin film equation. Eur J Appl Math 18: 273–321

    Article  MATH  MathSciNet  Google Scholar 

  59. Witelski TP, Bernoff AJ, Bertozzi AL (2004) Blowup and dissipation in a critical-case unstable thin film equation. Eur J Appl Math 15: 223–256

    Article  MATH  MathSciNet  Google Scholar 

  60. Hocherman T, Rosenau P (1993) On KS-type equations the evolution and rupture of a liquid interface. Physica D 67: 113–125

    Article  MATH  ADS  Google Scholar 

  61. Gandarias ML, Ibragimov NH (2008) Equivalence group of a fourth-order evolution equations unifying various non-linear models. Commun Nonlinear Sci Numer Simul 13: 259–268

    Article  MATH  MathSciNet  ADS  Google Scholar 

  62. Levine HA (1973) Some nonexistence and instability theorems for solutions of formally parabolic equations of the form \({Pu_{t}=-Au+{\mathcal F}(u)}\). Arch Ration Mech Anal 51: 371–386

    Article  MATH  Google Scholar 

  63. Evans JD, Galaktionov VA, Williams JF (2006) Blow-up and global asymptotics of the limit unstable Cahn–Hilliard equation. SIAM J Math Anal 38: 64–102

    Article  MATH  MathSciNet  Google Scholar 

  64. Witelski TP (2002) Computing finite-time singularities in interfacial flows. In: Bourlioux A, Gander MJ (eds) Modern methods in scientific computing and applications. Kluwer, Dordrecht, pp 451–487

    Google Scholar 

  65. Budd CJ, Piggott MD (2001) The geometric integration of scale-invariant ordinary and partial differential equations. J Comput Appl Math 128: 399–422

    Article  MATH  MathSciNet  Google Scholar 

  66. Budd CJ, Piggott MD (2003) Geometric integration and applications. In: Cucker F (eds) Handbook of numerical analysis, vol XI. Elsevier/North Holland, Amsterdam, pp 35–139

    Google Scholar 

  67. Bernis F, Peletier LA (1996) Two problems from draining flows involving third-order ordinary differential equations. SIAM J Math Anal 27: 515–527

    Article  MATH  MathSciNet  Google Scholar 

  68. Boatto S, Kadanoff LP, Olla P (1993) Traveling-wave solutions to thin-film equations. Phys Rev E 48: 4423–4431

    Article  MathSciNet  ADS  Google Scholar 

  69. Carr J (1981) Applications of centre manifold theory. Springer, New York, p 142

    MATH  Google Scholar 

  70. Guckenheimer J, Holmes P (1990) Nonlinear oscillations, dynamical systems, and bifurcations of vector fields. Springer, New York, p 459

    Google Scholar 

  71. Evans JD, Galaktionov VA, King JR (2007) Unstable sixth-order thin film equation. I. Blow-up similarity solutions. Nonlinearity 20: 1799–1841

    Article  MATH  MathSciNet  ADS  Google Scholar 

  72. Budd CJ, Galaktionov VA, Williams JF (2004) Self-similar blow-up in higher-order semilinear parabolic equations. SIAM J Appl Math 64: 1775–1809

    Article  MATH  MathSciNet  Google Scholar 

  73. Budd CJ, Rottschäfer V, Williams JF (2005) Multibump, blow-up, self-similar solutions of the complex Ginzburg-Landau equation. SIAM J Appl Dyn Syst 4: 649–678

    Article  MATH  MathSciNet  Google Scholar 

  74. Palais B (1988) Blowup for nonlinear equations using a comparison principle in Fourier space. Commun Pure Appl Math 41: 165–196

    Article  MATH  MathSciNet  Google Scholar 

  75. Chapman SJ, King JR, Adams KL (1998) Exponential asymptotics and Stokes lines in nonlinear ordinary differential equations. Proc R Soc Lond Ser A 454: 2733–2755

    Article  MATH  MathSciNet  Google Scholar 

  76. Ascher UM, Mattheij RMM, Russell RD (1995) Numerical solution of boundary value problems for ordinary differential equations. Society for Industrial and Applied Mathematics, Philadelphia, PA, p 595

    MATH  Google Scholar 

  77. Burke J, Knobloch E (2007) Homoclinic snaking:structure and stability. Chaos 17: 037102

    Article  MathSciNet  ADS  Google Scholar 

  78. Beck M, Knobloch J, Lloyd D, Sandstede B, Wagenknecht T (2009) Snakes, ladders, and isolas of localized patterns. SIAM J Math Anal 41: 936–972

    Article  MathSciNet  Google Scholar 

  79. Rottschäfer V, Kaper TJ (2003) Geometric theory for multi-bump, self-similar, blowup solutions of the cubic nonlinear Schrödinger equation. Nonlinearity 16: 929–961

    Article  MATH  MathSciNet  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Harvey Mudd College, Claremont, CA, 91711, USA

    Andrew J. Bernoff

  2. Department of Mathematics, Duke University, Durham, NC, 27708, USA

    Thomas P. Witelski

Authors
  1. Andrew J. Bernoff
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Thomas P. Witelski
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Thomas P. Witelski.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Bernoff, A.J., Witelski, T.P. Stability and dynamics of self-similarity in evolution equations. J Eng Math 66, 11–31 (2010). https://doi.org/10.1007/s10665-009-9309-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10665-009-9309-8

Keywords

Search

Navigation