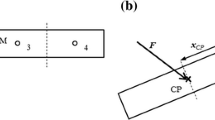
Abstract
Wood transport during high flow events is here treated as an advective–diffusive phenomenon. A theoretical definition of the governing equation is first provided, highlighting the dependence of floating wood diffusion on the adoption of adequate diffusion coefficients. To estimate these coefficients, an experimental investigation on large wood debris was carried out in a channel presenting a weakly sinusoidal plant, employing regular cylinders with various sizes under different flow conditions. For each configuration, a consistent number of trajectories for the floating wood were acquired and processed through imaging techniques, allowing for a statistical analysis of the wood dynamics. The tests showed that floating logs travel at a velocity lower than the water surface one, not completely aligned to the flow direction and tend to disperse streamwise and transversely. Under the assumption that wood dispersion can be derived from the analysis of wood trajectories fluctuations, the longitudinal, transversal and angular diffusion coefficients were computed. Finally, a preliminary dimensional analysis is presented discussing the relevant spatial and temporal scales for these coefficients.


















Similar content being viewed by others
References
Alonso CV (2004) Transport mechanics of stream-borne logs. Riparian Veg Fluv Geomorphol 8:59
Arnoux-Chiavassa S, Rey V, Fraunie P (1999) Modelling of suspended sediment fluxes off the Rhone river mouth. J Coast Res 15:61–73
Arya SP et al (1999) Air pollution meteorology and dispersion, vol 310. Oxford University Press, New York
Batchelor G, Binnie A, Phillips O (1955) The mean velocity of discrete particles in turbulent flow in a pipe. Proc Phys Soc Sect B 68(12):1095. https://doi.org/10.1088/0370-1301/68/12/314
Beer T, Young PC (1983) Longitudinal dispersion in natural streams. J Environ Eng 109(5):1049–1067. https://doi.org/10.1061/(ASCE)0733-9372(1983)109:5(1049)
Braudrick CA, Grant GE (2000) When do logs move in rivers? Water Resour Res 36(2):571–583. https://doi.org/10.1029/1999WR900290
Braudrick CA, Grant GE (2001) Transport and deposition of large woody debris in streams: a flume experiment. Geomorphology 41(4):263–283. https://doi.org/10.1016/S0169-555X(01)00058-7
Brunner GW (2010) HEC–RAS River Analysis System–Hyrdaulic Reference Manual. U.S. Army Corps of Engineers, Institute for Water Resources, Hydrologic Engineering Centre, Washington, DC
Comiti F, Andreoli A, Lenzi M, Mao L (2006) Spatial density and characteristics of woody debris in five mountain rivers of the dolomites (Italian alps). Geomorphology 78(1–2):44–63. https://doi.org/10.1016/j.geomorph.2006.01.021
Comiti F, Mao L, Preciso E, Picco L, Marchi L, Borga M (2008) Large wood and flash floods: evidence from the 2007 event in the Davča basin (Slovenia). WIT Trans Eng Sci 60:173–182
Comiti F, Lucía A, Rickenmann D (2016) Large wood recruitment and transport during large floods: a review. Geomorphology 269:23–39. https://doi.org/10.1016/j.geomorph.2016.06.016
Corsini A, Ciccarese G, Diena M, Truffelli G, Alberoni P, Amorati R (2017) Debris flows in Val Parma and Val Baganza (northern Apennines) during the 12–13th October 2014 alluvial event in Parma province (Italy). Ital J Eng Geol Environ. https://doi.org/10.4408/IJEGE.2017-01.S-03
Costabile P, Macchione F, Natale L, Petaccia G (2015) Comparison of scenarios with and without bridges and analysis of backwater effect in 1-d and 2-d river flood modeling. Comput Modell Eng Sci 109(2):181–204
Critchell K, Lambrechts J (2016) Modelling accumulation of marine plastics in the coastal zone; what are the dominant physical processes? Estuar Coast Shelf Sci 171:111–122. https://doi.org/10.1016/j.ecss.2016.01.036
Critchell K, Grech A, Schlaefer J, Andutta F, Lambrechts J, Wolanski E, Hamann M (2015) Modelling the fate of marine debris along a complex shoreline: lessons from the great barrier reef. Estuar Coast Shelf Sci 167:414–426. https://doi.org/10.1016/j.ecss.2015.10.018
D’Agostino V, Degetto M, Righetti M (2000) Experimental investigation on open check dam for coarse woody debris control. Dynamics of water and sediments in mountain basins. Quad Idronomia Mont 20:201–212. https://doi.org/10.1061/(ASCE)HY.1943-7900.0001049
Davidson S, MacKenzie L, Eaton B (2015) Large wood transport and jam formation in a series of flume experiments. Water Resour Res 51(12):10065–10077. https://doi.org/10.1002/2015WR017446
DeCicco P, Solari L, Paris E (2015) Bridge clogging caused by woody debris: experimental analysis on the effect of pier shape. In: Proceedings of the third international conference of wood in world rivers. https://doi.org/10.1061/(ASCE)HY.1943-7900.0000319
Degetto M, Righetti M (2004) Dynamic of wood transport in torrents. In: Proceedings of the 10th International Congress INTERPRAEVENT, Riva del Garda, pp 24–27
Division S (2000) Guideline for driftwood countermeasures (proposal and design). SC Sabo Department, Ed, Ministry of Construction, Japan, p 42
Elder J (1959) The dispersion of marked fluid in turbulent shear flow. J Fluid Mech 5(4):544–560. https://doi.org/10.1017/S0022112059000374
Fischer HB (1967) The mechanics of dispersion in natural streams. J Hydraul Div 93(6):187–216. https://doi.org/10.4236/jwarp.2010.211114
Fischer HB (1969) The effect of bends on dispersion in streams. Water Resour Res 5(2):496–506. https://doi.org/10.1029/WR005i002p00496
Fischer HB (1973) Longitudinal dispersion and turbulent mixing in open-channel flow. Annu Rev Fluid Mech 5(1):59–78. https://doi.org/10.1146/annurev.fl.05.010173.000423
Furlan P, Pfister M, Matos J, Amado C, Schleiss AJ (2018) Experimental repetitions and blockage of large stems at ogee crested spillways with piers. J Hydraul Res. https://doi.org/10.1080/00221686.2018.1478897
Goring DG, Nikora VI (2002) Despiking acoustic doppler velocimeter data. J Hydraul Eng 128(1):117–126. https://doi.org/10.1061/(ASCE)0733-9429(2002)128:1(117)
Gschnitzer T, Gems B, Mazzorana B, Aufleger M (2017) Towards a robust assessment of bridge clogging processes in flood risk management. Geomorphology 279:128–140. https://doi.org/10.1016/j.geomorph.2016.11.002
Gurnell A (2012) Fluvial geomorphology: wood and river landscapes. Nat Geosci 5(2):93. https://doi.org/10.1038/ngeo1382
Hartlieb A (2015) Schwemmholz in Fließgewässern: Gefahren und Lösungsmöglichkeiten/von Arnd Hartlieb.[TUM, Technische Universität München, Lehrstuhl für Wasserbau und Wasserwirtschaft; Versuchsanstalt für Wasserbau und Wasserwirtschaft (Oskar-von-Miller-Institut)]. TUM, Lehrstuhl für Wasserbau und Wasserwirtschaft
Kashefipour SM, Falconer RA (2002) Longitudinal dispersion coefficients in natural channels. Water Res 36(6):1596–1608. https://doi.org/10.1016/S0043-1354(01)00351-7
Kimura I, Kitazono K (2018) Studies on driftwood motions around obstacles by laboratory and numerical experiments. In: E3S Web of Conferences, vol 40. https://doi.org/10.1051/e3sconf/20184002032
Kramer N, Wohl E (2017) Rules of the road: a qualitative and quantitative synthesis of large wood transport through drainage networks. Geomorphology 279:74–97. https://doi.org/10.1016/j.geomorph.2016.08.026
Lollino G, Arattano M, Rinaldi M, Giustolisi O, Marechal JC, Grant GE (2014) Engineering geology for society and territory-volume 3: river basins, reservoir sedimentation and water resources. Springer, Berlin
Lucía A, Comiti F, Borga M, Cavalli M, Marchi L (2015) Dynamics of large wood during a flash flood in two mountain catchments. Nat Hazards Earth Syst Sci 15(8):1741. https://doi.org/10.5194/nhessd-3-1643-2015
Marchi L, Borga M, Preciso E, Sangati M, Gaume E, Bain V, Delrieu G, Bonnifait L, Pogačnik N (2009) Comprehensive post-event survey of a flash flood in Western Slovenia: observation strategy and lessons learned. Hydrol Process Int J 23(26):3761–3770
Mazzorana B (2009) Woody debris recruitment prediction methods and transport analysis. Dissertation, Institute of Mountain Risk Engineering, University of Natural Resources and Applied Life Sciences, Vienna
Mazzorana B, Comiti F, Volcan C, Scherer C (2011) Determining flood hazard patterns through a combined stochastic–deterministic approach. Nat Hazards 59(1):301–316. https://doi.org/10.1007/s11069-011-9755-2
Mazzorana B, Formaggioni O, Macconi P, Marangoni N, Lucía A, Comiti F, Rigon E, Tonon A, Garcia Rama A, Ravazzolo D, Rainato R, Moretto J, Delai F (2015) Retracing wood dynamics during an extreme flood event in South Tyrol, Italy. In: Proceedings of the third international conference wood in world rivers, pp 92–95
Morales-Hernández M, Murillo J, Garcıa-Navarro P (2018) Diffusion–dispersion numerical discretization for solute transport in transient shallow flows. Environ Fluid Mech. https://doi.org/10.1007/s10652-018-9644-2
Nakagawa H (1994) Driftwood behavior by overland flood flows. J Hydrosci Hydraul Eng 12(2):31–39. https://doi.org/10.2208/prohe.37.379
Nakagawa H, Inoue K, Ikeguchi M, Tsubono T (1995) Behavior of driftwood and the process of its damming up. J Hydrosci Hydraul Eng 13(2):55–67
Persi E, Petaccia G, Sibilla S (2018) Large wood transport modelling by a coupled Eulerian–Lagrangian approach. Nat Hazards 91(1):59–74. https://doi.org/10.1007/s11069-017-2891-6
Persi E, Petaccia G, Sibilla S, Brufau P, García-Navarro P (2018) Calibration of a dynamic Eulerian–Lagrangian model for the computation of wood cylinders transport in shallow water flow. J Hydroinform. https://doi.org/10.2166/hydro.2018.085
Pimpunchat B, Sweatman WL, Wake GC, Triampo W, Parshotam A (2009) A mathematical model for pollution in a river and its remediation by aeration. Appl Math Lett 22(3):304–308. https://doi.org/10.1016/j.aml.2008.03.026
Piton G, Recking A (2016) Design of sediment traps with open check dams. II: woody debris. J Hydraul Eng 142(2):04015046. https://doi.org/10.1061/(ASCE)HY.1943-7900.0001049
Ratia H, Murillo J, García-Navarro P (2014) Numerical modelling of bridges in 2D shallow water flow simulations. Int J Numer Methods Fluids 75(4):250–272
Ruiz-Villanueva V, Bodoque J, Díez-Herrero A, Eguibar M, Pardo-Igúzquiza E (2013) Reconstruction of a flash flood with large wood transport and its influence on hazard patterns in an ungauged mountain basin. Hydrol Process 27(24):3424–3437. https://doi.org/10.1002/hyp.9433
Ruiz-Villanueva V, Bladé E, Sanchez-Juny M, Marti-Cardona B, Díez-Herrero A, Bodoque JM (2014) Two-dimensional numerical modeling of wood transport. J Hydroinform 16(5):1077–1096. https://doi.org/10.2166/hydro.2014.026
Ruiz-Villanueva V, Piégay H, Gurnell AM, Marston RA, Stoffel M (2016) Recent advances quantifying the large wood dynamics in river basins: new methods and remaining challenges. Rev Geophys 54(3):611–652. https://doi.org/10.1002/2015RG000514
Ruiz-Villanueva V, Wyzga B, Hajdukiewicz H, Stoffel M (2016) Exploring large wood retention and deposition in contrasting river morphologies linking numerical modelling and field observations. Earth Surface Process Landf 41(4):446–459. https://doi.org/10.1002/esp.3832
Schmocker L, Hager WH (2011) Probability of drift blockage at bridge decks. J Hydraul Eng 137(4):470–479. https://doi.org/10.1061/(ASCE)HY.1943-7900.0000319
Schmocker L, Weitbrecht V (2013) Driftwood: risk analysis and engineering measures. J Hydraul Eng 139(7):683–695. https://doi.org/10.1061/(ASCE)HY.1943-7900.0000728
Shrestha BB, Nakagawa H, Kawaike K, Baba Y, Zhang H (2012) Driftwood deposition from debris flows at slit-check dams and fans. Nat Hazards 61(2):577–602. https://doi.org/10.1007/s11069-011-9939-9
Silvestro F, Rebora N, Giannoni F, Cavallo A, Ferraris L (2016) The flash flood of the Bisagno Creek on 9th october 2014: an “unfortunate” combination of spatial and temporal scales. J Hydrol 541:50–62. https://doi.org/10.1016/j.jhydrol.2015.08.004
Stockstill RL, Daly SF, Hopkins MA (2009) Modeling floating objects at river structures. J Hydraul Eng 135(5):403–414. https://doi.org/10.1061/(ASCE)0733-9429(2009)135:5(403)
Sullivan PJ (1971) Longitudinal dispersion within a two-dimensional turbulent shear flow. J Fluid Mech 49(3):551–576. https://doi.org/10.1017/S0022112071002258
Syvitski JP, Skene KI, Nicholson MK, Morehead MD (1998) Plume1.1: deposition of sediment from a fluvial plume. Comput Geosci 24(2):159–171. https://doi.org/10.1016/S0098-3004(97)00084-8
Taylor GI (1953) Dispersion of soluble matter in solvent flowing slowly through a tube. Proc R Soc Lond A 219(1137):186–203. https://doi.org/10.1098/rspa.1953.0139
Vanderwel C, Tavoularis S (2014) Measurements of turbulent diffusion in uniformly sheared flow. J Fluid Mech 754:488–514. https://doi.org/10.1017/jfm.2014.406
Vanzo D, Siviglia A, Toro EF (2016) Pollutant transport by shallow water equations on unstructured meshes: hyperbolization of the model and numerical solution via a novel flux splitting scheme. J Comput Phys 321:1–20. https://doi.org/10.1016/j.jcp.2016.05.023
Wohl E, Cenderelli DA, Dwire KA, Ryan-Burkett SE, Young MK, Fausch KD (2010) Large in-stream wood studies: a call for common metrics. Earth Surf Process Landf J Br Geomorphol Res Group 35(5):618–625. https://doi.org/10.1002/esp.1966
Yotsukura N, Sayre WW (1976) Transverse mixing in natural channels. Water Resour Res 12(4):695–704. https://doi.org/10.1029/WR012i004p00695
Acknowledgements
The authors would like to thank the EU and MIUR for funding, in the frame of the collaborative international consortium STEEP STREAMS financed under the ERA-NET Cofund WaterWorks2014 Call. This ERA-NET is an integral part of the 2015 Joint Activities developed by the Water Challenges for a Changing World Joint Programme Initiative (Water JPI). We are also grateful to the technicians of the Hydraulic Laboratory of the University of Trento Andrea Bampi, Lorenzo Forti, Fabio Sartori and Paolo Scarfiello for their support in the experimental investigation.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Below is the link to the electronic supplementary material.
Rights and permissions
About this article
Cite this article
Meninno, S., Persi, E., Petaccia, G. et al. An experimental and theoretical analysis of floating wood diffusion coefficients. Environ Fluid Mech 20, 593–617 (2020). https://doi.org/10.1007/s10652-019-09693-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10652-019-09693-x