State-dependent Control of a Single Stage Hybrid System with Poisson Arrivals

Abstract

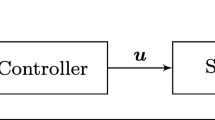
We consider a single-stage hybrid manufacturing system where jobs arrive according to a Poisson process. These jobs undergo a deterministic process which is controllable. We define a stochastic hybrid optimal control problem and decompose it hierarchically to a lower-level and a higher-level problem. The lower-level problem is a deterministic optimal control problem solved by means of calculus of variations. We concentrate on the stochastic discrete-event control problem at the higher level, where the objective is to determine the service times of jobs. Employing a cost structure composed of process costs that are decreasing and strictly convex in service times, and system-time costs that are linear in system times, we show that receding horizon controllers are state-dependent controllers, where state is defined as the system size. In order to improve upon receding horizon controllers, we search for better state-dependent control policies and present two methods to obtain them. These stochastic-approximation-type methods utilize gradient estimators based on Infinitesimal Perturbation Analysis or Imbedded Markov Chain techniques. A numerical example demonstrates the performance improvements due to the proposed methods.