Abstract
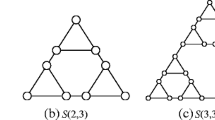
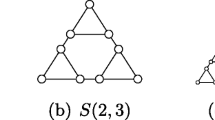
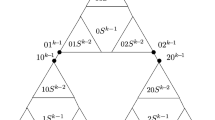
In this paper we study identifying codes, locating-dominating codes, and total-dominating codes in Sierpiński graphs. We compute the minimum size of such codes in Sierpiński graphs.
Article PDF
Similar content being viewed by others
References
Beaudou L., Gravier S., Klavžar S., Kovše M., Mollard M.: Covering codes in Sierpiński graphs. Discret. Math. Theor. Comput. Sci. 12(3), 63–74 (2010)
Ben-Haim Y., Gravier S., Lobstein A., Moncel J.: Adaptive identification in torii in the king lattice. Electron. J. Comb. 18(1), P116 (2011)
Ben-Haim Y., Litsyn S.: Exact minimum density of codes identifying vertices in the square grid. SIAM J. Discret. Math. 19(1), 69–82 (2005)
Bertrand N., Charon I., Hudry O., Lobstein A.: Identifying and locating-dominating codes on chains and cycles. Eur. J. Comb. 25, 969–987 (2004)
Charon I., Cohen G., Hudry O., Lobstein A.: New identifying codes in the binary hamming space. Eur. J. Comb. 31, 491–501 (2010)
Charon I., Honkala I., Hudry O., Lobstein A.: The minimum density of an identifying code in the king lattice. Discret. Math. 276(1–3), 95–109 (2004)
Chen C., Lua C., Miao Z.: Identifying codes and locating-dominating sets on paths and cycles. Discret. Appl. Math. 159, 1540–1547 (2011)
Cohen G., Honkala I., Litsyn S., Lobstein A.: Covering Codes. Elsevier, Amsterdam (1997)
Cull P., Nelson I.: Error-correcting codes on the Towers of Hanoi graphs. Discret. Math. 208(209), 157–175 (1999)
Exoo G., Junnila V., Laihonen T., Ranto S.: Improved bounds on identifying codes in binary Hamming spaces. Eur. J. Comb. 31, 813–827 (2010)
Foucaud F., Klasing R., Kosowski A., Raspaud A.: Bounds on the size of identifying codes in triangle-free graphs. (2010) (submitted for publication).
Gravier S., Klavžar S., Mollard M.: Codes and L(2, 1)-labelings in Sierpiński graphs. Taiwan. J. Math. 9, 671–681 (2005)
Gravier S., Kovše M., Parreau A.: Generalized Sierpiński graphs. (in preparation).
Gravier S., Moncel J., Semri A.: Identifying codes of cartesian product of two cliques of the same size. Electron. J. Comb. 15(1), N4 (2008)
Gravier S., Moncel J., Semri A.: Identifying codes of cycles. Eur. J. Comb. 27(5), 767–776 (2006)
Hinz A., Klavžar S., Milutinović U., Petr C.: The Tower of Hanoi: Myths and Maths (to appear).
Honkala I., Laihonen T.: On identifying codes in the king grid that are robust against edge deletions. Electron. J. Comb. 15(1), R3 (2008)
Jakovac M.: A 2-parametric generalization of Sierpiński gasket graphs. Ars Comb. (to appear).
Junnila V., Laihonen T.: Optimal identifying codes in cycles and paths. Graphs Comb. doi:10.1007/s00373-011-1058-6.
Klavžar S.: Coloring Sierpiński graphs and Sierpiński gasket graphs. Taiwan. J. Math. 12, 513–522 (2008)
Klavžar S., Jakovac M.: Vertex-, edge-, and total-colorings of Sierpiński-like graphs. Discret. Math. 309, 1548–1556 (2009)
Klavžar S., Milutinović U.: Graphs S(n, k) and a variant of the Tower of Hanoi problem. Czechoslov. Math. J. 47(122), 95–104 (1997)
Klavžar S., Milutinović U., Petr C.: 1-perfect codes in Sierpiński graphs. Bull. Aust. Math. Soc. 66, 369–384 (2002)
Klavžar S., Mohar B.: Crossing numbers of Sierpiński-like graphs. J. Graph Theory 50, 186–198 (2005)
Li C.-K., Nelson I.: Perfect codes on the Towers of Hanoi graphs. Bull. Aust. Math. Soc. 57, 367–376 (1998)
Lipscomb S.L., Perry J.C.: Lipscomb’s L(A) space fractalized in Hilbert’s l 2(A) space. Proc. Am. Math. Soc. 115, 1157–1165 (1992)
Lipscomb S.L.: Fractals and Universal Spaces in Dimension Theory. Springer, Berlin (2009)
Lobstein A.: Watching systems, identifying, locating-dominating and discriminating codes in graphs, a bibliography. Published electronically at http://perso.enst.fr/~lobstein/debutBIBidetlocdom.pdf. Accessed 12 March 2012.
Milutinović U.: Completeness of the Lipscomb space. Glas. Mat. Ser. III 27(47), 343–364 (1992)
Mollard M.: On perfect codes in Cartesian product of graphs. Eur. J. Comb. 32(3), 398–403 (2011)
Moncel J.: Monotonicity of the minimum cardinality of an identifying code in the hypercube. Discret. Appl. Math. 154(6), 898–899 (2006)
Roberts D.L., Roberts F.S.: Locating sensors in paths and cycles: the case of 2-identifying codes. Eur. J. Comb. 29(1), 72–82 (2008)
Teguia A.M., Godbole A.P.: Sierpiński gasket graphs and some of their properties. Aust. J. Comb. 35, 181–192 (2006)
Xu M., Thulasiraman K., Hu X.-D.: Identifying codes of cycles with odd orders. Eur. J. Comb. 29(7), 1717–1720 (2008)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by W. H. Haemers.
Rights and permissions
About this article
Cite this article
Gravier, S., Kovše, M., Mollard, M. et al. New results on variants of covering codes in Sierpiński graphs. Des. Codes Cryptogr. 69, 181–188 (2013). https://doi.org/10.1007/s10623-012-9642-1
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10623-012-9642-1