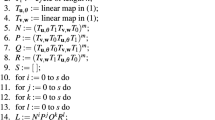Abstract
We present a new technique for constructing binary error correcting codes and give some examples of codes that can be constructed via this method. Among the examples is an infinite family of self-complementary codes with parameters (2u 2−u, 8u 2, u 2−u) that can be constructed whenever there exists a u × u Hadamard Matrix. These codes meet the Grey–Rankin bound and imply the existence of quasi-symmetric designs on 2u 2−u points.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Bracken C, McGuire G, Ward HN (2006) New quasi-symmetric designs constructed using mutually orthogonal Latin squares and Hadamard matrices. Designs, Codes and Crytography 41
Cameron PJ (1973) Near-regularity conditions for designs. Geom Ded 2:213–223
Hill R (1986) A first course in coding theory. Oxford Applied Mathematics and Computing Science Series, Oxford
McGuire G (1997) Quasi-symmetric designs and codes meeting the Grey-Rankin bound. J Combin Theory Ser A 72:280–291
MacWilliams FJ, Sloane NJA (1977) The theory of error-correcting codes. North Holland, Amsterdam
Shrikhande MS, Sane SS (1992) Quasi-symmetric designs. Cambridge University Press, Cambridge
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by D. Jungnickel.
Rights and permissions
About this article
Cite this article
Bracken, C. New classes of self-complementary codes and quasi-symmetric designs. Des Codes Crypt 41, 319–323 (2006). https://doi.org/10.1007/s10623-006-9020-y
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10623-006-9020-y