Abstract
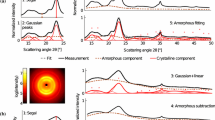
Analysis of microcrystalline cellulose (MCC) PH-101, ciprofloxacin (CIP) and quantitative phase analysis of predetermined mixtures of CIP/MCC were performed by the Rietveld method with a physically based background. Correction factors for absorption and air scattering under a symmetrical reflection geometry with a given sample thickness, divergence and receiving slit width, scale factors, average temperature factors and specimen density of packing, were considered to model the background. By this way, the whole diffraction pattern was evaluated on a physical basis, considering the Bragg and the diffuse scattering (thermal diffuse scattering plus static disorder, Compton and air scattering) using a Python code. Compton scattering was also corrected for the bandpass function of the monochromator. Quantitative phase analysis is discussed on the scope of the results obtained. The maximum absolute difference in the weight% composition obtained was of 3.4% in the CIP/MCC mixtures.


















Similar content being viewed by others
References
Advance Photon Source ANL (2013) Compute x ray absorption. http://11bm.xray.aps.anl.gov/absorb/absorb.php. Accesed Aug 2017
Ahvenainen P, Kontro I, Svedström K (2016) Comparison of sample crystallinity determination methods by X-ray diffraction for challenging cellulose I materials. Cellulose 23:1073–1086. https://doi.org/10.1007/s10570-016-0881-6
Armbruster T, Bürgi HB, Kunz M, Gnos E, Brönnimann S, Lienert C (1990) Variation of displacement parameters in structure refinements of low albite. Am Miner 75:135–140
Atalla R, Vanderhart DL (1984) Native cellulose. A composite of two distinct crystalline forms. Science 223:283–285. https://doi.org/10.1126/science.223.4633.283
Atalla R, Vanderhart DL (1987) Studies on the structure of cellulose using Raman spectroscopy and solid state 13C NMR. IPC technical paper series international symposium on wood and pulping chemistry in Paris, France. http://hdl.handle.net/1853/2525
Billinge SJL, Kanatzidis MG (2004) Beyond crystallography: the study of disorder, nanocrystallinity and crystallographically challenged materials with pair distribution functions. Chem Commun 7:749–760. https://doi.org/10.1039/b309577k
Bolhuis GK, Chowhan ZT (1996) Materials for direct compaction. In: Alderborn G, Nyström C (eds) Pharmaceutical powder compaction technology. Marcel Dekker Inc., New York, pp 419–500
Caglioti G, Paoletti A, Ricci FP (1958) Choice of collimators for a crystal spectrometer for neutron diffraction. Nucl Instrum 3:223–228
Cromer DT, Mann JB (1968) X-ray scattering factors computed from numerical Hartree-Fock wave functions. Acta Crystallogr A 24:321–324. https://doi.org/10.1107/S0567739468000550
De Figueiredo LP, Ferreira FF (2014) The rietveld method as a tool to quantify the amorphous amount of microcrystalline cellulose. J Pharm Sci 103:1394–1399
De la Torre AG, Bruque S, Aranda MAG (2001) Rietveld quantitative amorphous content analysis. J Appl Crystallogr 34:196–202. https://doi.org/10.1107/S0021889801002485
Driemeier C, Calligaris GA (2011) Theoretical and experimental developments for accurate determination of crystallinity of cellulose I materials. J Appl Crystallogr 44:184–192. https://doi.org/10.1107/S0021889810043955
Duchemin B (2017) Size, shape, orientation and crystallinity of cellulose Iβ by X-ray powder diffraction using a free spreadsheet program. Cellulose 24:2727–2741. https://doi.org/10.1007/s10570-017-1318-6
Dunitz JD, Schomaker V, Trueblood KN (1988) Interpretation of atomic displacement parameters from diffraction studies of crystals. J Phys Chem 92:856–867
Elazzouzi-Hafraoui S, Nishiyama Y, Putaux JL, Heux L, Dubreuil F, Rochas C (2008) The shape and size distribution of crystalline nanoparticles prepared by acid hydrolysis of native cellulose. Biomacromolecules 9:57–65. https://doi.org/10.1021/bm700769p
Ergun S (1951) Determination of particle density of crushed porous solids gas flow method. Anal Chem 23:151–156. https://doi.org/10.1021/ac60049a031
Ergun S (1967) X-ray studies of carbon. In: Walker PL Jr (ed) Chemistry and physics of carbon, vol III. Marcel Dekker, New York, pp 211–288
Farmacopea de los Estados Unidos Mexicanos (2016) 11th edition. ISBN: 978-607-460-454-2
Fawcett TG, Crowder CE and Kabbekodu S (2011) Reference Materials for the study of polymorphism and crystallinity of cellulose. 10th annual pharmaceutical powder X-ray diffraction symposium—XRD training for the pharmaceutical scientist. 16–19 May 2011. Lyon, France. http://www.icdd.com/ppxrd/10/presentations/PPXRD-10_Tim_Fawcett.pdf
Finger LW, Cox DE, Jephcoat AP (1994) A correction for powder diffraction peak asymmetry due to axial divergence. J Appl Crystallogr 27:892–900
Fink HP, Philipp B, Paul D, Serimaa R, Paakkari T (1987) The structure of amorphous cellulose as revealed by wide-angle X-ray scattering. Polymer 28:1265–1270. https://doi.org/10.1016/0032-3861(87)90435-6
French AD (2014) Idealized powder diffraction patterns for cellulose polymorphs. Cellulose 21(885):896
French AD (2018) Personal communication
Groom CR, Bruno IJ, Lightfoot MP, Ward SC (2016) The Cambridge structural database. Acta Crystallogr. B72:171–179. https://doi.org/10.1107/S2052520616003954
Gualtieri AF (2000) Accuracy of XRPD QPA using the combined Rietveld-RIR method. J Appl Crystallogr 33:267–278. https://doi.org/10.1107/S002188989901643X
Hermans PH, Weidinger A (1948) Quantitative X-ray investigations on the crystallinity of cellulose fibers. A background analysis. J Appl Phys 19:491–506
Herms G, Hajdu F (1984) Non-focusing diffractometer for X-ray studies on weakly absorbing amorphous materials. J Appl Cryst 19:140–146. https://doi.org/10.1107/S0021889884011201
Ioelovitch M (1992) Zur übermolekularen Struktur von nativen und isolierten Cellulosen. Acta Polym 43:110–113. https://doi.org/10.1002/actp.1992.010430212
Kern A, Madsen IC, Scarlett NVY (2012) Quantifying Amorphous Phases. In: Kolb U, Shankland K, Meshi L, Avilov A, David W (eds) Uniting electron crystallography and powder diffraction. Springer, Berlin, pp 219–231. ISBN 978-94-007-5585-7
Lee CM, Kafle K, Park YB, Kim SH (2014) Probing crystal structure and mesoscale assembly of cellulose micro fibrils in plant cell walls, tunicate tests, and bacterial films using vibrational sum frequency generation (SFG) spectroscopy. Phys Chem Chem Phys 16:10844–10853. https://doi.org/10.1039/c4cp00515e
Leppänen K, Andersson S, Torkkeli M, Knaapila M, Kotelnikova N, Serimaa R (2009) Structure of cellulose and microcrystalline cellulose from various wood species, cotton and flax studied by X-ray scattering. Cellulose 16:999–1015. https://doi.org/10.1007/s10570-009-9298-9
Mann J (1962) Modern methods of determining crystallinity in cellulose. Pure Appl Chem 5:91–106. https://doi.org/10.1351/pac196205010091
Newman RH (1999) Estimation of the lateral dimensions of cellulose crystallites using 13C NMR signal strengths. Solid State Nucl Mag 15:21–29. https://doi.org/10.1016/S0926-2040(99)00043-0
Nichols JB (1954) X-ray and infrared studies on the extent of crystallization of polymers. J Appl Phys 25:840–847. https://doi.org/10.1063/1.1721754
Nishiyama Y, Langan P, Chanzy H (2002) Crystal structure and hydrogen-bonding system in cellulose Iβ from synchrotron X-ray and neutron fiber diffraction. J Am Chem Soc 124:9074–9082. https://doi.org/10.1021/ja0257319
Nishiyama Y, Sugiyama J, Chanzy H, Langan P (2003) Crystal structure and hydrogen bonding system in cellulose Iα from synchrotron X-ray and neutron fiber diffraction. J Am Chem Soc 125:14300–14306. https://doi.org/10.1021/ja037055w
Nisizawa K (1973) Mode of action of cellulases. J Ferment Technol 51:267–304
Oliveira RP, Driemeier C (2013) CRAFS: a model to analyze two-dimensional X-ray diffraction patterns of plant cellulose. J Appl Crystallogr 46:1196–1210. https://doi.org/10.1107/S0021889813014805
Ottani S, Riello P, Polizzi S (1993) Complete sets of factors for absorption correction and air scattering subtraction in X-ray powder diffraction of loosely packed samples. Powder Differ 8:149–154. https://doi.org/10.1017/S0885715600018078
Park S, Baker JO, Himmel ME, Parilla PA, Johnson DK (2010) Cellulose crystallinity index: measurement techniques and their impact on interpreting cellulose performance. Biotechnol Biofuels 3:10. https://doi.org/10.1186/1754-6834-3-10
Riello P, Fagherazzi G, Clemente D, Canton P (1995) X-ray rietveld analysis with a physically based background. J Appl Crystallogr 28:115–120. https://doi.org/10.1107/s002188989401037x
Riello P, Canton P, Fagherazzi G (1997) Calibration of the monochromator bandpass function for the X-ray Rietveld analysis. Powder Diffr 12:160–166. https://doi.org/10.1017/s0885715600009647
Rietveld HM (1969) A profile refinement method for nuclear and magnetic structures. J Appl Crystallogr 2:65–71. https://doi.org/10.1107/S0021889869006558
Ruland W (1961) X-ray determination of crystallinity and diffuse disorder scattering. Acta Cryst 14:1180–1185. https://doi.org/10.1107/S0365110X61003429
Ruland W (1964) The separation of coherent and incoherent Compton X-ray scattering. Br J Appl Phys 15:1301–1307. https://doi.org/10.1088/0508-3443/15/11/306
Segal L, Creely JJ, Martin AE, Conrad CM (1959) An empirical method for estimating the degree of crystallinity of native cellulose using the X-ray diffractometer. Text Res J 29:786–794. https://doi.org/10.1177/004051755902901003
Smith VH, Thakkar AJ, Chapman DC (1975) A new analytic approximation to atomic incoherent X-ray scattering intensities. Acta crystallogr. A 31:391–392. https://doi.org/10.1107/s056773947500085x
Terinte N, Ibbett R, Schuster KC (2011) Overview on native cellulose and microcrystalline cellulose i structure studied by X-ray diffraction (WAXD): comparison between measurement techniques. Lenzinger Berichte 89:118–131
Thomas et al (2015) Diffraction evidence for the structure of cellulose microfibrils in bamboo, a model for grass and cereal celluloses. BMC Plant Biol 15:1. https://doi.org/10.1186/s12870-015-0538-x
Thompson P, Cox DE, Hastings JB (1987) Rietveld refinement of Debye–Scherrer synchrotron X-ray data from A12O3. J Appl Cryst 20:79–83. https://doi.org/10.1107/S0021889887087090
Thoorens G, Krier F, Leclercq B, Carlin B, Evrard B (2014) Microcrystalline cellulose, a direct compression binder in a quality by design environment-A review. Int J Pharm 473:64–72. https://doi.org/10.1016/j.ijpharm.2014.06.055
Thygesen A, Oddershede J, Lilholt H, Thomsen AB, Ståhl K (2005) On the determination of crystallinity and cellulose content in plant fibres. Cellulose 12:563–576. https://doi.org/10.1007/s10570-005-9001-8
Toby BH, Von Dreele RB (2013) GSAS-II: the genesis of a modern open-source all purpose crystallography software package. J Appl Crystallogr 46:544–549. https://doi.org/10.1107/S0021889813003531
Wallace JW (1990) Cellulose derivatives and natural products utilized in pharmaceutics. In: Swarbrick J, Boylan JC (eds) Encyclopedia of pharmaceutical technology, vol 2. Marcel Dekker, New York, pp 319–337
Ward K (1950) Crystallinity of cellulose and its significance for the fiber properties. Text Res J 20:363–372. https://doi.org/10.1177/00405175500200060
Warren BE (1990) X-ray diffraction. Dover Publications, Inc., New York
Zugenmaier P (2008) Crystalline cellulose and derivatives. Characterization and structures. Springer, Berlin
Acknowledgments
The authors acknowledge Prof. Xim Bokhimi, Antonio Morales Espino and Alejandro Herrera from LAREC laboratory at IF-UNAM for the XRD facilities as well as the financial support from the graduate program in Materials Science (PCeIM-UNAM) and DGAPA-PAPIIT project IN-110918. B. Ramírez acknowledges CONACYT for a PhD grant. The valuable collaboration of Prof. Sandra García Medina (ENCB-IPN), Octavio Graniel, Samuel Tehuacanero C., J. Eduardo L. Barriguete are gratefully appreciated. The authors thank Editor-in-Chief Alfred French and the anonymous reviewers for their helpful comments on this paper.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Ramírez, B., Bucio, L. Microcrystalline cellulose (MCC) analysis and quantitative phase analysis of ciprofloxacin/MCC mixtures by Rietveld XRD refinement with physically based background. Cellulose 25, 2795–2815 (2018). https://doi.org/10.1007/s10570-018-1761-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10570-018-1761-z