Abstract
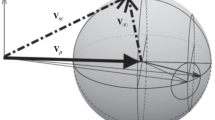
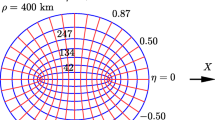
The existence of Lagrange coefficients under two-body dynamics is well known, and the concept forms the basis of robust algorithms for solving a variety of fundamental astrodynamics problems. The Lagrange coefficients are generalized to Vinti theory in the present work, where the Vinti potential describes the dynamics of small objects like spacecraft orbiting an oblate body. Exact expressions for the coefficients are provided in various forms alongside detailed derivations. Useful properties and geometrical interpretations are also established.
Similar content being viewed by others
Change history
25 June 2020
In the first paragraph of the Introduction, the last sentence should read as “ Vinti.
References
Bate, R.R., Mueller, D.D., White, J.E.: Fundamentals of Astrodynamics. Dover Publications Inc, Mineola (1971)
Battin, R.H.: An Introduction to the Mathematics and Methods of Astrodynamics. AIAA Education Series, revised edn. American Institute of Aeronautics and Astronautics Inc., Reston (1999)
Biria, A.D.: Revisiting Vinti theory: generalized equinoctial elements and applications to spacecraft relative motion. Ph.D. thesis, Supervisor: Dr. Ryan P. Russell, The Univrsity of Texas at Austin, Austin, TX (2017)
Biria, A.D., Russell, R.P.: A satellite relative motion model including \(J_2\) and \(J_3\) via Vinti’s intermediary. In: AAS/AIAA Space Flight Mechanics Meeting, Univelt, Inc., San Diego, CA, Advances in the Astronautical Sciences, vol. 158, pp. 3475–3494 (2016). (Paper AAS 16-537)
Biria, A.D., Russell, R.P.: Equinoctial elements for Vinti theory: generalizations to an oblate spheroidal geometry. Acta Astronaut. 153, 274–288 (2018a). https://doi.org/10.1016/j.actaastro.2017.11.013
Biria, A.D., Russell, R.P.: A satellite relative motion model including \(J_2\) and \(J_3\) via Vinti’s intermediary. Celest. Mech. Dyn. Astron. (2018b). https://doi.org/10.1007/s10569-017-9806-4
Biria, A.D., Russell, R.P.: Analytical solution to the Vinti problem in oblate spheroidal equinoctial orbital elements. J. Astronaut. Sci. (2019). https://doi.org/10.1007/s40295-019-00179-y
Bonavito, N.L.: Computational Procedure for Vinti’s Theory of an Accurate Intermediary Orbit. Technical report TN D-1177, National Aeronautics and Space Administration, Washington, D.C. (1962)
Der, G.J., Bonavito, N.L. (eds.): Orbital and Celestial Mechanics, Progress in Astronautics and Aeronautics, vol. 177. American Institute of Aeronautics and Astronautics, Reston (1998)
Getchell, B.C.: Orbit computation with the Vinti potential and universal variables. J. Spacecr. Rockets 7(4), 405–408 (1970). https://doi.org/10.2514/3.29954
Herrick, S.: Universal variables. Astron. J. 70(4), 309–315 (1965). https://doi.org/10.1086/109728
Pitkin, E.T.: Integration and Optimization of Sustained-thrust Rocket Orbits. Ph.D. thesis, University of California, Los Angeles (1964)
Pitkin, E.T.: A regularized approach to universal orbit variables. AIAA J. 3(8), 1508–1511 (1965). https://doi.org/10.2514/3.3176
Stumpff, K.: Neue formeln und hilfstafeln zur ephemeridenrechnung. Astron. Nachr. 275(7), 108–128 (1947). https://doi.org/10.1002/asna.19472750703
Sundman, K.F.: Mémoire sur le problème des trois corps. Acta Math. 36, 105–179 (1913). https://doi.org/10.1007/BF02422379
Vinti, J.P.: New method of solution for unretarded satellite orbits. J. Res. Natl. Bur. Stand. 63B(2), 105–116 (1959). https://doi.org/10.6028/jres.063B.012
Vinti, J.P.: Theory of an accurate intermediary orbit for satellite astronomy. J. Res. Natl. Bur. Stand. 65B(3), 169–201 (1961). https://doi.org/10.6028/jres.065B.017
Vinti, J.P.: Inclusion of the third zonal harmonic in an accurate reference orbit of an artificial satellite. J. Res. Natl. Bur. Stand. 70B(1), 17–46 (1966a). https://doi.org/10.6028/jres.070B.003
Vinti, J.P.: Invariant properties of the spheroidal potential of an oblate planet. J. Res. Natl. Bur. Stand. 70B(1), 1–16 (1966b). https://doi.org/10.6028/jres.070B.002
Vinti, J.P.: Improvement of the spheroidal method for artificial satellites. Astron. J. 74(1), 25–34 (1969). https://doi.org/10.1086/110770
Wiesel, W.E.: Numerical solution to Vinti’s problem. J. Guid. Control Dyn. 38(9), 1757–1764 (2015). https://doi.org/10.2514/1.G000661
Wright, S.P.: Orbit determination using Vinti’s solution. Ph.D. thesis, Supervisor: Dr. William E. Wiesel, Air Force Institute of Technology, Wright-Patterson Air Force Base, OH (2016)
Zurita, L.D.: Orbital resonances in the Vinti solution. In: Advanced Maui Optical and Space Surveillance Technologies (AMOS) Conference, Maui, Hawaii (2017)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
The original version of this article was revised: the detailed corrections have been given in the correction article.
Rights and permissions
About this article
Cite this article
Biria, A.D. The Lagrange coefficients of Vinti theory. Celest Mech Dyn Astr 132, 26 (2020). https://doi.org/10.1007/s10569-020-09966-4
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10569-020-09966-4