Skip to main content
SpringerLink
Log in

Adsorption of Homopolymer Chains on a Strip-Patterned Surface: A Monte Carlo Study

  • Published:
Catalysis Letters Aims and scope Submit manuscript

Abstract

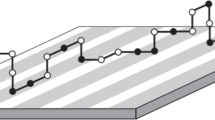
In this study we investigated the process of chemisorption of polymers on solid surfaces. The formation of a strongly adsorbed polymer film was studied by Monte Carlo simulations. The adsorbing planar surface was patterned with strip-like repulsive sites. The polymer chains were represented by a sequence of schematically constructed objects (united atoms) and we considered star-branched macromolecules with f = 3 arms of equal length. The chains were studied at good solvent conditions and thus the excluded volume was the only potential of interaction between the polymers. A Metropolis-like sampling algorithm was employed in order to calculate the properties of the adsorbed chains. The influence of the chain length, the system density and the type of the pattern on the adsorbing surface on size of chains and the structure of the polymer film were determined and discussed. We found that the roughness of the polymer film surface depends non-monotonics on the number of polymer beads in the system. The shape of this surface reflects the pattern imposed on the substrate.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Fleer GJ, Cohen Stuart MA, Scheutjens JMHM, Cosgrove T, Vincent B (1993) Polymers at interfaces. Chapman & Hall, Londo

    Google Scholar 

  2. Scheutjens JMHM, Fleer GJ (1979) J Phys Chem 83:1619

    Article  CAS  Google Scholar 

  3. Scheutjens JMHM, Fleer GJ (1980) J Phys Chem 84:178

    Article  CAS  Google Scholar 

  4. Muthukumar M (1995) J Chem Phys 103:4723

    Article  CAS  Google Scholar 

  5. Zheng H, Berg MC, Rubner MF, Hammond PT (2004) Langmuir 20:7215

    Article  CAS  Google Scholar 

  6. Chen KM, Jiang X, Kimerling LC, Hammond PT (2000) Langmuir 16:7825

    Article  CAS  Google Scholar 

  7. Sumithra K, Baumgaertner A (1998) J Chem Phys 109:1540

    Article  CAS  Google Scholar 

  8. Sumithra K, Straube E (2005) J Chem Phys 125 (art. no. 164701)

  9. Sumithra K, Straube E (2007) J Chem Phys 127 (art. no. 114908)

  10. Bhattacharya S, Hsu H-P, Milchev A, Rostiashvili VG, Vilgis TA (2008) Macromolecules 41:9220

    Article  Google Scholar 

  11. Kłos JS, Sommer JU (2008) J Chem Phys 128 (art. no. 164908)

  12. Sikorski A (2001) Macromol Theory Simul 10:38

    Article  CAS  Google Scholar 

  13. Sikorski A (2002) Macromol Theory Simul 11:359

    Article  CAS  Google Scholar 

  14. Sikorski A, Kolinski A, Skolnick J (1994) Macromol Theory Simul 3:715

    Article  CAS  Google Scholar 

  15. Romiszowski P, Sikorski A (2005) J Chem Phys 123 (art. no. 104905)

  16. Grest GS, Fetters LJ, Huang JS (1996) Adv Chem Phys 94:67

    Article  CAS  Google Scholar 

  17. Halperin A, Joanny JF (1991) J Phys II 1:623

    Article  CAS  Google Scholar 

  18. Sikorski A (1993) Makromol Chem Theory Simul 2:309

    Article  CAS  Google Scholar 

  19. O’Shaughnessy B, Vavylonis D (2003) Phys Rev Lett 90 (art. no. 056103)

  20. Erban R, Chapman SJ (2007) J Stat Phys 127:1255

    Article  CAS  Google Scholar 

Download references

Acknowledgments

The computational part of this work was done using the computer cluster at the Computing Center of the Department of Chemistry, University of Warsaw.

Author information

Authors and Affiliations

  1. Department of Chemistry, University of Warsaw, 02-093, Warszawa, Poland

    Piotr Adamczyk, Piotr Romiszowski & Andrzej Sikorski

Authors
  1. Piotr Adamczyk
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Piotr Romiszowski
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Andrzej Sikorski
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Andrzej Sikorski.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Adamczyk, P., Romiszowski, P. & Sikorski, A. Adsorption of Homopolymer Chains on a Strip-Patterned Surface: A Monte Carlo Study. Catal Lett 129, 130–134 (2009). https://doi.org/10.1007/s10562-008-9795-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10562-008-9795-8

Keywords

Search

Navigation