Skip to main content
SpringerLink
Log in

The Analyses of Turbulence Characteristics in the Atmospheric Surface Layer Using Arbitrary-Order Hilbert Spectra

  • Research Article
  • Published:
Boundary-Layer Meteorology Aims and scope Submit manuscript

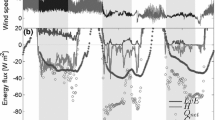
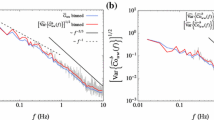
Abstract

Turbulent characteristics in the atmospheric surface layer are investigated using a data-driven method, Hilbert spectral analysis. The results from empirical mode decomposition display a set of intrinsic mode functions whose characteristic scales suggest a dyadic filter-bank property. It can be concluded from the joint probability density function of the intrinsic mode functions that the turbulent properties are totally different under different stratifications: the amplitudes (or energies) are arranged according to the stability parameter for stable conditions, but tend to cluster randomly for unstable cases. The intermittency analyses reveal that second-order Hilbert marginal spectra display a power-law behaviour in the inertial subrange, and that the scaling exponent functions deviate from the theoretical values due to the strong intermittency in the stable boundary layer.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  • Chen J, Zhang R, Wang H, Li J, Hong M, Li X (2014) Decadal modes of sea surface salinity and the water cycle in the tropical Pacific Ocean: the anomalous late 1990s. Deep Sea Res Part I Oceanogr Res Pap 84:38–49

    Article  Google Scholar 

  • Cohen L (1995) Time-frequency analysis. Prentice Hall, New Jersey, pp 153–161

    Google Scholar 

  • Dyer AJ (1974) A review of flux-profile relationships. Boundary-Layer Meteorol 7:363–372

    Article  Google Scholar 

  • Ferreres E, Soler MR, Terradellas E (2013) Analysis of turbulent exchange and coherent structures in the stable atmospheric boundary layer based on tower observations. Dyn Atmos Ocean 64:62–78

    Article  Google Scholar 

  • Flandrin P (1998) Time-frequency/time-scale analysis. Academic Press, San Diego, pp 12–18

    Google Scholar 

  • Flandrin P, Gonçalvés P (2004) Empirical mode decompositions as data-driven wavelet-like expansions. Int J Wavelets Multiresolut Inf Process 02:477–496

    Article  Google Scholar 

  • Flandrin P, Rilling G, Gonçalvés P (2004) Empirical mode decomposition as a filter bank. Signal Process Lett IEEE 11:112–114

    Article  Google Scholar 

  • Frisch U (1995) Turbulence: the legacy of AN Kolmogorov. Cambridge University Press, Cambridge, pp 72–97

    Google Scholar 

  • Garai A, Kleissl J (2011) Air and surface temperature coupling in the convective atmospheric boundary layer. J Atmos Sci 68:2945–2954

    Article  Google Scholar 

  • Haugen DA (1973) Workshop on micrometeorology. American Meteorological Society, Boston, pp 152–163

    Google Scholar 

  • Högström ULF (1988) Non-dimensional wind and temperature profiles in the atmospheric surface layer: a re-evaluation. Boundary-Layer Meteorol 42:55–78

    Article  Google Scholar 

  • Holtslag AAM, Nieuwstadt FTM (1986) Scaling the atmospheric boundary layer. Boundary-Layer Meteorol 36:201–209

    Article  Google Scholar 

  • Horiguchi M, Hayashi T, Adachi A, Onogi S (2014) Stability dependence and diurnal change of large-scale turbulence structures in the near-neutral atmospheric boundary layer observed from a meteorological tower. Boundary-Layer Meteorol 151:221–237

    Article  Google Scholar 

  • Hu W, Biswas A, Si BC (2014) Application of multivariate empirical mode decomposition for revealing scale-and season-specific time stability of soil water storage. Catena 113:377–385

    Article  Google Scholar 

  • Huang YX (2009) Arbitrary order Hilbert spectral analysis definition and application to fully developed turbulence and environmental time series. Université des Sciences et Technologie de Lille-Lille I, pp 31–32

  • Huang NE, Shen SSP (2005) Hilbert–Huang transform and its applications. World Scientific, Singapore, 32 pp

  • Huang NE, Shen Z, Long SR, Wu MC, Shin HH, Zheng Q, Yen N-C, Tung CC, Liu HH (1998) The empirical mode decomposition and the Hilbert spectrum for nonlinear and non-stationary time series analysis. Proc R Soc A Math Phys Eng Sci 454:903–995

    Article  Google Scholar 

  • Huang NE, Shen Z, Long SR (1999) A new view of nonlinear water waves: the Hilbert Spectrum 1. Annu Rev Fluid Mech 31:417–457

    Article  Google Scholar 

  • Huang NE, Wu M-LC, Long SR, Shen SS, Qu W, Gloersen P, Fan KL (2003) A confidence limit for the empirical mode decomposition and Hilbert spectral analysis. Proc R Soc A Math Phys Eng Sci 459:2317–2345

    Article  Google Scholar 

  • Huang YX, Schmitt FG, Lu ZM, Liu YL (2008) An amplitude-frequency study of turbulent scaling intermittency using Empirical Mode Decomposition and Hilbert Spectral Analysis. Europhys Lett (EPL) 84:40010

    Article  Google Scholar 

  • Huang YX, Schmitt FG, Lu ZM, Liu YL (2009) Analysis of daily river flow fluctuations using empirical mode decomposition and arbitrary order Hilbert spectral analysis. J Hydrol 373:103–111

    Article  Google Scholar 

  • Huang YX, Schmitt FG, Lu ZM, Fougairolles P, Gagne Y, Liu YL (2010) Second-order structure function in fully developed turbulence. Phys Rev E 82:026319

    Article  Google Scholar 

  • Huang YX, Schmitt FG, Hermand J-P, Gagne Y, Lu ZM, Liu YL (2011) Arbitrary-order Hilbert spectral analysis for time series possessing scaling statistics: comparison study with detrended fluctuation analysis and wavelet leaders. Phys Rev E 84:016208

    Article  Google Scholar 

  • Huang YX, Biferale L, Calzavarini E, Sun C, Toschi F (2013) Lagrangian single-particle turbulent statistics through the Hilbert–Huang transform. Phys Rev E 87:041003

    Article  Google Scholar 

  • Imberger J, Boashash B (1986) Application of the Wigner–Ville distribution to temperature gradient microstructure: a new technique to study small-scale variations. J Phys Oceanogr 16:1997–2012

    Article  Google Scholar 

  • Iyengar RN, Kanth STGR (2006) Seasonal forecasting of Indian summer monsoon rainfall: a review. Weather 91:350–356

    Google Scholar 

  • Kaimal JC, Wyngaard Y, Izumi Y, Coté OR (1972) Spectral characteristics of surface layer turbulence over the sea. Q J R Meteorol Soc 98:563–589

    Article  Google Scholar 

  • Kaimal JC, Wyngaard Y, Haugen DA, Coté OR, Izumi Y, Caughey SJ, Readings CJ (1976) Turbulence structure in convective boundary layer. J Atmos Sci 33:2152–2169

    Article  Google Scholar 

  • Kolmogorov AN (1941) Dissipation of energy in locally isotropic turbulence. Dokl Akad Nauk SSSR 32:16–18

    Google Scholar 

  • Kolmogorov AN (1962) A refinement of previous hypotheses concerning the local structure. J Fluid Mech 13:82–85

    Article  Google Scholar 

  • Klipp CL, Mahrt L (2004) Flux-gradient relationship, self-correlation and intermittency in the stable boundary layer. Q J R Meteorol Soc 130:2087–2103

    Article  Google Scholar 

  • Koracin D, Berkowicz R (1988) Nocturnal boundary-layer height: observations by acoustic sounders and predictions in terms of surface-layer parameters. Boundary-Layer Meteorol 43:65–83

    Article  Google Scholar 

  • Li X, Zhang H (2012) Seasonal variations in dust concentration and dust emission observed over Horqin Sandy Land area in China from December 2010 to November 2011. Atmos Environ 61:56–65

    Article  Google Scholar 

  • Mahrt L (1998) Nocturnal boundary-layer regimes. Boundary-Layer Meteorol 88:255–278

    Article  Google Scholar 

  • Mahrt L (2014) Stably stratified atmospheric boundary layers. Annu Rev Fluid Mech 46:23–45

    Article  Google Scholar 

  • Moeng C-H (1984) A large-eddy-simulation model for the study of planetary boundary-layer turbulence. J Atmos Sci 41:2052–2062

    Article  Google Scholar 

  • Molla MKI, Rahman MS, Sumi A, Banik P (2006) Empirical mode decomposition analysis of climate changes with special reference to rainfall data. Discret Dyn Nat Soc 2006:1–17

    Article  Google Scholar 

  • Panofsky HA, Dutton JA (1984) Atmospheric turbulence. Models and methods for engineering applications. Wiley, New York, pp 145–148

    Google Scholar 

  • Panofsky HA, Tennekes H, Lenschow DH, Wyngaard JC (1977) The characteristics of turbulent velocity components in the surface layer under convective conditions. Boundary-Layer Meteorol 11:355–361

    Article  Google Scholar 

  • Panofsky HA, Larko D, Lipschutz R, Stone G, Bradley EF, Bowen AJ, Højstrup J (1982) Spectra of velocity components over complex terrain. Q J R Meteorol Soc 108:215–230

    Article  Google Scholar 

  • Ramana MV, Krishnan P, Kunhikrishnan PK (2004) Surface boundary-layer characteristics over a tropical inland station: seasonal features. Boundary-Layer Meteorol 111:153–157

    Article  Google Scholar 

  • Rilling G, Flandrin P, Gonçalvès P (2003) On empirical mode decomposition and its algorithms. IEEE-EURASIP Work Nonlinear Signal Image Process NSIP-03 Grado 3:8–11

    Google Scholar 

  • Salmond JA (2005) Wavelet analysis of intermittent turbulence in a very stable nocturnal boundary layer: implications for the vertical mixing of ozone. Boundary-Layer Meteorol 114:463–488

    Article  Google Scholar 

  • Schmitt FG, Huang Y, Lu Z, Liu YL, Fernandez N (2009) Analysis of velocity fluctuations and their intermittency properties in the surf zone using empirical mode decomposition. J Mar Syst 77:473–481

    Article  Google Scholar 

  • Sun J, Mahrt L, Banta RM, Pichugina YL (2012) Turbulence regimes and turbulence intermittency in the stable boundary layer during CASES-99. J Atmos Sci 69:338–351

    Article  Google Scholar 

  • Vincent CL, Pinson P, Giebela G (2011) Wind fluctuations over the North Sea. Int J Climatol 31:1584–1595

    Google Scholar 

  • Weber S, Kordowski K (2009) Comparison of atmospheric turbulence characteristics and turbulent fluxes from two urban sites in Essen, Germany. Theor Appl Climatol 102:61–74

    Article  Google Scholar 

  • Wei W, Wu BG, Ye XX, Wang HX, Zhang HS (2013) Characteristics and mechanisms of low-level jets in the Yangtze River Delta of China. Boundary-Layer Meteorol 149:403–424

    Article  Google Scholar 

  • Wei W, Zhang HS, Ye XX (2014) Comparison of low-level jets along the north coast of China in summer. J Geophys Res Atmos 119:9692–9706

    Article  Google Scholar 

  • Wieringa J (1993) Representative roughness parameters for homogeneous terrain. Boundary-Layer Meteorol 63:323–363

    Article  Google Scholar 

  • Wu Z, Huang NE (2004) A study of the characteristics of white noise. Proc R Soc Lond A Math Phys Eng Sci 460:1597–1611

    Article  Google Scholar 

  • Zhang HS, Chen J, Park SU (2001) Turbulence structure in unstable conditions over various surface. Boundary-Layer Meteorol 100:243–261

    Article  Google Scholar 

  • Zhang HS, Zhu H, Peng Y, Kang L, Chen JY, Park SU (2007) Experiment on dust flux during duststorm periods over sand desert area. Acta Meteorol Sin 65:744–752

    Google Scholar 

Download references

Acknowledgments

This work was jointly funded by R&D Special Fund for Public Welfare Industry (meteorology) by Ministry of Finance and Ministry of Science and Technology (GYHY201506001), the Public Welfare Projects for Environmental Protection (201409001, 201309009), the National Natural Science Foundation of China (41475007, 91544216) and The Research Fund for the Doctoral Program of Higher Education (20110001130010). This work was performed during a visit of the first author in LOG (Wimereux, France). The China Scholarship Council (CSC) is thanked for financial support for this study.

Author information

Authors and Affiliations

  1. Laboratory for Climate and Ocean-Atmosphere Studies, Department of Atmospheric and Oceanic Sciences, School of Physics, Peking University, Beijing, People’s Republic of China

    W. Wei & H. S. Zhang

  2. Laboratoire d’Oceanologie et de Geosciences, CNRS, Univ. Lille, Univ. Littoral Cote d’Opale, UMR 8187, 62930, Wimereux, France

    F. G. Schmitt

  3. State Key Laboratory of Marine Environmental Science, Xiamen University, Xiamen, People’s Republic of China

    Y. X. Huang

Authors
  1. W. Wei
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. F. G. Schmitt
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Y. X. Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. H. S. Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to H. S. Zhang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wei, W., Schmitt, F.G., Huang, Y.X. et al. The Analyses of Turbulence Characteristics in the Atmospheric Surface Layer Using Arbitrary-Order Hilbert Spectra. Boundary-Layer Meteorol 159, 391–406 (2016). https://doi.org/10.1007/s10546-015-0122-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10546-015-0122-9

Keywords

Search

Navigation