Abstract
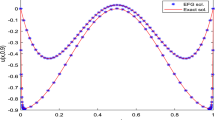
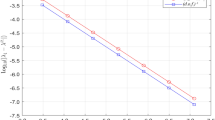
The localized exponential time differencing method based on overlapping domain decomposition has been recently introduced and successfully applied to parallel computations for extreme-scale numerical simulations of coarsening dynamics based on phase field models. In this paper, we focus on numerical solutions of a class of semilinear parabolic equations with the well-known Allen–Cahn equation as a special case. We first study the semi-discrete system under the standard central difference spatial discretization and prove the equivalence between the monodomain problem and the corresponding multidomain problem obtained by the Schwarz waveform relaxation iteration. Then we develop the fully discrete localized exponential time differencing schemes and, by establishing the maximum bound principle, prove the convergence of the fully discrete localized solutions to the exact semi-discrete solution and the convergence of the iterative solutions. Numerical experiments are carried out to verify the theoretical results in one-dimensional space and test the convergence and accuracy of the proposed algorithms with different numbers of subdomains in two-dimensional space.






Similar content being viewed by others
References
Al-Mohy, A.H., Higham, N.J.: Computing the action of the matrix exponential, with an application to exponential integrators. SIAM J. Sci. Comput. 33, 488–511 (2011)
Allen, S.M., Cahn, J.W.: A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsening. Acta Metall. 27, 1085–1095 (1979)
Cahn, J.W., Hilliard, J.E.: Free energy of a nonuniform system. I. Interfacial free energy. J. Chem. Phys. 28, 258–267 (1958)
Cox, S.M., Matthews, P.C.: Exponential time differencing for stiff systems. J. Comput. Phys. 176, 430–455 (2002)
Du, Q., Gunzburger, M.D., Peterson, J.S.: Analysis and approximation of the Ginzburg–Landau model of superconductivity. SIAM Rev. 34, 54–81 (1992)
Du, Q., Ju, L., Li, X., Qiao, Z.: Maximum principle preserving exponential time differencing schemes for the nonlocal Allen–Cahn equation. SIAM J. Numer. Anal. 57, 875–898 (2019)
Du, Q., Ju, L., Li, X., Qiao, Z.: Maximum bound principles for a class of semilinear parabolic equations and exponential time differencing schemes. SIAM Rev. (2020, to appear)
Elder, K.R., Katakowski, M., Haataja, M., Grant, M.: Modeling elasticity in crystal growth. Phys. Rev. Lett. 88, 245–701 (2002)
Evans, L.C., Soner, H.M., Souganidis, P.E.: Phase transitions and generalized motion by mean curvature. Commun. Pure Appl. Math. 45, 1097–1123 (1992)
Gander, M.J.: A waveform relaxation algorithm with overlapping splitting for reaction diffusion equations. Numer. Linear Algebra Appl. 6, 125–145 (1999)
Gander, M.J., Stuart, A.M.: Space-time continuous analysis of waveform relaxation for the heat equation. SIAM J. Sci. Comput. 19, 2014–2031 (1998)
Gottlieb, S., Shu, C.W., Tadmor, E.: Strong stability-preserving high-order time discretization methods. SIAM Rev. 43, 89–112 (2001)
Gustafsson, B., Kreiss, H.O., Oliger, J.: Time-Dependent Problems and Difference Methods, 2nd edn. Wiley, Hoboken (2013)
Hoang, T.T.P., Ju, L., Wang, Z.: Overlapping localized exponential time differencing methods for diffusion problems. Commum. Math. Sci. 16, 1531–1555 (2018)
Hoang, T.T.P., Ju, L., Wang, Z.: Nonoverlapping localized exponential time differencing methods for diffusion problems. J. Sci. Comput. 82, 37 (2020)
Hochbruck, M., Lubich, C.: On Krylov subspace approximations to the matrix exponential operator. SIAM J. Numer. Anal. 34, 1911–1925 (1997)
Hochbruck, M., Ostermann, A.: Explicit exponential Runge—Kutta methods for semilinear parabolic problems. SIAM J. Numer. Anal. 43, 1069–1090 (2005)
Hochbruck, M., Ostermann, A.: Exponential integrators. Acta Numer. 19, 209–286 (2010)
Horn, R.A., Johnson, C.R.: Topics in Matrix Analysis. Cambridge University Press, Cambridge (1994)
Hu, G.Y., O’Connell, R.F.: Analytical inversion of symmetric tridiagonal matrices. J. Phys. A 29, 1511–1513 (1996)
Isherwood, L., Grant, Z.J., Gottlieb, S.: Strong stability preserving integrating factor Runge-Kutta methods. SIAM J. Numer. Anal. 56, 3276–3307 (2018)
Ju, L., Li, X., Qiao, Z., Zhang, H.: Energy stability and error estimates of exponential time differencing schemes for the epitaxial growth model without slope selection. Math. Comput. 87, 1859–1885 (2018)
Ju, L., Zhang, J., Du, Q.: Fast and accurate algorithms for simulating coarsening dynamics of Cahn–Hilliard equations. Comput. Mater. Sci. 108, 272–282 (2015)
Ju, L., Zhang, J., Zhu, L., Du, Q.: Fast explicit integration factor methods for semilinear parabolic equations. J. Sci. Comput. 62, 431–455 (2015)
Kleefeld, B., Martín-Vaquero, J.: SERK2v2: a new second-order stabilized explicit Runge–Kutta method for stiff problems. Numer. Methods Part. Differ. Equ. 29, 170–185 (2013)
Lawson, J.D.: Generalized Runge–Kutta processes for stable systems with large Lipschitz constants. SIAM J. Numer. Anal. 4, 372–380 (1967)
Lazer, A.C.: Characteristic exp nents and diagonally dominant linear differential systems. J. Math. Anal. Appl. 35, 215–229 (1971)
Li, B., Liu, J.G.: Thin film epitaxy with or without slope selection. Eur. J. Appl. Math. 14, 713–743 (2003)
Medovikov, A.A.: High order explicit methods for parabolic equations. BIT 38, 372–390 (1998)
Shu, C.W., Osher, S.: Efficient implementation of essentially nonoscillatory shock-capturing schemes. J. Comput. Phys. 77, 439–471 (1988)
Temam, R.: Navier–Stokes E quations: Theory and Numerical Analysis. North-Holland Publishing Co., New York (1977)
Zhang, J., Zhou, Z., Wang, Y., Ju, L., Du, Q., Chi, X., Xu, D., Chen, D., Liu, Y., Liu, Z.: Extreme-scale phase field simulations of coarsening dynamics on the Sunway Taihulight supercomputer. In: Proceedings of the International Conference for High Performance Computing, Networking, Storage and Analysis (SC’16). IEEE Press, Piscataway (2016)
Zhu, L., Ju, L., Zhao, W.: Fast high-order compact exponential time differencing Runge–Kutta methods for second-order semilinear parabolic equations. J. Sci. Comput. 67, 1043–1065 (2016)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Christian Lubich.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
X. Li’s work is partially supported by National Natural Science Foundation of China grant 11801024. L. Ju’s work is partially supported by US National Science Foundation grant DMS-1818438 and US Department of Energy grants DE-SC0016540 and DE-SC0020270.
Rights and permissions
About this article
Cite this article
Li, X., Ju, L. & Hoang, TTP. Overlapping domain decomposition based exponential time differencing methods for semilinear parabolic equations. Bit Numer Math 61, 1–36 (2021). https://doi.org/10.1007/s10543-020-00817-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10543-020-00817-0
Keywords
- Semilinear parabolic equation
- Overlapping domain decomposition
- Localized exponential time differencing
- Parallel Schwarz iteration
- Waveform relaxation
- Convergence analysis