Skip to main content
SpringerLink
Log in

An efficient fourth-order in space difference scheme for the nonlinear fractional Ginzburg–Landau equation

  • Published:
BIT Numerical Mathematics Aims and scope Submit manuscript

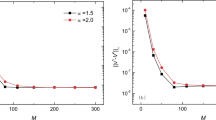
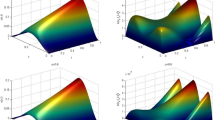
Abstract

This paper proposes and analyzes a high-order implicit-explicit difference scheme for the nonlinear complex fractional Ginzburg–Landau equation involving the Riesz fractional derivative. For the time discretization, the second-order backward differentiation formula combined with the explicit second-order Gear’s extrapolation is adopted. While for the space discretization, a fourth-order fractional quasi-compact method is used to approximate the Riesz fractional derivative. The scheme is efficient in the sense that, at each time step, only a linear system with a coefficient matrix independent of the time level needs to be solved. Despite of the explicit treatment of the nonlinear term, the scheme is shown to be unconditionally convergent in the \(l^2_h\) norm, semi-\(H^{\alpha /2}_h\) norm and \(l^\infty _h\) norm at the order of \(O(\tau ^2+h^4)\) with \(\tau \) time step and h mesh size. Numerical tests are provided to confirm the accuracy and efficiency of the scheme.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. Aranson, I.S., Kramer, L.: The world of the complex Ginzburg–Landau equation. Rev. Mod. Phys. 74(1), 99–143 (2002)

    Article  MathSciNet  Google Scholar 

  2. Ascher, U.M., Ruuth, S.J., Wetton, B.T.R.: Implicit-explicit methods for time-dependent partial differential equations. SIAM J. Numer. Anal. 32, 797–823 (1995)

    Article  MathSciNet  Google Scholar 

  3. Bueno-Orovio, A., Kay, D., Burrage, K.: Fourier spectral methods for fractional-in-space reaction–diffusion equations. BIT 54, 937–954 (2014)

    Article  MathSciNet  Google Scholar 

  4. Çelik, C., Duman, M.: Crank-Nicolson method for the fractional diffusion equation with the Riesz fractional derivative. J. Comput. Phys. 231(4), 1743–1750 (2012)

    Article  MathSciNet  Google Scholar 

  5. Chen, M., Deng, W.: Fourth order accurate scheme for the space fractional diffusion equations. SIAM J. Numer. Anal. 52, 1418–1438 (2014)

    Article  MathSciNet  Google Scholar 

  6. Defterli, O., D’Elia, M., Du, Q., Gunzburger, M., Lehoucq, R., Meerschaert, M.M.: Fractional diffusion on bounded domains. Fract. Calc. Appl. Anal. 18, 342–360 (2015)

    Article  MathSciNet  Google Scholar 

  7. Ding, H., Li, C., Chen, Y.: High-order algorithms for Riesz derivative and their applications (II). J. Comput. Phys. 293, 218–237 (2015)

    Article  MathSciNet  Google Scholar 

  8. Ding, H., Li, C., Chen, Y.: High-order algorithms for Riesz derivative and their applications (III). Fract. Calc. Appl. Anal. 19, 19–55 (2016)

    Article  MathSciNet  Google Scholar 

  9. Du, Q., Gunzburger, M., Lehoucq, R.B., Zhou, K.: Analysis and approximation of nonlocal diffusion problems with volume constraints. SIAM Rev. 54(4), 667–696 (2012)

    Article  MathSciNet  Google Scholar 

  10. Ervin, V.J., Heuer, N., Roop, J.P.: Numerical approximation of a time dependent, nonlinear, space-fractional diffusion equation. SIAM J. Numer. Anal. 45, 572–591 (2007)

    Article  MathSciNet  Google Scholar 

  11. Guo, B., Huo, Z.: Well-posedness for the nonlinear fractional Schrödinger equation and inviscid limit behavior of solution for the fractional Ginzburg-Landau equation. Fract. Calc. Appl. Anal. 16, 226–242 (2013)

    MathSciNet  MATH  Google Scholar 

  12. Hairer, E., Wanner, G.: Solving Ordinary Differential Equations II: Stiff and Differential-Algebraic Problems. Springer Series in Computational Mathematics, vol. 14, 2nd edn. Springer, Berlin (1996)

    Book  Google Scholar 

  13. Hao, Z.P., Sun, Z.Z.: A linearized high-order difference scheme for the fractional Ginzburg–Landau equation. Numer. Methods Partial Differ. Equ. 33, 105–124 (2017)

    Article  MathSciNet  Google Scholar 

  14. Hao, Z.P., Sun, Z.Z., Cao, W.R.: A fourth-order approximation of fractional derivatives with its applications. J. Comput. Phys. 281, 787–805 (2015)

    Article  MathSciNet  Google Scholar 

  15. Hao, Z.P., Sun, Z.Z., Cao, W.R.: A three-level linearized compact difference scheme for the Ginzburg–Landau equation. Numer. Methods Partial Differ. Equ. 31, 876–899 (2015)

    Article  MathSciNet  Google Scholar 

  16. Kirkpatrick, K., Lenzmann, E., Staffilani, G.: On the continuum limit for discrete NLS with long-range lattice interactions. Commun. Math. Phys. 317, 563–591 (2013)

    Article  MathSciNet  Google Scholar 

  17. Lord, G.J.: Attractors and inertial manifolds for finite-difference approximations of the complex Ginzburg–Landau equation. SIAM J. Numer. Anal. 34(4), 1483–1512 (1997)

    Article  MathSciNet  Google Scholar 

  18. Lu, H., Bates, P.W., Lü, S., Zhang, M.: Dynamics of the 3-D fractional complex Ginzburg–Landau equation. J. Differ. Equ. 259(10), 5276–5301 (2015)

    Article  MathSciNet  Google Scholar 

  19. Meerschaert, M.M., Tadjeran, C.: Finite difference approximations for fractional advection–dispersion flow equations. J. Comput. Appl. Math. 172, 65–77 (2004)

    Article  MathSciNet  Google Scholar 

  20. Milovanov, A., Rasmussen, J.: Fractional generalization of the Ginzburg–Landau equation: an unconventional approach to critical phenomena in complex media. Phys. Lett. A 337, 75–80 (2005)

    Article  Google Scholar 

  21. Mvogo, A., Ben-Bolie, G.H., Kofané, T.C.: Coupled fractional nonlinear differential equations and exact Jacobian elliptic solutions for excitoncphonon dynamics. Phys. Lett. A 378, 2509–2517 (2014)

    Article  MathSciNet  Google Scholar 

  22. Mvogo, A., Tambue, A., Ben-Bolie, G.H., Kofané, T.C.: Localized numerical impulse solutions in diffuse neural networks modeled by the complex fractional Ginzburg–Landau equation. Commun. Nonlinear Sci. Numer. Simul. 39, 396–410 (2016)

    Article  MathSciNet  Google Scholar 

  23. Podlubny, I.: Fractional Differential Equations. Academic Press, San Diego (1999)

    MATH  Google Scholar 

  24. Pu, X., Guo, B.: Well-posedness and dynamics for the fractional Ginzburg–Landau equation. Appl. Anal. 92(2), 318–334 (2013)

    Article  MathSciNet  Google Scholar 

  25. Quarteroni, A., Valli, A.: Numerical Approximation of Partial Differential Equations. Springer Series in Computational Mathematics, vol. 23. Springer, Berlin (1994)

    MATH  Google Scholar 

  26. Sun, Z.Z., Zhu, Q.: On Tsertsvadze’s difference scheme for the Kuramoto–Tsuzuki equation. J. Comput. Appl. Math. 98(2), 289–304 (1998)

    Article  MathSciNet  Google Scholar 

  27. Tadjeran, C., Meerschaert, M.M., Scheffler, H.P.: A second-order accurate numerical approximation for the fractional diffusion equation. J. Comput. Phys. 213, 205–213 (2006)

    Article  MathSciNet  Google Scholar 

  28. Tarasov, V., Zaslavsky, G.: Fractional Ginzburg–Landau equation for fractal media. Phys. A 354, 249–261 (2005)

    Article  Google Scholar 

  29. Tarasov, V., Zaslavsky, G.: Fractional dynamics of coupled oscillators with long-range interaction. Chaos 16(2), 023110 (2006)

    Article  MathSciNet  Google Scholar 

  30. Tian, W., Zhou, H., Deng, W.: A class of second order difference approximation for solving space fractional diffusion equations. Math. Comput. 84, 1703–1727 (2015)

    Article  MathSciNet  Google Scholar 

  31. Wang, D., Xiao, A., Yang, W.: Crank-Nicolson difference scheme for the coupled nonlinear Schrödinger equations with the Riesz space fractional derivative. J. Comput. Phys. 242, 670–681 (2013)

    Article  MathSciNet  Google Scholar 

  32. Wang, D., Xiao, A., Yang, W.: A linearly implicit conservative difference scheme for the space fractional coupled nonlinear Schrödinger equations. J. Comput. Phys. 272, 644–655 (2014)

    Article  MathSciNet  Google Scholar 

  33. Wang, D., Xiao, A., Yang, W.: Maximum-norm error analysis of a difference scheme for the space fractional CNLS. Appl. Math. Comput. 257, 241–251 (2015)

    MathSciNet  MATH  Google Scholar 

  34. Wang, H., Basu, T.S.: A fast finite difference method for two-dimensional space-fractional diffusion equations. SIAM J. Sci. Comput. 34, A2444–A2458 (2012)

    Article  MathSciNet  Google Scholar 

  35. Wang, P., Huang, C.: A conservative linearized difference scheme for the nonlinear fractional Schrödinger equation. Numer. Algorithm 69, 625–641 (2015)

    Article  Google Scholar 

  36. Wang, P., Huang, C.: An energy conservative difference scheme for the nonlinear fractional Schrödinger equations. J. Comput. Phys. 293, 238–251 (2015)

    Article  MathSciNet  Google Scholar 

  37. Wang, P., Huang, C.: An implicit midpoint difference scheme for the fractional Ginzburg–Landau equation. J. Comput. Phys. 312, 31–49 (2016)

    Article  MathSciNet  Google Scholar 

  38. Wang, P., Huang, C., Zhao, L.: Point-wise error estimate of a conservative difference scheme for the fractional Schrödinger equation. J. Comput. Appl. Math. 306, 231–247 (2016)

    Article  MathSciNet  Google Scholar 

  39. Wang, T., Guo, B.: Analysis of some finite difference schemes for two-dimensional Ginzburg–Landau equation. Numer. Methods Partial Differ. Equ. 27, 1340–1363 (2011)

    Article  MathSciNet  Google Scholar 

  40. Xu, Q., Hesthaven, J.S.: Discontinuous Galerkin method for fractional convection–diffusion equations. SIAM J. Numer. Anal. 52, 405–423 (2014)

    Article  MathSciNet  Google Scholar 

  41. Yang, Q., Liu, F., Turner, I.: Numerical methods for fractional partial differential equations with Riesz space fractional derivatives. Appl. Math. Model. 34(1), 200–218 (2010)

    Article  MathSciNet  Google Scholar 

  42. Zeng, F., Liu, F., Li, C., Burrage, K., Turner, I., Anh, V.: A Crank-Nicolson ADI spectral method for a two-dimensional Riesz space fractional nonlinear reaction-diffusion equation. SIAM J. Numer. Anal. 52, 2599–2622 (2014)

    Article  MathSciNet  Google Scholar 

  43. Zhao, X., Sun, Z.Z., Hao, Z.P.: A fourth-order compact ADI scheme for two-dimensional nonlinear space fractional Schrödinger equation. SIAM J. Sci. Comput. 36(6), A2865–A2886 (2014)

    Article  Google Scholar 

  44. Zhou, H., Tian, W., Deng, W.: Quasi-compact finite difference schemes for space fractional diffusion equations. J. Sci. Comput. 56, 45–66 (2013)

    Article  MathSciNet  Google Scholar 

  45. Zhuang, P., Liu, F., Anh, V., Turner, I.: Numerical methods for the variable-order fractional advection–diffusion equation with a nonlinear source term. SIAM J. Numer. Anal. 47(3), 1760–1781 (2009)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work was supported by National Natural Science Foundation of China (No. 11771163).

Author information

Authors and Affiliations

  1. College of Mathematics and Information Science, Henan University of Economics and Law, Zhengzhou, 450000, China

    Pengde Wang

  2. School of Mathematics and Statistics, Huazhong University of Science and Technology, Wuhan, 430074, China

    Chengming Huang

  3. Hubei Key Laboratory of Engineering Modeling and Scientific Computing, Huazhong University of Science and Technology, Wuhan, 430074, China

    Chengming Huang

Authors
  1. Pengde Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Chengming Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Chengming Huang.

Additional information

Communicated by Jan Hesthaven.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, P., Huang, C. An efficient fourth-order in space difference scheme for the nonlinear fractional Ginzburg–Landau equation. Bit Numer Math 58, 783–805 (2018). https://doi.org/10.1007/s10543-018-0698-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10543-018-0698-9

Keywords

Mathematics Subject Classification

Search

Navigation