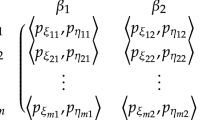Skip to main content
SpringerLink
Log in

Simple noncooperative games with intuitionistic fuzzy information and application in ecological management

  • Published:
Applied Intelligence Aims and scope Submit manuscript

Abstract

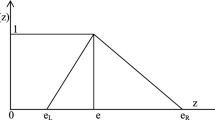
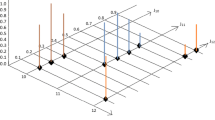
The matrix game and bi-matrix game are typical non-cooperative games. Nowadays, interactive game decisions on social management are mainly concerned, especially when players are faced with uncertain payoffs. The aim of this paper is to develop simple and effective parameterized linear programming methods for solving two types of matrix games with payoffs expressed by intuitionistic fuzzy (IF) information. In these methods, the expected values of players are regarded as monotonic functions with their risk preferences. Hereby we construct two models of auxiliary bilinear programming, and the corresponding optimal strategies can be easily obtained by explicit computation. The models and methods proposed in this paper are applied in two ecological management problems, and the validity and applicability are verified.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Paulson EC, Linkov I, Keisler JM (2016) A game theoretic model for resource allocation among countermeasures with multiple attributes. Eur J Oper Res 252(2):610–622

    Article  MathSciNet  Google Scholar 

  2. Yue Q, Zhang L, Yu B, Zhang LJ, Zhang J (2019) Two-sided matching for triangular intuitionistic fuzzy numbers in smart environmental protection. IEEE Access 7:42426–42435

    Article  Google Scholar 

  3. Nazari S, Ahmadi A, Kamrani Rad S, Ebrahimi B (2020) Application of non-cooperative dynamic game theory for groundwater conflict resolution. J Environ Manag 270:110889

    Article  Google Scholar 

  4. Xue L, Sun C, Yu F (2017) A game theoretical approach for distributed resource allocation with uncertainty. Int J Intell Comput Cyber 10(1):52–67

    Article  Google Scholar 

  5. Liu J, Zhao W (2016) Cost-sharing of ecological construction based on trapezoidal intuitionistic fuzzy cooperative games. Int J Environ Res Public Health 13(11):1102

    Article  Google Scholar 

  6. Yang J, Kilgour DM (2019) Bi-fuzzy graph cooperative game model and application to profit allocation of ecological exploitation. Int J Fuzzy Syst 21(6):1858–1867

    Article  MathSciNet  Google Scholar 

  7. Zadeh LA (1965) Fuzzy sets. Inf Control 8(3):338–353

    Article  Google Scholar 

  8. Atanassov KT (1986) Intuitionistic fuzzy sets. Fuzzy Sets Syst 20(1):87–96

    Article  Google Scholar 

  9. Bustince H, Barrenechea E, Pagola M, Fernandez J, Xu Z, Bedregal B, Montero J, Hagras H, Herrera F, de Baets B (2016) A historical account of types of fuzzy sets and their relationships. IEEE Trans Fuzzy Syst 24(1):179–194

    Article  Google Scholar 

  10. Li DF, Nan JX (2009) A nonlinear programming approach to matrix games with payoffs of Atanassov’s intuitionistic fuzzy sets. Int J Uncertain Fuzziness Knowl Based Syst 17(4):585–607

    Article  MathSciNet  Google Scholar 

  11. Li DF, Liu JC (2015) A parameterized nonlinear programming approach to solve matrix games with payoffs of I-Fuzzy numbers. IEEE T Fuzzy Syst 23(4):885–896

    Article  Google Scholar 

  12. Yang J, Li DF, Lai LB (2016) parameterized bilinear programming methodology for solving triangular intuitionistic fuzzy number bimatrix games. J Intell Fuzzy Syst 31(1):115–125

    Article  Google Scholar 

  13. Xu Z (2007) Intuitionistic preference relations and their application in group decision making. Inf Sci 177(11):2363–2379

    Article  MathSciNet  Google Scholar 

  14. Meng F, Tang J, Fujita H (2019) Linguistic intuitionistic fuzzy preference relations and their application to multi-criteria decision making. Inform Fusion 46:77–90

    Article  Google Scholar 

  15. Zhai Y, Xu Z, Liao H (2018) Measures of probabilistic interval-valued intuitionistic hesitant fuzzy sets and the application in reducing excessive medical examinations. IEEE Trans Fuzzy Syst 26(3):1651–1670

    Article  Google Scholar 

  16. Fujita H, Gaeta A, Loia V, Orciuoli F (2020) Hypotheses analysis and assessment in counter-terrorism activities: a method based on OWA and fuzzy probabilistic rough sets. IEEE Trans Fuzzy Syst 28(5):831–845

    Article  Google Scholar 

  17. Xu Z (2005) Extended C-OWA operators and their use in uncertain multi-attribute decision making. Syst Engine Theo Pract 25(11):7–13

    Google Scholar 

  18. Xu Z, Cai XQ (2008) Intuitionistic fuzzy information: aggregation theory and applications, science press, Beijing. Springer, New York

    Google Scholar 

  19. Hao Z, Xu Z, Zhao H, Fujita H (2018) A dynamic weight determination approach based on the intuitionistic fuzzy Bayesian network and its application to emergency decision making. IEEE Trans Fuzzy Syst 26(4):1893–1907

    Article  Google Scholar 

  20. Son L, Ngan RT, Ali M, Fujita H, Priyan MK (2019) A new representation of intuitionistic fuzzy systems and their applications in critical decision making. IEEE Intell Syst 35(1):6–17

    Article  Google Scholar 

  21. Xu Z, Chen J (2008) An overview of distance and similarity measures of intuitionistic fuzzy sets. Int J Uncertain Fuzz 16(4):529–555

    Article  MathSciNet  Google Scholar 

  22. Aggarwal A, Mehra A, Chandra S (2012) Application of linear programming with I-fuzzy sets to matrix games with I-fuzzy goals. Fuzzy Optim Decis Making 11:465–480

    Article  MathSciNet  Google Scholar 

  23. Yang J, Fei W, Li DF (2016) Non-linear programming approach to solve bi-matrix games with payoffs represented by I-fuzzy numbers. Int J Fuzzy Syst 18(3):492–503

    Article  MathSciNet  Google Scholar 

  24. Li DF (2010) Mathematical-programming approach to matrix games with payoffs represented by Atanassov’s interval-valued intuitionistic fuzzy sets. IEEE Trans Fuzzy Syst 18(6):1112–1128

    Article  Google Scholar 

  25. Nan JX, Zhang L, Li DF (2019) The method for solving bi-matrix games with intuitionistic fuzzy set payoffs. In: Li DF (ed) Game theory, Communications in Computer and Information Science. Springer, Singapore

    Google Scholar 

  26. Xu Z, Yager RR (2006) Some geometric aggregation operators based on intuitionistic fuzzy sets. Int J Gen Syst 35:417–433

    Article  MathSciNet  Google Scholar 

  27. Chen SM, Tan JM (1994) Handling multicriteria fuzzy decision-making problems based on vague set theory. Fuzzy Sets Syst 67:163–172

    Article  MathSciNet  Google Scholar 

  28. Yager RR (2004) OWA aggregation over a continuous interval argument with applications to decision making. IEEE Trans Syst Man Cybern Part B 34(5):1952–1963

    Article  Google Scholar 

  29. Owen G (1982) Game theory, 2nd edn. Academic Press, New York

    MATH  Google Scholar 

  30. Mangasarian OL, Stone H (1964) Two-person nonzero-sum games and quadratic programming. J Math Anal Appl 9:348–355

    Article  MathSciNet  Google Scholar 

  31. Nash JF (1950) Equilibrium points in n-person games. Proc Natl Acad Sci U S A 36:48–49

    Article  MathSciNet  Google Scholar 

  32. Vijay V, Chandra S, Bector CR (2005) matrix games with fuzzy goals and fuzzy payoffs. Omega 33:425–429

    Article  Google Scholar 

Download references

Acknowledgments

This work presented in this paper is supported by the Science Foundation of Ministry of Education of China (No.19YJC630201), the China Postdoctoral Science Foundation (No.227348), and the Program for Distinguished Young Scholars in University of Fujian Province (No. K80SCC55A). We appreciate the comments and suggestions will give by the reviewers and editor of this journal.

Author information

Authors and Affiliations

  1. College of Management, Fujian Agriculture and Forestry University, Fuzhou, 350002, China

    Jie Yang & Yongwu Dai

  2. Business School, Sichuan University, Chengdu, 610064, China

    Jie Yang & Zeshui Xu

Authors
  1. Jie Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zeshui Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yongwu Dai
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jie Yang.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, J., Xu, Z. & Dai, Y. Simple noncooperative games with intuitionistic fuzzy information and application in ecological management. Appl Intell 51, 6685–6697 (2021). https://doi.org/10.1007/s10489-021-02215-7

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10489-021-02215-7

Keywords

Search

Navigation