Skip to main content
SpringerLink
Log in

Exact solutions of multi-term fractional diffusion-wave equations with Robin type boundary conditions

  • Published:
Applied Mathematics and Mechanics Aims and scope Submit manuscript

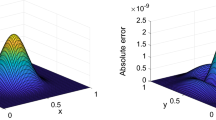
Abstract

General exact solutions in terms of wavelet expansion are obtained for multiterm time-fractional diffusion-wave equations with Robin type boundary conditions. By proposing a new method of integral transform for solving boundary value problems, such fractional partial differential equations are converted into time-fractional ordinary differential equations, which are further reduced to algebraic equations by using the Laplace transform. Then, with a wavelet-based exact formula of Laplace inversion, the resulting exact solutions in the Laplace transform domain are reversed to the time-space domain. Three examples of wave-diffusion problems are given to validate the proposed analytical method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Huang, F. H. and Guo, B. L. General solutions to a class of time fractional partial differential equations. Applied Mathematics and Mechanics (English Edition), 31, 815–826 (2010) DOI 10.1007/s10483-010-1316-9

    Article  MATH  MathSciNet  Google Scholar 

  2. Mainardi, F. Fractional relaxation-oscillation and fractional diffusion-wave phenomena. Chaos, Solitons & Fractals, 7, 1461–1477 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  3. Caputo, M. Linear models of dissipation whose Q is almost frequency independent-II. Geophysical Journal of the Royal Astronomical Society, 13, 529–539 (1967)

    Article  Google Scholar 

  4. Caputo, M. and Mainardi, F. Linear models of dissipation in anelastic solids. La Rivista del Nuovo Cimento, 1, 161–198 (1971)

    Article  Google Scholar 

  5. Nigmatullin, R. R. The realization of the generalized transfer equation in a medium with fractal geometry. Physica Status Solidi (B), 133, 425–430 (1986)

    Article  Google Scholar 

  6. Nigmatullin, R. R. To the theoretical explanation of the universal response. Physica B, 123, 739–745 (1984)

    Google Scholar 

  7. Agrawal, O. P. Solution for a fractional diffusion-wave equation defined in a bounded domain. Nonlinear Dynamics, 29, 145–155 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  8. Chen, W. Time-space fabric underlying anomalous diffusion. Chaos, Solitons & Fractals, 28, 923–929 (2006)

    Article  MATH  Google Scholar 

  9. Chen, W., Sun, H., Zhang, X., and Koroak, D. Anomalous diffusion modeling by fractal and fractional derivatives. Computers and Mathematics with Applications, 59, 1754–1758 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  10. Chen, W. An intuitive study of fractional derivative modeling and fractional quantum in soft matter. Journal of Vibration and Control, 14, 1651–1657 (2008)

    Article  MATH  Google Scholar 

  11. Chen, W. A speculative study of 2/3-order fractional Laplacian modeling of turbulence: some thoughts and conjectures. Chaos, 16, 023126 (2006)

    Article  Google Scholar 

  12. Chen, W. and Holm, S. Modified Szaboo wave equation models for lossy media obeying frequency power law. Journal of the Acoustical Society of America, 114, 2570–2574 (2003)

    Article  Google Scholar 

  13. Chen, W. and Holm, S. Fractional Laplacian time-space models for linear and nonlinear lossy media exhibiting arbitrary frequency dependency. Journal of the Acoustical Society of America, 115, 1424–1430 (2004)

    Article  Google Scholar 

  14. Li, C., Zhang, F., Kurths, J., and Zeng, F. Equivalent system for a multiple-rational-order fractional differential system. Philosophical Transactions of the Royal Society A, 371, 20120156 (2013)

    Article  MathSciNet  Google Scholar 

  15. Schneider, W. R. and Wyss, W. Fractional diffusion and wave equations. Journal of Mathematical Physics, 30, 134–144 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  16. Mainardi, F. The fundamental solutions for the fractional diffusion-wave equation. Applied Mathematics Letters, 9, 23–28 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  17. Daftardar-Gejji, V. and Bhalekar, S. Solving multi-term linear and non-linear diffusion-wave equations of fractional order by Adomian decomposition method. Applied Mathematics and Computation, 202, 113–120 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  18. Jafari, H. and Seifi, S. Homotopy analysis method for solving linear and nonlinear fractional diffusion-wave equation. Communications in Nonlinear Science and Numerical Simulation, 14, 2009–2012 (2009)

    Google Scholar 

  19. Daftardar-Gejji, V. and Bhalekar, S. Boundary value problems for multi-term fractional differential equations. Journal of Mathematical Analysis and Applications, 345, 754–765 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  20. Welch, S. W. J., Ropper, R. A. L., and Duren, R. G. Application of time-based fractional calculus methods to viscoelastic creep and stress relaxation of materials. Mechanics of Time-Dependent Materials, 3, 279–303 (1999)

    Article  Google Scholar 

  21. Ford, N. J., Xiao, J., and Yan, Y. A finite element method for time fractional partial differential equations. Fractional Calculus and Applied Analysis, 14, 454–474 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  22. Esen, A., Ucar, Y., Yagmurlu, N., and Tasbozan, O. A Galerkin finite element method to solve fractional diffusion and fractional diffusion-wave equations. Mathematical Modelling and Analysis, 18, 260–273 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  23. Li, C. and Zeng, F. The finite difference methods for fractional ordinary differential equations. Numerical Functional Analysis and Optimization, 34, 149–179 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  24. Li, C. and Zeng, F. Finite difference methods for fractional differential equations. International Journal of Bifurcation and Chaos, 22, 1230014 (2012)

    Article  MathSciNet  Google Scholar 

  25. Zhou, Y. H., Wang, X. M., Wang, J. Z., and Liu, X. J. A wavelet numerical method for solving nonlinear fractional vibration, diffusion and wave equations. Computer Modeling in Engineering and Sciences, 77, 137–160 (2011)

    MathSciNet  Google Scholar 

  26. Li, Y. Solving a nonlinear fractional differential equation using Chebyshev wavelets. Communications in Nonlinear Science and Numerical Simulation, 15, 2284–2292 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  27. Wang, J. Z., Zhou, Y. H., and Gao, H. J. Computation of the Laplace inverse transform by application of the wavelet theory. Communications in Numerical Methods in Engineering, 19, 959–975 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  28. Koziol, P. and Hryniewicz, Z. Analysis of bending waves in beam on viscoelastic random foundation using wavelet technique. International Journal of Solids and Structures, 43, 6965–6977 (2006)

    Article  MATH  Google Scholar 

  29. Koziol, P., Mares, C., and Esat, I. Wavelet approach to vibratory analysis of surface due to a load moving in the layer. International Journal of Solids and Structures, 45, 2140–2159 (2008)

    Article  MATH  Google Scholar 

  30. Koziol, P., Hryniewicz1, Z., and Mares, C. Wavelet analysis of beam-soil structure response for fast moving train. Journal of Physics: Conference Series, 181, 012052 (2009)

    Google Scholar 

  31. Hong, D. P., Kim, Y. M., and Wang, J. Z. A new approach for the analysis solution of dynamic systems containing fractional derivative. Journal of Mechanical Science and Technology, 20, 658–667 (2006)

    Article  Google Scholar 

  32. Wang, J. Z. Fractional stochastic description of hinge motions in single protein molecules. Chinese Science Bulletin, 56, 495–501 (2011)

    Article  Google Scholar 

  33. Wei, D. Coiflet-Type Wavelets: Theory, Design, and Applications, Ph. D. dissertation, The University of Texas, Austin (1998)

    Google Scholar 

  34. Donoho, D. L. Interpolating Wavelet Transforms, Report, Stanford University, Stanford (1992)

    Google Scholar 

  35. Xu, C. F., Cai, C., Pi, M. H., Zhu, C. X., and Li, G. K. Interpolating wavelet and its applications. Conference of International Symposium on Multispectral Image Process, 3545, 428–432 (1998)

    Article  Google Scholar 

  36. Daubechies, I. Orthonormal bases of compactly supported wavelets. Communications on Pure and Applied Mathematics, 41, 909–996 (1988)

    Article  MATH  MathSciNet  Google Scholar 

  37. Comincioli, V., Naldi, G., and Scapolla, T. A wavelet-based method for numerical solution of nonlinear evolution equations. Applied Numerical Mathematics, 33, 291–297 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  38. Metzler, R. and Klafter, J. The random walk’s guide to anomalous diffusion: a fractional dynamics approach. Physics Reports, 339, 1–77 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  39. Tarasov, V. E. Review of some promising fractional physical models. International Journal of Modern Physics B, 27, 1330005 (2013)

    Article  MathSciNet  Google Scholar 

  40. Metzler, R. and Klafter, J. Boundary value problems for fractional diffusion equations. Physica A, 278, 107–125 (2000)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Key Laboratory of Mechanics on Disaster and Environment in Western China, Ministry of Education, School of Civil Engineering and Mechanics, Lanzhou University, Lanzhou, 730000, P. R. China

    Xiao-jing Liu  (刘小靖), Ji-zeng Wang  (王记增), Xiao-min Wang  (王晓敏) & You-he Zhou  (周又和)

Authors
  1. Xiao-jing Liu  (刘小靖)
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ji-zeng Wang  (王记增)
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xiao-min Wang  (王晓敏)
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. You-he Zhou  (周又和)
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ji-zeng Wang  (王记增).

Additional information

Project supported by the National Natural Science Foundation of China (Nos. 11032006, 11072094, and 11121202), the Ph.D. Program Foundation of Ministry of Education of China (No. 20100211110022), the National Key Project of Magneto-Constrained Fusion Energy Development Program (No. 2013GB110002), the Fundamental Research Funds for the Central Universities (Nos. lzujbky-2012-202 and lzujbky-2013-1), and the Scholarship Award for Excellent Doctoral Student Granted by Lanzhou University

Rights and permissions

Reprints and permissions

About this article

Cite this article

Liu, Xj., Wang, Jz., Wang, Xm. et al. Exact solutions of multi-term fractional diffusion-wave equations with Robin type boundary conditions. Appl. Math. Mech.-Engl. Ed. 35, 49–62 (2014). https://doi.org/10.1007/s10483-014-1771-6

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10483-014-1771-6

Key words

Chinese Library Classification

2010 Mathematics Subject Classification

Search

Navigation