Abstract
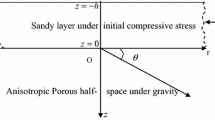
The paper studies the propagation of Love waves in a non-homogeneous substratum over an initially stressed heterogeneous half-space. The dispersion equation of phase velocity is derived. The velocities of Love waves are calculated numerically as a function of kH and presented in a number of graphs, where k is the wave number, and H is the thickness of the layer. The case of Gibson’s half-space is also considered. It is observed that the speed of Love waves is finite in the vicinity of the surface of the half-space and vanishes as the depth increases for a particular wave number. It is also observed that an increase in compressive initial stresses causes decreases of Love waves velocity for the same frequency, and the tensile initial stress of small magnitude in the half-space causes increase of the velocity.
Similar content being viewed by others
References
Love, A. E. H. A Treatise on Mathematical Theory of Elasticity, 4th ed., Dover Publication, New York (1944)
Biot, M. A. The influence of initial stress on elastic wave. J. Appl. Phys., 11(8), 522–530 (1940)
Dey, S. and Addy, S. K. Love waves under initial stresses in a visco-elastic medium overlying an elastic half-space. Gerlands Beiträge zur Geophysik, 87(4), 305–311 (1978)
Dey, S. and Addy, S. K. Love waves under initial stresses. Acta Geophysica Polonica, XXVI(1), 47 (1978)
Dey, S., Roy, N., and Dutta, A. Propagation of Love waves in an initially stressed anisotropic porous layer lying over a pre-stressed non-homogeneous elastic half-space. Acta Geophysica Polonica, XXXVII(1) 21–36 (1989)
Chttopadhyay, A. and Kar, B. K. On the dispersion curves of Love type waves in an initially stressed crustal layer having an irregular interface. Geophysical Research Bulletin, 16(1), 13–23 (1978)
Chakraborty, S. K. and Dey, S. Love waves in dissipative media under gravity. Gerlands Beiträge zur Geophysik, 90(6), 521–528 (1981)
Chttopadhyay, A. and De, R. K. Propagation of Love type waves in a visco-elastic initially stressed layer overlying a visco-elastic half-space with irregular interface. Rev. Roum. Sci. Tech. Mec. Appl. Tome, 26(3), 449–460 (1981)
Abd-Alla, A. M. and Ahmed, S. M. Propagation of Love waves in a non-homogeneous orthotropic elastic layer under initial stress overlying semi-infinite medium. Applied Mathematics and Computation, 106(2), 265–275 (1999)
Destrade, M. Surface waves in orthotropic incompressible materials. J. Acoust. Soc. Am., 110(2), 837–840 (2001)
Khurana, P. Love wave propagation in a pre-stressed medium. Indian Journal of Pure and Applied Mathematics, 32(8), 1201–1207 (2001)
Sharma, M. D. and Garg, N. Wave velocities in a pre-stressed anisotropic elastic medium. Journal of Earth System Science, 115(2), 257–265 (2007)
Gupta, S., Vishwakarma, S. K., Majhi, D. K., and Kundu, S. Influence of rigid boundary and initial stress on the propagation of Love wave. Applied Mathematics, 2, 586–594 (2011)
Biot, M. A. Mechanics of Incremental Deformation, John Wiley and Sons, New York (1965)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Gupta, S., Majhi, D.K., Kundu, S. et al. Propagation of Love waves in non-homogeneous substratum over initially stressed heterogeneous half-space. Appl. Math. Mech.-Engl. Ed. 34, 249–258 (2013). https://doi.org/10.1007/s10483-013-1667-7
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10483-013-1667-7
Key words
- Love waves
- initial stress
- heterogeneous half-space
- Gibson’s half-space
- dispersion equation
- phase velocity