Skip to main content

Advertisement

SpringerLink
Log in

Projected subgradient method for non-Lipschitz set-valued mixed variational inequalities

  • Published:
Applied Mathematics and Mechanics Aims and scope Submit manuscript

Abstract

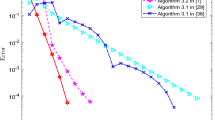
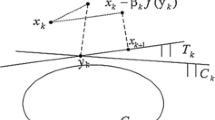
A projected subgradient method for solving a class of set-valued mixed variational inequalities (SMVIs) is proposed when the mapping is not necessarily Lipschitz. Under some suitable conditions, it can be proven that the sequence generated by the method can strongly converge to the unique solution to the problem in the Hilbert spaces.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Han, W. and Reddy, B. On the finite element method for mixed variational inequalities arising in elastoplasticity. SIAM J. Numer. Anal., 32(6), 1778–1807 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  2. Cohen, G. Nash equilibria: gradient and decomposition algorithms. Large Scale Systems, 12(2), 173–184 (1987)

    MathSciNet  MATH  Google Scholar 

  3. Facchinei, F. and Pang, J. S. Finite-Dimensional Variational Inequalities and Complementary Problems, Springer-Verlag, New York (2003)

    Google Scholar 

  4. Iusem, A. N. and Svaiter, B. F. A variant of Korpelevich’s method for solving variational inequalities with a new search strategy. Optimization, 42(4), 309–321 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  5. Xia, F. Q., Huang, N. J., and Liu, Z. B. A projected subgradient method for solving generalized mixed variational inequalities. Oper. Res. Lett., 36(5), 637–642 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  6. He, Y. R. A new projection algorithm for mixed variational inequalities (in Chinese). Acta Math. Sci., 27A(2), 215–220 (2007)

    Google Scholar 

  7. Konnov, I. A combined relaxation method for a class of nonlinear variational inequalities. Optimization, 51(1), 127–143 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  8. Maingé, P. E. Projected subgradient techniques and viscosity methods for optimization with variational inequality constraints. European J. Oper. Res., 205(3), 501–506 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  9. Anh, P. N., Muu, L.D., and Strodiot, J. J. Generalized projection method for non-Lipschitz multivalued monotone variational inequalities. Acta Math. Vietnam., 34(1), 67–79 (2009)

    MathSciNet  MATH  Google Scholar 

  10. Farouq, N. E. Pseudomonotone variational inequalities: convergence of the auxiliary problem method. J. Optim. Theory Appl., 111(2), 306–325 (2001)

    Google Scholar 

  11. Hue, T. T., Strodiot, J. J., and Nguyen, V. H. Convergence of the approximate auxiliary problem method for solving generalized variational inequalities. J. Optim. Theory Appl., 121(1), 119–145 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  12. Konnov, I. Combined Relaxation Methods for Variational Inequalities, Springer-Verlag, Berlin (2001)

    Book  MATH  Google Scholar 

  13. Marcotte, P. A new algorithm for solving variational inequalities with application to the traffic assignment problem. Math. Program., 33(3), 339–351 (1985)

    Article  MathSciNet  Google Scholar 

  14. Patriksson, M. Merit functions and descent algorithms for a class of variational inequality problems. Optimization, 41(1), 37–55 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  15. Patriksson, M. Nonlinear Programming and Variational Inequality Problems, Kluwer Academic Publishers, Dordrecht (1999)

    MATH  Google Scholar 

  16. Salmon, G., Strodiot, J. J., and Nguyen, V. H. A bundle method for solving variational inequalities. SIAM J. Optim., 14(3), 869–893 (2003)

    Article  MathSciNet  Google Scholar 

  17. Solodov, M. V. and Svaiter, B. F. A new projection method for variational inequalities. SIAM J. Control Optim., 37(3), 765–776 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  18. Taji, K. and Fukushima, M. A new merit function and a successive quadratic programming algorithm for variational inequality problems. SIAM J. Optim., 6(3), 704–713 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  19. Ding, X. P. Existence and algorithm of solutions for nonlinear mixed variational-like inequalities in Banach spaces. J. Comput. Appl. Math., 157(2), 419–434 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  20. Ding, X. P. Predictor-corrector iterative algorithms for solving generalized mixed variational-like inequalities. Appl. Math. Comput., 152(3), 855–865 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  21. Ding, X. P., Lin, Y. C., and Yao, J. C. Three-step relaxed hybrid steepest-descent methods for variational inequalities. Appl. Math. Mech. -Engl. Ed., 28(8), 1029–1036 (2007) DOI 10.1007/s10483-007-0805-x

    Article  MathSciNet  MATH  Google Scholar 

  22. Zhang, S. S., Wang, X. R., Lee, H. W. J., and Chan, C. K. Viscosity method for hierarchical fixed point and variational inequalities with applications. Appl. Math. Mech. -Engl. Ed., 32(2), 241–250 (2011) DOI 10.1007/s10483-011-1410-8

    Article  MathSciNet  MATH  Google Scholar 

  23. Chang, S. S., Lee, H. W. J., Chan C. K., and Liu, J. A. A new method for solving a system of generalized nonlinear variational inequalities in Banach spaces. Appl. Math. Comput., 217(15–16), 6830–6837 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  24. Verma, R. U. General convergence analysis for two-step projection methods and applications to variational problems. Appl. Math. Lett., 18(11), 1286–1292 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  25. Lan, H. Y., Cho, Y. J., and Verma, R. U. Nonlinear relaxed cocoercive variational inclusions involving (A, η)-accretive mappings in Banach spaces. Comput. Math. Appl., 51(9–10), 1529–1538 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  26. Alber, Y. I., Iusem, A. N., and Solodov, M. V. On the projected subgradient method for nonsmooth convex optimization in a Hilbert space. Math. Program., 81(1), 23–35 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  27. Yin, H. Y., Xu, C. X., and Zhang, Z. X. The F-complementarity problem and its equivalence with the least element problem (in Chinese). Acta Math. Sinica, 44(4), 679–686 (2001)

    MathSciNet  MATH  Google Scholar 

  28. Zhong, R. Y. and Huang, N. J. Stability analysis for Minty mixed variational inequality in reflexive Banach spaces. J. Optim. Theory Appl., 147(3), 454–472 (2010)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Sichuan University, Chengdu, 610064, P. R. China

    Guo-ji Tang  (唐国吉) & Nan-jing Huang  (黄南京)

Authors
  1. Guo-ji Tang  (唐国吉)
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Nan-jing Huang  (黄南京)
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Nan-jing Huang  (黄南京).

Additional information

Communicated by Shi-sheng ZHANG

Project supported by the Key Program of National Natural Science Foundation of China (No. 70831005), the National Natural Science Foundation of China (No. 10671135), and the Fundamental Research Funds for the Central Universities (No. 2009SCU11096)

Rights and permissions

Reprints and permissions

About this article

Cite this article

Tang, Gj., Huang, Nj. Projected subgradient method for non-Lipschitz set-valued mixed variational inequalities. Appl. Math. Mech.-Engl. Ed. 32, 1345–1356 (2011). https://doi.org/10.1007/s10483-011-1505-x

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10483-011-1505-x

Key words

Chinese Library Classification

2010 Mathematics Subject Classification

Search

Navigation