Classical Schwarz reflection principle for Jenkins–Serrin type minimal surfaces

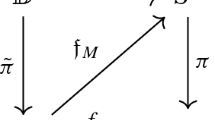
Abstract

We give a proof of the classical Schwarz reflection principle for Jenkins–Serrin type minimal surfaces in the homogeneous three manifolds $\mathbb E(\kappa ,\tau )$ for $\kappa \leqslant 0$ and $\tau \geqslant 0$. In our previous paper, we proved a reflection principle in Riemannian manifolds. The statements and techniques in the two papers are distinct.

1 Introduction

In this paper, we focus the classical Schwarz reflection principle across a geodesic line in the boundary of a minimal surface in $\mathbb R^3$ and more generally in three-dimensional homogeneous spaces $\mathbb E(\kappa ,\tau )$ for $\kappa <0$ and $\tau \geqslant 0$.

The Schwarz reflection principle was shown in some special cases. One kind of examples arise for the solutions of the classical Plateau problem in $\mathbb R^3$ containing a segment of a straight line in the boundary, see Lawson [8, Chapter II, Section 4, Proposition 10]. Another kind occurs for vertical graphs in $\mathbb R^3$ and ${\mathbb {H}}^2\times \mathbb R$ containing an arc of a horizontal geodesic, see [20, Lemma 3.6].

On the other hand, there is no proof of the reflection principle for general minimal surfaces in $\mathbb R^3$ containing a straight line in its boundary.

The goal of this paper is to provide a proof of the reflection principle about vertical geodesic lines for Jenkins–Serrin type minimal surfaces in $\mathbb R^3$ and other three-dimensional homogeneous manifolds such as ${\mathbb {H}}^2 \times \mathbb R$, $\widetilde{\mathrm{PSL}}_2(\mathbb R, \tau )$ and ${\mathbb {S}}^2\times \mathbb R$, see Theorem 4.1. The proof also holds for horizontal geodesic lines.

We observe that this classical Schwarz reflection principle was used by many authors, including the present authors, in $\mathbb R^3$ and ${\mathbb {H}}^2 \times \mathbb R$.

We recall that the authors proved another reflection principle for minimal surfaces in general three-dimensional Riemannian manifold with quite different statement and techniques, see [21].

We are grateful to the referee of our paper whose remarks greatly improved this work.

2 A brief description of the three-dimensional homogeneous manifolds $\mathbb E(\kappa ,\tau )$

For any $r>0$, we denote by $\mathbb D(r)\subset \mathbb R^2$ the open disc of $\mathbb R^2$ with center at the origin and with radius r (for the Euclidean metric).

For any $\kappa \leqslant 0$ and $\tau \geqslant 0$, we consider the model of ${\mathbb {E}}(\kappa ,\tau )$ given by ${\mathbb {D}}(\dfrac{2}{\sqrt{-\kappa }}) \times \mathbb R$ equipped with the metric

$$\begin{aligned} \nu _\kappa ^2(\mathrm{d}x^2 + \mathrm{d}y^2) + \left( \tau \nu _\kappa (y\mathrm{d}x - x\mathrm{d}y) + \mathrm{d}t \right) ^2. \end{aligned}$$

(1)

where $\nu _\kappa = \dfrac{1}{1 +\kappa \frac{x^2+y^2}{4}}$. We observe that ${\mathbb {E}}(-1,\tau )= \widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )$. By abuse of notations, we set $\mathbb D(\frac{1}{0})= \mathbb D(+\infty )= \mathbb R^2$. Thus, ${\mathbb {E}}(0,\tau ) = \mathrm{Nil}_3(\tau )$. Also, $\mathbb R^3$ equipped with the Euclidean metric is a model of ${\mathbb {E}}(0,0)$.

We denote by $\mathbb M(\kappa )$ the complete, connected and simply connected Riemannian surface with constant curvature $\kappa$. Notice that for $\kappa <0$ a model of $\mathbb M(\kappa )$ is given by the disc ${\mathbb {D}}(\dfrac{2}{\sqrt{-\kappa }})$ equipped with the metric $\nu _\kappa ^2(\mathrm{d}x^2 + \mathrm{d}y^2)$.

We recall that ${\mathbb {E}}(\kappa ,\tau )$ is a fibration over $\mathbb M(\kappa )$, and the projection $\Pi : {\mathbb {E}}(\kappa ,\tau ) \longrightarrow \mathbb M(\kappa )$ is a Riemannian submersion, see for example [2]. Moreover, the unit vertical field $\frac{\partial }{\partial t}$ is a Killing field generating a one-parameter group of isometries given by the vertical translations.

We have seen in [21, Example 2.2-(2)] that the horizontal geodesics and the vertical geodesics of $\mathbb E(\kappa ,\tau )$ admit a reflection. That is, for any such a geodesic L, there exists a non- trivial isometry $I_L$ of $\mathbb E(\kappa ,\tau )$ satisfying

• $I_L$ is orientation preserving,

• $I_L (p) =p$ for any $p\in L$,

• $I_L \circ I_L= \mathrm{Id}$.

Let $\Omega$ be any domain of $\mathbb M(\kappa )$ and let $u:\Omega \longrightarrow \mathbb R$ be a $C^2$-function. We say that the set $\Sigma := \{(p, u(p)),\ p\in \Omega \} \subset {\mathbb {D}}(\dfrac{2}{\sqrt{-\kappa }}) \times \mathbb R$ is a vertical graph. Note that the Killing field $\frac{\partial }{\partial t}$ is transverse to $\Sigma$. Thus, by the well-known criterium of stability, if $\Sigma$ is a minimal surface, then $\Sigma$ is stable.

Consider some arbitrary local coordinates $(x_1,x_2,x_3)$ of $\mathbb E(\kappa ,\tau )$. Let u be a $C^2$ function defined on a domain $\Omega$ contained in the $x_1,x_2$ plane of coordinates. Let $S\subset \mathbb E(\kappa ,\tau )$ be the graph of u. Then, S is a minimal surface if u satisfies an elliptic PDE (called minimal surface equation)

$$\begin{aligned} F(x,u,u_1,u_2,u_{11}, u_{12}, u_{22})=0, \end{aligned}$$

see [21, Equation (13)]. Furthermore, if u has bounded gradient, then the PDE is uniformly elliptic.

3 Jenkins–Serrin type minimal surfaces

The original Jenkins–Serrin’s theorem was conceived in $\mathbb R^3$, see [7, Theorems 1, 2 and 3]. It was extended in ${\mathbb {H}}^2 \times \mathbb R$ by Nelli and Rosenberg [11, Theorem 3] and in $\mathbb M^2\times \mathbb R$ by Pinheiro [15, Theorem 1.1] where $\mathbb M^2$ is a complete Riemannian surface. Later on, it was established in $\widetilde{\mathrm{PSL}}_2(\mathbb R)$ by Younes [25, Theorem 1.1] and in $\text {Sol}_3$ by Nguyen [13, Section 3.6]. As a matter of fact, the same proof also works in the homogeneous spaces $\mathbb E(\kappa ,\tau )$ for any $\kappa < 0$ and $\tau \geqslant 0$.

We state briefly below the Jenkins–Serrin type theorem in the homogeneous spaces $\mathbb E(\kappa ,\tau )$ for $\kappa <0$ and $\tau \geqslant 0$ (same statement holds in $\mathbb R^3$ and in $\mathbb M^2\times \mathbb R$).

Let $\Omega$ be a bounded convex domain in $\mathbb M^2(\kappa )$; thus, for any point $p\in \Gamma := \partial \Omega$ there is a complete geodesic line $\Gamma _p\subset \mathbb M^2(\kappa )$ such that $\Omega$ remains in one open component of $\mathbb M^2(\kappa ) \setminus \Gamma _p$.

We assume that the $C^0$ Jordan curve $\Gamma \subset \mathbb M^2(\kappa )$ is constituted of two families of open geodesic arcs $A_1,\dots ,A_a$, $B_1,\dots ,B_b$ and a family of open arcs $C_1,\dots ,C_c$ with their endpoints. We assume also that no two $A_i$ and no two $B_j$ have a common endpoint.

On each open arc $C_k$, we assign a continuous boundary data $g_k$.

Let $P\subset \overline{\Omega }$ be any polygon whose vertices are chosen among the endpoints of the open geodesic arcs $A_i, B_j$, we call P an admissible polygon. We set

$$\begin{aligned} \alpha (P) = \sum _{A_i\subset P} \Vert A_i\Vert ,\ \beta (P) = \sum _{B_j\subset P} \Vert B_j\Vert , \ \gamma (P)= \text {perimeter of }\ P. \end{aligned}$$

With the above notations, the Jenkins–Serrin’s theorem asserts the following:

If the family $\{C_k \}$ is not empty, then there exists a function $u: \Omega \longrightarrow ~\mathbb R$ whose graph is a minimal surface in $\mathbb E(\kappa ,\tau )$ and such that

$$\begin{aligned} u_{\vert A_i}=+\infty ,\ u_{\vert B_j}=-\infty ,\ u_{\vert C_k}=g_k \end{aligned}$$

if and only if

$$\begin{aligned} 2\alpha (P)< \gamma (P),\ \ 2\beta (P) < \gamma (P) \end{aligned}$$

(2)

for any admissible polygon P. In this case, the function u is unique.

If the family $\{C_k \}$ is empty such a function u exists if and only if $\alpha (\Gamma ) = \beta (\Gamma )$ and condition (2) holds for any admissible polygon $P\not = \Gamma$. In this case, the function u is unique up to an additive constant.

We denote by $\Sigma \subset \mathbb E(\kappa ,\tau )$ the graph of u over $\Omega$, and we call such a surface a Jenkins–Serrin type minimal surface.

Remark 3.1

We observe that when the family $\{C_k \}$ is empty, the boundary of $\Sigma$ is the union of vertical geodesic line $\{q\} \times \mathbb R$ for any common endpoint q between geodesic arcs $A_i$ and $B_j$.

Suppose that the family $\{C_k \}$ is not empty and let $x_0$ be a common vertex between $A_i$ and $C_k$, if any. If $g_k$ has a finite limit at $x_0$, say $\alpha$, then the half vertical line $\{x_0 \}\times [\alpha , +\infty [\,$ lies in the boundary of $\Sigma$. Now if $x_0$ is a common vertex between $B_j$ and $C_k$ and if $g_k$ has a finite limit at $x_0$, say $\beta$, then the half vertical line $\{x_0 \}\times \,]-\infty , \beta ]$ lies in the boundary of $\Sigma$. At last, if $x_0$ is a common vertex between $C_i$ and $C_k$ and if $g_i$ and $g_k$ have different finite limits at $x_0$, say $\alpha < \beta$, then the vertical segment $\{x_0 \}\times [\alpha , \beta ]$ lies in the boundary of $\Sigma$.

4 Main theorem

For any vertical geodesic line L of $\mathbb E(\kappa ,\tau )$, we denote by $I_L$ the reflection about the line L.

Theorem 4.1

Using the notations of Sect. 3 and under the assumptions of Remark 3.1, let $\gamma \subset \{x_0 \}\times \mathbb R:= L \subset \mathbb E(\kappa ,\tau )$ be a vertical component of the boundary of the open minimal vertical graph $\Sigma \subset \mathbb E(\kappa ,\tau )$, where $\kappa <0$ and $\tau \geqslant 0$.

Then, we can extend minimally $\Sigma$ by reflection about L. More precisely, $S := \Sigma \, \cup \gamma \cup I_L (\Sigma )$ is a smooth minimal surface invariant by the reflection about $\Gamma$, containing $\mathrm{int}( \gamma )$ in its interior.

Furthermore, the same statement and proof hold for $\Sigma \subset \mathbb R^3$ or $\Sigma \subset {\mathbb {S}}^2\times \mathbb R$.

Observe that the possible cases for $\gamma$ are the following: the whole line L, a half line of L or a closed geodesic arc of L.

Observe also that since we are under the assumptions of Remark 3.1, if $x_0$ is an endpoint of some arc $C_i$, then $g_i$ has a finite limit at $x_0$.

Remark 4.2

We use the same notations as in Theorem 4.1. Suppose that the boundary of $\Sigma$ contains an open arc $\delta$ (graph over an arc $C_k$) of a horizontal geodesic line $\Upsilon$ of $\widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )$.

We denote by $I_\Upsilon$ the reflection in $\widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )$ about $\Upsilon$.

We can prove as in [20, Lemma 3.6] (in ${\mathbb {H}}^2 \times \mathbb R)$ that we can extend $\Sigma$ by reflection about $\Upsilon$: $\Sigma \, \cup \, \delta \cup I_\Upsilon (\Sigma )$ is a connected smooth minimal surface containing $\delta$ in its interior. The same observation holds also in Heisenberg space and ${\mathbb {S}}^2\times \mathbb R$.

On the other hand, we can verify that the proof of Theorem 4.1 also works for reflection about horizontal geodesic lines.

Proof

For the sake of clarity and simplicity of notations, we provide the proof in $\widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )=\mathbb E(-1,\tau )$. Nevertheless, all arguments and constructions hold in $\mathbb E(\kappa ,\tau )$ for any $\kappa <0$ and $\tau \geqslant 0$, in $\mathbb R^3$, that is for $\kappa =\tau =0$ and in ${\mathbb {S}}^2\times \mathbb R$, that is for $\kappa =1$ and $\tau =0$.

We assume that the family $C_k$ is not empty. The other situation can be handled in a similar way.

Recall that, by assumption, if $x_0$ is an endpoint of some arc $C_i$ (if any), then $g_i$ has a finite limit at $x_0$.

We suppose that all functions $g_k$ admit also a finite limit at the endpoints of $C_k$ different of $x_0$ (if any). It is possible to carry out a proof without this assumption, but the details are cumbersome, as we can see in the following.

Suppose, for instance, that $x_1$ ($\not = x_0$) is an endpoint of some arc $C_k$, that $g_k$ has no limit at $x_1$ and that $g_k$ is bounded near $x_1$. Setting $\alpha =\frac{1}{2}(\liminf _{x \rightarrow x_1} g_k(x) + \limsup _{x \rightarrow x_1} g_k(x))$, we can find a sequence $(p_n)$ on $C_k$ such that

$$\begin{aligned} p_n \rightarrow x_1 \quad \text {and} \quad g_k(p_n)=\alpha \text { for any } n. \end{aligned}$$

Then, we consider the new function $g_{k,n}$ on $C_k$ setting $g_{k,n}(x)=\alpha$ on the segment $[x_1,p_n]$ of $C_k$ and $g_{k,n}=g_k$ outside this segment. Now the continuous function $g_{k,n}$ has a limit at $x_1$. Observe that for any $x\in C_k$, we have $g_{k,n}(x)=g_k(x)$ for any n large enough.

If $g_k$ is not bounded near $x_1$, we first truncate, for any $n>0$, the function $g_k$ above by n and below by -n. We obtain a new continuous and bounded function $h_{k,n}$ on $C_k$. Then, we proceed as above.

For any integer n, we consider the Jordan curve $\Gamma _n$ obtained by the union of the geodesic arcs $A_i$ at height n, the geodesic arcs $B_j$ at height $-n$, the graphs of functions $g_k$ over the open arcs $C_k$ (or $g_{k,n}$ if $g_k$ has no finite limit at some endpoint of $C_k$), and the vertical segments necessary to form a Jordan curve. Thus, $\Gamma$ is the projection of $\Gamma _n$ on ${\mathbb {H}}^2$.

Let $\Sigma _n\subset \widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )$ be the embedded area minimizing disc with boundary $\Gamma _n$ given by Proposition 6.1 in “Appendix.” We have

• $\Sigma _n\subset \overline{\Omega }\times \mathbb R$,

• $\mathring{\Sigma }_n:=\Sigma _n\setminus \Gamma _n=\Sigma _n \cap (\Omega \times \mathbb R)$ is a vertical graph over $\Omega$.

We set $\gamma _n := \Sigma _n \cap L$, where $L:=\{x_0\} \times \mathbb R$, thus $\gamma _n \subset \gamma$ for any n. Due to the fact that $\Sigma _n$ is area minimizing, we can apply the reflection principle about the vertical line L, and this is proven in detail in [21, Proposition 3.4]. Thus, $S_n:= \Sigma _n \cup I_L ( \Sigma _n)$ is an embedded minimal surface containing $\mathrm{int}(\gamma _n)$ in its interior. By construction, $S_n$ is invariant under the reflection $I_L$ and is orientable.

Let $u_n : \Omega \longrightarrow \mathbb R$ be the function whose the graph is $\mathring{\Sigma }_n$. Thus, $u_n$ extends continuously by n on the edges $\mathrm{int} (A_i)$, by $-n$ on the edges $\mathrm{int} (B_j)$ and by $g_k$ (or $g_{k,n}$) over the open arcs $C_k$. Using the lemmas derived in [25], following the original proof of [7, Theorem 2], it can be proved that, up to considering a subsequence, the sequence of functions $(u_n)$ converges to a function $u:\Omega \longrightarrow \mathbb R$ in the $C^2$-topology, uniformly over any compact subset of $\Omega$.

Let $d_n$ be the intrinsic distance on $S_n$. For any $p\in S_n$ and any $r>0$, we denote by $B_n(p,r)\subset S_n$ the open geodesic disc of $S_n$ centered at p with radius r. By construction, for any $p\in \mathrm{int}(\gamma )$ there exist $n_p\in \mathbb N$ and a real number $c_p>0$ such that for any integer $n\geqslant n_p$ we have $p\in \mathrm{int}(\gamma _n)\subset \mathrm{int} (S_n)$ and $d_n (p, \partial S_n) > 2 c_p$.

We assert that the Gaussian curvature $K_n$ of the surfaces $S_n$ is uniformly bounded in the neighborhood of each point of $\mathrm{int}(\gamma )$, independently of n.

Proposition 4.3

For any $p\in \mathrm{int}(\gamma )$, there exist $R_p, K_p >0$, and there exists $n_p \in \mathbb N$ satisfying $p \in \mathrm{int} (\gamma _{n_p}) \subset S_{n_p}$ and $d_{n_p}(p,\partial S_{n_p}) > 2R_p$, such that for any integer $n\geqslant n_p$ we have $p\in \mathrm{int} (\gamma _n )\subset S_n$ and

$$\begin{aligned} |K_n (x) |\leqslant K_p, \end{aligned}$$

for any $x\in B_n (p,R_p)$.

We postpone the proof of Proposition 4.3 until Sect. 5.

Assuming Proposition 4.3, we will prove that for any $p \in \mathrm{int}(\gamma )$ there exists an embedded minimal disc D(p), containing p in its interior, such that $D(p) \subset \Sigma \cup \gamma \cup I_L(\Sigma )$. This will prove that $\Sigma \cup \gamma \cup I_L(\Sigma )$ is a minimal surface, that is smooth along $\mathrm{int} (\gamma )$.

Let $p\in \mathrm{int}(\gamma )$, we deduce from Proposition 4.3 that there exist real numbers $R_p, K_p >0$ and $n_p\in \mathbb N$ such that for any integer $n \geqslant n_p$ and for any point $x \in B_n(p,R_p)$, we have $|K_n(x)|\leqslant K_p$. By construction, $B_n(p,R_p)$ is an embedded minimal disc.

Therefore, it can be proved as in [18], using [18, Proposition 2.3, Lemma 2.4] and the discussion that follows that up to taking a subsequence, the geodesic discs $B_n(p,R_p)$ converge for the $C^2$-topology to a minimal disc $D(p)\subset \mathbb R^3$ containing p in its interior. We recall that each geodesic disc $B_n(p,R_p)$ is embedded, contains an open subarc $\gamma (p)$ of $\gamma$ (which does not depend on n) passing through p, and $B_n(p,R_p)$ is invariant under the reflection $I_L$. Thereby, the minimal disc D(p) also is embedded, contains the subarc $\gamma (p)$ and inherits the same symmetry.

We set $S:=\Sigma \cup \gamma \cup I_L(\Sigma )$.

By construction, the surfaces $\mathrm{int}( S_n)\setminus L$ converge to $\Sigma \cup I_L (\Sigma )$. We observe that $B_n(p,R_p)\setminus L \subset S_n\setminus L$ and then $D(p) \setminus L \subset \Sigma \cup I_L(\Sigma )$. Then, we have $D(p)\subset S$. We conclude henceforth that S is a smooth minimal surface invariant under the reflection $I_L$, and this accomplishes the proof of the theorem. $\square$

Remark 4.4

Theorem 4.1 holds also in case where $x_0$ is and endpoint of some arc $C_i$ and $g_i$ has an infinite limit at $x_0$.

Indeed, assume that $\lim _{x \rightarrow x_0} g_i(x)= +\infty$. We denote by $g_{i,n}$ the new function on $C_i$ obtained by truncating the function $g_i$ above by n. Then, in the proof of Theorem 4.1, we consider the embedded area minimizing disc $\Sigma _n$ constructed with the function $g_{i,n}$ on $C_i$ (instead of $g_i)$. Then, we can proceed the proof in the same way.

Remark 4.5

We do not know if the Jenkins–Serrin type theorem was established in the Heisenberg spaces $Nil_3(\tau )=\mathbb E(0,\tau )$ for $\tau > 0$. Assuming the Jenkins–Serrin type theorem, the proof of Theorem 4.1 works to establish the same reflection principle for vertical geodesic lines in $Nil_3(\tau )$.

5 Proof of Proposition 4.3

We argue by absurd.

Suppose by contradiction that there exists $p\in \mathrm{int}(\gamma )$ such that for any $k\in \mathbb N^*$, there exist an integer $n_k > k$ and $x_k \in B_{n_k}(p, \frac{1}{k})$ such that $|K_{n_k}(x_k) |> k^2$.

There exist $c>0$ and $k_0 \in \mathbb N^*$ such that for any integer $k\geqslant k_0$, we have $p\in \mathrm{int} (\gamma _{n_k})$ and $d_{n_k}(p,\partial S_{n_k})>2c$. Thus, $\overline{B}_{n_k}(p,c) \subset \mathrm{int} (S_{n_k})$.

Moreover, there exists an integer $k_1 > k_0$ such that for any integer $k \geqslant k_1$ we have $d_{n_k}(x_k,\partial B_{n_k}(p,c)) > c/2$.

From now on, we are going to use classical blow-up techniques.

Define the continuous function $f_k: \overline{B}_{n_k}(p,c) \longrightarrow [0, + \infty [\,$ for any $k\geqslant k_1$, setting: $f_k(x)=\sqrt{|K_{n_k}(x)|} \,d_{n_k}(x,\partial B_{n_k}(p,c))$.

Clearly, $f_k\equiv 0$ on $\partial B_{n_k}(p,c)$ and

$$\begin{aligned} f_k(x_k)=\sqrt{|K_{n_k} (x_k)|}\, d_{n_k}(x_k,\partial B_{n_k}(p,c)) \geqslant k \frac{c}{2}. \end{aligned}$$

We fix a point $p_k\in B_{n_k}(p,c)$ where the function $f_k$ attains its maximum value; hence,

$$\begin{aligned} f_k(p_k)\geqslant k\frac{c}{2}. \end{aligned}$$

(3)

We deduce therefore

$$\begin{aligned} \lambda _k:=\sqrt{|K_{n_k}(p_k)|} \geqslant \frac{k c}{2d_{n_k}(p_k,\partial B_{n_k}(p,c))} \geqslant \frac{kc}{2c}=\frac{k}{2} . \end{aligned}$$

(4)

Notation 5.1

We set $\rho _k = d_{n_k}(p_k,\partial B_{n_k}(p,c))$, and we denote by $D_k \subset B_{n_k}(p,c) \subset S_{n_k}$ the open geodesic disc with center $p_k$ and radius $\rho _k /2$. Notice that $D_k$ is embedded.

For further purpose, we emphasize that $D_k$ is an orientable minimal surface of $\widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )$.

Let us consider the model of $\widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )={\mathbb {E}}(-1,\tau )$ given by (1) for $\kappa =-1$, that is the product set $\mathbb D(2)\times \mathbb R$ equipped with the metric

$$\begin{aligned} \mathrm{d}s^2:= \mu ^2(\mathrm{d}x^2 + \mathrm{d}y^2) + \big (\tau \mu (y\mathrm{d}x - x\mathrm{d}y) +\mathrm{d}t \big )^2 \end{aligned}$$

(5)

where $\mu =\mu (x,y)= \dfrac{1}{1-\frac{x^2+y^2}{4}}$.

For any integer $k\geqslant k_1$, we set $\mu _k= \mu _k(u,v)=\dfrac{1}{1-\frac{u^2+v^2}{4\lambda _k^2}}$. We consider, as in the Nguyen’s thesis [13, Section 2.2.3], the product set $\mathbb D(2\lambda _k) \times \mathbb R$ equipped with the metric

$$\begin{aligned} \mathrm{d}s_k^2 := \mu _k^2(\mathrm{d}u^2 + \mathrm{d}v^2) + \left( \frac{\tau }{\lambda _k}\, \mu _k (v\mathrm{d}u - u\mathrm{d}v) + \mathrm{d}w \right) ^2. \end{aligned}$$

(6)

Thus, $(\mathbb D(2\lambda _k) \times \mathbb R,\, \mathrm{d}s_k^2)$ is a model of $\mathbb E(\frac{-1}{\lambda _k^2}, \frac{\tau }{\lambda _k})$.

Remark 5.2

Since $\widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )$ is a homogeneous space, for any integer $k\geqslant k_1$, up to considering an isometry of $\widetilde{\mathrm{PSL}}_2(\mathbb R,\tau )$ which sends $p_k$ to the origin $0_3:=(0,0,0)$, we can assume that $p_k=0_3$. See, for example, [14, Chapter 5] or [13, Proposition 1.1.7].

Let us consider the homothety

$$\begin{aligned} H_k : \mathbb D(2) \times \mathbb R&\longrightarrow \mathbb D(2\lambda _k) \times \mathbb R\\ (x,y,t)&\longmapsto (u,v,w)= \lambda _k\, (x,y,t). \end{aligned}$$

We have $H_k^*(\mathrm{d}s_k^2)= \lambda _k^2\, \mathrm{d}s^2$, see (5) and (6). Then, it follows that $\widetilde{D}_k := H_k (D_k)$ is an embedded minimal surface of $(\mathbb D(2\lambda _k) \times \mathbb R,\, \mathrm{d}s_k^2)$.

By construction, $\widetilde{D}_k$ is a geodesic disc with center the origin $0_3$ of $\mathbb D(2\lambda _k) \times \mathbb R$ : $0_3 \in \widetilde{D}_k \subset \mathbb D(2\lambda _k) \times \mathbb R$. Moreover, the radius of $\widetilde{D}_k$ is $\widetilde{\rho }_k =\lambda _k \cdot$(radius of $D_k$), that is $\widetilde{\rho }_k=\lambda _k \, \rho _k/2$.

Using the estimate (3), we get

$$\begin{aligned} \widetilde{\rho }_k=\lambda _k \, \rho _k/2 =\sqrt{|K_{n_k}(p_k)|}\,\, d_{n_k}(p_k,\partial B_{n_k}(p,c))/2 = \frac{f_k(p_k)}{2} \geqslant \frac{kc}{4}, \end{aligned}$$

(7)

thus $\widetilde{\rho }_k \rightarrow \infty$ if $k \rightarrow \infty$.

Let $g_{\mathrm{euc}}= \mathrm{d}u^2 + \mathrm{d}v^2 + \mathrm{d}w^2$ be the Euclidean metric of $\mathbb R^3$. We observe that $(\mathbb D(2\lambda _k) \times \mathbb R,\, \mathrm{d}s_k^2)$ converges to $(\mathbb R^2\times \mathbb R, \, g_{\mathrm{euc}})$ for the $C^2$-topology, uniformly on any compact subset of $\mathbb R^3$.

We denote by $\widetilde{K}_{n_k}$ the Gaussian curvature of $\widetilde{D}_k$. For any $x\in D_k\subset \mathbb D(2) \times \mathbb R$, setting $X=H_k(x) \in \widetilde{D}_k \subset \mathbb D(2\lambda _k) \times \mathbb R$, we get $\widetilde{K}_{n_k} (X) = \frac{K_{n_k}(x)}{\lambda _k^2}$. Hence, for any $X\in \widetilde{D}_k$ we obtain

$$\begin{aligned} \sqrt{|\widetilde{K}_{n_k} (X)|}&=\frac{\sqrt{|K_{n_k}(x)|}}{\lambda _k} \leqslant \frac{f_k(p_k)}{\lambda _k \,d_{n_k}(x,\partial B_{n_k}(p,c)) } \nonumber \\&= \frac{d_{n_k}(p_k,\partial B_{n_k}(p,c))}{d_{n_k}(x,\partial B_{n_k}(p,c))} < 2, \end{aligned}$$

(8)

since $d_{n_k}(x,\partial B_{n_k}(p,c)) >\dfrac{\rho _k}{2}$.

Furthermore, for any integer $k\geqslant k_1$ we have

$$\begin{aligned} \sqrt{|\widetilde{K}_{n_k} (0_3)|}=\frac{\sqrt{|K_{n_k} (p_k)|}}{\lambda _k}=1. \end{aligned}$$

(9)

We summarize some facts derived before:

Lemma 5.3

• each $\widetilde{D}_k$ is an embedded and orientable minimal surface of $(\mathbb D(2\lambda _k) \times \mathbb R,\, \mathrm{d}s_k^2)= \mathbb E(-\frac{1}{\lambda _k^2},\frac{\tau }{\lambda _k})$,

• there is a uniform estimate of Gaussian curvature, see (8),

• the radius $\widetilde{\rho }_k$ of the geodesic disc $\widetilde{D}_k$ go to $+\infty$ if $k \rightarrow \infty$, see (7),

• the metrics $\mathrm{d}s_k^2$ converge to $g_{\mathrm{euc}}$ for the $C^2$-topology, uniformly on any compact subset of $\mathbb R^3$, see (6).

Therefore, it can be proved as in [18] (using [18, Proposition 2.3, Lemma 2.4] and the discussion that follows) that up to considering a subsequence, the $\widetilde{D}_k$ converge for the $C^2$-topology to a complete, connected and orientable minimal surface $\widetilde{S}$ of $\mathbb R^3$.

Remark 5.4

From the construction described in [18], the surface $\widetilde{S}$ has the following properties.

There exist $r, r_0>0$ such that for any $q\in \widetilde{S}$, a piece $\widetilde{G} (q)$ of $\widetilde{S}$, containing the geodesic disc with center q and radius $r_0$, is a graph over the open disc D(q, r) of $T_q \widetilde{S}$ with center q and radius r (for the Euclidean metric of $\mathbb R^3)$. Furthermore,

• for k large enough, a piece $\widetilde{G}_k (q)$ of $\widetilde{D}_k\subset \mathbb D(2\lambda _k) \times \mathbb R$ is also a graph over D(q, r) and the surfaces $\widetilde{G}_k(q)$ converge for the $C^2$-topology to $\widetilde{G}(q)$,

• for any $y\in \widetilde{G} (q)$ there exists $k_y\in \mathbb N$ such that for any $k\geqslant k_y$, we can choose the piece $\widetilde{G}_k (y)$ of $\widetilde{D}_k$ such that $\widetilde{G}_k (q) \cup \widetilde{G}_k(y)$ is connected.

By construction, we have $0_3\in \widetilde{S}$ and, denoting by $\widetilde{K}$ the Gaussian curvature of $\widetilde{S}$ in $(\mathbb R^3, g_{\mathrm{euc}})$, we deduce from (9)

$$\begin{aligned} |\widetilde{K}(0_3)|=1. \end{aligned}$$

(10)

For any integer $k\geqslant k_1$, we set $\widetilde{L}_k:= H_k (L)$. Thus, $\widetilde{L}_k$ is a vertical straight line of $\mathbb R^3$.

Definition 5.5

Let $\delta _k$ be the distance in $\mathbb D(2\lambda _k) \times \mathbb R$ induced by the metric $\mathrm{d}s_k^2$.

We say that the sequence of vertical lines $(\widetilde{L}_k)$ in $\mathbb R^3$disappears to infinity if $\delta _k (0_3, \, \widetilde{L}_k) \rightarrow +\infty$ when $k \rightarrow +\infty$

There are two possibilities: The sequence $(\widetilde{L}_k)$ disappears or not to infinity. We are going to show that either case cannot occur, we will find therefore a contradiction.

First case:$(\widetilde{L}_k)$ disappears to infinity.

Observe that, by construction, the geodesic discs $B_{n_k}(p,c)$ are invariant under the reflection $I_L$ and $f_k(q)=f_k( I_L(q))$ for any $q\in B_{n_k}(p,c)$. Since $p_k=0_3$ by assumption, we can assume that $0_3\in \Sigma _{n_k} \subset S_{n_k}$ for any $k\geqslant k_1$.

Let $q \in \widetilde{S}$ and consider a minimizing geodesic arc $\delta \subset \widetilde{S}$ joining $0_3$ to q. It follows from Remark 5.4 that there exist a finite number of points $q_1=0_3,\dots , q_n=q$ belonging to $\delta$, and there exists $k_q \in \mathbb N$ such that:

• for any integer $k \geqslant k_q$, the subset $\cup _j \widetilde{G}_k (q_j)\subset \widetilde{D}_k$ is connected and converges for the $C^2$-topology to the subset $\cup _j \widetilde{G} (q_j)\subset \widetilde{S}$,

• for any integer $k \geqslant k_q$, we have $\big (\cup _j \widetilde{G}_k (q_j)\big )\cap \widetilde{L}_k=\emptyset$.

Thus, for any integer $k \geqslant k_q$ we obtain that $H_k^{-1}(\cup _j \widetilde{G}_k (q_j)) \cap L=\emptyset$, that is $H_k ^{-1}(\cup _j \widetilde{G}_k (q_j))\subset D_k\cap \Sigma _{n_k}$.

Setting $\widehat{D}_k := H_k (D_k \cap \Sigma _{n_k})$, we deduce that the sequence $(\widehat{D}_k)$ converges to $\widetilde{S}$ for the $C^2$-topology too. Observe that $\widehat{D}_k \cap \widetilde{L}_k \subset \partial \widehat{D}_k$. Therefore, any minimal surface $\widehat{D}_k \setminus \widetilde{L}_k$ is a Killing graph, and thus, $\widehat{D}_k$ is a stable minimal surface of $\mathbb E(\frac{-1}{\lambda _k^2}, \frac{\tau }{\lambda _k})$.

Therefore, it can be proved as in the discussion following Lemma 2.4 in [18] that $\widetilde{S}$ is a connected, complete, orientable and stable minimal surface of $\mathbb R^3$. Thanks to the results of do Carmo and Peng [3], Fischer-Colbrie and Schoen [4] and Pogorelov [16], $\widetilde{S}$ is a plane. But this gives a contradiction with the curvature relation (10).

Second case:$(\widetilde{L}_k)$ does not disappear to infinity.

We will prove that the Gauss map of $\widetilde{S}$ omits infinitely many points, and hence, $\widetilde{S}$ would be a plane (see [5, Corollary 1.3] or [24]), contradicting the curvature relation (10).

Let $\alpha \in (0,\pi ]$ be the interior angle of $\Gamma$ at vertex $x_0$. ($\alpha$ exists since $\Gamma$ is the boundary of a convex domain.) Observe that the case where $\alpha =\pi$ is under consideration.

Since $\Omega$ is convex, there exists a geodesic line $C_{x_0} \subset {\mathbb {H}}^2$ at $x_0$ such that $C_{x_0}\cap \Omega =\emptyset$. Let $\Pi$ be the product $C_{x_0} \times \mathbb R$ in $(\mathbb D(2) \times \mathbb R, \mathrm{d}s^2)=\mathbb E(-1,\tau )$. When $\tau =0$ notice that $\Pi$ is a vertical totally geodesic plane in ${\mathbb {H}}^2 \times \mathbb R$. We recall that there are no totally geodesic surfaces in $\mathbb E(-1,\tau )$ if $\tau \not =0$, see [23, Theorem 1].

Under our assumption, up to considering a subsequence, we can assume that the sequence $(\widetilde{L}_k)$ converges to a vertical straight line $\widetilde{L} \subset \mathbb R^3$ and that $\big (H_k (\Pi )\big )$ converges to a vertical plane $\widetilde{\Pi }\subset \mathbb R^3$ containing $\widetilde{L}$. Let us denote by $\widetilde{\Pi }^+$ and $\widetilde{\Pi }^-$ the two open halfspaces of $\mathbb R^3$ bounded by $\widetilde{\Pi }$.

Lemma 5.6

We have $(\widetilde{S} \cap \widetilde{\Pi })\setminus \widetilde{L}=\emptyset$.

Proof

Otherwise, assume there exists a point $q\in \widetilde{S} \cap \widetilde{\Pi }$ such that $q\not \in \widetilde{L}$. From the structure of the intersection of two minimal surfaces tangent at a point, see [1, Theorem 7.3] or [22, Lemma, p. 380], we may suppose that $\widetilde{\Pi }$ is transverse to $\widetilde{S}$ at q. Thus, there is an open piece $\widetilde{F}(q)$ of $\widetilde{S}$ containing q which is transverse to the plane $\widetilde{\Pi }$. Hence, for any integer k large enough, a piece $\widetilde{F}_k(q)$ of $\widetilde{D}_k \subset \mathbb D(2\lambda _k) \times \mathbb R$ is so close to $\widetilde{F}(q)$ that it is transverse to $\widetilde{\Pi }$ too. Consequently, we would have $\mathrm{int} (\widetilde{D}_k )\cap \big (H_k (\Pi )\setminus \widetilde{L}_k\big ) \not = \emptyset$, that is $\mathrm{int} (D_k) \cap (\Pi \setminus L)\not = \emptyset$. But by construction, we have $\mathrm{int}(S_{n_k}) \cap (\Pi \setminus L)=\emptyset$, which leads to a contradiction since $D_k \subset S_{n_k}$. $\square$

Lemma 5.7

We have $\widetilde{S} \cap \widetilde{\Pi }=\widetilde{L}$.

Proof

Assume first that $\widetilde{S} \cap \widetilde{\Pi }=\emptyset$. Hence, $\widetilde{S}$ stay in an open halfspace, say $\widetilde{\Pi }^+$, of $\mathbb R^3$ bounded by $\widetilde{\Pi }$. Observe that the halfspace $\widetilde{\Pi }^+$ is the limit of open subspaces $H_k(\Pi ^+)$ of $\mathbb D(2\lambda _k)\times \mathbb R$ where $\Pi ^+$ is one of the two open halfspaces of $\mathbb D(2) \times \mathbb R$ bounded by $\Pi$. Consequently, $\widetilde{S}$ is the limit of the graphs $\widetilde{D}_k \cap H_k (\Pi ^+)$. Therefore, as in the first case, we obtain that $\widetilde{S}$ is stable and thus is a plane, giving a contradiction with (10). We obtain therefore $\widetilde{S} \cap \widetilde{\Pi }\not =\emptyset$.

Let $q\in \widetilde{S} \cap \widetilde{\Pi }$. We deduce from Lemma 5.6 that $q\in \widetilde{L}$. If $\widetilde{\Pi }$ were the tangent plane of $\widetilde{S}$ at q, then the intersection $\widetilde{S} \cap \widetilde{\Pi }$ would consist in a even number $\geqslant 4$ of arcs issued from q, see [1, Theorem 7.3] or [22, Lemma, p. 380]. Then, we infer that $\widetilde{S} \cap (\widetilde{\Pi }\setminus \widetilde{L})\not =\emptyset$ which is not possible due to Lemma 5.6.

Thus, $\widetilde{\Pi }$ is transverse to $\widetilde{S}$ at q. Since $\widetilde{S}\cap \widetilde{\Pi }\subset \widetilde{L}$, we deduce from Lemma 5.6 that $\widetilde{S}\cap \widetilde{\Pi }$ contains an open arc of $\widetilde{L}$ containing q. This proves that $\widetilde{S}\cap \widetilde{\Pi }$ contains a segment of $\widetilde{L}$. It is well known that if a complete minimal surface of $\mathbb R^3$ contains a segment of a straight line, then it contains the whole straight line, see Proposition 6.3 in “Appendix.” We conclude that $\widetilde{S}\cap \widetilde{\Pi }=\widetilde{L}$ as desired. $\square$

Remark 5.8

To prove that $\widetilde{S} \cap \widetilde{\Pi }\not =\emptyset$, we can alternatively argue as follows. Assume that $\widetilde{S} \cap \widetilde{\Pi }=\emptyset$. By construction, $\widetilde{S}$ is a complete and connected minimal surface in $\mathbb R^3$ without self-intersection. Furthermore, we deduce from the estimates (8) that $\widetilde{S}$ has bounded curvature. It follows from [17, Remark] that $\widetilde{S}$ is properly embedded. Since $\widetilde{S}$ lies in a halfspace, we deduce from the halfspace theorem [6, Theorem 1] that $\widetilde{S}$ is a plane, which gives a contradiction with (10). Thus, $\widetilde{S} \cap \widetilde{\Pi }\not =\emptyset$.

We deduce from Lemma 5.7 that $\widetilde{S}\setminus \widetilde{\Pi }=\widetilde{S}\setminus \widetilde{L}$ has two connected components, say $\widetilde{S}^- \subset \widetilde{\Pi }^-$ and $\widetilde{S}^+ \subset \widetilde{\Pi }^+$. In the same way, we denote by $\Pi ^+$ and $\Pi ^-$ the two open halfspaces of $\mathbb D(2) \times \mathbb R$ bounded by $\Pi$. We have that $\widetilde{\Pi }^+$ (resp. $\widetilde{\Pi }^-$) is the limit of $H_k (\Pi ^+)$ (resp. $H_k (\Pi ^-)$).

We set $D_k^{\pm } := D_k \cap \Pi ^{\pm }$ and $\widetilde{D}_k^{\pm }:= H_k (D_k^{\pm })=\widetilde{D}_k\cap H_k(\Pi ^\pm )$. We observe that $\widetilde{D}_k^+$ and $\widetilde{D}_k^-$ are vertical graphs and that $\widetilde{S}^+$ (resp. $\widetilde{S}^-$) is the limit of $(\widetilde{D}_k^+)$ (resp. $(\widetilde{D}_k^-$)) for the $C^2$-topology.

For any integer $k\geqslant k_1$, we denote by $\widetilde{N}^k$ a smooth unit normal vector field on $\widetilde{D}_k$ with respect to the metric $\mathrm{d}s_k^2$, see (6). Let $\widetilde{N}_3^k$ be the vertical component of $\widetilde{N}^k$, this means that $\widetilde{N}^k -\widetilde{N}_3^k\frac{\partial }{\partial t}$ and $\frac{\partial }{\partial t}$ are orthogonal vector fields along $\widetilde{D}_k$.

Since $(\widetilde{D}_k)$ converges to $\widetilde{S}$ for the $C^2$-topology, we can define a unit normal field $\widetilde{N}$ on $\widetilde{S}$ as the limit of the fields $\widetilde{N}^k$.

Lemma 5.9

We have $\widetilde{N}_3\not =0$ on $\widetilde{S}^+ \cup \widetilde{S}^-$. Furthermore, $\widetilde{S}^+$ and $\widetilde{S}^-$ are vertical graphs.

Proof

Indeed, we know that $\widetilde{D}_k^+$ is a vertical graph. So we can assume that $\widetilde{N}_3^k >0$ along $\widetilde{D}_k^+$ for any $k \geqslant k_1$. By considering the limit of the fields $\widetilde{N}^k$, we get that $\widetilde{N}_3 \geqslant 0$ on $\widetilde{S}^+$.

Let $q\in \widetilde{S}^+$ be a point such that $\widetilde{N}_3 (q)=0$, if any. Recall that the Gauss map of a non-planar minimal surface of $\mathbb R^3$ is an open map. Therefore, in any neighborhood of q in $\widetilde{S}^+$ it would exist points $y \in \widetilde{S}^+$ such that $\widetilde{N}_3(y) <0$, which leads to a contradiction.

Thus, we have $\widetilde{N}_3\not =0$ on $\widetilde{S}^+$. We prove in the same way that $\widetilde{N}_3\not =0$ on $\widetilde{S}^-$ too.

Assume by contradiction that $\widetilde{S}^+$ is not a vertical graph. Then, there exist two points $q, \overline{q} \in \widetilde{S}^+$ lying to same vertical straight line. As the tangent planes of $\widetilde{S}^+$ at q and $\overline{q}$ are not vertical, there exists a real number $\delta >0$ such that a neighborhood $V_{\overline{q}} \subset \widetilde{S}^+$ of $\overline{q}$ and a neighborhood $V_q \subset \widetilde{S}^+$ of q are vertical graphs over an Euclidean disc of radius $\delta$ in the (u, v)-plane.

But, by construction, for k large enough a piece $U_{\overline{q}}$ of $\widetilde{D}_k^+$ is $C^2$-close of $V_{\overline{q}}$ and a piece $U_q$ of $\widetilde{D}_k^+$ is $C^2$-close of $V_q$. Clearly, this would imply that the vertical projections of $U_{\overline{q}}$ and $U_q$ on the (u, v)-plane have non-empty intersection. But this is not possible since $\widetilde{D}_k^+$ is a vertical graph. We conclude therefore that $\widetilde{S}^+$ is a vertical graph.

We can prove in the same way that $\widetilde{S}^-$ is a vertical graph. $\square$

End of the proof of the proposition

Let $P\subset \mathbb R^3$ be any vertical plane verifying $\widetilde{L}\subset P$ and $P\not = \widetilde{\Pi }$. We deduce from Lemmas 5.7 and 5.9 that $(\widetilde{S}\cap P)\setminus \widetilde{L}$ is a vertical graph. Therefore, the structure of the intersection of two minimal surfaces tangent at a point, see [1, Theorem 7.3] or [22, Lemma, p. 380], shows that there cannot be two distinct points of $\widetilde{L}$ where the tangent plane of $\widetilde{S}$ is P.

Let $\nu$ and $-\nu$ be the two unit vectors orthogonal to P. Since $\widetilde{N}_3\not =0$ on $\widetilde{S} \setminus \widetilde{L}$, we deduce that $\nu$ and $-\nu$ are not both assumed by the Gauss map of $\widetilde{S}$. By varying the vertical planes P, we obtain that the Gauss map of $\widetilde{S}$ omits infinitely many points (belonging to the equator of the 2-sphere). Then, $\widetilde{S}$ must be a plane, see [24, Theorem] or [5, Theorem I]. On account of (10), we arrive to a contradiction. This accomplishes the proof of the proposition. $\square$

Observe that, actually, the proof of Proposition 4.3 demonstrates the following result.

Proposition 5.10

Let $\Omega \subset \mathbb M^2(\kappa )$, $\kappa \leqslant 0$, be a convex domain. Let $C_1, C_2 \subset \partial \Omega$ be two open arcs admitting a common endpoint $x_0\in \partial \Omega$.

Let $(\Sigma _n)\subset \mathbb E(\kappa ,\tau )$, $\tau \geqslant 0$, be a sequence of minimal surfaces satisfying for any n:

• $\Sigma _n$ is a minimal surface with boundary, whose interior is the graph of a function $u_n$ defined on $\Omega$,

• the function $u_n$ extends continuously up to the open arcs $C_1$ and $C_2$,

• the restrictions of the map $u_n$ to $C_1$ and $C_2$ have both a finite limit at $x_0$,

• there exists a fixed open vertical segment $\gamma := {x_0}\times (a,b) \subset \mathbb E(\kappa ,\tau )$ such that $\gamma \subset \partial \Sigma _n$ and $\gamma$ is independent of n.

Then, the Gaussian curvature $K_n$ of the surfaces $S_n$ is uniformly bounded in the neighborhood of each point of $\gamma$. Namely, for any $p\in \gamma$ there exist $R_p, K_p >0$, satisfying for any n

$$\begin{aligned} d_n (p,\partial \Sigma _n \setminus \gamma ) > 2R_p\, ; \quad \mathrm{and} \quad |K_n (x) |\leqslant K_p \end{aligned}$$

for any $x\in \Sigma _n$ such that $d_n(p,x) < R_p$, where $d_n$ denotes the intrinsic distance on $\Sigma _n$.

Outline of the proof. We first choose points $p_i \in C_i$, $i=1,2$, and we denote by $\widetilde{C}_i$ the open arc of $C_i$ with endpoints $p_i$ and $x_0$, $i=1,2$. Then, we choose an open arc $\widetilde{C}_3 \subset \Omega$ with endpoints $p_1$ and $p_2$ such that the bounded domain $\widetilde{\Omega }\subset \Omega$ with boundary $\widetilde{C}_1 \cup \widetilde{C}_2 \cup \widetilde{C}_3 \cup \{ x_0, p_1, p_2\}$ is convex.

Let $\widetilde{\Gamma }_n \subset \mathbb E(\kappa ,\tau )$ be the Jordan curve constituted with the graph of $u_n$ over $\widetilde{C}_i$, $i=1,2,3$, the points $(p_j, u_n (p_j))$, $j=1,2$, and a vertical closed segment $\widetilde{\gamma }_n$ above $x_0$. Thus, $\gamma \subset \widetilde{\gamma }_n$ for any n.

Since $\widetilde{\Omega }$ is convex, we deduce from Proposition 6.1 in “Appendix” that $\widetilde{\Sigma }_n := \Sigma _n \cap \overline{\widetilde{\Omega }\times \mathbb R}$ is an area minimizing disc, solution of the Plateau problem for the Jordan curve $\widetilde{\Gamma }_n$. Thus, we can apply the reflection principle around $\gamma$ to $\widetilde{\Sigma }_n$, see [21, Proposition 3.4]. Then, we proceed as in the proof of Proposition 4.3.

Observe that the same result holds also for ${\mathbb {S}}^2\times \mathbb R$.

Appendix

Let $\Omega \subset \mathbb M(\kappa )$, $\kappa \leqslant 0$, be a bounded convex domain bounded by a $C^0$ Jordan curve $\Gamma := \partial \Omega$, and let $f : \Gamma \longrightarrow \mathbb R$ be a piecewise continuous function, allowing a finite number of discontinuities.

We denote by $\widetilde{\Gamma }\subset \mathbb E(\kappa , \tau )$, $\tau \geqslant 0$, the graph of f. Namely, if f is continuous, then $\widetilde{\Gamma }$ is a Jordan curve with a one-to-one projection on $\Gamma$. If f has discontinuity points, then $\widetilde{\Gamma }$ is constituted of a finite number of simple arcs admitting a one-to-one vertical projection on some subarc of $\Gamma$, and a vertical segment over each point of $\Gamma$ where f is not continuous.

We consider also a $C^0$ bounded convex domain $\Omega$ in ${\mathbb {S}}^2$. In this case, $\widetilde{\Gamma }\subset {\mathbb {S}}^2\times \mathbb R$.

Proposition 6.1

There exists an embedded area minimizing disc $\Sigma$ in $\overline{\Omega }\times \mathbb R\subset \mathbb E(\kappa , \tau )$, $\kappa \leqslant 0$, $\tau \geqslant 0$, (or in ${\mathbb {S}}^2\times \mathbb R)$ with boundary $\widetilde{\Gamma }$. Furthermore

• $\mathrm{int}(\Sigma )= \Sigma \setminus \widetilde{\Gamma }$ is a vertical graph over $\Omega$,

• If $\Sigma _0\subset \mathbb E(\kappa , \tau )$, (or in ${\mathbb {S}}^2\times \mathbb R)$ is any minimal surface bounded by $\widetilde{\Gamma }$ such that $\Sigma _0\setminus \widetilde{\Gamma }$ is a vertical graph over $\Omega$, then $\Sigma _0=\Sigma$.

Remark 6.2

For the existence of an embedded minimal disc in $\overline{\Omega }\times \mathbb R$ bounded by $\widetilde{\Gamma }$, we cannot use the well-known result of Meeks-Yau [9, Theorem 1], since the boundary of $\overline{\Omega }\times \mathbb R$ does not have the required regularity.

Proof

We perform the proof in $\mathbb E(\kappa ,\tau )$, and the proof in ${\mathbb {S}}^2\times \mathbb R$ is analogous.

Assume first that f has no discontinuity points. Since $\mathbb E(\kappa , \tau )$ is homogeneous, we deduce from a result of Morrey [10] that there exists a minimizing area disc $\Sigma$ bounded by $\widetilde{\Gamma }$. Since $\Omega$ is a convex domain, we deduce from the maximum principle that $\mathrm{int}(\Sigma )\subset \Omega \times \mathbb R$. (We use also the fact that if $\gamma \subset \mathbb M(\kappa )$ is a geodesic line, then $\gamma \times \mathbb R$ is a minimal surface of $\mathbb E(\kappa , \tau )$.)

Furthermore, as $\widetilde{\Gamma }$ has a one-to-one vertical projection on $\Gamma$, we infer that $\mathrm{int}(\Sigma )$ is a vertical graph over $\Omega$, as in Rado’s theorem [8, Theorem 16].

Thus, $\Sigma$ is an embedded area minimizing disc bounded by $\widetilde{\Gamma }$ and is a vertical graph over $\overline{\Omega }$. Clearly, $\Sigma$ is the unique minimal graph bounded by $\widetilde{\Gamma }$. The same affirmation holds in ${\mathbb {S}}^2\times \mathbb R$.

Assume now that f has a finite number of discontinuity points, let $x_0\in \Gamma$ be such a point. Giving an orientation to $\Gamma$, we have therefore

$$\begin{aligned} \lim _{\begin{array}{c} x \rightarrow x_0\\ x<x_0 \end{array}} f(x) \not = \lim _{\begin{array}{c} x \rightarrow x_0\\ x > x_0 \end{array}} f(x). \end{aligned}$$

Let $(p_n)$ be a sequence on $\Gamma$ such that

• $p_n \rightarrow x_0$ and $p_n > x_0$,

• for any n, the function f is continuous on the arc $(x_0, p_n]$ of $\Gamma$.

Now we modify f on the closed arc $[x_0, p_n]$ in such a way that the new function $f_n$ is strictly monotonous on $[x_0, p_n]$ and satisfies

$$\begin{aligned} f_n(x_0)= \lim _{\begin{array}{c} x \rightarrow x_0\\ x<x_0 \end{array}} f(x) , \qquad f_n(p_n) = f(p_n)\qquad \text {and} \qquad f_n(x)\not = f(x) \end{aligned}$$

for any $x\in (x_0,p_n)$.

We assume that we have modified in the same way f in a neighborhood of each point of discontinuity and we continue denoting $f_n$ the new function. Clearly, we have

• for each point $x\in \Gamma$ where f is continuous: $f_n(x) \rightarrow f(x)$,

• the function $f_n$ is continuous on $\Gamma$.

We denote by $\widetilde{\Gamma }_n$ the graph of $f_n$.

We know from the beginning of the proof that for any n, there exists an (unique) embedded area minimizing disc $\Sigma _n$ bounded by the Jordan curve $\widetilde{\Gamma }_n$, which is a vertical graph over $\overline{\Omega }$. Let $u_n : \overline{\Omega }\longrightarrow \mathbb R$ be the function whose the graph is $\Sigma _n$.

Since the sequence $(f_n)$ is uniformly bounded on $\Gamma$, we deduce from the maximum principle that the sequence $(u_n)$ is uniformly bounded on $\overline{\Omega }$ too. In [12, Section 4.1], it is stated a compactness principle in the case where the ambient space is Heisenberg space, but it can be stated and proved in the same way in $\mathbb E(\kappa ,\tau )$, $\kappa \leqslant 0$, $\tau \geqslant 0$, and also in ${\mathbb {S}}^2\times \mathbb R$.

Therefore, up to considering a subsequence, we can assume that the sequence of restricted maps $(u_{n|\Omega })$ converges on $\Omega$, for the topology $C^2$ and uniformly on any compact subset of $\Omega$, to a function $u : \Omega \longrightarrow \mathbb R$ satisfying the minimal surface equation. Thus, the graph of u is a minimal surface $\mathring{\Sigma }$ which has a one-to-one projection on $\Omega$.

Now we recall a result in $\mathbb E(\kappa ,\tau )$ for $\kappa < 0$ and $\tau >0$ proved in [25, Lemma 5.3], but which holds more generally for $\kappa \leqslant 0$ and $\tau \geqslant 0$, and also in ${\mathbb {S}}^2\times \mathbb R$.

Let $T\subset \mathbb M(\kappa )$ be an isosceles geodesic triangle and let $U\subset \mathbb M(\kappa )$ be the bounded convex domain with boundary T. We denote by A, B, C the open sides of T and by a, b, c the vertices of T. Assume that A and B have same length and that c is the common endpoint of A and B.

Then, for any real number $\alpha$ there exists a continuous function $v : U\cup (T\setminus \{a,b\}) \longrightarrow [0, \alpha ] \subset \mathbb R$ such that

• (1) v is $C^2$ on U and satisfies the minimal surface equation,

• (2) $v=0$ on $A\cup B\cup \{c\}$, and $v=\alpha$ on C.

Using those surfaces as barrier at each point $x\in \Gamma$ where f is continuous, as in the proof of [19, Theorem 3.4], we infer that u extends continuously up to x setting $u(x)=f(x)$. We deduce that the topological boundary of $\mathring{\Sigma }$ is the Jordan curve $\widetilde{\Gamma }$.

Setting $\Sigma := \mathring{\Sigma }\cup \widetilde{\Gamma }$, we have

• $\Sigma$ is an embedded minimal disc in $\overline{\Omega }\times \mathbb R$,

• $\partial \Sigma = \widetilde{\Gamma }$,

• $\mathrm{int}(\Sigma )= \mathring{\Sigma }\subset \Omega \times \mathbb R$, and $\mathrm{int}(\Sigma )$ is a vertical graph over $\Omega$.

Now we want to prove that $\Sigma$ is an area minimizing disc.

Observe first that $(\mathrm{Area} (\Sigma _n))$ is a bounded sequence. Thus, up to considering a subsequence, we can assume that $(\mathrm{Area} (\Sigma _n))$ is a convergent sequence. Recall that the sequence $(u_n)$ converges for the $C^2$ topology to u, uniformly on any compact subset of $\Omega$. Therefore, for any compact subset $K\subset \Omega$ we have

$$\begin{aligned} \mathrm{Area} (\mathrm{graph} (u_{|K}))= \lim \mathrm{Area} (\mathrm{graph} (u_{n|K})) \leqslant \lim (\mathrm{Area} (\Sigma _n)). \end{aligned}$$

Thus we get

$$\begin{aligned} \mathrm{Area} (\Sigma ) \leqslant \lim (\mathrm{Area} (\Sigma _n)). \end{aligned}$$

Suppose by contradiction that $\Sigma$ is not a minimizing area disc. Thus, considering a solution of the Plateau problem for $\widetilde{\Gamma }$, there exists a minimal immersed disc $S\subset \mathbb E(\kappa ,\tau )$ (or in ${\mathbb {S}}^2\times \mathbb R$), with

• $\partial S =\widetilde{\Gamma }$,

• $\mathrm{Area} (S) < \mathrm{Area} (\Sigma )$.

Using the maximum principle, we get

• $S \subset \overline{\Omega }\times \mathbb R$,

• $S\setminus \widetilde{\Gamma }\subset \Omega \times \mathbb R$.

Let again $x_0\in \Gamma$ be a point where f is not continuous, we use the same notations as in the beginning of the proof. For any n, we denote by $s_n$ the bounded subset of the cylinder $\Gamma \times \mathbb R$ bounded by the following curves:

• the graph of $f_n$ over the arc $[x_0, p_n]$ of $\Gamma$,

• the graph of f over the arc $(x_0, p_n]$,

• the vertical closed segment above $x_0$, with endpoints $(x_0,\lim _{\begin{array}{c} x \rightarrow x_0\\ x<x_0 \end{array}} f(x))$ and $(x_0,\lim _{\begin{array}{c} x \rightarrow x_0\\ x>x_0 \end{array}} f(x))$.

Clearly, we have $\mathrm{Area} (s_n) \rightarrow 0$. Furthermore, by the foregoing discussion there exists $\varepsilon >0$ such that $\mathrm{Area} (S) < \mathrm{Area} (\Sigma _n) -\varepsilon$ for any n large enough.

Let $\{x_i\}\subset \Gamma$ be the finite set of points where f is not continuous. For any point $x_i$, we denote by $s_n (x_i)$ the piece of $\Gamma \times \mathbb R$ constructed as above, corresponding to $x_i$.

Observe now that $S_n := S \cup \left( \cup _i s_n (x_i)\right)$ is a disc with boundary the Jordan curve $\widetilde{\Gamma }_n$. By construction, we have $\mathrm{Area} (S_n) < \mathrm{Area} (\Sigma _n)$ for any n large enough, contradicting the fact that $\Sigma _n$ is a minimizing area disc with boundary $\widetilde{\Gamma }_n$.

Thus, $\Sigma$ is an embedded minimizing area disc with boundary $\widetilde{\Gamma }$ such that $\Sigma \setminus \widetilde{\Gamma }$ is a vertical graph over $\Omega$.

It remains to prove that if $\Sigma _0\subset \mathbb E(\kappa , \tau )$ (or in ${\mathbb {S}}^2\times \mathbb R$) is a minimal surface bounded by $\widetilde{\Gamma }$ such that $\Sigma _0\setminus \widetilde{\Gamma }$ is a vertical graph over $\Omega$, then $\Sigma _0=\Sigma$.

Let $u_0: \Omega \longrightarrow \mathbb R$ be the function whose the graph is $\Sigma _0\setminus \widetilde{\Gamma }$. In [15, Theorem 1.3], A.L. Pinheiro proves a general maximum principle in a Riemannian product $\mathbb M\times \mathbb R$, where $\mathbb M$ is Riemannian surface. The proof can be adapted to $\mathbb E(\kappa , \tau )$, $\kappa \leqslant 0$, $\tau \geqslant 0$, thus $u_0=u$, that is $\Sigma _0=\Sigma$.

Observe also that in $\widetilde{\mathrm{PSL}}(2,\mathbb R)$, R. Younes proved directly that $u_0=u$, see [25, Theorem 1.1]. The proof can be adapted in $\mathbb E(\kappa , \tau )$, $\kappa \leqslant 0$, $\tau \geqslant 0$, and also in ${\mathbb {S}}^2\times \mathbb R$.

Thus, $\Sigma _0=\Sigma$, which concludes the proof. $\square$

Proposition 6.3

Let $M\subset \mathbb R^3$ be a complete minimal surface containing a segment of a straight line D. Then, the whole line D belongs to M: $D\subset M$.

Proof

We denote by x, y, z the coordinates on $\mathbb R^3$. Up to an isometry of $\mathbb R^3$, we can assume that D is the x-axis: $D=\{(x,0,0),\ x \in \mathbb R\}$.

By assumption, there exist real numbers $a<b$ such that $(x,0,0)\in M$ for any $x\in [a,b]$.

We set

$$\begin{aligned} B&:=\sup \{t >a,\ (x,0,0)\in M \ \text {for any } x\in [a,t]\},\\ A&:=\inf \{t<b,\ (x,0,0)\in M \ \text {for any } x\in [t,b] \}. \end{aligned}$$

We are going to prove that $A=-\infty$ and $B=+\infty$ to conclude that $D\subset M$.

We have $B\geqslant b$. Assume by contradiction that $B\not =+\infty$. Since M is a complete surface, we have $(B,0,0) \in M$. Let $P\subset \mathbb R^3$ be the plane containing D and the orthogonal direction of M at (B, 0, 0).

Since the surfaces M and P are transverse at (B, 0, 0), their intersection in a neighborhood of (B, 0, 0) is an analytic arc $\gamma$. Furthermore, up to choosing a smaller arc, we can assume that $\gamma$ is the graph, in P, of an analytic function f over the interval $[B-\varepsilon , B+\varepsilon ]$ for $\varepsilon >0$ small enough. Since f is an analytic function satisfying $f(x)=0$ for any $x\in [B-\varepsilon , B]$, we deduce that $f(x)=0$ for any $x\in [B-\varepsilon , B+\varepsilon ]$. Therefore, we have $(x,0,0) \in M$ for any $x\in [a,B+\varepsilon ]$, contradicting the definition of B.

Thus, we have $B=+\infty$. We prove in the same way that $A=-\infty$, concluding the proof. $\square$