Skip to main content
SpringerLink
Log in

Railway irregularity measuring using Rauch–Tung–Striebel smoothed multi-sensors fusion system: quad-GNSS PPP, IMU, odometer, and track gauge

  • Original Article
  • Published:
GPS Solutions Aims and scope Submit manuscript

Abstract

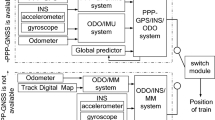
Precise track irregularity measuring is a pivotal technique to protect dynamic safety for railway transportation applications, especially those on high-speed railways. Current methods, such as the total station-based automatic inspection systems, commonly have a high cost and are inefficient. To reduce the costs and improve the measuring efficiency, a multi-sensors fusion system consisting of multi-constellation global navigation satellite systems (GNSS)-based precise point positioning, inertial navigation system, odometer, and track gauge is proposed and is further enhanced by using the Rauch–Tung–Striebel smoother. By using this system, one not only obtains high measuring accuracy, but also obtains increased efficiency of track irregularity measuring tens of times than achieved by conventional methods. After the principle investigations, a set of high-speed railway track measuring data containing quad-GNSS (GPS, BDS, GLONASS, and Galileo) observations, laser inertial measurement unit data, odometer measurements, and track gauge data are processed and analyzed to evaluate the capability of the proposed system. The results illustrate that the proposed system, which provided approximately 0.25, 0.59, 0.65, and 0.48 mm measuring accuracy in the super elevation, horizontal, vertical, and gauge components, respectively, can be used for high-accuracy track irregularity measuring.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

References

  • Alfi S, Bruni S (2008) Estimation of long wavelength track irregularities from on board measurement. In: 4th IET international conference on railway condition monitoring (RCM 2008), pp 1–6

  • Blewitt G (1990) An automatic editing algorithm for GPS data. Geophys Res Lett 17(3):199–202

    Article  Google Scholar 

  • Brown RG, Hwang PYC (1992) Introduction to random signals and applied Kalman filtering. Wiley, New York

    Google Scholar 

  • Chen Q, Niu X, Zhang Q, Cheng Y (2015) Railway track irregularity measuring by GNSS/INS integration. Navigation 62(1):83–93

    Article  Google Scholar 

  • Cheng S, Wang J, Peng W (2017) Statistical analysis and quality control for GPS fractional cycle bias and integer recovery clock estimation with raw and combined observation models. Adv Space Res 60(12):2648–2659

    Article  Google Scholar 

  • Chiang KW, Lin YC, Huang YW, Chang HW (2009) An ANN–RTS smoother scheme for accurate INS/GPS integrated attitude determination. GPS Solut 13(3):199–208

    Article  Google Scholar 

  • Cox DB (1978) Integration of GPS with inertial navigation systems. Navigation 25(2):236–245

    Article  Google Scholar 

  • Du S, Gao Y (2012) Inertial aided cycle slip detection and identification for integrated PPP GPS and INS. Sensors 12(11):14344–14362

    Article  Google Scholar 

  • Eom BG, Lee HS (2010) Assessment of running safety of railway vehicles using multibody dynamics. Int J Precis Eng Manuf 11(2):315–320

    Article  Google Scholar 

  • Europeenne BSN (2005) Testing and approval of railway vehicles from the point of view of their dynamic behavior-safety-track fatigue-ride quality. International Union of Railways, UIC Code, 518

  • Faiz RB, Singh S (2009) Condition monitoring of track geometry in UK rail. Condition monitoring of track geometry in UK rail. In: IEEE international conference on computing, engineering and information ICC’09, pp 182–190

  • Gao Z, Zhang H, Ge M, Niu X, Shen W, Wickert J, Schuh H (2015) Tightly coupled integration of ionosphere-constrained precise point positioning and inertial navigation systems. Sensors 15(3):5783–5802

    Article  Google Scholar 

  • Gao Z, Shen W, Zhang H, Ge M, Niu X (2016) Application of Helmert variance component based adaptive Kalman filter in multi-GNSS PPP/INS tightly coupled integration. Remote Sens 8(7):553

    Article  Google Scholar 

  • Gao Z, Zhang H, Ge M, Niu X, Shen W, Wickert J, Schuh H (2017) Tightly coupled integration of multi-GNSS PPP and MEMS inertial measurement unit data. GPS Solut 21(2):377–391

    Article  Google Scholar 

  • Ge M, Gendt G, Rothacher MA, Shi C, Liu J (2008) Resolution of GPS carrier phase ambiguities in precise point positioning (PPP) with daily observations. J Geod 82(7):389–399

    Article  Google Scholar 

  • Gelb A (1974) Applied optimal estimation. The Massachusetts Institute of Technology Press, Cambridge

    Google Scholar 

  • Geng J, Meng X, Dodson AH, Ge M, Teferle FN (2010) Rapid re-convergences to ambiguity-fixed solutions in precise point positioning. J Geod 84(12):705–714

    Article  Google Scholar 

  • Godha S (2006) Performance evaluation of low cost MEMS-based IMU integrated with GPS for land vehicle navigation application. UCGE report, 20239

  • Han H, Wang J, Wang J, Tan X (2015) Performance analysis on carrier phase-based tightly-coupled GPS/BDS/INS integration in GNSS degraded and denied environments. Sensors 15(4):8685–8711

    Article  Google Scholar 

  • Héroux P, Kouba J (1995) GPS precise point positioning with a difference. Natural Resources Canada, Geomatics Canada, Geodetic Survey Division

  • Kaewunruen S, Remennikov AM (2010) Dynamic properties of railway track and its components: recent findings and future research direction. Insight Non Destr Test Cond Monit 52(1):20–22

    Article  Google Scholar 

  • Kouba J (2009) A guide to using international GNSS service (IGS) products. ftp://ww.igs.org/pub/resource/pubs/UsingIGSProductsVer21.pdf. Accessed 12 June 2017

  • Li X, Ge M, Zhang H, Wickert J (2013) A method for improving uncalibrated phase delay estimation and ambiguity-fixing in real-time precise point positioning. J Geod 87(5):405–416

    Article  Google Scholar 

  • Li T, Wang J, Laurichesse D (2014a) Modeling and quality control for reliable precise point positioning integer ambiguity resolution with GNSS modernization. GPS Solut 18(3):429–442

    Article  Google Scholar 

  • Li X, Ge M, Douša J, Wickert J (2014b) Real-time precise point positioning regional augmentation for large GPS reference networks. GPS Solut 18(1):61–71

    Article  Google Scholar 

  • Li Z, Wang J, Li B, Gao J, Tan X (2014c) GPS/INS/Odometer integrated system using fuzzy neural network for land vehicle navigation applications. J Navig 67(6):967–983

    Article  Google Scholar 

  • Liu CY, Lin CA, Chiang KW, Huang SC, Chang CC, Cai JM (2012) Performance evaluation of real-time MEMS INS/GPS integration with ZUPT/ZIHR/NHC for land navigation. In: 2012 12th international conference on ITS telecommunications (ITST), pp 584–588. IEEE

  • Luber B (2009) Railway track quality assessment method based on vehicle system identification. e & i Elektrotech Informationstech 126(5):180–185

    Article  Google Scholar 

  • Luck T, Lohnert E, Eissfeller B, Meinke P (2000) Track irregularity measurement using an INS-GPS integration technique. WIT Trans Built Environ 50. https://doi.org/10.2495/CR000101

  • Montenbruck O, Steigenberger P, Prange L, Deng Z, Zhao Q, Perosanz F, Schmid R (2017) The multi-GNSS experiment (MGEX) of the international GNSS service (IGS)—achievements, prospects and challenges. Adv Space Res 59(7):1671–1697

    Article  Google Scholar 

  • Mori H, Tsunashima H, Kojima T, Matsumoto A, Mizuma T (2010) Condition monitoring of railway track using in-service vehicle. J Mech Syst Transp Logist 3(1):154–165

    Article  Google Scholar 

  • Naganuma Y, Kobayashi M, Okumura T (2010) Inertial measurement processing techniques for track condition monitoring on shinkansen commercial trains. J Mech Syst Transp Logist 3(1):315–325

    Article  Google Scholar 

  • Prange L, Orliac E, Dach R, Arnold D, Beutler G, Schaer S, Jäggi A (2017) CODE’s five-system orbit and clock solution—the challenges of multi-GNSS data analysis. J Geod 91(4):345–360

    Article  Google Scholar 

  • Rabbou MA, El-Rabbany A (2015) Tightly coupled integration of GPS precise point positioning and MEMS-based inertial systems. GPS Solut 19(4):601–609

    Article  Google Scholar 

  • Roesler G, Martell H (2009) Tightly coupled processing of precise point position (PPP) and INS data. ION GNSS 2009 institute of navigation, Savannah, Georgia, USA, September 22–25, 2009, pp 1898–1905

  • Schaer S, Gurtner W, Feltens J (1998) IONEX: the ionosphere map exchange format version 1. In: Proceedings of the IGS AC workshop, vol 9, no 11, Darmstadt, Germany

  • Scherzinger BM (2000) Precise robust positioning with inertial/GPS RTK. In: Proceedings of the 13th international technical meeting for the satellite division of the institute of navigation (ION GPS), Salt Lake City, UT, USA, September 19–22, pp 115–162

  • Schmidt GT, Phillips RE (2010) INS/GPS integration architectures. Massachusetts Institute of Technology, Lexington

    Google Scholar 

  • Seo J, Lee HK, Lee JG, Park CG (2006) Lever arm compensation for GPS/INS/odometer integrated system. Int J Control Autom Syst 4(2):247–254

    Google Scholar 

  • Shin EH (2005) Estimation techniques for low-cost inertial navigation. University of Calgary Geomatics Engineering report, 20219

  • Shin EH, Scherzinger B (2001) Inertially aided precise point positioning. In: Proceedings of the 22nd international technical meeting of the satellite division of the institute of navigation (ION GNSS 2009), Savannah, GA, USA, September 22–25, pp 1892–1897

  • Tregoning P, Herring TA (2006) Impact of a priori zenith hydrostatic delay errors on GPS estimates of station heights and zenith total delays. Geophys Res Lett 33(23). https://doi.org/10.1029/2006GL027706

  • Tu R, Ge M, Zhang H, Huang G (2013) The realization and convergence analysis of combined PPP based on raw observation. Adv Space Res 52(1):211–221

    Article  Google Scholar 

  • Wang Y, Qin Y, Wei X (2012) Track irregularities estimation based on acceleration measurements. Int Conf Meas Inf Control 1:83–87. https://doi.org/10.1109/MIC.2012.6273305

    Google Scholar 

  • Weston PF, Ling CS, Goodman CJ, Roberts C, Li P, Goodall RM (2007) Monitoring lateral track irregularity from in-service railway vehicles. Proc Inst Mech Eng Part F J Rail Rapid Transit 221(1):89–100

    Article  Google Scholar 

  • Witchayangkoon B (2000) Elements of GPS precise point positioning (Doctoral dissertation, University of Maine)

  • Zhang XH, Li XX (2012) Instantaneous re-initialization in real-time kinematic PPP with cycle slip fixing. GPS Solut 16(3):315–327

    Article  Google Scholar 

  • Zhang H, Gao Z, Ge M, Niu X, Huang L, Tu R, Li X (2013) On the Convergence of Ionospheric Constrained Precise Point Positioning (IC-PPP) Based on Undifferential Uncombined Raw GNSS Observations. Sensors 13(11):15708–15725

    Article  Google Scholar 

  • Zumberge JF, Heflin MB, Jefferson DC, Watkins MM, Webb FH (1997) Precise point positioning for the efficient and robust analysis of GPS data from large networks. J Geophys Res Solid Earth (1978–2012) 102(B3):5005–5017

    Article  Google Scholar 

Download references

Acknowledgements

Many thanks to GNSS Research Center, Wuhan University, China for providing track irregularity experiment data and precise multi-GNSS orbit and clock products for this study. This work was supported partly by National Natural Science Foundation of China (No. 41631072), National 973 Project of China (Grant Nos. 2013CB733301 and 2013CB733305), and National Key Research and Development Program of China (Grant No. 2016YFB0501804).

Author information

Authors and Affiliations

  1. School of Land Science and Technology, China University of Geosciences Beijing, 29 Xueyuan Road, Beijing, 100083, China

    Zhouzheng Gao

  2. German Research Centre for Geosciences (GFZ), Telegrafenberg, 14473, Potsdam, Germany

    Zhouzheng Gao, Maorong Ge & Harald Schuh

  3. Department of Geomatics Engineering, University of Calgary, 2500 University Dr. N.W., Calgary, AB, Canada

    You Li

  4. School of Geodesy and Geomatics, Wuhan University, 129 Luoyu Road, Wuhan, 430079, China

    Wenbin Shen

  5. GNSS Research Center, Wuhan University, 129 Luoyu Road, Wuhan, 430079, China

    Hongping Zhang

Authors
  1. Zhouzheng Gao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Maorong Ge
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. You Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Wenbin Shen
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Hongping Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Harald Schuh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhouzheng Gao.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Gao, Z., Ge, M., Li, Y. et al. Railway irregularity measuring using Rauch–Tung–Striebel smoothed multi-sensors fusion system: quad-GNSS PPP, IMU, odometer, and track gauge. GPS Solut 22, 36 (2018). https://doi.org/10.1007/s10291-018-0702-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10291-018-0702-5

Keywords

Search

Navigation