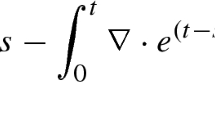On the continuity of the solutions to the Navier–Stokes equations with initial data in critical Besov spaces

Abstract

It is well known that there exists a unique local-in-time strong solution u of the initial boundary value problem for the Navier–Stokes system in a three-dimensional smooth bounded domain when the initial velocity $u_0$ belongs to critical Besov spaces. A typical space is $B=B^{-1+3/q}_{q,s}$ with $3<q<\infty$, $2<s<\infty$ satisfying $2/s+3/q \le 1$ or $B=\mathring{B}^{-1+3/q}_{q,\infty }$. In this paper, we show that the solution u is continuous in time up to initial time with values in B. Moreover, the solution map $u_0\mapsto u$ is locally Lipschitz from B to $C\left( [0,T];B\right)$. This implies that in the range $3<q<\infty$, $2<s\le \infty$ with $3/q +2/s \le 1$ the problem is well posed which is in strong contrast to norm inflation phenomena in the space $B^{-1}_{\infty ,s}$, $1\le s <\infty$ proved in the last years for the whole space case.

1 Introduction

We consider the initial boundary value problem of the Navier–Stokes equations in a bounded domain $\Omega \subset \mathbb {R}^3$ with $C^{2,1}$ boundary $\partial \Omega$,

$$\begin{aligned} u_t -\Delta u+u \cdot \nabla u + \nabla p = f,&\quad \mathop {\mathrm {div}}u=0 \quad \text {in}\quad (0,T)\times \Omega \nonumber \\ u \big |_{\partial \Omega } = 0,&\quad u(0)=u_0, \end{aligned}$$

(1.1)

where $T\in (0,\infty ]$. We are interested in local-in-time strong solutions in a Bochner space $L^s\left( 0,T;L^q(\Omega )\right)$ or, more generally, a weighted Bochner space with weight in time,

$$\begin{aligned} L^s_\alpha (L^q)&:= L^s_\alpha \left( 0,T; L^q(\Omega )\right) =\left\{ v\text { measurable in }(0,T)\times \Omega : \Vert v\Vert _{L^s_\alpha (L^q)}<\infty \right\} \end{aligned}$$

with

$$\begin{aligned} \Vert v\Vert _{L^s_\alpha (L^q)}:= \left( \int ^T_0\big (\tau ^\alpha \left\| v(\tau ) \right\| _q\big )^s \,\mathrm {d}\tau \right) ^{1/s}, \end{aligned}$$

where $\alpha \ge 0$ and $1\le s<\infty$; for $s=\infty$, the standard modification for the norm $\Vert \cdot \Vert _{L^\infty _\alpha (L^q)}$ is to be used. By definition, $L^s_0(L^q)=L^s(L^q)=L^s\left( 0,T; L^q(\Omega )\right)$.

There is a large literature on the existence of a local-in-time strong solution under various regularity condition on the initial data and the external force f [2, 13,14,15, 17, 18, 21, 25,26,27]. The first contribution in this direction seems to be the work of Kiselev and Ladyzhenskaya [19]. Since then, the condition on initial data and the external force f has been weakened; in other words, $u_0$ can be taken in a larger space.

In the scale of Besov spaces, it is shown in [11, 12] that a necessary and sufficient condition to get $L^s(L^q)$-strong solutions is that the initial data $u_0$ belong to a solenoidal Besov space $\mathbb {B}^{-1+3/q}_{q,s}(\Omega )$ provided that $s=s_q$ where $2/s_q+3/q=1\ (3<q<\infty )$. In this case, the so-called Serrin class $L^s(L^q)$ allows to prove regularity and uniqueness of weak solutions of the Navier–Stokes system. See also [7] for a review.

The existence of strong solutions is extended for s larger than $s_q$ by introducing a weighted Bochner space. In fact, in [8] a local-in-time strong solution in $L^s_\alpha (L^q)$ is constructed if the initial data belong to $\mathbb {B}^{-1+3/q}_{q,s}$ for $3<q<\infty$, $s_q\le s<\infty$ with $2/s_q+3/q=1$ and $2/s+3/q=1-2\alpha$. In [9], this result is extended to the case $s=\infty$ by replacing $\mathbb {B}^{-1+3/q}_{q,s}$ by $\mathring{\mathbb {B}}^{-1+3/q}_{q,\infty }$ which is obtained as a continuous interpolation space. In [8, 9], $u_0$ is assumed to belong also to the space $L^2_\sigma$ to compare with weak solutions. However, just for existence of a strong solution, this additional $L^2_\sigma$ assumption is unnecessary to get an $L^s_\alpha (L^q)$-solution. The explanation of the Besov spaces will be given in appendix for the reader’s convenience.

In this paper, we shall prove that $L^s_\alpha (L^q)$-solutions are indeed in $C\big ([0,T];{\mathbb {B}}^{-1+3/q}_{q,s}\big )$ for initial data $u_0\in {\mathbb {B}}^{-1+3/q}_{q,s}$ when $s_q\le s<\infty$, or in $C\big ([0,T];\mathring{{\mathbb {B}}}^{-1+3/q}_{q,\infty }\big )$ for $u_0\in \mathring{{\mathbb {B}}}^{-1+3/q}_{q,\infty }$ when $s=\infty$; see Theorems 1.1 and 1.2, respectively. Moreover, we will show in Theorems 1.3 ($s_q\le s<\infty )$ and 1.4 ($s=\infty$) that they are globally well posed for small initial data. Theorems 1.1 and 1.2 are in strong contrast to the so-called norm inflation phenomenon in limiting—homogeneous or inhomogeneous—Besov spaces for the corresponding Cauchy problem on ${\mathbb {R}}^n$, $n\ge 2$. Bourgain and Pavlovič [4] construct for any $\delta >0$ mild solutions with initial values $u_0$ in the Schwartz class such that $\Vert u_0\Vert _{\dot{B}^{-1}_{\infty ,\infty }}\le \delta$, but $\Vert u(t)\Vert _{\dot{B}^{-1}_{\infty ,\infty }}> 1/\delta$ for some $0<t<\delta$. Note that on the one hand, $\dot{B}^{-1}_{\infty ,\infty }$ is the largest scale-invariant Banach space of tempered distributions; see Meyer [24]. On the other hand, $BMO^{-1} \subset \dot{B}^{-1}_{\infty ,\infty }$ is the largest scale-invariant space for which global well-posedness for small initial data in $BMO^{-1}$ has been proved so far, cf. Koch and Tataru [20]. Yoneda [32] clarifies the approach in [4] and extends the result to $\dot{B}^{-1}_{\infty ,s}$, $s>2$, to be more precise, to a space V satisfying $\dot{B}^{-1}_{\infty ,2}\subset V \subset \dot{B}^{-1}_{\infty ,s}$. Wang [31] proves this norm inflation phenomenon even for all $1\le s<\infty$. Finally, Cheskidov and Shvidkoy [5] consider weak solutions of Leray–Hopf type such that $\limsup _{t\rightarrow 0}\Vert u(t)-u_0\Vert _{B^{-1}_{\infty ,\infty }} \ge \delta _0$ for some $\delta _0>0$ independent of $u_0$. Since $B^{-1+3/q}_{q,\infty }$, $1< q<\infty$, is continuously embedded into $B^{-1}_{\infty ,\infty }$ on ${\mathbb {R}}^3$, this result also yields the ill-posedness of weak solutions at $t=0$ measured in the space $B^{-1+3/q}_{q,\infty }$. This negative result underlines the importance of using the continuous interpolation space $\mathring{\mathbb {B}}^{-1+3/q}_{q,\infty }$ rather than $\mathbb {B}^{-1+3/q}_{q,\infty }$ in Theorem 1.2.

Theorem 1.1

Let $\Omega \subset \mathbb {R}^3$ be a bounded domain with $C^{2,1}$ boundary. Let $0<T\le \infty ,$$2<s<\infty ,\ 3<q<\infty$ and $0\le \alpha <1/2$ satisfy $2/s+3/q=1-2\alpha$. Moreover, let u be an $L^s_\alpha (L^q)$-strong solution with initial data $u_0\in \mathbb {B}^{-1+3/q}_{q,s}$ and $f=\mathop {\mathrm {div}}F$ satisfying $F\in L^{s/2}_{2\alpha }\left( 0,T;L^{q/2}(\Omega )\right)$. Then

$$\begin{aligned} u\in C\big ([0,T];\mathbb {B}^{-1+3/q}_{q,s}\big ). \end{aligned}$$

(1.2)

Theorem 1.2

($s=\infty$) Let $\Omega$, T, q be as in Theorem 1.1, and let $2\alpha =1-3/q$. Then an $L^\infty _\alpha (L^q)$-strong solution u with initial data $u_0 \in \mathring{\mathbb {B}}^{-1+3/q}_{q,\infty }$ and $f=\mathop {\mathrm {div}}F$ satisfying $F \in L^\infty _{2\alpha }\left( [0,T];L^{q/2}(\Omega )\right)$ and $\Vert F\Vert _{L^\infty _{2\alpha }(0,t;L^{q/2})}\rightarrow 0$ as $t\downarrow 0$ satisfies

$$\begin{aligned} u \in C\left( [0,T];\mathring{\mathbb {B}}^{-1+3/q}_{q,\infty }\right) . \end{aligned}$$

(1.3)

We further observe the continuity of solutions with respect to initial data and external forces.

Theorem 1.3

Under the assumptions of Theorem 1.1, let v be an $L^s_\alpha (L^q)$-strong solution with initial data $v_0 \in \mathbb {B}^{-1+3/q}_{q,s}$ and external force $G \in L^{s/2}_{2\alpha }\left( 0,T;L^{q/2}(\Omega )\right)$. Then there are constants $\varepsilon _*$ and C depending only on $\Omega$ such that if $T_0\le T$ is taken so that $\Vert u\Vert _{L^s_\alpha (0,T_0;L^q)}\le \varepsilon _*$, $\Vert v\Vert _{L^s_\alpha (0,T_0;L^q)}\le \varepsilon _*$, then for all $t\in (0,T_0)$

$$\begin{aligned} \Vert (u-v) (t)\Vert _{\mathbb {B}^{-1+3/q}_{q,s}} \le C \left( \Vert u_0-v_0 \Vert _{\mathbb {B}^{-1+3/q}_{q,s}} + \Vert F-G \Vert _{L^{s/2}_{2\alpha }(0,T_0;L^{q/2})}\right) . \end{aligned}$$

(1.4)

Theorem 1.4

($s=\infty$) Under the assumptions of Theorem 1.2, let v be an $L^\infty _\alpha (L^q)$-strong solution with initial data $v_0 \in \mathring{\mathbb {B}}^{-1+3/q}_{q,\infty }$ and external force $G \in L^\infty _{2\alpha }\big (0,T;L^{q/2}(\Omega )\big )$ such that $\Vert G\Vert _{L^\infty _{2\alpha }(0,t;L^{q/2})}\rightarrow 0$ as $t\downarrow 0$. Then there are constants $\varepsilon _*$ and C depending only on $\Omega$ such that if $T_0\le T$ is taken so that $\Vert u\Vert _{L^\infty _\alpha (0,T_0;L^q)}\le \varepsilon _*$, $\Vert v\Vert _{L^\infty _\alpha (0,T_0;L^q)}\le \varepsilon _*$, then

$$\begin{aligned} \Vert (u-v)(t) \Vert _{\mathbb {B}^{-1+3/q}_{q,\infty }} \le C \big ( \Vert u_0-v_0 \Vert _{\mathbb {B}^{-1+3/q}_{q,\infty }} + \Vert F-G \Vert _{L^\infty _{2\alpha }(0,T_0;L^{q/2})} \big ), \quad t \in (0,T_0).\nonumber \\ \end{aligned}$$

(1.5)

Theorems 1.1 and 1.2 are proved directly avoiding deep tools from interpolation theory and interpolation–extrapolation scales. The terminology of Besov spaces is only used in the statements of the theorems and in appendix where it is shown that Besov spaces are behind the norm estimates in Sects. 2, 3 and 4; see (1.6). This context is well known from the optimal spaces of initial values for a classical parabolic equation like the heat equation where $u_0\in B^{1+3/q}_{q,s_q}=B^{2-2/s_q}_{q,s_q}=(L^q,{\mathcal {D}}(\Delta ))_{1-1/s_q,s_q}$ allows for a strong solution u in the maximal regularity class $L^{s_q}(0,\infty ;{\mathcal {D}}(\Delta ))$ over $L^q$. In this article, working in scale-invariant function spaces close to Serrin’s class $L^{s_q}(0,T;L^q_\sigma )$, the initial values are chosen from Besov spaces of solenoidal vector fields, $\mathbb {B}^{-1+3/q}_{q,s}$ and $\mathring{\mathbb {B}}^{-1+3/q}_{q,\infty }$.

Let us recall the Helmholtz projection ${\mathbb {P}}_q: L^q(\Omega )\rightarrow L^q_\sigma (\Omega )$ and the Stokes operator $A=A_q=-{\mathbb {P}}_q \Delta$ in $L^q_\sigma (\Omega )$, the closure of $C^\infty _{c,\sigma }(\Omega )$ in $L^q(\Omega )$; here, $C^\infty _{c,\sigma }(\Omega )$ denotes the space of smooth solenoidal vector fields with compact support. The semigroup generated by $-A_q$ is denoted by $e^{-tA_q}$ and defines the solution operator $u_0\mapsto u(t)$ for the Stokes equations in case that $f=0$. Then, if $2/s+3/q = 1-2\alpha$,

$$\begin{aligned} u_0 \in {\mathbb {B}}^{-1+3/q}_{q,s}\quad \text { iff } \quad \int ^T_0 \left( \tau ^\alpha \big \Vert e^{-\tau A}u_0\big \Vert _q \right) ^s \,\mathrm {d}\tau <\infty \end{aligned}$$

(1.6)

with the usual modification if $s=\infty$; for more details, see Appendix in Sect. 5. The results on continuity and well-posedness hold for a (mild) solution $u\in L^s_\alpha (L^q)$ of the corresponding integral equation

$$\begin{aligned} u(t) = e^{-tA}u_0 -\int ^t_0 e^{-(t-\tau )A} \left( \mathbb {P}\mathop {\mathrm {div}}(u \otimes u)-\mathbb {P}\mathop {\mathrm {div}}F \right) (\tau ) \,\mathrm {d}\tau . \end{aligned}$$

(1.7)

In Sect. 2, we prepare abstract lemmata for Theorems 1.1–1.4 to be proved in Sect. 4. The essential technical estimates will be performed in Sect. 3. In appendix, the abstract interpolation spaces introduced in Sect. 2 are identified with solenoidal Besov spaces; these results are taken from papers by Amann [2, 3] and his monograph [1].

Note that $L^s_\alpha (L^q)$-strong solutions in [9] are defined as the subset of classical weak solutions of Leray–Hopf type in which $u\in L^s_\alpha (L^q)$. Finally, related results can be found in articles by Amann [2] and Haak and Kunstmann [16] as special cases of a more general abstract theory using interpolation–extrapolation scales of Banach spaces; see Remark 4.1 for more details.

2 Abstract spaces

Let X be a Banach space equipped with the norm $\Vert \cdot \Vert _X$, and let $-A$ denote the generator of a $C_0$-analytic semigroup $e^{-tA}$ in X. Assume that $\left\{ z\in \mathbb {C}: {\text {Re}}z\ge 0 \right\}$ is included in the resolvent set of A. Then $A^{-1}: X\rightarrow {\mathcal {D}}(A)$ is bounded and $A:{\mathcal {D}}(A) \rightarrow X$ is an isometry when ${\mathcal {D}}(A)$ is equipped with the homogeneous graph norm $\Vert A\cdot \Vert _X$. Moreover, the semigroup $e^{-tA}$ decays exponentially in time, i.e.$\left\| e^{-tA}\right\| _{{\text {op}}(X)}\le C_0 e^{-\nu t}$ with some positive constants $C_0$ and $\nu$; here, $\Vert \cdot \Vert _{{\text {op}}(X)}$ denotes the operator norm on X.

Under these assumptions, we define the extrapolation space $Z=X_{-1}$ with norm $\Vert z\Vert _Z = \Vert A^{-1}z\Vert _X$ as the completion $\overline{\big (X,\Vert \cdot \Vert _Z\big )}$. Then $A_{-1}$, defined as the closure of A in $X_{-1}$, is the unique continuous extension of the isometry $A: {\mathcal {D}}(A) \rightarrow X$ and yields an isometry $A_{-1}: X={\mathcal {D}}(A_{-1})\subset X_{-1} \rightarrow X_{-1}$. The semigroup operators $e^{-tA}$ possess continuous extensions from X to $X_{-1}$ defining an exponentially decaying analytic semigroup with infinitesimal generator $A_{-1}$; see Proposition 2.1. For simplicity, we will denote this semigroup by $e^{-tA}$ again. For details, we refer to [1, Chapter V, p. 262], [6, Chapter II.5]. If X is reflexive, then Z is isomorphic to $\big ({\mathcal {D}}(A')\big )'$; see [1, Theorem V.1.4.6] or [6, Chapter II, Exercise 5.9(4)].

Hence, with an abuse of notation, we will write

$$\begin{aligned} A: X\rightarrow Z=AX = \big ({\mathcal {D}}(A')\big )' \end{aligned}$$

defining the isometry $\Vert Ax\Vert _Z = \Vert x\Vert _X$ for $x\in X$.

For $1\le s \le \infty$ and $\alpha \in \mathbb {R}$ such that $0<\alpha +\frac{1}{s}<1$, we define for $f\in Z$ the norm

$$\begin{aligned} \Vert f\Vert _{{\mathcal {X}}_{s,\alpha }} := \Vert A^{-1}f\Vert _X + {\left\{ \begin{array}{ll} \displaystyle \left( \int ^\infty _0 \tau ^{\alpha s} \big \Vert e^{-\tau A}f\big \Vert ^s_X \,\mathrm {d}\tau \right) ^{1/s} &{} \text { when }\;s<\infty , \\ \sup _{0<\tau <\infty } \tau ^\alpha \left\| e^{-\tau A}f\right\| _X &{} \text { when }\;s=\infty , \end{array}\right. } \end{aligned}$$

(2.1)

and the space

$$\begin{aligned} {\mathcal {X}}_{s,\alpha } = \{f\in Z: \Vert f\Vert _{s,\alpha } < \infty \}. \end{aligned}$$

We note that the term $\Vert A^{-1}f\Vert _X$ in (2.1) can be omitted. The idea well known from interpolation theory uses the identity $f = -\int _0^\infty \frac{\mathrm{d}}{\,\mathrm {d}\tau } e^{-\tau A}f \,\mathrm {d}\tau = \int _0^\infty Ae^{-\tau A}f\,\mathrm {d}\tau$ in Z which holds if $\Vert Ae^{-\tau A}f\Vert _Z = \Vert e^{-\tau A}f\Vert _X$ is integrable on $(0,\infty )$. To control $\int _0^\infty \Vert e^{-\tau A}f\Vert _X\,\mathrm {d}\tau$, we use Hölder’s inequality and $\alpha s'<1$ to see that

$$\begin{aligned} \int _0^1 \Vert e^{-\tau A}f\Vert _X \,\mathrm {d}\tau \le C \left( \int _0^1 \tau ^{\alpha s}\, \Vert e^{-\tau A}f\Vert _X^s \,\mathrm {d}\tau \right) ^{1/s}{;} \end{aligned}$$

moreover, by the exponential decay of $e^{-\tau A}$ an estimate similar to the above one holds also on $(1,\infty )$. Using the exponential decay of $e^{-\tau A}$ again and an argument by geometric series, we obtain that—for any $0<T<\infty$—the norm in (2.1) can be replaced by the equivalent norm

$$\begin{aligned} \Vert f\Vert _{{\mathcal {X}}_{s,\alpha }^T} = {\left\{ \begin{array}{ll} \displaystyle \left( \int ^T_0 \tau ^{\alpha s} \big \Vert e^{-\tau A}f\big \Vert ^s_X \,\mathrm {d}\tau \right) ^{1/s} &{} \text { when }\;s<\infty , \\ \sup _{0<\tau <T} \tau ^\alpha \left\| e^{-\tau A}f\right\| _X &{} \text { when }\;s=\infty . \end{array}\right. } \end{aligned}$$

(2.2)

To indicate which equivalent norm is used in ${\mathcal {X}}_{s,\alpha }$, we also use the notation ${\mathcal {X}}_{s,\alpha }^T$ instead of ${\mathcal {X}}_{s,\alpha }$ when ${\mathcal {X}}_{s,\alpha }$ is equipped with the norm $\Vert \cdot \Vert _{{\mathcal {X}}_{s,\alpha }^T}$.

From real interpolation theory applied to the spaces Z and $X={\mathcal {D}}(A_{-1})$, for example, see [23, Proposition 6.2], we conclude that ${\mathcal {X}}_{s,\alpha }^T = {\mathcal {X}}_{s,\alpha }$ also coincides with the real interpolation space $(Z,X)_{1-\alpha -1/s,s}$ endowed with the equivalent norm

$$\begin{aligned} \Vert f\Vert _{(Z,X)_{1-\alpha -1/s,s}} = \left( \int _0^\infty \big (\tau ^{\alpha +1/s} \Vert Ae^{-\tau A}f\Vert _Z\big )^s \frac{\,\mathrm {d}\tau }{\tau } \right) ^{1/s}, \end{aligned}$$

(2.3)

where the term $\Vert A^{-1}f\Vert _X$ is omitted. In the limit case when $s=\infty$, [23, Exercise 6.1.1 (1)] implies that ${\mathcal {X}}_{\infty ,\alpha }^T = (Z,X)_{1-\alpha ,\infty }$ for all $0<T\le \infty$ with equivalent norms. Thus, for fixed $\theta =1-\alpha -\frac{1}{s} \in (0,1)$, i.e.$\alpha =\alpha (s)=1-\theta -\frac{1}{s}\in [0,1-\theta ]$, we get the scale of interpolation spaces $(Z,X)_{\theta ,s}$ for $\frac{1}{1-\theta } =:s_1\le s\le \infty$ for all $0<T\le \infty$ and with continuous embeddings

$$\begin{aligned} X \subset (Z, X)_{\theta ,s_1} \subset (Z, X)_{\theta ,s} \subset (Z, X)_{\theta ,\infty } \subset Z, \end{aligned}$$

(2.4)

or, since $(Z, X)_{\theta ,s}={\mathcal {X}}_{s,\alpha (s)}^T$, the scale $X \subset {\mathcal {X}}_{s_1,\alpha (s_1)}^T \subset {\mathcal {X}}_{s,\alpha (s)}^T \subset {\mathcal {X}}_{\infty ,\alpha (\infty )}^T \subset Z$.

Proposition 2.1

• (i) For $t>0$ and $f\in Z$, we have that $e^{-tA} f\in Z$ such that

  $$\begin{aligned} \Vert e^{-tA} f\Vert _Z \le \Vert e^{-tA}\Vert _{{\text {op}}(X)} \Vert f\Vert _Z. \end{aligned}$$

  Moreover, $e^{-tA}$ extends to a bounded linear operator from Z to X. To be more precise, there exists a constant $c>0$ independent of t and $f\in Z$ such that

  $$\begin{aligned} \Vert e^{-tA}f\Vert _X \le c t^{-1}\Vert f\Vert _Z, \quad t>0. \end{aligned}$$

• (ii) The space X is continuously embedded into ${\mathcal {X}}_{s,\alpha }^T$ for all $\alpha \ge 0$, $1\le s\le \infty$ and $0<T\le \infty$.

Proof

(i) By analyticity, we observe that for $f\in Z=X_{-1}$ and for $t>0$

$$\begin{aligned} \Vert e^{-t A}f\Vert _Z&= \Vert A_{-1}^{-1} e^{-t A}f\Vert _X = \Vert e^{-t A}A_{-1}^{-1}f\Vert _X \\&\le \Vert e^{-tA}\Vert _{{\text {op}}(X)} \Vert A^{-1}f\Vert _X \le \Vert e^{-tA}\Vert _{{\text {op}}(X)} \Vert f\Vert _Z. \end{aligned}$$

If $f=A_{-1}x\in Z$ with $x\in X$, then

$$\begin{aligned} \Vert e^{-t A}f\Vert _X = \Vert A_{-1} e^{-t A}x \Vert _X \le ct^{-1}\Vert x\Vert _X = ct^{-1}\Vert f\Vert _Z, \end{aligned}$$

with some constant c independent of f and t.

(ii) It is well known from real interpolation theory since $X\subset Z$; see also (2.4). $\square$

Besides the spaces ${\mathcal {X}}_{\infty ,\alpha }$, we also need the closed subspace $\mathring{{\mathcal {X}}}_{\infty ,\alpha }$ defined by

$$\begin{aligned} \mathring{{\mathcal {X}}}_{\infty ,\alpha } = \left\{ f\in {\mathcal {X}}_{\infty ,\alpha } : \sup _{0<\tau <\tau _0}\tau ^\alpha \big \Vert e^{-\tau A}f \big \Vert _X \rightarrow 0 \quad \text {as}\;\; \tau _0\rightarrow 0 \right\} . \end{aligned}$$

By [23, Exercise 6.1.1 (1)], $\mathring{{\mathcal {X}}}_{\infty ,\alpha }$ coincides with the continuous interpolation space $(Z,X)^0_{1-\alpha ,\infty }$ with equivalent norms. Thus, obviously $X\subset \mathring{{\mathcal {X}}}_{\infty ,\alpha } \subset {\mathcal {X}}_{\infty ,\alpha } \subset Z$.

In view of (2.1), (2.2), we often suppress the T-dependence of ${\mathcal {X}}_{s,\alpha }^T$ and assume that $0<T<\infty$ is fixed. The notation ${\mathcal {X}}_{s,\alpha }^T$ is important in the construction of strong $L^s_\alpha (L^q)$-solution where T yields a control of the interval of existence; see [8, 9] and [11, 12].

3 Estimates of continuity

In the following, we fix $0<T<\infty$ and simply write ${\mathcal {X}}_{s,\alpha }$ for ${\mathcal {X}}_{s,\alpha }^T$. The first continuity result considers the homogeneous part $e^{-tA}u_0$ in (1.7) and can be proved by general interpolation theory since by (2.2), (2.4) ${\mathcal {X}}_{s,\alpha }=A\left( X,{\mathcal {D}}(A)\right) _{1-\alpha -\frac{1}{s},s} = (Z,X)_{1-\alpha -\frac{1}{s},s}$. However, we present a direct proof for completeness.

Proposition 3.1

Let $s\in [1,\infty ]$ and $\alpha \ge 0$. Assume that $u_0 \in {\mathcal {X}}_{s,\alpha }$.

• (i) For $t\in (0,T]$, the estimate

  $$\begin{aligned} \big \Vert e^{-tA}u_0 \big \Vert _{{\mathcal {X}}_{s,\alpha }^T} \le C_T \Vert u_0\Vert _{{\mathcal {X}}_{s,\alpha }^T} \end{aligned}$$

  holds with the constant $C_T = \sup _{t\in (0,T)} \Vert e^{-tA}\Vert _{{\text {op}}(X)}$.

• (ii) $e^{-tA}u_0 \in C \big ( [0,\infty );{\mathcal {X}}_{s,\alpha } \big )$ if $u_0\in {\mathcal {X}}_{s,\alpha }$ and $s<\infty$.

• (iii) $e^{-tA}u_0 \in C \big ( [0,\infty );\mathring{{\mathcal {X}}}_{\infty ,\alpha } \big )$ if $u_0\in \mathring{{\mathcal {X}}}_{\infty ,\alpha }$.

• (iv) For $u_0\in {\mathcal {X}}_{\infty ,\alpha }$, continuity holds except at $t=0$, i.e. $e^{-tA} \in C \big ( (0,\infty );{\mathring{{\mathcal {X}}}}_{\infty ,\alpha }\big )$. Moreover, $e^{-tA}u_0{\mathop {\rightharpoonup }\limits ^{*}} u_0$ as $t\rightarrow 0$ in ${\mathcal {X}}_{\infty ,\alpha }$; for the latter result X is assumed to be reflexive.

To prove Proposition 3.1, we use the strong continuity of the semigroup $e^{-tA}$ on X and on ${\mathcal {D}}(A)$ near $t=0$.

Lemma 3.2

• (i) $\left\| \left( e^{-tA}-I \right) f\right\| _X \le c_{\beta ,T} t^\beta \left\| A^\beta f \right\| _X$ for each $\beta \in (0,1]$, $t\in (0,T)$ and $f\in {\mathcal {D}}(A^\beta )$ with a constant $c_{\beta ,T}>0$ independent of f and $t>0$.

• (ii) $\displaystyle \big \Vert \big (e^{-tA}-I \big )e^{-\tau A}f\big \Vert _X \le c_{\beta ,T}\left( \frac{t}{\tau }\right) ^\beta \Vert f\Vert _X$ for each $\beta \in (0,1]$, $t\in (0,T)$ and $f\in X$ with $c_{\beta ,T}$ independent of t, $\tau$ and f.

Proof of Lemma 3.2

(i) By the fundamental theorem of calculus,

$$\begin{aligned} e^{-tA}f-f = -\int ^t_0 Ae^{-\tau A}f\;\,\mathrm {d}\tau = -\int ^t_0 A^{1-\beta }e^{-\tau A}A^\beta f\;\,\mathrm {d}\tau . \end{aligned}$$

Since $\left\| A^\lambda e^{-tA}\right\| _{{\text {op}}(X)} \le c_\lambda \tau ^{-\lambda }\ (\lambda >0)$ by analyticity, we observe that

$$\begin{aligned} \big \Vert e^{-tA}f-f\big \Vert _X \le c_{1-\beta } \int ^t_0 \frac{\,\mathrm {d}\tau }{\tau ^{1-\beta }} \left\| A^\beta f\right\| _X = c_\beta ' t^\beta \left\| A^\beta f\right\| _X. \end{aligned}$$

(ii) This follows from (i) since $\left\| A^\beta e^{-\tau A}\right\| _{{\text {op}}(X)} \le c_\beta \tau ^{-\beta }$. $\square$

Proof of Proposition 3.1

(i) This estimate is easy; for example, for $s<\infty$ we have

$$\begin{aligned} \big \Vert e^{-tA}u_0\big \Vert ^s_{{\mathcal {X}}_{s,\alpha }^T} = \int ^{T}_0 \tau ^{\alpha s} \big \Vert e^{-(\tau +t) A}u_0 \big \Vert ^s_X \,\mathrm {d}\tau \le C_T^s \Vert u_0\Vert ^s_{{\mathcal {X}}_{s,\alpha }^T}. \end{aligned}$$

(ii) Let $t_0,t\ge 0$. Then

$$\begin{aligned} \big \Vert e^{-tA}u_0 - e^{-t_0A} u_0\big \Vert ^s_{{\mathcal {X}}_{s,\alpha }^T} = \int ^T_0 \tau ^{\alpha s} \big \Vert \left( e^{-tA} - e^{-t_0A}\right) e^{-\tau A}u_0\big \Vert ^s_X\,\mathrm {d}\tau \end{aligned}$$

converges to 0 as $t\rightarrow t_0$ by Lebesgue’s theorem on dominated convergence since the integrand is uniformly estimated by an integrable function in (0, T) and converges to 0 in the pointwise sense. This proves the continuity of $e^{-tA}u_0$ in $[0,\infty )$ with values in ${\mathcal {X}}_{s,\alpha }$.

(iii) Let $t,t_0 \ge 0$. We take $\delta \in (0,T)$ and divide the supremum into two parts:

$$\begin{aligned} \big \Vert e^{-tA}u_0-e^{-t_0A}u_0\big \Vert _{{\mathcal {X}}_{\infty ,\alpha }^T} \!\le \!\left( \sup _{\delta<\tau<T} \!+\! \sup _{0<\tau <\delta }\right) \tau ^\alpha \big \Vert \left( e^{-tA}\!-\!e^{-t_0A}\right) e^{-\tau A}u_0\big \Vert _X =: J_1+J_2. \end{aligned}$$

Similarly to the case $s<\infty$, we observe that $J_1\rightarrow 0$ as $t\rightarrow t_0$. The second term is estimated as

$$\begin{aligned} J_2 \le 2C_0 \sup _{0<\tau <\delta } \tau ^\alpha \big \Vert e^{-\tau A}u_0 \big \Vert _X. \end{aligned}$$

If $u_0 \in \mathring{{\mathcal {X}}}_{\infty ,\alpha }$, the right-hand side (which is independent of t, $t_0$) tends to zero as $\delta \rightarrow 0$. Thus, we conclude the continuity of $e^{-tA}u_0$ up to $t=0$ with values in $\mathring{{\mathcal {X}}}_{\infty ,\alpha }$.

(iv) If $u_0\in {\mathcal {X}}_{\infty ,\alpha }$, the function $e^{-tA}u_0$ may not be continuous at $t=0$ with values in ${\mathcal {X}}_{\infty ,\alpha }$. However, since $e^{-tA}u_0\in X$ by Proposition 2.1 for $t>0$ and $X\subset \mathring{{\mathcal {X}}}_{s,\alpha }$, the assertion $e^{-tA}u_0\in C\big ((0,\infty );\mathring{{\mathcal {X}}}_{s,\alpha }\big )$ holds.

For the analysis at $t=0$, we apply the duality theorem of real interpolation, see [30, Theorem 1.11.2], and consider ${\mathcal {X}}_{\infty ,\alpha } = (Z,X)_{1-\alpha ,\infty }$ as the dual space of $(Z',X')_{1-\alpha ,1} = (X',{\mathcal {D}}(A'))_{\alpha ,1}$ with the weighted norm $\int _0^T \tau ^{-\alpha } \Vert A'e^{-\tau A'} \varphi \Vert _{X'}\,\mathrm {d}\tau$ for $\varphi \in (X',{\mathcal {D}}(A'))_{\alpha ,1}$, cf. (2.3). Given $\varphi$ we get that

$$\begin{aligned} |\langle e^{-tA}u_0-u_0,\varphi \rangle |&= |\langle u_0,e^{-tA'}\varphi -\varphi \rangle | \\&\le \Vert u_0\Vert _{{\mathcal {X}}_{\infty ,\alpha }} \Vert e^{-tA'}\varphi -\varphi \Vert _{(X',{\mathcal {D}}(A'))_{\alpha ,1}}. \end{aligned}$$

To show that the latter term converges to 0 as $t\rightarrow 0$ we note that part (ii) of this proposition holds also for negative $\alpha$ as is easily seen. $\square$

To estimate nonlinear terms as on the right-hand side of (1.7), we consider for $\mu >0$ the integral operator

$$\begin{aligned} (Nf)(t) = \int ^t_0 A^\mu e^{-(t-\tau )A} f(\tau )\,\mathrm {d}\tau \end{aligned}$$

(3.1)

for $f\in L^{s_1}_{\alpha _1}(0,T;Y)$. Here Y is another Banach space containing X and $e^{-tA}$ can be extended to Y having a regularizing estimate

$$\begin{aligned} \big \Vert e^{-tA} a\big \Vert _X \le c_T t^{-\eta } \Vert a\Vert _Y, \quad a\in Y, \quad t\in (0,T) \end{aligned}$$

(3.2)

for some $\eta >0$ with $c_T$ independent of a.

We recall the weighted Hardy–Littlewood–Sobolev inequality [28, 29]. The limit cases $s_1,s_2 \in \{1,\infty \}$ are considered in [29, Theorem 5].

Lemma 3.3

Assume that $\lambda \in (0,1)$ satisfies the scale balance of exponents $1/s_1+\alpha _1+\lambda = 1/s_2+\alpha _2 +1$ under the restrictions of exponents $1\le s_1\le s_2\le \infty$, $\alpha _2\le \alpha _1$ and $0<\alpha _1+1/s_1<1$, $0<\alpha _2+1/s_2<1$. Then the integral operator

$$\begin{aligned} (I_\lambda f)(t) = \int _{\mathbb {R}} |t-\tau |^{-\lambda } f(\tau ) \,\mathrm {d}\tau \end{aligned}$$

is bounded from $L^{s_1}_{\alpha _1}(\mathbb {R})$ to $L^{s_2}_{\alpha _2}(\mathbb {R})$. If $\alpha _2=\alpha _1$, then the stricter condition $1<s_1,s_2<\infty$ is needed.

By $\left\| A^\mu e^{-tA}\right\| _{{\text {op}}(X)}\le Ct^{-\mu }$ and (3.2),

$$\begin{aligned} \left\| (Nf)(t)\right\| _X \le C\int ^t_0 (t-\tau )^{-\mu -\eta } \left\| f(\tau )\right\| _Y \,\mathrm {d}\tau , \end{aligned}$$

(3.3)

so that Lemma 3.3 yields the following:

Proposition 3.4

Assume that $\lambda =\mu +\eta \in (0,1)$ for positive $\mu ,\eta$ as in (3.1), (3.2). Then N defined by (3.1) is a bounded operator from $L^{s_1}_{\alpha _1}(0,T;Y)$ to $L^{s_2}_{\alpha _2}(0,T;X)$. Here the exponents $s_j,\alpha _j$ satisfy the assumptions in Lemma 3.3, and the operator norm of N is independent of T.

We claim that $Nf(\cdot )$ belongs to $C\left( [0,T];{\mathcal {X}}_{s_2,\alpha _2}\right)$ and start with the case $s_2<\infty$.

Theorem 3.5

Assume that $\lambda =\mu +\eta \in (0,1)$ for positive $\mu ,\eta$ as in (3.1), (3.2) satisfies the scale balance $1/s_1+\alpha _1+\lambda = 1/s_2+\alpha _2+1$ for exponents $1<s_1\le s_2<\infty$, $\alpha _2\le \alpha _1$ where $0<\alpha _1+1/s_1<1$, $0<\alpha _2+1/s_2<1$. If $f\in L^{s_1}_{\alpha _1}(0,T;Y)$, then

$$\begin{aligned} \Vert Nf(t)\Vert _{{\mathcal {X}}_{s_2,\alpha _2}^T} \le C\Vert f\Vert _{L^{s_1}_{\alpha _1}(0,t;Y)},\quad t\in [0,T]. \end{aligned}$$

(3.4)

Moreover,

$$\begin{aligned} Nf\in C\left( [0,T]; {\mathcal {X}}_{s_2,\alpha _2} \right) . \end{aligned}$$

Proof

By definition, we get from (3.3) that

$$\begin{aligned} \Vert Nf(t)\Vert _{{\mathcal {X}}_{s_2,\alpha _2}^T}&= \left( \int ^T_0 \tau ^{\alpha _2 s_2} \big \Vert e^{-\tau A} (Nf)(t) \big \Vert ^{s_2}_X \,\mathrm {d}\tau \right) ^{1/s_2} \nonumber \\&\le C \left( \left\| \int ^t_0 (t+\tau -\rho )^{-\mu -\eta } \left\| f(\rho )\right\| _Y \,\mathrm {d}\rho \right\| _{L^{s_2}_{\alpha _2}(0,T)}^{s_2} \right) ^{1/s_2} \nonumber \\&= C\left( \int _0^T \left( \tau ^{\alpha _2} \int _{{\mathbb {R}}} |t+\tau -\rho |^{-\lambda } \Vert (f\chi )(\rho )\Vert _Y \,\mathrm {d}\rho \right) ^{s_2} \,\mathrm {d}\tau \right) ^{1/s_2} \end{aligned}$$

(3.5)

with $\chi =\chi _{(0,t)}$, the characteristic function of the interval (0, t). Using the change of variables $\tau '=\tau +t$ and that $0\le \tau '-t\le \tau '$ Lemma 3.3 implies that

$$\begin{aligned} \Vert Nf(t)\Vert _{{\mathcal {X}}_{s_2,\alpha _2}^T}&\le C \left( \int _t^{t+T} \left( (\tau '-t)^{\alpha _2} \int _{{\mathbb {R}}} |\tau '-\rho |^{-\lambda } \Vert (f\chi )(\rho )\Vert _Y \,\mathrm {d}\rho \right) ^{s_2} \,\mathrm {d}\tau ' \right) ^{1/s_2} \\&\le C\big \Vert I_\lambda ( \Vert f\chi \Vert _Y) \big \Vert _{L^{s_2}_{\alpha _2}(t,t+T)}\\&\le C \big \Vert \,\Vert f\chi \Vert _Y\big \Vert _{L^{s_1}_{\alpha _1}} = C\Vert f\Vert _{L^{s_1}_{\alpha _1}(0,t;Y)}. \end{aligned}$$

The proof of continuity is based on the previous estimates. By definition for $t_1\ge t_2\ge 0$, we observe that

$$\begin{aligned}&(Nf)(t_1)-(Nf)(t_2) \\&= \int ^{t_1}_{t_2} A^\mu e^{-(t_1-\rho )A} f(\rho )\,\mathrm {d}\rho + \int ^{t_2}_0 \left( A^\mu e^{-(t_1-\rho )A} -A^\mu e^{-(t_2-\rho )A} \right) f(\rho )\,\mathrm {d}\rho \\&=: {\mathcal {I}}_1 + {\mathcal {I}}_2. \end{aligned}$$

The first term is easy to estimate. Replacing f by $f\chi _{(t_2,t_1)}$ and rewriting ${\mathcal {I}}_1$ as an integral for $f\chi _{(t_2,t_1)}(\rho )$ with $\rho \in (0,t_1)$, (3.4) proves that

$$\begin{aligned} \Vert {\mathcal {I}}_1\Vert _{{\mathcal {X}}_{s_2,\alpha _2}^T}&\le C\left\| \int ^{t_1}_{0} (t_1+\tau -\rho )^{-\mu -\eta } \Vert f(\rho )\chi _{(t_2,t_1)}(\rho )\Vert _Y \,\mathrm {d}\rho \right\| _{L^{s_2}_{\alpha _2}(0,T)} \\&\le C\Vert f\Vert _{L^{s_1}_{\alpha _1}(t_2,t_1;Y)}\rightarrow 0 \end{aligned}$$

as $t_1-t_2\rightarrow 0$. The integral ${\mathcal {I}}_2$ is divided into two parts:

$$\begin{aligned} \Vert {\mathcal {I}}_2\Vert ^{s_2}_{{\mathcal {X}}_{s_2,\alpha _2}^T} = \int ^T_0 \tau ^{\alpha _2 s_2} \big \Vert e^{-\tau A}{\mathcal {I}}_2 \big \Vert ^{s_2}_X \,\mathrm {d}\tau =\left( \int ^\delta _0 + \int ^T_\delta \right) \tau ^{\alpha _2 s_2} \big \Vert e^{-\tau A}{\mathcal {I}}_2 \big \Vert ^{s_2}_X \,\mathrm {d}\tau . \end{aligned}$$

The first part is estimated as follows: for $t_{1,2}=t_1$ and $t_{1,2}=t_2$,

$$\begin{aligned} C\int ^\delta _0&\tau ^{\alpha _2 s_2} \left\| \int _0^{t_2} A^\mu e^{-(t_{1,2}+\tau -\rho )A } f(\rho ) \,\mathrm {d}\rho \right\| _X^{s_2} \,\mathrm {d}\tau \\&\le C\int ^\delta _0 \tau ^{\alpha _2 s_2}\Big (\int ^{t_2}_0 (t_2+\tau - \rho )^{-\lambda } \Vert f(\rho )\Vert _Y \,\mathrm {d}\rho \Big ) ^{s_2} \,\mathrm {d}\tau ; \end{aligned}$$

for $t_1$, we used that $(t_1+\tau -\rho )^{-\lambda }\le (t_2+\tau -\rho )^{-\lambda }$ since $t_1\ge t_2$. Replacing $\delta$ by T, we conclude—as for the estimate of $\Vert {\mathcal {I}}_1\Vert _{{\mathcal {X}}_{s_2,\alpha _2}^T}$—from Lemma 3.3 that the right-hand double integral is bounded by $C\Vert f\Vert ^{s_2}_{L^{s_1}_{\alpha _1}(0,t_2;Y)}$. Hence, as a function of $\delta$, the right-hand side converges to 0 as $\delta \rightarrow 0$, uniformly in $0\le t_2\le t_1\le T$.

To estimate the integral over $(\delta ,T)$ in $\Vert {\mathcal {I}}_2\Vert ^{s_2}_{{\mathcal {X}}_{s_2,\alpha _2}^T}$, we observe that

$$\begin{aligned} \int ^T_\delta \tau ^{\alpha _2 s_2} \big \Vert e^{-\tau A} {\mathcal {I}}_2 \big \Vert ^{s_2}_X \,\mathrm {d}\tau = \int ^T_\delta \tau ^{\alpha _2 s_2} \varphi (\tau ,t_1,t_2)\,\mathrm {d}\tau , \end{aligned}$$

where by Lemma 3.2 (ii) for any $\nu _1\in (0,1)$

$$\begin{aligned} \varphi (\tau ,t_1,t_2)&= \left\| \int ^{t_2}_0 \left( e^{-(t_1-t_2)A}-I \right) e^{-\tau A} A^\mu e^{-(t_2-\rho )A} f(\rho )\,\mathrm {d}\rho \right\| _X^{s_2} \\&\le C \left| \frac{t_2-t_1}{\tau } \right| ^{\nu _1 s_2} \left( \int ^{t_2}_0 \left\| e^{-\tau A/2} A^\mu e^{-(t_2-\rho )A} f(\rho )\right\| _X \,\mathrm {d}\rho \right) ^{s_2}. \end{aligned}$$

Thus,

$$\begin{aligned} \int ^T_\delta&\tau ^{\alpha _2 s_2} \varphi (\tau ,t_1,t_2)\,\mathrm {d}\tau \\&\le C \left| \frac{t_2-t_1}{\delta } \right| ^{\nu _1 s_2} \int ^T_0 \left( \int ^{t_2}_0 \big (t_2+\frac{\tau }{2} - \rho \big )^{-\lambda } \Vert f(\rho )\Vert _Y \,\mathrm {d}\rho \right) ^{s_2}\,\mathrm {d}\tau \\&\le C \left| \frac{t_2-t_1}{\delta } \right| ^{\nu _1 s_2} \Vert f\Vert ^{s_2}_{L^{s_1}_{\alpha _1}(0,t_2;Y)} \end{aligned}$$

converges to 0 as $t_2-t_1\rightarrow 0$ for fixed $\delta >0$.

Now the proof of continuity in the case of finite $s_2$ is complete. $\square$

Next we handle the case ${\mathcal {X}}_{\infty ,\alpha }$.

Theorem 3.6

Assume that $\lambda =\mu +\eta \in (0,1)$ for positive $\mu ,\eta$ as in (3.1), (3.2), and that $0< \alpha _2=\lambda +\alpha _1-1$, $0<\alpha _1<1$. Let $f\in L^\infty _{\alpha _1}(0,T;Y)$ satisfy the condition

$$\begin{aligned} \Vert f\Vert _{L^\infty _{\alpha _1}(t)} := \Vert f\Vert _{L^\infty _{\alpha _1}(0,t;Y)} \rightarrow 0 \;\text { as }\; t\rightarrow 0. \end{aligned}$$

(3.6)

(i) For $t\in (0,T)$,

$$\begin{aligned} \Vert Nf(t)\Vert _{{\mathcal {X}}_{\infty ,\alpha _2}^T} \le C_T\Vert f\Vert _{L^\infty _{\alpha _1}(t)}. \end{aligned}$$

(3.7)

Particularly, $Nf(t)\rightarrow 0$ as $t\rightarrow 0$ in ${{\mathcal {X}}}_{\infty ,\alpha _2}$ and $Nf(t)\in \mathring{{\mathcal {X}}}_{\infty ,\alpha _2}$.

(ii) $Nf \in C \big ( [0,T], \mathring{{\mathcal {X}}}_{\infty ,\alpha _2} \big ).$

Proof

(i) We first observe, by (3.2) and the analyticity of $e^{-tA}$, that for $0\le \tau <T$

$$\begin{aligned} \tau ^{\alpha _2} \big \Vert e^{-\tau A}Nf(t)\big \Vert _X \le C\tau ^{\alpha _2} \int ^t_0 (t+\tau -\rho )^{-\lambda } \rho ^{-\alpha _1} \,\mathrm {d}\rho \; \Vert f\Vert _{L^\infty _{\alpha _1}(t)}. \end{aligned}$$

Thus, for $t\le \tau <T$,

$$\begin{aligned} \sup _{t\le \tau<T} \tau ^{\alpha _2} \big \Vert e^{-\tau A}Nf(t)\big \Vert _X&\le C\sup _{t\le \tau <T} \tau ^{\alpha _2} \int ^\tau _0 (\tau -\rho )^{-\lambda } \rho ^{-\alpha _1} \,\mathrm {d}\rho \;\Vert f\Vert _{L^\infty _{\alpha _1}(t)} \\&\le CB \Vert f\Vert _{L^\infty _{\alpha _1}(t)} \end{aligned}$$

by the scale balance, where $B=B(1-\lambda ,1-\alpha _1)$ is the beta function. For $\tau \le t$, we have

$$\begin{aligned} \sup _{0<\tau<t} \tau ^{\alpha _2} \big \Vert e^{-\tau A} Nf(t)\big \Vert _X&\le C\sup _{0<\tau<t} \tau ^{\alpha _2} \int ^t_0 (t-\rho )^{-\lambda } \rho ^{-\alpha _1} \,\mathrm {d}\rho \;\Vert f\Vert _{L^\infty _{\alpha _1}(t)} \nonumber \\&= CB \sup _{0<\tau <t} \tau ^{\alpha _2}t^{-\alpha _2} \Vert f\Vert _{L^\infty _{\alpha _1}(t)}\nonumber \\&= CB \Vert f\Vert _{L^\infty _{\alpha _1}(t)}. \end{aligned}$$

(3.8)

Hence, under assumption (3.6),

$$\begin{aligned} \Vert Nf(t)\Vert _{{\mathcal {X}}_{\infty ,\alpha _2}^T} \le CB \Vert f\Vert _{L^\infty _{\alpha _1}(t)} \rightarrow 0 \quad \text {as}\quad t\rightarrow 0. \end{aligned}$$

For fixed $t>0$, a modification of (3.8) also yields for $0<\tau<\tau _0<t$ the estimate

$$\begin{aligned} \sup _{0<\tau<\tau _0} \tau ^{\alpha _2} \big \Vert e^{-\tau A} Nf(t)\big \Vert _X \le C(t) \sup _{0<\tau <\tau _0} \tau ^{\alpha _2} \cdot \Vert f\Vert _{L^\infty _{\alpha _1}(t)}, \end{aligned}$$

i.e.$Nf(t) \in \mathring{{\mathcal {X}}}_{\infty ,\alpha _2}$.

(ii) It remains to prove the continuity of Nf(t) in ${{\mathcal {X}}}_{\infty ,\alpha _2}$ for $t\ge \delta >0$ for arbitrary $\delta >0$. By definition for $t_1\ge t_2\ge \delta >0$, we observe that

$$\begin{aligned} (Nf)(t_1)&-(Nf)(t_2) \\&= \int ^{t_1}_{t_2} A^\mu e^{-(t_1-\rho )A} f(\rho )\,\mathrm {d}\rho + \int ^{t_2}_0 \left( e^{-(t_1-t_2)A}-I \right) A^\mu e^{-(t_2-\rho )A} f(\rho )\,\mathrm {d}\rho \\&=:{\mathcal {I}}_1 + {\mathcal {I}}_2. \end{aligned}$$

The term ${\mathcal {I}}_1$ is easy to treat. Due to the boundedness of the operator family $e^{-\tau A}$, $0\le \tau \le T$, on X it suffices to consider $\Vert {\mathcal {I}}_1\Vert _X$ directly. If $0<\tau < T$,

$$\begin{aligned} \left\| {\mathcal {I}}_1 \right\| _X&\le c \int ^{t_1}_{t_2} (t_1-\rho )^{-\lambda } \rho ^{-\alpha _1}\,\mathrm {d}\rho \; \Vert f\Vert _{L^\infty _{\alpha _1}(T)} \\&\le c_\delta \int ^{t_1}_{t_2} (t_1-\rho )^{-\lambda } \,\mathrm {d}\rho \; \Vert f\Vert _{L^\infty _{\alpha _1}(T)} \\&\le C_\delta (t_1-t_2)^{1-\lambda } \Vert f\Vert _{L^\infty _{\alpha _1}(T)}. \end{aligned}$$

Thus,

$$\begin{aligned} \limsup _{{\mathop {t_1,t_2\ge \delta }\limits ^{t_1-t_2\rightarrow 0}}}\sup _{0<\tau <T} \tau ^{\alpha _2} \left\| e^{-\tau A}{\mathcal {I}}_1 \right\| _X =0. \end{aligned}$$

For the estimate of ${\mathcal {I}}_2$, we consider $\Vert {\mathcal {I}}_2\Vert _X$ directly. By Lemma 3.2 (ii) and with $0<\beta <1-\lambda$,

$$\begin{aligned} \Vert {\mathcal {I}}_2\Vert _X&\le c \int _0^{t_2} \left( \frac{t_1-t_2}{t_2-\rho }\right) ^\beta (t_2-\rho )^{-\lambda } \Vert f(\rho )\Vert _Y\,\mathrm {d}\rho \\&\le c (t_1-t_2)^\beta \int _0^{t_2}(t_2-\rho )^{-\lambda -\beta } \rho ^{-\alpha _1} \,\mathrm {d}\rho \;\Vert f\Vert _{L^\infty _{\alpha _1}(T)} \\&\le c_\delta (t_1-t_2)^\beta \Vert f\Vert _{L^\infty _{\alpha _1}(T)} \end{aligned}$$

since $t_2\ge \delta >0$. We thus conclude that

$$\begin{aligned} \limsup _{{\mathop {t_1,t_2\ge \delta }\limits ^{t_1-t_2\rightarrow 0}}}\sup _{0<\tau <T} \big \Vert \tau ^{\alpha _2} e^{-\tau A} {\mathcal {I}}_2 \big \Vert _X =0. \end{aligned}$$

Now the assertion $Nf\in C\big ((0,T];{{\mathcal {X}}}_{\infty ,\alpha _2} \big )$ is proved. $\square$

4 Proof of main theorems

We shall prove Theorems 1.1 and 1.2 based on the abstract results given in the previous section.

Proof of Theorem 1.1

We first note that if $X=L^q_\sigma (\Omega )$, A is taken as the Stokes operator and if $2/s+3/q=1-2\alpha$, the Besov space $\mathbb {B}^{-1+3/q}_{q,s}$ is identical with the weighted space ${\mathcal {X}}_{s,\alpha }^T = {\mathcal {X}}_{s,\alpha }$ (for all $0<T\le \infty$) with equivalent norms; see Sect. 2 and Theorem 5.1. For $u_0 \in \mathbb {B}^{-1+3/q}_{q,s}$, the $L^s_\alpha (L^q)$-strong solution u satisfies the integral equation

$$\begin{aligned} u(t)&= e^{-tA} u_0 - \int ^t_0 e^{-(t-\rho )A}\; \mathbb {P}\nabla \cdot \left( (u\otimes u)(\rho )-F(\rho )\right) \,\mathrm {d}\rho \nonumber \\&= e^{-tA} u_0 -\int ^t_0 A^{1/2} e^{-(t-\rho )A}\; A^{-1/2}\mathbb {P}\nabla \cdot \left( (u\otimes u)(\rho )-F(\rho )\right) \,\mathrm {d}\rho , \end{aligned}$$

(4.1)

where $A^{-1/2}\mathbb {P}\nabla$ is bounded in any $L^r(\Omega )$-space, $1<r<\infty$ (see Giga and Miyakawa [15] and Sohr [26]). We observe from the assumptions $u\in L^s_\sigma (L^q)$ and $F\in L^{s/2}_{2\alpha }(L^{q/2})$ that

$$\begin{aligned} f := A^{-1/2}\mathbb {P}\nabla \cdot \left( (u\otimes u)-F\right) \in L^{s/2}_{2\alpha }\big (0,T; L^{q/2}_\sigma (\Omega )\big ). \end{aligned}$$

We take $Y=L^{q/2}_\sigma (\Omega )$ and $X=L^q_\sigma (\Omega )$ and rewrite (4.1) as

$$\begin{aligned} u(t) = e^{-tA} u_0 + Nf(t) \end{aligned}$$

with $\mu =1/2$. By Proposition 3.1, $e^{-tA} u_0 \in C \left( [0,\infty ),X_{s,\alpha }\right)$. Since, see [15],

$$\begin{aligned} \big \Vert e^{-tA} v\big \Vert _X \le C_T t^{-\eta } \Vert v\Vert _Y \end{aligned}$$

with $\eta =3/2q$, the operator N satisfies assumptions (3.1), (3.3) with $\mu =1/2, \eta =3/2q$. Thus, Theorem 3.5 implies that $Nf \in C \left( [0,\infty ),{\mathcal {X}}_{s,\alpha }\right)$. $\square$

Proof of Theorem 1.2

Obviously, the condition $u_0\in \mathring{\mathbb {B}}^{-1+3/q}_{q,\infty }$ is equivalent to say that $u_0\in \mathring{{\mathcal {X}}}_{\infty ,\alpha }$ with $3/q=1-2\alpha$. We recall the construction of the solution of (4.1) by the iteration

$$\begin{aligned} u_1(t)&= e^{-tA} u_0,\\ u_{m+1}(t)&= e^{-tA} u_0 -\int ^t_0 A^{1/2} e^{-(t-\rho )A}\; A^{-1/2}\mathbb {P}\nabla \cdot (u_m\otimes u_m-F)(\rho )\,\mathrm {d}\rho \quad (m\ge 1). \end{aligned}$$

Since $u_0\in \mathring{{\mathcal {X}}}_{\infty ,\alpha }$, by Proposition 3.1 (iii) $u_1(t)= e^{-tA} u_0\in C\big ([0,T];\mathring{{\mathcal {X}}}_{\infty ,\alpha }\big )$ and even $\Vert u_{1}\Vert _{L^\infty _\alpha (0,t;X)}\rightarrow 0$ as $t\rightarrow 0$. By the assumption on F in Theorem 1.2, we conclude from Proposition 3.4 inductively that $\Vert u_{m+1}\Vert _{L^\infty _\alpha (0,t;X)}\rightarrow 0$ as $t\rightarrow 0$ for every $m\in {\mathbb {N}}$. Hence, also the limit solution u which is the limit of $(u_m)$ in $L^\infty _\alpha (0,T;X)$ has the same property at $t=0$.

We now consider (4.1) and apply Proposition 3.1 and Theorem 3.6, under assumption (3.6) satisfied by u, F, to get the desired continuity. $\square$

Proof of Theorem 1.3

Let u, v be $L^s_\alpha (L^q)$-strong solutions of (1.1) with data $f=\mathop {\mathrm {div}}F, u_0$ and $g=\mathop {\mathrm {div}}G, v_0$. Being mild solutions of (4.1), the difference $w=u-v$ solves the integral equation

$$\begin{aligned} w(t)&= e^{-tA} w_0 -\int ^t_0 A^{1/2} e^{-(t-\rho )A}\; A^{-1/2}\mathbb {P}\nabla \cdot (w\otimes u + v\otimes w -(F-G))(\rho )\,\mathrm {d}\rho \nonumber \\&= e^{-tA} w_0 - (Ng)(t), \end{aligned}$$

(4.2)

where the linear operator N is defined by (3.1) with $\mu =\frac{1}{2}$, $X=L^q(\Omega )$, $Y=L^{q/2}(\Omega )$, $\eta = \frac{3}{2q}$ in (3.2), and

$$\begin{aligned} g = A^{-1/2}\mathbb {P}\nabla \cdot (w\otimes u + v\otimes w -(F-G)) \end{aligned}$$

satisfies the elementary estimate

$$\begin{aligned} \Vert g\Vert _{L^{s/2}_{2\alpha }(L^{q/2})} \le c \big (\Vert w\Vert _{L^{s}_\alpha (L^{q})} \big (\Vert u\Vert _{{L^{s}_\alpha }(L^{q})} + \Vert v\Vert _{L^{s}_\alpha (L^{q})}\big ) + \Vert F-G\Vert _{L^{s/2}_{2\alpha }(L^{q/2})}\big ). \end{aligned}$$

(4.3)

Now Lemma 3.3 with exponents

$$\begin{aligned} s_1=\frac{s}{2},\; \alpha _1=2\alpha ,\; s_2=s,\; \alpha _2=\alpha \quad \text { and } \lambda =\frac{1}{2}+\frac{3}{2q} \end{aligned}$$

(4.4)

implies that

$$\begin{aligned} \Vert Ng\Vert _{L^{s_2}_{\alpha _2}(L^q)} \le C\Vert g\Vert _{L^{s_1}_{\alpha _1}(L^{q/2})}. \end{aligned}$$

(4.5)

Since $\Vert e^{-tA} w_0\Vert _{L^s_\alpha (L^q)} \le \Vert w_0\Vert _{{\mathcal {X}}_{s,\alpha }^T}$, Proposition 3.4 and (4.3) lead to the estimate

$$\begin{aligned} \Vert w\Vert _{L^{s}_\alpha (L^{q})} \le \Vert w_0\Vert _{{\mathcal {X}}_{s,\alpha }^T} + C\big (\Vert w\Vert _{L^{s}_\alpha (L^{q})} \big (\Vert u\Vert _{{L^{s}_\alpha }(L^{q})} + \Vert v\Vert _{L^{s}_\alpha (L^{q})}\big ) + \Vert F-G\Vert _{L^{s/2}_{2\alpha }(L^{q/2})}\big ), \end{aligned}$$

(4.6)

where the $L^{s}_\alpha (L^{q})$-norm is considered on a time interval (0, T). Choosing $T_0\le T$ such that, as in the assumption of Theorem 1.3, $\Vert u, v\Vert _{{L^{s}_\alpha }(0,T_0;L^{q})} \le \varepsilon _*$ with $2C\varepsilon _* \le \frac{1}{2}$ the term involving w on the right-hand side of (4.6) can be absorbed. This proves the estimate (1.4), but still with the left-hand side replaced by $\Vert u-v\Vert _{L^{s}_\alpha (L^{q})}$.

Next we apply (3.4) from Theorem 3.5 to (4.2) to get that

$$\begin{aligned} \Vert w(t)\Vert _{{\mathcal {X}}_{s,\alpha }^T}&\le \Vert u_0-v_0\Vert _{{\mathcal {X}}_{s,\alpha }^T} + \Vert Ng(t)\Vert _{{\mathcal {X}}_{s,\alpha }^T} \nonumber \\&\le \Vert u_0-v_0\Vert _{{\mathcal {X}}_{s,\alpha }^T} + C\Vert g\Vert _{L^{s/2}_{2\alpha }(0,T; L^{q/2})} \nonumber \\&\le \Vert u_0-v_0\Vert _{{\mathcal {X}}_{s,\alpha }^T} + C\Vert F-G\Vert _{L^{s/2}_{2\alpha }(0,T; L^{q/2})} \nonumber \\&\quad +\, C\Vert w\Vert _{L^s_\alpha (0,T;L^q)} (\Vert u\Vert _{L^s_\alpha (0,T;L^q)} + \Vert v\Vert _{L^s_\alpha (0,T;L^q)} \big ). \end{aligned}$$

(4.7)

Under the smallness assumption on $\Vert u,v\Vert _{L^s_\alpha (0,T_0;L^q)}$ for a suitable $0<T_0\le T$, we can insert (4.6), i.e. (1.4) with the left-hand side $\Vert u-v\Vert _{L^s_\alpha (0,T_0;L^q)}$ instead of $\Vert u-v\Vert _{{\mathcal {X}}_{s,\alpha }^T}$, into (4.7) and conclude the proof of Theorem 1.3. $\square$

Proof of Theorem 1.4

The proof is similar to the proof of Theorem 1.3. Here $s_1'=s_2'=1$ and (3.7) from Theorem 3.6 is applied to (4.6). $\square$

Remark 4.1

(i) Our continuity results (Theorems 1.1 and 1.2) have an overlap with results in [16, Remark 4.19, Theorem 4.20]. Their external force f is allowed to be of the form $f=f_0 + \mathop {\mathrm {div}}F$, where both f and F are t-dependent but with values in $L^2(\Omega )$. If there are no external forces, our results are contained in their results. However, in [16] a heavy, technical machinery based on interpolation and extrapolation spaces is applied to abstract parabolic equations with quadratic nonlinearity. Our approach is much more direct and simple and uses only basic terminology from interpolation theory including—in one step—the duality theorem of real interpolation.

(ii) The results of Amann [2] cannot be compared with ours. In [2, Sect. 5], he considers more regular solutions with initial value in $\mathring{{\mathbb {B}}}^{-1+3/q}_{q,\infty }$ but with forces in weighted $C^0$ spaces so that solutions are classically regular for $t>0$; see [2, Theorem 6.1]. Although a weaker force is discussed in [2, Remark 7.3], his space is not of Besov type but of Bessel potential type in the analysis of the Navier–Stokes system on a domain.

5 Appendix: Besov spaces

For $1<q<\infty$, $1\le r\le \infty$ and $t\in \mathbb {R}$, let $B^t_{q,r}(\mathbb {R}^3)$ denote the usual Besov spaces, see [30, 2.3.1], and define for the bounded domain $\Omega \subset \mathbb {R}^3$ the space $B^t_{q,r}(\Omega )$ by restriction of elements in $B^t_{q,r}(\mathbb {R}^3)$ in the sense of distributions to $\Omega$; the norm of $u\in B^t_{q,r}(\Omega )$ is defined by $\Vert u\Vert _{B^t_{q,r}(\Omega )} = \inf \big \{\Vert v\Vert _{B^t_{q,r}(\mathbb {R}^3)}: v\in B^t_{q,r}(\mathbb {R}^3), v_{|_\Omega }=u\big \}$. Concerning Besov spaces on $\Omega$ with vanishing trace—if possible—the definition is modified as follows: Considering only vector fields rather than scalar-valued functions and the range $t\in [-2,2]$, we follow Amann [2, 3] and define

$$\begin{aligned} {\mathbf {B}}^t_{q,r}(\Omega ) = {\left\{ \begin{array}{ll} \{u\in B^t_{q,r}(\Omega )^3;\; u_{|_{\partial \Omega }}=0\}, &{}\quad 1/q<t\le 2,\\ \\ \{u\in B^{1/q}_{q,r}(\mathbb {R}^3)^3;\; \mathop {\mathrm {supp}}(u) \subset {\overline{\Omega }}\}, &{}\quad 1/q=t,\\ \\ B^t_{q,r}(\Omega )^3, &{}\quad 0\le t<1/q, \\ \\ \big ({\mathbf {B}}^{-t}_{q',r'}(\Omega )\big )' \;\; (1<r\le \infty ), &{} \quad -2\le t<0. \end{array}\right. } \end{aligned}$$

(5.1)

For spaces of solenoidal vector fields on $\Omega$, let

$$\begin{aligned} {\mathbb {B}}^t_{q,r}(\Omega ) = {\left\{ \begin{array}{ll} {\mathbf {B}}^t_{q,r}(\Omega ) \cap L^q_\sigma (\Omega ), &{}\quad 0< t\le 2,\\ \\ \text {cl} \big (C^\infty _{c,\sigma }(\Omega )\big ) \text { in } {\mathbf {B}}^0_{q,r}(\Omega ), &{}\quad t=0,\\ \\ \big ({\mathbb {B}}^{-t}_{q',r'}(\Omega )\big )' \;\; (1<r\le \infty ), &{}\quad -2\le t<0, \end{array}\right. } \end{aligned}$$

(5.2)

where “cl” denotes the closure. Note that $u\in {\mathbb {B}}^t_{q,r}(\Omega )$ with $\frac{1}{q}<t\le 2$ vanishes on $\partial \Omega$ by (5.1), but that only the normal component of u vanishes on $\partial \Omega$ when $0<t\le \frac{1}{q}$ since $u\in L^q_\sigma (\Omega )$. The definition for $t=0$ is more involved since the inclusion $L^q(\Omega )\subset B^0_{q,r}(\Omega )$ holds if and only if either $2\le q \le r$ or $q\le 2\le r$. For $r=\infty$, the space is also called Nikol’skii space and denoted by $\mathbb {N}^t_q(\Omega )$.

Moreover, we need the spaces (little Nikol’skii spaces, also denoted as )

$$\begin{aligned} \mathring{{\mathbb {B}}}^t_{q,\infty }(\Omega ) := \text {cl} \big ({\mathbf {H}}^t_q(\Omega )\cap L^q_\sigma (\Omega )\big ) \text { in } {\mathbb {B}}^t_{q,\infty } (\Omega ), \end{aligned}$$

where ${\mathbf {H}}^t_q(\Omega )$ is a Bessel potential space defined by restriction of the usual Bessel potential space $H^t_q(\mathbb {R}^3)^3$ to vector fields on $\Omega$ (vanishing on $\partial \Omega$ if $t>\frac{1}{q}$ as in (5.1)), cf. [3, pp. 3–4]. Using the notation $(\cdot ,\cdot )_{\theta ,r}$, $1\le r<\infty$, of real interpolation, and $(\cdot ,\cdot )_{\theta ,\infty }^0$ for the continuous interpolation functor, Theorem 3.4 in [2] states that for $0<\theta <1$

$$\begin{aligned} (L^q_\sigma (\Omega ), {\mathcal {D}}(A_q))_{\theta ,r}&= {\mathbb {B}}^{2\theta }_{q,r} (\Omega ), \end{aligned}$$

(5.3)

$$\begin{aligned} (L^q_\sigma (\Omega ), {\mathcal {D}}(A_q))_{\theta ,\infty }^0&= \mathring{{\mathbb {B}}}^{2\theta }_{q,\infty }(\Omega ). \end{aligned}$$

(5.4)

Note that ${\mathcal {D}}(A_{q})$ is equipped with its graph norm, and that for a bounded domain this graph norm can be simplified to $\Vert A_q \cdot \Vert _q$ since $0\in \rho (A_q)$. As is well known ([23, Proposition 6.2, Exercise 6.1.1 (1)], equivalent norms on the spaces $(L^q_\sigma (\Omega ), {\mathcal {D}}(A_q))_{\theta ,r}$, $1\le r\le \infty$, are given by

$$\begin{aligned} \Vert u\Vert _{{\mathbb {B}}^{2\theta }_{q,r}}&\sim \displaystyle \Big (\int _0^T \big (\tau ^{1-\theta } \Vert A_q e^{-\tau A_q}u\Vert _q\big )^r \frac{{\mathrm{d}}\tau }{\tau }\Big )^{1/r} \text { if } \;1\le r<\infty , \end{aligned}$$

(5.5)

and for $r=\infty$

$$\begin{aligned} \Vert u\Vert _{(L^q_\sigma ,{\mathcal {D}}(A_q))_{\theta ,\infty }}&\sim \sup _{(0,T)} \tau ^{1-\theta }\Vert A_q e^{-\tau A_q}u\Vert _q \qquad \text { if }r=\infty , \end{aligned}$$

(5.6)

where $T\in (0,\infty )$ can be chosen arbitrarily. The space $\mathring{{\mathbb {B}}}^{2\theta }_{q,\infty }(\Omega )$ is equipped with the norm of $(L^q_\sigma (\Omega ),{\mathcal {D}}(A_q))_{\theta ,\infty }$, but elements $u\in \mathring{{\mathbb {B}}}^{2\theta }_{q,\infty }(\Omega )$ enjoy the further property that

$$\begin{aligned} \lim _{\tau \rightarrow 0} \tau ^{1-\theta }\Vert A_q e^{-\tau A_q}u\Vert _q =0. \end{aligned}$$

(5.7)

We note that the identity (5.4) for the usual interpolation space $(L^q_\sigma (\Omega ),{\mathcal {D}}(A_q))_{\theta ,\infty }$ and the Besov space ${\mathbb {B}}^{2\theta }_{q,\infty }(\Omega )$ is not found in the literature. This remark also applies to (5.12) dealing with negative exponents $\theta$.

To obtain similar representations for negative exponents of regularity as well recall that for $-1<\theta <0$ and $1<r\le \infty$ by (5.2), (5.3) and the duality theorem of real interpolation ([30, Theorem 1.11.2])

$$\begin{aligned} \big (L^q_\sigma (\Omega ),{\mathcal {D}}(A_{q'})'\big )_{-\theta ,r} = \big (\big (L^{q'}_\sigma (\Omega ),{\mathcal {D}}(A_{q'})\big )_{-\theta ,r'}\big )' = \big ({\mathbb {B}}^{-2\theta }_{q',r'}(\Omega )\big )' = {\mathbb {B}}^{2\theta }_{q,r}(\Omega ). \end{aligned}$$

Then we get the characterizations (here $-1<\theta <0$):

$$\begin{aligned} \big ({\mathcal {D}}(A_{q'})', L^q_\sigma (\Omega )\big )_{1+\theta ,r}&= {\mathbb {B}}^{2\theta }_{q,r}(\Omega ), \quad 1\le r<\infty , \end{aligned}$$

(5.8)

$$\begin{aligned} \big ({\mathcal {D}}(A_{q'})', L^q_\sigma (\Omega )\big )_{1+\theta ,\infty }&= {\mathbb {B}}^{2\theta }_{q,\infty }(\Omega ) \cong {\mathbf {B}}^{2\theta }_{q,\infty }(\Omega )/\big ({\mathbb {B}}^{-2\theta }_{q',1}(\Omega )\big )^\perp , \end{aligned}$$

(5.9)

$$\begin{aligned} \big ({\mathcal {D}}(A_{q'})', L^q_\sigma (\Omega )\big )_{1+\theta ,\infty }^0&= \mathring{{\mathbb {B}}}^{2\theta }_{q,\infty }(\Omega ) = \text {cl} \big ({\mathbf {H}}^2_q(\Omega )\big ) \text { in } \big ({\mathbb {B}}^{-2\theta }_{q',1}(\Omega )\big )'. \end{aligned}$$

(5.10)

Actually, (5.8) for $r=1$ and (5.10) follow from [2, Theorem 3.4], [3, p. 4], for all $-1<\theta <0$; the space $\mathring{{\mathbb {B}}}^{2\theta }_{q,\infty }(\Omega )$ also coincides with the closure $\text {cl} \big (L^q_\sigma (\Omega ))$ in ${\mathbb {B}}^{2\theta }_{q,\infty }(\Omega )$. To prove (5.9), we exploit the isomorphism

$$\begin{aligned} {\mathbb {B}}^{2\theta }_{q,\infty }(\Omega ) = \big ({\mathbb {B}}^{-2\theta }_{q',1}(\Omega )\big )' \cong {\mathbf {B}}^{2\theta }_{q,\infty }(\Omega ) /({\mathbb {B}}^{-2\theta }_{q',1}(\Omega ))^\perp ; \end{aligned}$$

see [2, Remark 3.6] and its proof as well as definitions (5.1), (5.2).

Furthermore, note from Sect. 2 that $A_q$ is an isomorphism from ${\mathcal {D}}(A_{q})$ to $L^q_\sigma (\Omega )$ and also from $L^q_\sigma (\Omega )$ to ${\mathcal {D}}(A_{q'})'$. Hence, for all $1\le r\le \infty$ and $-1<\theta <0$

$$\begin{aligned} \big ({\mathcal {D}}(A_{q'})', L^q_\sigma (\Omega )\big )_{1+\theta ,r} = A\big (\big (L^q_\sigma (\Omega ),{\mathcal {D}}(A_{q}) \big )_{1+\theta ,r}\big ), \end{aligned}$$

(5.11)

with a similar result for the continuous interpolation functor $(\cdot ,\cdot )_{\theta ,\infty }^0$.

Thus, for any $1\le r\le \infty$ and $-1<\theta <0$, by (5.11), (5.8), (5.9) and (5.3), $\big ({\mathcal {D}}(A_{q'})' ,L^q_\sigma (\Omega ) \big )_{1+\theta ,r} = A\big (\big (L^q_\sigma (\Omega ),{\mathcal {D}}(A_{q}) \big )_{1+\theta ,r}\big ) = {\mathbb {B}}^{2\theta }_{q,r}(\Omega )$ with equivalent norm

$$\begin{aligned} \Vert u\Vert _{A((L^q_\sigma (\Omega ),{\mathcal {D}}(A_{q}) )_{1+\theta ,r})} \sim {\left\{ \begin{array}{ll} \displaystyle \Big (\int _0^T \big ( \tau ^{-\theta }\Vert e^{-\tau A_q}u\Vert _q\big )^r \, \frac{\mathrm{d} \tau }{\tau }\Big )^{1/r} &{} \text { if } 1\le r<\infty , \\ \sup _{\tau \in (0,T)} \tau ^{-\theta }\Vert e^{-\tau A_q}u\Vert _q &{} \text { if } r=\infty . \end{array}\right. }\quad \quad \end{aligned}$$

(5.12)

This result was used in [12] when $\frac{2}{r}+\frac{3}{q}=1,\, \theta =0$, $2<r<\infty$. For the continuous interpolation space $\big ({\mathcal {D}}(A_{q'})', L^q_\sigma (\Omega )\big )_{1+\theta ,\infty }^0 = \,\mathring{{\mathbb {B}}}^{2\theta }_{q,\infty }(\Omega )$, we have the norm defined in (5.12), with the additional property that

$$\begin{aligned} \lim _{\tau \rightarrow 0}\tau ^{-\theta } \Vert e^{-\tau A_q}u\Vert _q =0. \end{aligned}$$

Summarizing the previous arguments, we get the following theorem.

Theorem 5.1

Choose any $T\in (0,\infty )$.

• (i) Let $2<s<\infty$, $3<q<\infty$ and $0<\alpha <\frac{1}{2}$ such that $\frac{2}{s} + \frac{3}{q}=1-2\alpha$. Then the real interpolation space $\big ({\mathcal {D}}(A_{q'})', L^q_\sigma (\Omega )\big )_{1-\alpha ,s}$ coincides with the Besov space ${\mathbb {B}}^{-1+3/q}_{q,s}(\Omega )$ and has the equivalent norm $\big (\!\int _0^T (\tau ^{\alpha }\Vert e^{-\tau A_q}u\Vert _q)^s \,\mathrm {d}\tau \big )^{1/s}$.

• (ii) If $3<q<\infty$ and $0<\alpha <\frac{1}{2}$ such that $\frac{3}{q}=1-2\alpha$, the real interpolation space $\big ({\mathcal {D}}(A_{q'})', L^q_\sigma (\Omega )\big )_{1-\alpha ,\infty }$ coincides with the space of Besov type ${\mathbb {B}}^{-1+3/q}_{q,\infty }(\Omega )$ and has the equivalent norm $\sup _{\tau \in (0,T)} \tau ^{\alpha }\Vert e^{-\tau A_q}u\Vert _q$.

• (iii) The interpolation space $\big ({\mathcal {D}}(A_{q'})', L^q_\sigma (\Omega )\big )_{1-\alpha ,\infty }^0$ equals the Besov space $\mathring{{\mathbb {B}}}^{-1+3/q}_{q,\infty }(\Omega )$, equipped with the norm of ${\mathbb {B}}^{-1+3/q}_{q,\infty }(\Omega )$ such that the property $\lim _{\tau \rightarrow 0} \tau ^{\alpha } \Vert e^{-\tau A_q}u\Vert _q =0$ additionally holds for $u\in \, \mathring{{\mathbb {B}}}^{-1+3/q}_{q,\infty }(\Omega )$.