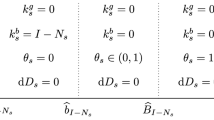A mathematical treatment of bank monitoring incentives

Abstract

In this paper, we take up the analysis of a principal/agent model with moral hazard introduced by Pagès (J. Financ. Intermed. doi:10.1016/j.jfi.2012.06.001, 2012), with optimal contracting between competitive investors and an impatient bank monitoring a pool of long-term loans subject to Markovian contagion. We provide here a comprehensive mathematical formulation of the model and show, using martingale arguments in the spirit of Sannikov (Rev. Econ. Stud. 75:957–984, 2008), how the maximization problem with implicit constraints faced by investors can be reduced to a classical stochastic control problem. The approach has the advantage of avoiding the more general techniques based on forward-backward stochastic differential equations described by Cvitanić and Zhang (Contract Theory in Continuous Time Models, Springer 2012) and leads to a simple recursive system of Hamilton–Jacobi–Bellman equations. We provide a solution to our problem by a verification argument and give an explicit description of both the value function and the optimal contract. Finally, we study the limit case where the bank is no longer impatient.

Appendix A

Proof of Proposition 3.5

In this particular case, Problem (3.2) becomes

(A.1)

Consider first the subproblem derived from (A.1) by abstracting from the initial payment D ₀ and ignoring the incentive compatibility constraint u _t ≥b ₁, i.e.,

The constraint can be written equivalently as

$$ \mathbb{E}^{\mathbb{P}} \biggl[ \int_{0^{+}}^{\tau}e^{-rt} \bigl( dD_{t}-(r+\lambda_{1})u\,dt \bigr) \biggr] \geq0. $$

The corresponding Lagrangian is

$$ \mathcal{L}_{t}=\mu\,dt-dD_{t}+\nu_{t}e^{-rt} \bigl( dD_{t}-u(r+\lambda_{1})\,dt \bigr) , $$

where ν _t is the Lagrange multiplier at time t. Optimizing with respect to D, we get ν _t =e ^rt and the complementary slackness conditions imply that the dividend process is absolutely continuous and constant, namely dD _t =δ _t   dt, with δ _t =(r+λ ₁)u. Because the process D obtained in this manner is clearly admissible, this yields $\widetilde{v}_{1}(u)= ( \mu-(r+\lambda_{1})u ) /\lambda_{1}$.

Turning now to (A.1), but still ignoring the incentive compatibility constraint, we have

$$ v_{1}(u)=\sup_{D_{0}} \bigl(-D_{0}+ \widetilde{v}_{1}(u-D_{0}) \bigr), $$

which is increasing in D ₀ when r>0. Since u ₀=u−D ₀ from the bank’s promise-keeping constraint (3.4), the highest initial payment consistent with the incentive compatibility constraint at time 0 is D ₀=u−b ₁. This yields

$$ v_{1}(u) =b_{1}-u+\widetilde{v}_{1}(b_{1}) = b_{1}-u+\overline{v}_{1}, $$

where $\overline{v}_{1}$ is defined as in the proposition. Finally, one verifies that δ _t =b ₁(r+λ ₁) yields u _t =b ₁ on [0,τ), so that the incentive compatibility condition binds at all times before default, as desired. □

Proof of Proposition 3.13(i)

We show the result by induction.

• Initialization with j=2

The solution of the ODE (3.16) for j=2 and a given fixed value of γ≥b ₂ can be easily calculated and is given by $\widetilde{v}_{2}(u,\gamma)=\gamma-u+v_{2}(\gamma)$ for u>γ and

Now since we have shown that v ₁ is everywhere twice differentiable except at b ₁, we have for every γ≠b ₁+b ₂ and every b ₂<u≤γ that

$$\frac{\partial\widetilde{v}_2}{\partial\gamma}(u,\gamma)= \biggl(v'_1( \gamma-b_2)+1-\frac{r}{\lambda_2} \biggr) \biggl( \biggl( \frac{ru+\lambda_2b_2}{r\gamma+\lambda_2b_2} \biggr)^{\frac {\lambda_2}{r}}1_{\{u\leq\gamma\}}+1_{\{u>\gamma\}} \biggr). $$

Thus the above expression always has the sign of $v'_{1}(\gamma -b_{2})+1-\frac{r}{\lambda_{2}}$, that is to say it is positive for γ<b ₁+b ₂ and negative for γ>b ₁+b ₂. Hence, we clearly have for all b ₂<u that

$$\sup_{\gamma\geq b_2}\widetilde{v}_2(u, \gamma)=\widetilde{v}_2(u,b_1+b_2), $$

which means that the maximal solution of (3.16) for j=2 corresponds to the choice γ ₂=b ₁+b ₂, which also happens to correspond to the unique solution of

$$\frac{r}{\lambda_{2}}-1\in\partial v_{1}(\gamma_{2}-b_1). $$

Then, after some calculations, we find that for all b ₂<u<b ₁+b ₂,

$$v''_2(u)=- \biggl(\lambda_2-r+ \lambda_2\frac{\overline{v}_1}{b_1} \biggr)\frac{ (ru+\lambda_2b_2 )^{\frac{\lambda_2}{r}-1}}{ (r(b_1+b_2)+\lambda_2b_2 )^{\frac{\lambda_2}{r}}}\leq0, $$

because of (3.18). Hence, since v ₂ is linear on [b ₁+b ₂,+∞) and differentiable at b ₁+b ₂, it is concave on (b ₂,+∞). Now if we consider the linear extrapolation of v ₂ over [0,b ₁] by (3.15), we just need to verify that the left derivative of v ₂ at b ₂ is less than its right derivative to obtain the concavity of v ₂ over [0,+∞]. Taking the limit for u↓b ₂ in (3.16), we obtain

$$v'_2 \bigl(b_2^+ \bigr)=\frac{\lambda_2\overline{v}_2-2\mu }{b_2(r+\lambda_2)}. $$

This implies that

$$v'_2 \bigl(b_2^- \bigr)-v'_2 \bigl(b_2^+ \bigr)=\frac{2\mu}{b_2\lambda_2}+v'_2 \bigl(b_2^+ \bigr)\frac {r}{\lambda_2}\geq\frac{\mu\varepsilon}{B} - \frac{r}{\lambda_2}. $$

Now recall Assumption 2.3, which implies that

$$\frac{r}{\lambda_j}<\frac{r}{\overline{\alpha}_j}\leq\frac{\mu \varepsilon-B}{B}\frac{\varepsilon}{1+\varepsilon}< \frac{\mu\varepsilon}{B} $$

for any j so that $v'_{2}(b_{2}^{-})-v'_{2}(b_{2}^{+})\geq0$.

• Heredity for j≥3

Let us now suppose that the maximal solution v _j−1 of (3.16) has been constructed for some j≥3, that it is globally concave on [0,+∞), everywhere differentiable except at b _j−1, everywhere twice differentiable except at b _j−1 and b _j−1+b _j−2, and that the corresponding γ _j−1≥b _j−1+b _j−2. Let us now construct the maximal solution corresponding to j. Exactly as in the case j=2, the solution of the ODE (3.16) for a given fixed value of γ≥b _j can be easily calculated and is given by

for b _j <u≤γ, and $\widetilde{v}_{j}(u,\gamma)=\gamma -u+v_{j}(\gamma)$ for u>γ. Note also that it is clear from (3.16) that v _j is differentiable everywhere except at b _j , and twice differentiable everywhere except at b _j and b _j +b _j−1.

Now since we assumed that v _j−1 is everywhere differentiable except at b _j−1, we have for every γ≠b _j−1+b _j and every b _j <u≤γ that

$$\frac{\partial\widetilde{v}_j}{\partial\gamma}(u,\gamma)= \biggl(v'_{j-1}( \gamma-b_j)+1-\frac{r}{\lambda_j} \biggr) \biggl( \biggl( \frac {ru+\lambda_jb_j}{r\gamma+\lambda_jb_j} \biggr)^{\frac{\lambda _j}{r}}1_{\{u\leq\gamma\}}+1_{\{u>\gamma\}} \biggr). $$

Thus, since v _j−1 is concave and its derivative is therefore nonincreasing, we can conclude as in the case j=2 that the maximal solution is uniquely determined by the choice γ _j which corresponds to the solution of

$$\frac{r}{\lambda_{j}}-1\in\partial v_{j-1}(\gamma_{j}-b_j). $$

More precisely, using (3.18), we have only two cases. Either

$$v'_{j-1} \bigl(b_{j-1}^+ \bigr)\leq \frac{r}{\lambda_j}-1\leq\frac{\overline {v}_{j-1}}{b_{j-1}} $$

and γ _j =b _j−1+b _j , or

$$\frac{r}{\lambda_j}-1<v'_{j-1} \bigl(b_{j-1}^+ \bigr) $$

and b _j−1+b _j <γ _j ≤γ _j−1+b _j .

Let us now study the concavity. We can differentiate twice equation (3.16) on (b _j ,b _j +b _j−1) since v _j−1(u−b _j ) is linear and thus twice differentiable on this open interval. We then obtain easily that

(A.2)

There are then two cases. If γ _j =b _j +b _j−1, differentiating once (3.16) and then taking the limit u↑b _j +b _j−1, we get

$$\bigl(r(b_j+b_{j-1})+\lambda_jb_j \bigr)v''_j \bigl((b_j+b_{j-1})^- \bigr)=\lambda_j \biggl(\frac{r}{\lambda_j}-1-\frac{\overline{v}_{j-1}}{b_{j-1}} \biggr)\leq0. $$

Since $v^{\prime\prime}_{j}(u)=0$ for $u>b_{j}+b_{j_{1}}$, we have proved concavity on (b _j ,+∞). If γ _j >b _j +b _j−1, differentiating once (3.16) and taking limits on both sides of b _j +b _j−1, we obtain

(A.3)

where the right-hand side is positive by the concavity of v _j−1. Next, we differentiate twice (3.16) on (b _j +b _j−1,γ _j ]. We obtain easily

$$ v''_j(u)= \lambda_j(ru+\lambda_jb_j)^{\frac{\lambda_j}{r}-2}\int _u^{\gamma_j}\frac{v''_{j-1}(x-b_j)}{(ru+\lambda_jb_j)^{\frac {\lambda _j}{r}-1}}\,dx. $$

(A.4)

Note that we should normally distinguish between the cases b _j +b _j−1+b _j−2≤γ _j or not, since v _j−1 is not twice differentiable at b _j−1+b _j−2. However, since we know that v _j is twice differentiable at b _j +b _j−1+b _j−2, this actually does not change the result. Since v _j−1 is concave, (A.4) implies that v _j is concave on (b _j +b _j−1,+∞). Then with (A.3) we see that the left second derivative of v _j at b _j +b _j−1 is negative, which thanks to (A.2) finally shows the concavity on (b _j ,+∞).

Finally, it remains to show that $v'_{j}(b_{j}^{+})\leq\frac{\overline {v}_{j}}{b_{j}}$. We take the limit for u↓b _j in (3.16) to obtain

$$v'_j \bigl(b_j^+ \bigr)=\frac{\lambda_j\overline{v}_j-j\mu }{b_j(r+\lambda_j)}. $$

Since $v'_{j}\geq-1$, this implies that

$$v'_j \bigl(b_j^- \bigr)-v'_j \bigl(b_j^+ \bigr)=\frac{j\mu}{b_j\lambda_j}+v'_j \bigl(b_j^+ \bigr)\frac {r}{\lambda_j}\geq\frac{\mu\varepsilon}{B}- \frac{r}{\lambda_j}, $$

which has already been shown to be positive under Assumption 2.3. Hence v _j is concave on [0,+∞). □

Proof of Proposition 3.13(ii)

First of all, by the properties of the function ψ ₁ recalled in Remark 3.12, it is clear that we can always find a λ _j such that (3.19) is satisfied. Then if for a fixed j≥2, we have $v'_{j-1}(b_{j-1}^{+})\leq0$, differentiating (3.16) immediately gives for u>b _j and u≠b _j +b _j−1 that

$$ \lambda_j \bigl(v'_j(u)-v'_{j-1}(u-b_j) \bigr)=(ru+\lambda_jb_j)v''_j(u)+rv'_j(u). $$

(A.5)

Since we have proved in (i) that the v _j are concave, it is clear that if $v'_{j-1}(b_{j-1}^{+})\leq0$, the right-hand side above is negative. Then by left- and right-continuity of $v'_{j-1}$ at b _j−1, the result extends to u=b _j +b _j−1. Hence the desired property (3.20) follows. In particular, this proves the result for j=2 since $v'_{1}(b_{1}^{+})=-1$. Note also that the property (3.20) clearly holds for v _j when u>γ _j . Indeed, we have then

$$v'_{j}=-1, $$

and we know that the derivative of v _j−1 is always greater than −1.

Let us now show the rest of the result by induction. Since (3.20) is true for j=2, let us fix a j≥3 and assume that

$$ v'_{j-1}(u)-v'_{j-2}(u-b_{j-1}) \leq0,\quad u>b_{j-1}. $$

(A.6)

Now if $v'_{j-1}(b_{j-1}^{+})\leq0$, we already know that the property (3.20) is true for v _j , so we assume that $v'_{j-1}(b_{j-1}^{+})> 0$. Moreover, by our remark above, we know that (3.20) holds true for v _j when u>γ _j . Let us then first prove (3.20) for v _j when u>b _j +b _j−1. If γ _j =b _j +b _j−1, there is nothing to do. Otherwise, we have, using successively (A.5) and (A.4),

(A.7)

Now if we differentiate (3.16) and solve the corresponding ODE for $v'_{j}$, we obtain

$$ v'_j(u)=(ru+\lambda_jb_j)^{\frac{\lambda_j}{r}-1} \int_u^{\gamma_j}\frac {\lambda_jv'_{j-1}(x-b_j)}{(rx+\lambda_jb_j)^{\frac{\lambda_j}{r}}}\,dx - \biggl( \frac{ru+\lambda_jb_j}{r\gamma_j+\lambda_jb_j} \biggr)^{\frac{\lambda_j}{r}-1}. $$

(A.8)

Using (A.8) in (A.7), we obtain for u>b _j +b _j−1 that

(A.9)

Then we have for all x≥u>b _j +b _j−1 and x≠b _j +b _j−1+b _j−2 that

where we used the induction hypothesis (A.6) in the last inequality. Since v _j−1 is concave, the sign of the right-hand side above is given by the sign of

Using this in (A.9) implies

$$v'_j(u)-v'_{j-1}(u-b_j) \leq0,\quad u>b_j+b_{j-1}. $$

It remains to prove (3.20) when b _j <u<b _j +b _j−1. In that case, (3.20) can be written as

$$v'_j(u)-\frac{\overline{v}_{j-1}}{b_{j-1}}\leq0,\quad b_j<u<b_j+b_{j-1}, $$

which is equivalent by concavity of v _j to

$$v'_j \bigl(b_j^+ \bigr)-\frac{\overline{v}_{j-1}}{b_{j-1}} \leq0. $$

Now using (A.8), we also have

And thus we get with (3.17)

which implies

$$v'_j \bigl(b_j^+ \bigr)-\frac{\overline{v}_{j-1}}{b_{j-1}} \leq\phi_{\frac {b_{j-1}}{b_j}} \biggl(\frac{r}{\lambda_j} \biggr)\frac{\overline {v}_{j-1}}{b_{j-1}} \biggl(\frac{v'_{j-1}(b_{j-1}^+)}{\frac{\overline {v}_{j-1}}{b_{j-1}}}-\psi_{\frac{b_{j-1}}{b_j}} \biggl(\frac {r}{\lambda_j} \biggr) \biggr). $$

By Assumption 2.4, we know that b _j ≥b _j−1. Hence with (3.19) and by what we recalled earlier about the functions ψ _β in Remark 3.12, we have

$$\frac{\overline{v}_{j-1}}{b_{j-1}}\leq\psi \biggl(\frac{r}{\lambda_j} \biggr)\leq \psi_{\frac{b_{j-1}}{b_j}} \biggl(\frac{r}{\lambda_j} \biggr), $$

which implies the desired property and ends the proof. □