Skip to main content
SpringerLink
Log in

Application of radial basis functions in solving fuzzy integral equations

  • Original Article
  • Published:
Neural Computing and Applications Aims and scope Submit manuscript

Abstract

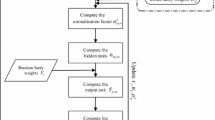
In the present paper, a numerical method based on radial basis functions (RBFs) is proposed to approximate the solution of fuzzy integral equations. By applying RBF in fuzzy integral equation, a linear system \(\Psi C=G \) is obtained. Then target function would be approximated by defining coefficient vector C. Error estimation of the method has been shown which is based on exponential convergence rates of RBFs. Finally, validity of the method is illustrated by some examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Park JY, Kwan YC, Jeong JV (1995) Existence of solutions of fuzzy integral equations in Banach spaces. Fuzzy Sets Syst 72:373–378

    Article  MathSciNet  Google Scholar 

  2. Congxin W, Ming M (1990) On the integrals, series and integral equations of fuzzy set-valued functions. J Harbin Inst Technol 21:11–19

    MATH  Google Scholar 

  3. Zadeh LA (1983) Linguistic variables, approximate reasoning and disposition. Med Inform 8:173–186

    Article  Google Scholar 

  4. Abbasbandy S, Allahviranloo T (2004) Numerical solution of fuzzy differential equation by Runge–Kutta method. Nonlin Stud 11:117–129

    MathSciNet  MATH  Google Scholar 

  5. Babolian E, Sadeghi H, Javadi S (2004) Numerically solution of fuzzy differential equations by Adomian method. Appl Math Comput 149:547–557

    MathSciNet  MATH  Google Scholar 

  6. Bede B (2006) A note on two point boundary value problems associated with non-linear fuzzy differential equations. Fuzzy Sets Syst 157:986–989

    Article  MathSciNet  Google Scholar 

  7. Bede B, Rudas IJ, Bencsik AL (2007) First order linear fuzzy differential equations under generalized differentiability. Inf Sci 177:1648–1662

    Article  MathSciNet  Google Scholar 

  8. Wu C, Ma M (1990) On the integrals, series and integral equations of fuzzy set-valued functions. J Harbin Inst Technol 21:11–19

    MATH  Google Scholar 

  9. Matloka M (1987) On fuzzy integrals. In: Proceedings of 2nd polish Symposium on interval and fuzzy mathematics, Politechnika Poznansk, pp 167–170

  10. Nanda S (1989) On integration of fuzzy mappings. Fuzzy Sets Syst 32:95–101

    Article  MathSciNet  Google Scholar 

  11. Balasubramaniam P, Muthukumar P, Ratnavelu K (2015) Theoretical and practical applications of fuzzy fractional integral sliding mode control for fractional-order dynamical system. Nonlin Dyn 80:249–267

    Article  MathSciNet  Google Scholar 

  12. Dubois D, Prade H (1987) Fuzzy number: an overview. The analysis. CRC Press, Boca Raton, pp 3–39

    MATH  Google Scholar 

  13. Kaleva O (1987) Fuzzy differential equation. Fuzzy Sets Syst 24:301–317

    Article  MathSciNet  Google Scholar 

  14. Congxin W, Ming M (1991) On embedding problem of fuzzy number spaces. Fuzzy Sets Syst 44:33–8

    Article  MathSciNet  Google Scholar 

  15. Friedman M, Ma M, Kandel A (1996) Numerical methods for calculating the fuzzy integral. Fuzzy Sets Syst 83:57–62

    Article  MathSciNet  Google Scholar 

  16. Molabahrami A, Shidfar A, Ghyasi A (2011) An analytical method for solving Fredholm fuzzy integral equations of the second kind. Comput Math Appl 61:2754–2761

    Article  MathSciNet  Google Scholar 

  17. Attari H, Yazdani A (2011) A computational method for fuzzy Volterra–Fredholm integral equations. Fuzzy Inf Eng 2:147–156

    Article  MathSciNet  Google Scholar 

  18. Nayroles B, Touzot G, Villon P (1992) Generalizing the finite element method: diffuse approximation and diffuse elements. Comput Mech 10:307–318

    Article  MathSciNet  Google Scholar 

  19. Shivanian E (2013) Analysis of meshless local radial point interpolation (MLRPI) on a nonlinear partial integro-differential equation arising in population dynamics. Eng Anal Bound Elem 37:1693–1702

    Article  MathSciNet  Google Scholar 

  20. Dehghan M, Ghesmati A (2010) Numerical simulation of two-dimensional sine-gordon solitons via a local weak meshless technique based on the radial point interpolation method (RPIM). Comput Phys Commun 181:772–786

    Article  MathSciNet  Google Scholar 

  21. Buhmann MD (1993) Spectral convergence of multiquadric interpolation. Proc Edinb Math Soc 36:319–333

    Article  MathSciNet  Google Scholar 

  22. Buhmann MD (2003) Radial basis functions. Cambridge University Press, Cambridge

    Book  Google Scholar 

  23. Fasshauer GE, Zhang JG (2007) On choosing optimal shape parameters for RBF approximation. Numer Alg 45:346–68

    Article  MathSciNet  Google Scholar 

  24. Wendland H (2005) Scattered data approximation. Cambridge University Press, Cambridge

    MATH  Google Scholar 

  25. Stefanini L, Sorinia L, Guerraa ML (2006) Parametric representation of fuzzy numbers and application to fuzzy calculus. Fuzzy Sets Syst 157:2423–2455

    Article  MathSciNet  Google Scholar 

  26. Stefanini L, Bede B (2009) Generalized Hukuhara differentiability of interval-valued functions and interval differential equations. Nonlin Anal 71:1311–1328

    Article  Google Scholar 

  27. Nieto JJ, Khastan A, Ivaz K (2009) Numerical solutions of fuzzy differential equations under generalized differentiability. Nonlin Anal Hybrid Syst 3:700–707

    Article  MathSciNet  Google Scholar 

  28. Puri ML, Ralescu D (1986) Fuzzy random variables. J Math Anal Appl 114:409–422

    Article  MathSciNet  Google Scholar 

  29. Diamond P, Kloeden P (1994) Theory and application. Metric spaces of fuzzy sets. World Scientic, Singapore

    MATH  Google Scholar 

  30. Friedman M, Ma M, Kandel A (1999) Numerical solutions of fuzzy differential and integral equations. Fuzzy Sets Syst 106:35–48

    MathSciNet  MATH  Google Scholar 

  31. Friedman M, Ming M, Kandel A (1999) Solutions to fuzzy integral equations with arbitrary kernels. Int J Approx Reason 20:249–262

    MATH  Google Scholar 

  32. Schoenberg IJ (1938) Metric space sand completely monotone functions. Ann Math 39:811–41

    Article  MathSciNet  Google Scholar 

  33. Hansen PC (2007) Regularization tools version 4.0 for Matlab 7.3. Numer Alg 46:189–194

    Article  MathSciNet  Google Scholar 

  34. Salahshour S, Allahviranloo T (2013) Application of fuzzy differential transform method for solving fuzzy Volterra integral equations. Appl Math Model 37:1016–1027

    Article  MathSciNet  Google Scholar 

  35. Malinowski MT (2015) Random fuzzy fractional integral equations—theoretical foundations. Fuzzy Sets Syst 265:39–62

    Article  MathSciNet  Google Scholar 

  36. Rivaz A, Bidgoli TA (2016) Solving fuzzy fractional differential equations by a generalized differential transform method. SeMA J 73:149–170

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Islamic Azad University, Central Tehran Branch, Tehran, 14168-94351, Iran

    Sh. S. Asari & M. Amirfakhrian

  2. Department of Mathematics, National Institute of Technology, Rourkela, Orissa, 769008, India

    S. Chakraverty

Authors
  1. Sh. S. Asari
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. Amirfakhrian
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. S. Chakraverty
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Sh. S. Asari, M. Amirfakhrian or S. Chakraverty.

Ethics declarations

Conflict of interest

All authors declare that they have no conflict of interest.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Asari, S.S., Amirfakhrian, M. & Chakraverty, S. Application of radial basis functions in solving fuzzy integral equations. Neural Comput & Applic 31, 6373–6381 (2019). https://doi.org/10.1007/s00521-018-3459-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00521-018-3459-4

Keywords

Search

Navigation