Fuzzy topological structures via fuzzy graphs and their applications

Abstract

Fuzzy graphs are an individual of application tools in the area of mathematics, which permit the users to define the relative between concepts because the wildlife of fuzziness is satisfactory for any situation. They are helpful to give more exactness and suppleness to the classification as associated with the traditional models. A topological structure is a set model for graphs. The main purpose of this paper is to introduce a new kind of fuzzy topological structures in terms of fuzzy graphs called fuzzy topological graphs due to a class of fuzzy subsets, and some of their properties are investigated. Also, a new procedure to calculate the number of edges in fuzzy graphs will be defined. Further, we consider the concept of a homeomorphic between fuzzy topological graphs as a fuzzy topological property that can be used to prove the isomorphic between fuzzy graphs. Moreover, an algorithm based on the proposed operations that build some fuzzy topological graphs will be presented. Finally, we give a new method to explain the homeomorphic between some fuzzy topological graphs which will be applied in smart cities.

Appendix: Proofs

In this section, we prove the results declared in previous sections.

Proof

(Continue proof Theorem 2) If $\mathcal {C}_1$ and $\mathcal {C}_2$ are two parallel classes, then there exists a bijective fuzzy function F from $\mathcal {C}_1$ to $\mathcal {C}_2$, i.e., ${F}:\mathcal {C}_1 \rightarrow \mathcal {C}_2$, such that ${F}(\mathcal {C}_1)=$ $\mathcal {C}_2$, where $\mathcal {C}_1=$ $\{\mathcal {A}_i: i \in I\}$ and $\mathcal {C}_2=$ $\{{F}(\mathcal {A}_i): i \in I\}$. Then, every vertex $v_{\mathcal {A}_i}$ in $\mathcal {G}_1$, the graph generated by $\mathcal {C}_1$, we have a corresponding vertex $v_{{F}(\mathcal {A}_i)}$ in $\mathcal {G}_2$, the fuzzy graph generated by $C_2$. So $\mu _{R}(v_{\mathcal {A}_i},$ $v_{\mathcal {A}_j})=$ $\mu _{R}(\mathcal {A}_i \cap \mathcal {A}_j)=$ $\mu _{R}({F}(\mathcal {A}_i \cap \mathcal {A}_j))$ $=\mu _{R}({F}(\mathcal {A}_i)$ $\cap {F}(\mathcal {A}_j))$ $=\mu _{R}(v_{{F}(\mathcal {A}_i)},$ $v_{{F}(\mathcal {A}_j)})$. Also, $\sigma _{v_{\mathcal {A}_i}}=$ $\sigma _{v_{{F}(\mathcal {A}_i)}}$ and $\sigma _{v_{\mathcal {A}_j}}=$ $\sigma _{v_{{F}(\mathcal {A}_j)}}$. Thus, F is an isomorphism. $\square$

Fig. 17

Relationship between fuzzy sets

Proof

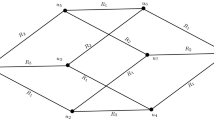
(Continue proof Theorem 3) The relation between fuzzy sets is shown in Fig. 17.

• (i) Let $A \subseteq X$, $|A| = m$ and $|A^c| = n-m$

  $$\begin{aligned} deg\left( V_A\right)= & {} \sum R\left( V_A,V_B\right) \\&+\sum R\left( V_A,V_E\right) \\&+\sum R\left( V_A,V_L\right) \\&+\sum R\left( V_A,V_K\right) \\= & {} \sum |A \cap B|+\sum |A \cap E|\\&+\sum |(A \cap L|+\sum |A \cap K|\\= & {} \sum |B|+\sum |A|+\sum |A \cap L|.\\ \sum |B|= & {} \sum _{i=1}^{m-1} i ^mC_i\\= & {} \sum _{i=1}^{m-1} i\frac{m!}{i!(m-i)!}\\= & {} m\sum _{i=1}^{m-1} \frac{m-1)!}{(i-1)!(m-i)!}\\= & {} m\sum _{i=1}^{m-1} {}^{m-1}C_{i-1}\\= & {} m\sum _{j=0}^{m-2} \left( {}^{m-1}C_j-1\right) \\= & {} m(2^{m-1}-1), \end{aligned}$$

  since $B \subseteq A$, then$\sum |A|$ = $m(2^{m-1} - 1 )$. But $A \subseteq E$, then by complement $\sum |A|$=$m(2^{n-m} - 1)$ and $\sum |A \cap L|$=$(2^{n-m} - 1)\sum _{i=1}^{m-1} i$ $^mC_i$ $=m(2^{n-m} - 1 )(2^{m-1} -1 )$. So $deg(V_A)$ = $m(2^{n-1} - 1 ) + 2m$. It follows that the number of edges of pseudographs G equals

  $$\begin{aligned} |E_p(G)|= & {} \frac{1}{2}\sum _{m=1}^{n}{}^nC_mdeg(V_A)\\= & {} \frac{1}{2}\sum _{m=1}^{n}{}^nC_{m}\left( m\left( 2^{n-1} -1\right) +2m\right) \\= & {} \frac{1}{2}\sum _{m=1}^{n}{}^nC_{m} m\left( 2^{n-1} -1\right) \\&+\frac{1}{2} \sum _{m=1}^{n}{}^nC_m 2m\\= & {} n2^{n-2}\left( 2^{n-1}-1\right) \\&+\sum _{m=1}^{n}m{}^nC_m\\= & {} n2^{n-2}\left( 2^{n-1}-1\right) \\&+n\sum _{m=1}^{n}{}^{n-1}C_{m-1}\\= & {} n2^{n-2}\left( 2^{n-1}-1\right) \\&+n\sum _{j=0}^{n-1}{}^{n-1}C_j\\= & {} n2^{n-2}\left( 2^{n-1} -1\right) +n2^{n-1}. \end{aligned}$$

• (ii) $deg(V_A)$ = $m(2^{n-1} - 1 )$.

  It follows that the number of edges of discrete graphs equals

  $$\begin{aligned} |E_d(G)|= & {} \frac{1}{2} \sum _A deg(V_A)\\= & {} \frac{1}{2} \sum _A {}^nC_m m.\left( 2^{n-1}-1\right) \\= & {} \frac{1}{2}\left( 2^{n-1} -1\right) \sum _{m=1}^{n}m {}^nC_m\\= & {} \frac{1}{2}\left( 2^{n-1} -1\right) \sum _{m=1}^{n}m {}^nC_m\\= & {} \frac{1}{2}\left( 2^{n-1}-1 \right) \sum _{m=1}^{n} m\frac{n!}{m!(n-m)!}\\= & {} \frac{n}{2}\left( 2^{n-1}-1\right) \sum _{m=1}^{n} \frac{(n-1)!}{(m-1)!(n-m)!)}\\= & {} \frac{n}{2} \left( 2^{n-1}-1\right) \sum _{m=1}^{n} {}^{n-1}C_{m-1}\\= & {} \frac{n}{2}\left( 2^{n-1}-1\right) \sum _{j=0}^{n-1} {}^{n-1}C_j\\= & {} \frac{n}{2} \left( 2^{n-1}-1\right) 2^{n-1}\\= & {} n2^{n-2}\left( 2^{n-1}-1\right) . \end{aligned}$$

• (iii) $deg (V_A) = 2^n - 2^{n-m}- 1$.

  It follows that the number of edges of simple graphs G equals

  $$\begin{aligned} |E_s(G)|= & {} \frac{n}{2} \sum _{m=1}^{n} {}^nC_m deg \left( V_A\right) \\= & {} \frac{1}{2}\sum _{m=1}^{n} {}^nC_m \left( 2^n-2^{n-m}-1\right) \\= & {} \frac{1}{2}\left( 2^n-1\right) \sum _{m=1}^{n} {}^nC_m\\&-\frac{1}{2}2^n \sum _{m=1}^{n} {}^nC_m 2^{-m}\\= & {} \frac{1}{2}\left( 2^n-1\right) \left( 2^{n-1}-1\right) \\&- 2^{n-1}\sum _{m=1}^{n}\left( \frac{1}{2}\right) ^m {}^nC_m\\= & {} \frac{1}{2}\left( 2^{2n}-2^{n+1}+1\right) \\&-2^{n-1}\left( \left( 1+\frac{1}{2}\right) ^n-1\right) \\= & {} \frac{1}{2}\left( \left( 2^{2n}-2.2^n +1\right) -\left( 3^n-2^n\right) \right) \\= & {} \frac{1}{2}(2^{2n}-2^n-3^n+1). \end{aligned}$$

The total degree of edges of fuzzy topological graphs can be calculated for the three different types of fuzzy graphs as follows:

[For fuzzy pseudographs:] Let $\mathcal {B} \le \mathcal {A} \le \mathcal {E} \le X$, $|\mathcal {A}| = m$ and $|\mathcal {A}^c|= n-m$

$$\begin{aligned} deg\left( V_{\mathcal {A}}\right)= & {} \sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {B}}\right) \\&+ 2\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {A}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {E}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {L}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {K}}\right) \\= & {} \sum \mu _{R}\left( V_{\mathcal {A}} \cap V_{\mathcal {B}}\right) \\&+ 2\sum \mu _{R}\left( V_{\mathcal {A}} \cap V_{\mathcal {A}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}}\cap V_{\mathcal {E}}\right) \\&+\mu _{R}\left( V_{\mathcal {A}}\cap V_{\mathcal {L}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}} \cap V_{\mathcal {K}}\right) \\= & {} \sum \mu _{R}\left( V_{\mathcal {B}}\right) \\&+ 2\sum \mu _{R}\left( V_{\mathcal {A}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}}\cap V_{\mathcal {L}}\right) \\= & {} \sum \limits _{i=1}^{|B|}\mu _{R}\left( V_{\mathcal {B}}\right) \\&+ 2\sum \limits _{i=1}^{|A|}\mu _{R}\left( V_{\mathcal {A}}\right) \\&+\sum \limits _{i=1}^{|A \cap E|}\mu _{R}\left( V_{\mathcal {A}}\right) \\&+\sum \limits _{i=1}^{|A \cap L|}\mu _{R}\left( V_{\mathcal {A\cap L}}\right) . \end{aligned}$$

$\forall x\in \mathcal {B}, y \in \mathcal {A}, w \in \mathcal {E}$ and $z \in \mathcal {L}$ we have,

$$\begin{aligned} deg\left( V_{\mathcal {A}}\right)= & {} \sum \limits _{i=1}^{|B|}\mu _{R}(xy)+ 2\sum \limits _{i=1}^{|A|}\mu _{R}(yy)\\&+\sum \limits _{i=1}^{|A \cap E|}\mu _{R}(yw)+\sum \limits _{i=1}^{|A \cap L|}\mu _{R}(yz). \end{aligned}$$

It follows that the total degree of edges of fuzzy pseudographs $\mathcal {G}$ equals

$$\begin{aligned} \sum \mathcal {E}_p(\mathcal {G})= \sum \limits _{i=1}^{|V(\mathcal {G})|}deg{(V_i)}. \end{aligned}$$

[For fuzzy discrete graphs:]

$$\begin{aligned} deg\left( V_{\mathcal {A}}\right)= & {} \sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {B}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {E}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {L}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {K}}\right) \\= & {} \sum \mu _{R}\left( V_{\mathcal {A}} \cap V_{\mathcal {B}}\right) \\&+ \sum \mu _{R}\left( V_{\mathcal {A}}\cap V_{\mathcal {E}}\right) \\&+\mu _{R}\left( V_{\mathcal {A}}\cap V_{\mathcal {L}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}} \cap V_{\mathcal {K}}\right) \\= & {} \sum \mu _{R}\left( V_{\mathcal {B}}\right) \\&+ \sum \mu _{R}\left( V_{\mathcal {A}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}}\cap V_{\mathcal {L}}\right) \\= & {} \sum \limits _{i=1}^{|B|}\mu _{R}\left( V_{\mathcal {B}}\right) \\&+\sum \limits _{i=1}^{|A \cap E|}\mu _{R}\left( V_{\mathcal {A}}\right) \\&+\sum \limits _{i=1}^{|A \cap L|}\mu _{R}\left( V_{\mathcal {A\cap L}}\right) . \end{aligned}$$

$\forall x\in \mathcal {B}, y \in \mathcal {A}, w \in \mathcal {E}$ and $z \in \mathcal {L}$ we have,

$$\begin{aligned} deg\left( V_{\mathcal {A}}\right)= & {} \sum \limits _{i=1}^{|B|}\mu _{R}(xy)+ \sum \limits _{i=1}^{|A \cap E|}\mu _{R}(yw)\\&+\sum \limits _{i=1}^{|A \cap L|}\mu _{R}(yz) \end{aligned}$$

It follows that the summation of edges of fuzzy discrete graphs $\mathcal {G}$ equals

$$\begin{aligned} \sum \mathcal {E}_d\left( \mathcal {G}\right) =\sum \limits _{i=1}^{|V(\mathcal {G})|}deg{\left( V_i\right) } \end{aligned}$$

[For fuzzy simple graphs:] Let the number of the fuzzy subset of length $|B|=k$, $|A\cap E|=l$, and $|A \cap L|=r$. Then, we have

$$\begin{aligned} deg\left( V_{\mathcal {A}}\right)= & {} \sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {B}}\right) +\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {E}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {L}}\right) +\sum \mu _{R}\left( V_{\mathcal {A}},V_{\mathcal {K}}\right) \\= & {} \sum \mu _{R}\left( V_{\mathcal {A}} \cap V_{\mathcal {B}}\right) + \sum \mu _{R}\left( V_{\mathcal {A}}\cap V_{\mathcal {E}}\right) \\&+\mu _{R}(V_{\mathcal {A}}\cap V_{\mathcal {L}}) +\sum \mu _{R}\left( V_{\mathcal {A}} \cap V_{\mathcal {K}}\right) \\= & {} \sum \mu _{R}\left( V_{\mathcal {B}}\right) + \sum \mu _{R}\left( V_{\mathcal {A}}\right) \\&+\sum \mu _{R}\left( V_{\mathcal {A}}\cap V_{\mathcal {L}}\right) \\= & {} \sum \limits _{i=1}^{k}\mu _{R}\left( V_{\mathcal {B}}\right) +\sum \limits _{i=1}^{l}\mu _{R}\left( V_{\mathcal {A}}\right) \\&+\sum \limits _{i=1}^{r}\mu _{R}\left( V_{\mathcal {A\cap L}}\right) . \end{aligned}$$

$\square$

Proof

(Continue proof Theorem 4) Let $\mathcal {G}$ be a fuzzy graph. To complete the proof, it is sufficient to prove three conditions of a fuzzy topology on $\tau$.

• (i) By Definitions 3 and 7, $\mathcal {G}$ can be represented by different classes. Suppose that the graph number of $\mathcal {G}$ is m. Then, there exists a class, say $\tau$, such that $\tau \le P(\bigg \{\displaystyle \frac{a}{x_1},$ $\frac{b}{x_2},$ $\frac{c}{x_3}, \ldots , \frac{d}{x_m}\bigg \})$ and represents $\mathcal {G}$. Each $\mathcal {A}_i \in {\tau }$ represents $v_i$ and $X \in {\tau }$ represents the set of vertices $V(\mathcal {G})$. So, by Definition 6, $\mu _{R}(v_i,V(\mathcal {G}))=$ $\mu _{R}(v_i,X)$. If $N= \bigg \{\displaystyle \frac{a}{x_1},$ $\frac{b}{x_2},$ $\frac{c}{x_3}, \ldots , \frac{d}{x_m}\bigg \}$, then $\mu _{R}(\mathcal {A} \cap X)=$ $\mu _{R}(\mathcal {A}\cap {\mathbb {N}})=$ $\mu _{R}(\mathcal {A})$. This means that each $\mathcal {A} \in {\tau }$ satisfies that $\mathcal {A} \le X$. Since every vertex in $\mathcal {G}$ is a fuzzy graph subset of $V(\mathcal {G})$, i.e., for every $v_i \in V(\mathcal {G})$ the singleton $\{v_i\} \subset V(\mathcal {G})$ and each element in ${\tau }$ is a fuzzy subset from $X \in {\tau }$, then $X= \bigg \{\displaystyle \frac{a}{x_1}, \frac{b}{x_2},\frac{c}{x_3}, \ldots , \frac{d}{x_m}\bigg \}$. Also, the isolated vertex $v_0$ can be represented by $0 \in {\tau }$.

• (ii) Let $v_1, v_2, \ldots$ be an arbitrary different vertices in $V(\mathcal {G})$ represented by $\mathcal {A}_{v_1} ,\mathcal {A}_{v_2}, \ldots$. Since $v_1 \vee v_2 \vee \cdots = v_{({\mathcal {A}_{v_1} \vee \mathcal {A}_{v_2} \vee \cdots })}$, then $\mathcal {A}_{v_1} \vee \mathcal {A}_{v_2} \vee \cdots \in {\tau }$.

• (iii) If $v_i$ and $v_j$ are two different vertices in $V(\mathcal {G})$ and represented by $\mathcal {A}_i$ and $\mathcal {A}_j$, respectively. Since $v_i \wedge v_j=$ $v_{{\mathcal {A}_i} \wedge {\mathcal {A}_j}}$, then $\mathcal {A}_i \wedge \mathcal {A}_j \in {\tau }$. Therefore, ${\tau }$ is a fuzzy topology. $\square$

Proof

(Continue proof Theorem 5) Let $\mathcal {A}_m=$ $\{\frac{a}{x_1},$ $\frac{b}{x_2},\ldots ,\frac{c}{x_m}\}$, $1\le k\le m$, $k< m < n$ and $B_k\subseteq A_m \subseteq C_n$

$$\begin{aligned} deg V_{\mathcal {A}_m}= & {} \sum \mu _{R}\left( B_k,A_m\right) + \sum \mu _{R}\left( A_m,C_n\right) \\= & {} \sum \mu _{R}\left( B_k\cap A_m\right) +\sum \mu _{R}\left( A_m\cap C_n\right) \\= & {} \sum \limits _{i=1}^{|B_k \cap A_m|} \mu _{R}\left( B_k\right) +\sum \limits _{i=1}^{|A_m \cap C_n|} \mu _{R}\left( A_m,C_n\right) \\= & {} \sum \limits _{i=1}^{|B_k \cap A_m|} \mu _{R}(xy) +\sum \limits _{i=1}^{|A_m \cap C_n|} \mu _{R}(yz). \end{aligned}$$

$\forall x \in B_k, y \in A_m$ and $z \in C_n$. The total degree of edges is $\sum E(\mathcal {G})= \frac{1}{2}\sum \limits _{i=1}^n deg V_{\mathcal {A}_i}$. $\square$