Skip to main content
SpringerLink
Log in

Nerve Complexes of Circular Arcs

  • Published:
Discrete & Computational Geometry Aims and scope Submit manuscript

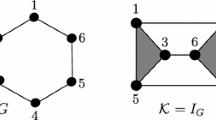
Abstract

We show that the nerve and clique complexes of n arcs in the circle are homotopy equivalent to either a point, an odd-dimensional sphere, or a wedge sum of spheres of the same even dimension. Moreover this homotopy type can be computed in time \(O(n\log n)\). For the particular case of the nerve complex of evenly-spaced arcs of the same length, we determine explicit homology bases and we relate the complex to a cyclic polytope with n vertices. We give three applications of our knowledge of the homotopy types of nerve complexes of circular arcs. First, we show that the Lovász bound on the chromatic number of a circular complete graph is either sharp or off by one. Second, we use the connection to cyclic polytopes to give a novel topological proof of a known upper bound on the distance between successive roots of a homogeneous trigonometric polynomial. Third, we show that the Vietoris–Rips or ambient Čech simplicial complex of n points in the circle is homotopy equivalent to either a point, an odd-dimensional sphere, or a wedge sum of spheres of the same even dimension, and furthermore this homotopy type can be computed in time \(O(n\log n)\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Notes

  1. Attali and Lieutier [4] refer to the ambient Čech complex as a restricted Čech complex.

References

  1. Adamaszek, M.: Clique complexes and graph powers. Isr. J. Math. 196(1), 295–319 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  2. Adamaszek, M., Adams, H.: The Vietoris–Rips complex of the circle. Preprint, arXiv:1503.03669

  3. Adamaszek, M., Adams, H., Motta, F.: Random cyclic dynamical systems. Preprint, arXiv:1511.07832

  4. Attali, D., Lieutier, A.: Geometry driven collapses for converting a Čech complex into a triangulation of a shape. Discrete Comput. Geom. 54(4), 798–825 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  5. Attali, D., Lieutier, A., Salinas, D.: Vietoris–Rips complexes also provide topologically correct reconstructions of sampled shapes. Comput. Geom. 46(4), 448–465 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  6. Babenko, A.G.: An extremal problem for polynomials. Math. Notes 35(3), 181–186 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  7. Babson, E., Kozlov, D.N.: Complexes of graph homomorphisms. Isr. J. Math. 152(1), 285–312 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  8. Bagchi, B., Datta, B.: Minimal triangulations of sphere bundles over the circle. J. Comb. Theory, Ser. A 115(5), 737–752 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  9. Barmak, J.A.: On Quillen’s Theorem A for posets. J. Comb. Theory, Ser. A 118(8), 2445–2453 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  10. Barmak, J.A., Minian, E.G.: Strong homotopy types, nerves and collapses. Discrete Comput. Geom. 47(2), 301–328 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  11. Björner, A.: Topological Methods. Handbook of Combinatorics, vol. 2. Elsevier, Amsterdam (1995)

    Google Scholar 

  12. Borsuk, K.: On the imbedding of systems of compacta in simplicial complexes. Fundam. Math. 35(1), 217–234 (1948)

    MathSciNet  MATH  Google Scholar 

  13. Carlsson, G.: Topology and data. Bull. Am. Math. Soc. 46(2), 255–308 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  14. Chazal, F., de Silva, V., Oudot, S.: Persistence stability for geometric complexes. Geom. Dedicata 173(1), 193–214 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  15. Chazal, F., Oudot, S.: Towards persistence-based reconstruction in Euclidean spaces. In: Proceedings of the 24th Annual Symposium on Computational Geometry, pp. 232–241. ACM, New York (2008)

  16. Colin de Verdière, É., Ginot, G., Goaoc, X.: Multinerves and Helly numbers of acyclic families. In: Proceedings of the 28th Annual Symposium on Computational Geometry, pp. 209–218. ACM, New York (2012)

  17. Edelsbrunner, H., Harer, J.L.: Computational Topology: An Introduction. American Mathematical Society, Providence (2010)

    MATH  Google Scholar 

  18. Gale, D.: Neighborly and cyclic polytopes. Proc. Symp. Pure Math. 7, 225–232 (1963)

    Article  MathSciNet  MATH  Google Scholar 

  19. Gilbert, A.D., Smyth, C.J.: Zero-mean cosine polynomials which are non-negative for as long as possible. J. Lond. Math. Soc. 62(2), 489–504 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  20. Golumbic, M.C., Hammer, P.L.: Stability in circular arc graphs. J. Algorithms 9(3), 314–320 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  21. Hatcher, A.: Algebraic Topology. Cambridge University Press, Cambridge (2002)

    MATH  Google Scholar 

  22. Hell, P., Nešetřil, J.: Graphs and Homomorphisms. Oxford University Press, Oxford (2004)

    Book  MATH  Google Scholar 

  23. Kozlov, D.N.: Combinatorial Algebraic Topology. Algorithms and Computation in Mathematics, vol. 21. Springer, Berlin (2008)

    MATH  Google Scholar 

  24. Kozma, G., Oravecz, F.: On the gaps between zeros of trigonometric polynomials. Real Anal. Exch. 28(2), 447–454 (2002)

    MathSciNet  MATH  Google Scholar 

  25. Kühnel, W.: Higherdimensional analogues of Császár’s torus. Result. Math. 9, 95–106 (1986)

    Article  MATH  Google Scholar 

  26. Kühnel, W., Lassmann, G.: Permuted difference cycles and triangulated sphere bundles. Discrete Math. 162(1–3), 215–227 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  27. Latschev, J.: Vietoris-Rips complexes of metric spaces near a closed Riemannian manifold. Arch. Math. 77(6), 522–528 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  28. Lovász, L.: Kneser’s conjecture, chromatic number, and homotopy. J. Comb. Theory, Ser. A 25(3), 319–324 (1978)

    Article  MATH  Google Scholar 

  29. Matoušek, J.: LC reductions yield isomorphic simplicial complexes. Contrib. Discrete Math. 3(2), 37–39 (2008)

    MathSciNet  MATH  Google Scholar 

  30. Montgomery, H.L., Ulrike, M.A.: Biased trigonometric polynomials. Am. Math. Mon. 114(9), 804–809 (2007)

    MathSciNet  MATH  Google Scholar 

  31. Previte-Johnson, C.: The \(D\)-Neighborhood Complex of a Graph. PhD thesis, Colorado State University, Fort Collins (2014)

  32. Taylan, D.: Matching trees for simplicial complexes and homotopy type of devoid complexes of graphs. Order (2015). doi:10.1007/s11083-015-9379-3

  33. Ziegler, G.M.: Lectures on Polytopes. Graduate Texts in Mathematics, vol. 152. Springer, Berlin (1995)

    MATH  Google Scholar 

Download references

Acknowledgments

We would like to thank Anton Dochtermann for encouraging us to consider the connection to the Lovász bound in Sect. 6, and we would like to thank Arnau Padrol and Yuliy Baryshnikov for helpful conversations about cyclic polytopes. We are grateful to the referees for suggestions regarding the paper, and in particular for bringing [30] to our attention. Research of MA was carried out while at the Max Planck Institut für Informatik, Saarbrücken, Germany. Research of HA was supported by the Institute for Mathematics and its Applications with funds provided by the National Science Foundation. FF was supported by the German Science Foundation DFG via the Berlin Mathematical School.

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Copenhagen, Universitetsparken 5, 2100, Copenhagen, Denmark

    Michał Adamaszek

  2. Department of Mathematics, Colorado State University, Fort Collins, CO, 80523, USA

    Henry Adams & Chris Peterson

  3. Department of Mathematics, Cornell University, Ithaca, NY, 14853, USA

    Florian Frick

  4. Department of Mathematics, California State University, San Bernardino, CA, 92407, USA

    Corrine Previte-Johnson

Authors
  1. Michał Adamaszek
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Henry Adams
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Florian Frick
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Chris Peterson
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Corrine Previte-Johnson
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Florian Frick.

Additional information

Editor in Charge: Günter M. Ziegler

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Adamaszek, M., Adams, H., Frick, F. et al. Nerve Complexes of Circular Arcs. Discrete Comput Geom 56, 251–273 (2016). https://doi.org/10.1007/s00454-016-9803-5

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00454-016-9803-5

Keywords

Mathematics Subject Classification

Search

Navigation