Vortex dynamics in the wake of a pivoted cylinder undergoing vortex-induced vibrations with elliptic trajectories

Abstract

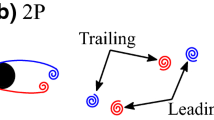
Vortex-induced vibrations of a pivoted cylinder are investigated experimentally at a fixed Reynolds number of 3100, a mass ratio of 10.8, and a range of reduced velocities, $4.42 \le U^* \le 9.05$. For these conditions, the cylinder traces elliptic trajectories, with the experimental conditions producing three out of four possible combinations of orbiting direction and primary axis alignment relative to the incoming flow. The study focuses on the quantitative analysis of wake topology and its relation to this type of structural response. Velocity fields were measured using time-resolved, two-component particle image velocimetry (TR-PIV). These results show that phase-averaged wake topology generally agrees with the Morse and Williamson (J Fluids Struct 25(4):697–712, 2009) shedding map for one-degree-of-freedom vortex-induced vibrations, with 2S, $2{\hbox {P}}_{\mathrm{o}}$, and 2P shedding patterns observed within the range of reduced velocities studied here. Vortex tracking and vortex strength quantification are used to analyze the vortex shedding process and how it relates to cylinder response. In the case of 2S vortex shedding, vortices are shed when the cylinder is approaching the maximum transverse displacement and reaches the streamwise equilibrium. 2P vortices are shed approximately half a period earlier in the cylinder’s elliptic trajectory. Leading vortices shed immediately after the peak in transverse oscillation and trailing vortices shed near the equilibrium of transverse oscillation. The orientation and direction of the cylinder’s elliptic trajectory are shown to influence the timing of vortex shedding, inducing changes in the 2P wake topology.