Entanglement swapping and teleportation using Mach–Zehnder interferometer assisted with a cross-Kerr cell: generation of tripartite entangled state

Abstract

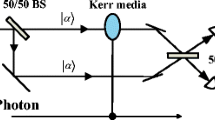
In this paper, we outline new implementations for entanglement swapping and quantum teleportation using the Mach–Zehnder interferometer, where an external mode is coupled to an internal mode of the interferometer through a nonlinear cross-Kerr cell in the absence of losses and noises. The initial state of the total system contains two distinctly atom–field entangled states $((AF)_{1,2})$, each previously generated via the Jaynes–Cummings model, besides an ancillary a-mode as the external mode of the Mach–Zehnder interferometer. Injecting the two-field states and a-mode into the Mach–Zehnder interferometer and then detecting both fields, the subset including the a-mode and the two atoms forms a tripartite entangled state. Therefore, entanglement swapping from $(AF)_{1,2}$ to the subsystem of two atoms and a-mode is appropriately performed. Next, we calculate success probability and fidelity. It is demonstrated that the maximum values of fidelity is achieved for the intensities of coherent field larger than 2. Finally, we show that the Mach–Zehnder interferometer may be used to teleport an entangled state with complete fidelity, by applying a quantum channel with an unknown state. The complete fidelity can be obtained by assuming that the dissipative factors are ignorable in the applied setups.

Appendix A: Calculation of the output state of the Mach–Zehnder interferometer for the input state \(\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\,\left| {\alpha }\right\rangle _a\)

As is stated in Sect. 4, the total state which is injected into the Mach–Zehnder interferometer, is a combination of the field states ${F}_1$, ${F}_2$ and ${F}_a$ indicated as $\,\left| {m}\right\rangle _1\,\left| {n}\right\rangle _2\,\left| {\alpha }\right\rangle _a$ in (9). Here, we obtain the output state of the interferometer, in detail, in which the input state is $\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\,\left| {\alpha }\right\rangle _a$. If the state $\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\,\left| {\alpha }\right\rangle _a$ is injected in the interferometer, the input state to $\text {BS}_1$ is $\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2$. Then after passing the $\text {BS}_1$, we have

$$\begin{aligned} \left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\,\left| {\alpha }\right\rangle _a\xrightarrow {\text {BS}_1}\,\left| {\text {out}}\right\rangle _1=\dfrac{1}{\sqrt{2}}\big (i\,\left| {1}\right\rangle _1\,\left| {0}\right\rangle _2+\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\big )\,\left| {\alpha }\right\rangle _a. \end{aligned}$$

(30)

The action of the phase shifter $\theta$ with unitary operator in (1) yields the state³

$$\begin{aligned} \,\left| {\text {out}}\right\rangle _1\xrightarrow {\text {PS}}\,\left| {\text {out}}\right\rangle _2=\dfrac{1}{\sqrt{2}}\big (i\,\left| {1}\right\rangle _1\,\left| {0}\right\rangle _2+\text {e}^{i\theta }\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\big )\,\left| {\alpha }\right\rangle _a. \end{aligned}$$

(31)

Now, we import the above state into the cross-Kerr medium which is described by the unitary operator in (2). Then, the resultant state is as below⁴

$$\begin{aligned} \,\left| {\text {out}}\right\rangle _2\xrightarrow {\text {CK}}\,\left| {\text {out}}\right\rangle _3=\dfrac{1}{\sqrt{2}}\big (i\,\left| {1}\right\rangle _1\,\left| {0}\right\rangle _2\,\left| {\alpha \text {e}^{-iKt}}\right\rangle _a+\text {e}^{i\theta }\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\,\left| {\alpha }\right\rangle _a\big ). \end{aligned}$$

(32)

Choosing $Kt=\pi$ in (32), reduces it to the state

$$\begin{aligned} \,\left| {\text {out}}\right\rangle _3=\dfrac{1}{\sqrt{2}}\big (i\,\left| {1}\right\rangle _1\,\left| {0}\right\rangle _2\,\left| {-\alpha }\right\rangle _a+\text {e}^{i\theta }\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\,\left| {\alpha }\right\rangle _a\big ). \end{aligned}$$

(33)

In the next step, by crossing the state in (33) from the $\text {BS}_2$, the resultant state reads as

$$\begin{aligned} \,\left| {\text {out}}\right\rangle _3\xrightarrow {\text {BS}_2}\,\left| {\text {out}}\right\rangle _4&=\dfrac{1}{2}\big [\big (i\,\left| {1}\right\rangle _1\,\left| {0}\right\rangle _2-\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\big )\,\left| {-\alpha }\right\rangle _a\nonumber \\&\quad +\text {e}^{i\theta }\big (\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2+i\,\left| {1}\right\rangle _1\,\left| {0}\right\rangle _2\big )\,\left| {\alpha }\right\rangle _a\big ]. \end{aligned}$$

(34)

Finally, considering $\theta =\pi$, the output state of the Mach–Zehnder interferometer is simplified to

$$\begin{aligned} \,\left| {\text {out}}\right\rangle _{\text {MZI}_1}=-\dfrac{1}{2}\big [i\,\left| {\widetilde{\psi _o}}\right\rangle _a\,\left| {1}\right\rangle _1\,\left| {0}\right\rangle _2+\,\left| {\widetilde{\psi _e}}\right\rangle _a\,\left| {0}\right\rangle _1\,\left| {1}\right\rangle _2\big ]. \end{aligned}$$

(35)